Whole Numbers And Its Properties

WHOLE NUMBERS
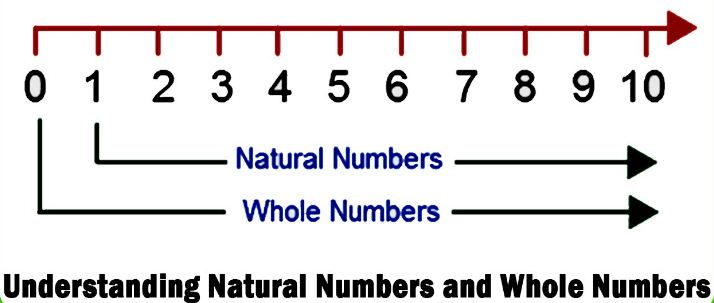
Now if we add zero (0) in the set of natural numbers, we get a new set of numbers called the whole numbers. Hence the set of whole numbers consists of zero and the set of natural numbers. It is denoted by W. i.e., W = {0, 1, 2, 3, . . .}. Smallest whole number is zero.

Read More:

Properties of whole numbers

All the properties of numbers satisfied by natural numbers are also satisfied by whole numbers. Now we shall learn some fundamental properties of numbers satisfied by whole numbers.

Properties of Addition

(a) Closure Property: The sum of two whole numbers is always a whole number. Let a and b be two whole numbers, then a + b = c is also a whole number.
This property is called the closure property of addition

Example: 1 + 5 = 6 is a whole number.

(b) Commutative Property: The sum of two whole numbers remains the same if the order of numbers is changed. Let a and b be two whole numbers, then
a + b = b + a
This property is called the commutative property of addition.

(c) Associative Property: The sum of three whole numbers remains the same even if the grouping is changed. Let a, b, and c be three whole numbers, then
(a + b) + c = a + (b + c)
This property is called the associative property of addition.

(d) Identity Element: If zero is added to any whole number, the sum remains the number itself. As we can see that 0+a=a=a+0 where a is a whole number.
Therefore, the number zero is called the additive identity, as it does not change the value of the number when addition is performed on the number.

Properties of Subtraction

(a) Closure Property: The difference of two whole numbers will not always be a whole number. Let a and b be two whole numbers, then a – b will be a whole number if a > b or a = b. If a < b, then the result will not be a whole number. Hence, closure property does not hold good for subtraction of whole numbers.
Examples
17 – 5 = 12 is a whole number.
5 – 17 = – 12 is not a whole number.

(b) Commutative Property: If a and b are two whole numbers, then a – b ≠ b – a. It shows that subtraction of two whole numbers is not commutative. Hence, commutative property does not hold good for subtraction of whole numbers, i.e.,
a – b ≠ b – a.
Example: 3 – 4 = – 1 and 4 – 3 = 1
∴ 3 – 4 ≠ 4 – 3

(c) Associative Property: If a, b, and c are whole numbers, then (a – b) – c ≠ a – (b – c). It shows that subtraction of whole numbers is not associative. Hence, associative property does not hold good for subtraction of whole numbers.
Example: (40 – 25) – 10 = 15 – 10 = 5
40 – (25 – 10) =40- 15 = 25
∴ (40 – 25) – 10 ≠ 40 – (25 – 10)

(d) Property of Zero: If we subtract zero from any whole number, the result remains the number itself.
Example: 7 – 0 = 7
5 – 0 = 5

Properties of Multiplication

(a) Closure Property: If a and b are two whole numbers, then a × b = c will always be a whole number. Hence, closure property holds good for multiplication of whole numbers.
Example: 5 × 7 = 35 (a whole number)
6 × 1 = 6 (a whole number)

(b) Commutative Property: If a and b are two whole numbers, then the product of two whole numbers remains unchanged if the order of the numbers is interchanged, i.e.,
a × b = b × a.
Example: 6 × 5 = 5 × 6
30 = 30
i. e., 6 rows of 5 or 5 rows of 6 give the same results.
so, 6 × 5 = 30 = 5 × 6

(c) Associative Property: If a, b, and c are whole numbers, then the product of three whole numbers remains unchanged even if they are multiplied in any order. Hence, associative property does hold good for multiplication of whole numbers, i.e.,
(a × b) × c = a × (b × c)
Example:
(4 × 5) × 8 = 4 × (5 × 8)
20 × 8 = 4 × 40
160 = 160

(d) Multiplicative Identity: If any whole number is multiplied by 1, the product remains the number itself. Let a whole number be a, then
a × 1 = a = 1 × a.
3 × 1 = 3 = 1 × 3
Examples
75 × 1 = 75 = 1 × 75
3 × 1 = 3 = 1 × 3
Hence, 1 is called the multiplicative identity.

(e) Multiplicative Property of Zero: Any whole number multiplied by zero gives the product as zero.If a is any whole number, then 0 × a = a × 0 = 0.
Example: 3 × 0 = 0 × 3 = 0

Properties of Division

(a) Closure Property: If a and b are whole numbers, then a ÷ b is not always a whole number. Hence, closure property does not hold good for division of whole numbers.
Example: 7 ÷ 5 = \(\frac { 7 }{ 5 }\) is not a whole number.
7 ÷ 7 = 1 is a whole number.

(b) Commutative Property: If a and b are whole numbers, then a ÷ b ≠ b ÷ a. Hence, commutative property does not hold good for division of whole numbers.
Example: 18 ÷ 3 = 6 is a whole number.
3 ÷ 18 = \(\frac { 3 }{ 18 }\) = \(\frac { 1 }{ 6 }\) is not a whole number.
∴ 3 ÷ 18 ≠ 18 ÷ 3

(c) Associative Property: If a, b, and c are whole numbers then (a ÷ b) ÷ c ≠ a ÷ (b ÷ c). Hence, associative property does not hold good for division of whole numbers.

Example: (15 ÷ 3) ÷ 5 = 5 ÷ 5 = 1
15 ÷ (3 ÷ 5) = 15 ÷ 3/5 = 15 × 5/3
= 25
∴ (15 ÷ 3) ÷ 5 ≠ 15 ÷ (3 ÷ 5)

(d) Property of Zero: If a is a whole number then 0 ÷ a = 0 but a ÷ 0 is undefined.
Example: 6 ÷ 0 is undefined.

Note:

Product of zero and a whole number gives zero.
a × 0 = 0
Zero divided by any whole number gives zero.
0 ÷ a = 0
a ÷ 0 = undefined
Any number divided by 1 is the number itself.
a ÷ 1 = a

DISTRIBUTIVE PROPERTY

You are distributing something as you separate or break it into parts.
Example: Raj distributes 4 boxes of sweets. Each box comprises 6 chocolates and 10 candies. How many sweets are there in these 4 boxes?
∴ Chocolates in 1 box = 6
Chocolates in 4 boxes = 4 × 6 = 24
Candies in 1 box =10
Candies in 4 boxes = 4 × 10 = 40
Total number of sweets in 4 boxes
= 4 × 6 + 4 × 10 = 4 × (6 + 10)
= 4 × 16 = 64

Hence, we conclude the following:
(a) Multiplication distributes over addition, i.e., a(b + c) = ab + ac, where a, b, c are whole numbers.
Example: 10 × (6 + 5) = 10 × 6 + 10 × 5
10 × 11 = 60 + 50
110 = 110
This property is called the distributive property of multiplication over addition.
(b) Similarly, multiplication distributes over subtraction, i.e., a × (b – c) = ab – ac where a, b, c are whole numbers and b > c.
Example: 10 × (6 – 5) = 10 × 6 – 10 × 5
10 × 1 = 60 – 50
10 = 10
This property is called the distributive property of multiplication over subtraction.

Example 1: Determine the following by suitable arrangement.
2 × 17 × 5
Solution: 2 × 17 × 5 = (2 × 5) × 17
= 10 × 17 = 170

Example 2: Solve the following using distributive property.
97 × 101
Solution: 97 × 101 = 97 × (100 + 1)
= 9700 + 97 = 9797

Example 3: Tina gets 78 marks in Mathematics in the half-yearly Examination and 92 marks in the final Examination. Reena gets 92 marks in the half- yearly Examination and 78 marks in the final Examination in Mathematics. Who has got the higher total marks?
Solution: Tina gets the following marks = 78 + 92 = 170 Total marks
Reena gets the following marks = 92 + 78 = 170 Total marks
So, both of them got equal marks.

Example 4: A fruit seller placed 12 bananas, 10 oranges, and 6 apples in a fruit basket. Tarun buys 3 fruit baskets for a function. What is the total number of fruits in these 3 baskets?
Solution: Number of bananas in 3 baskets = 12 × 3 = 36 bananas
Number of oranges in 3 baskets = 10 × 3 = 30 oranges
Number of apples in 3 baskets = 6×3 = 18 apples
Total number of fruits = 36 + 30+ 18 = 84
Alternative Method
Total number of fruits in 3 baskets
= 3 × [ 12 +(10 + 6)]
= 3 × [ 12 + 16]
= 3 × 28 = 84

Representation Of Whole Numbers On A Number Line

We can represent whole numbers-on a straight line. To represent a set of whole numbers on a number line, let’s first draw a straight line and mark a point O on it. After that, mark points A, B, C, D, E, F on the line at equal distance, on the right side of point O.

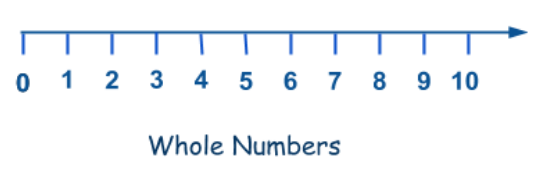
Now, OA = AB = BC = CD and so on
Let OA = 1 unit
OB = OA + AB = 1 + 1 = 2 units
OC = OB + BC = 2 + 1 = 3 units
OD = OC + CD = 3 + 1 = 4 units and so on.
Let the point O correspond to the whole number 0, then points A, B, C, D, E, ….. correspond to the whole numbers 1, 2, 3, 4, 5,…. In this way every whole number can be represented on the number line.