Strategies for Dealing with Similar Triangles
Triangles are similar if their corresponding (matching) angles are congruent (equal in measure) and the ratio of their corresponding sides are in proportion.
There are many different types of problems that involve similar triangles. And, fortunately, there are several different ways to arrive at an answer.
Let’s look at some strategies for arriving at answers!
Style 1: The similar triangles are two separate triangles:
Find x:
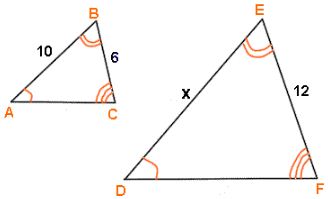
Create a proportion matching the corresponding sides.
Two possible answers:
- Small triangle on top:
10x=612
x = 20 - Large triangle on top:
x10=126
x = 20
HINT: These two triangles are sitting such that their corresponding parts are in the same position in each triangle. If the triangles are not sitting in this manner, you can match the corresponding sides by looking across from the angles which are equal in each triangle.
Style 2: The similar triangles overlap:
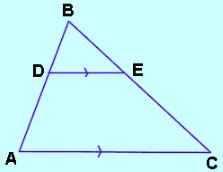
Many problems involving similar triangles have one triangle ON TOP OF (overlapping) another triangle.
Since ¯DE is marked to be parallel to ¯AC, we know that we have ∠BDE congruent to ∠DAC (by corresponding angles). ∠B is shared by both triangles, so the two triangles are similar by AA.
There are two ways to attack this type of problem.
Easiest method to use:
Use FULL sides of the two triangles when dealing with the problem. Do not use DA or EC since they are not sides of triangles.
Easy to forget:
Use a theorem relating to parallel lines, which says that If a line is parallel to one side of a triangle, and intersects the other two sides, the line divides these two sides proportionally.
Let’s try some problems with this type of diagram:
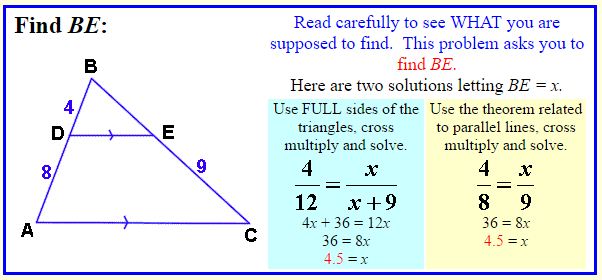
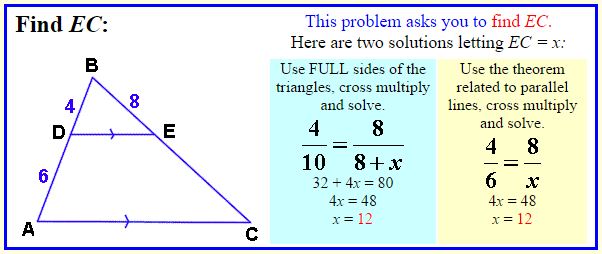
Find x.
This problem MUST use the full sides of triangles as a solution. The parallel theorem does not work here. The problem asks you to find x where x is a FULL side.
Here is the solution:
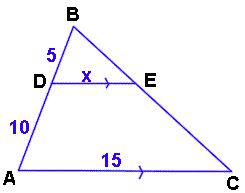
515=x15
x = 5
