Rounding and Error in Measurement
Rounding as associated with Error:
Error in measurement may also be indicated by the terms “rounded” or “to the nearest“. When dealing with measurements that have been rounded, remember what happens when rounding occurs. The basic rule for rounding states:
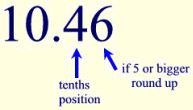
“When rounding to a place value, look at the value immediately to the right of that position. If this value is 5 or bigger, round up.”
(In the example at the right, to the nearest tenth, 10.46 becomes 10.5)
 So, if you know that a measurement to the nearest tenth is 10.5 inches, the actual measurement might be:
So, if you know that a measurement to the nearest tenth is 10.5 inches, the actual measurement might be:
10.46 inches
or 10.53435 inches
or 10.4961 inches
or 10.54 inches,
since all of these values (and others) round to 10.5.
The actual measurement could be anywhere in a range of values that all round to 10.5:
 Notice how the range of the actual answer was formed in the example above.
Notice how the range of the actual answer was formed in the example above.
No value smaller than 10.45 can be rounded to 10.5, so 10.45 is INCLUDED in the range.
The largest value, however, is not 10.49, as you might suspect. What about 10.498? It also rounds to 10.5. Any value up to, but NOT INCLUDING 10.55 can be rounded to 10.5.
The range is found by taking half of the unit to which you are rounding (such as ±0.5, ±0.05, ±0.005). Remember that rounding always looks at one place value BEYOND the rounded location.
Avail the easy to use and straightforward tool Rounding to the Nearest Unit Calculator for all your calculations and make your work easy. You just need to provide the inputs and tap on the calculate button to see the result instantly.
π and the calculator
When working with formulas that involve π, such as:
v=πr2h
remember that using the π key on the calculator will give a different answer than using the approximation of 3.14. Using the π key will give a more accurate answer.
Unless stated otherwise, always use the full calculator entry when dealing with π.
Examples:
- The length of a side of a square to the nearest inch is 10 inches. What is the range of values that will contain the “actual” length of the side of the square?
Answer:
The “actual” length of the side could be in the range:
9.5 inches ≤ actual length < 10.5 inches
(since all values in this range round to 10). - The width of a flower is measured to be 2.5 inches, to the nearest tenth of an inch. Which of the numbers listed cannot be the actual width of the flower?
a) 2.46 inches b) 2.53 inches c) 2.498 inches d) 2.445 inches
Answer:
The “actual” width of the flower must fall in the range:
2.45 inches ≤ actual width < 2.55 inches
(since all values in this range round to 2.5). Choice (d) 2.445 inches does not fall in this range since it rounds to 2.4 inches to the nearest tenth. - The exact dimensions of a rectangular plot of land are listed to be 10.45 m by 32.7 m. The value of property where this land resides is listed at $28.35 per square meter. To the nearest dollar, what is the value of this plot of land?
Answer:
The area of this plot of land is 341.715 square meters. At $28.35 per square meter, the value of this land will be $9687.62025 or $9688 to the nearest dollar.
NOTE: When a problem calls for a rounded answer, be sure to perform all of your calculations first and round ONLY the final answer. If you continually “round” separate calculations within a problem, larger and larger errors will be produced.
Look what happens to this answer when we round too soon:
This problem involved rounding to the nearest integer (nearest dollar). Had the area been rounded to the nearest integer (342 sq. meters) BEFORE the value was computed, the value would have been 342 • $28.35 = $9695.7 rounded to $9696.
Had the dimensions been rounded to the nearest integer BEFORE the calculations were made, the area would have been 10 m by 33 m for 330 square meters. The value, at $28.35 per square meter, would then have been $9356 to the nearest dollar.
If both the dimensions and the cost had been rounded to the nearest integer BEFORE the calculations, the area would have been 330 square meters and the value, at $28 per square meter, would have been $9240.
The more we rounded, the less accurate the answer became. - You measure your bedroom to be 10 feet by 12 feet, rounded to the nearest whole foot. What is the range of the actual area of your room?
Answer:
The “actual” measurements fall within the following ranges:
9.5 feet ≤ actual width < 10.5 feet
11.5 feet ≤ actual length < 12.5 feet
The smallest possible area = 109.25 square feet.
The largest possible area < 131.25 square feet.
109.25 ≤ actual area < 131.25
