Where did the Roman Numerals come from

Where did the Roman Numerals come from

Roman Numbers

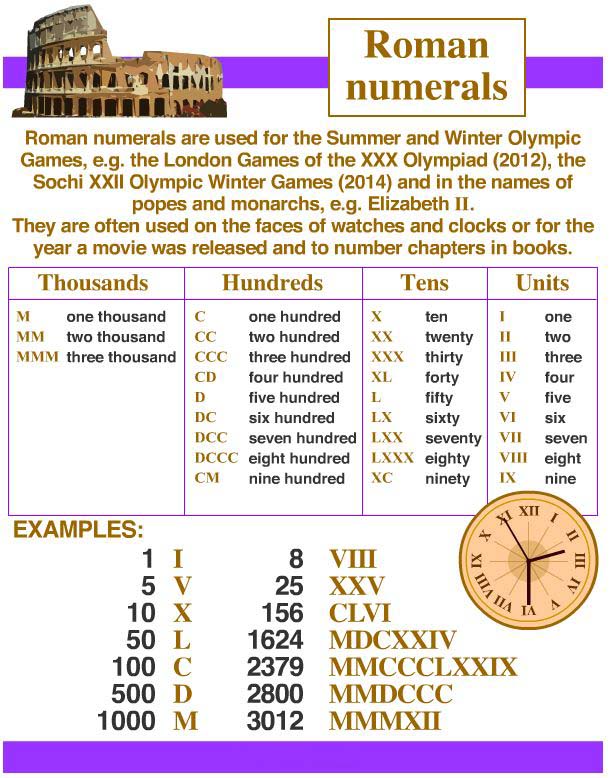We have already learnt about the Indian and the International systems of numeration. There is another numeration system, called the System of Roman Numeration. This is the oldest system of numeration, developed by the Romans and is still in common use. Roman numbers did not have ‘0’ and so they did not have the concept of place values. In this system seven basic symbols are used for writing the numerals. These symbols are represented as shown in the table:

Note:

• K is also used to denote 1000.
• \(\overline { V }\) is used to denote 5000.

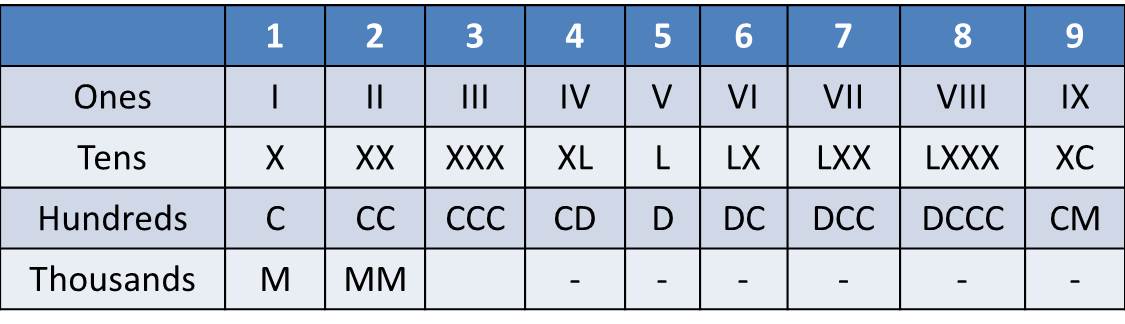
With the help of these symbols, we may write any number in Roman numeral but we need to follow some rules. These rules are:
Rule 1: Putting a symbol ‘after’ a symbol of bigger value means adding the values of the symbols.
LX = 50 + 10 = 60 (Here X is placed after L, and X represents 10 whereas L represents 50, that is, L has a bigger value than X, so we add their values.)
LXXVI =50+10+10 + 5 + 1
= 76

Rule 2: Putting a symbol ‘before’ a symbol of bigger value means subtracting the value of the first symbol from that of the second.
XL = 50 – 10 = 40
(Here, X is placed before L, and X represents 10 whereas L represents 50, that is, L has a bigger value than X, so we subtract the smaller value from the greater one.)
XCV =(100- 10)+ 5 = 95
(Here, X is placed before C and V is placed after C. We subtract the value of X from the value of C and add the value of V to it.)

Rule 3: A symbol can be repeated a maximum of three times in a numeral.
LXXX = 50 + 10 + 10 + 10 = 80 > LIII = 50 + 1 + 1 + 1 = 53

Rule 4: If a symbol of smaller value comes in between two symbols of larger values, then its value is always subtracted from the value of the symbol immediately following it.
LXXIV = 50 + 10 + 10 + (5 – 1)
=74
(Here, 1, the value of I is subtracted from 5, the value of V and not from 10, the value of X because I is followed by V).
XIX = 10 + (10- 1)= 19
XLIX =(50-10)+ (10-1)
= 49

Note:

• I can be subtracted from X and V only.
• X can be subtracted from L and C only.
• C can be subtracted from D and M only.
• V, L, D can never be subtracted.
• A symbol of smaller value can be subtracted from a symbol of greater value just once.

Let us consider some examples.

Example 1: Write the following Roman numerals as Hindu-Arabic numerals:
(a) LXIII     (b) DCCXLVI
Solution:
(a) LXIII = 50 + 10 + 3 = 63
(b) DCCXLVI = 500 + 100 + 100 + (50 – 10) + 5 + 1 = 746

Example 2: Write the Roman numeral for each of the following:
(a) 98     (b) 587     (c) 964
Solution:
(a) 98 = 90 + 8 = (100-10) + 5 + 3
= XCVIII
(ib) 587 =500 + 50+ 10+ 10+ 10 + 5 + 2
= DLXXXVII
(c) 964 =900 + 50 + 10 + 4
= CMLXIV

Example 3: Compare the following, using >, <, =
(a) LX, XL     (b) LVIII, C
Solution:
(a) LX > XL     (b) LVIII < C