Reflection in a Line

Reflection in a Line

A reflection over a line k (notation r_k) is a transformation in which each point of the original figure (pre-image) has an image that is the same distance from the line of reflection as the original point but is on the opposite side of the line. Remember that a reflection is a flip. Under a reflection, the figure does not change size.

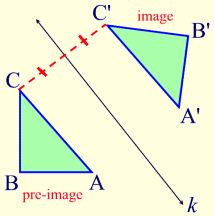
r_k(∆ABC) = ∆A’B’C’

The line of reflection is the perpendicular bisector of the segment joining every point and its image.

A line reflection creates a figure that is congruent to the original figure and is called an isometry (a transformation that preserves length). Since naming (lettering) the figure in a reflection requires changing the order of the letters (such as from clockwise to counterclockwise), a reflection is more specifically called a non-direct or opposite isometry.

Properties preserved (invariant) under a line reflection:

1. distance (lengths of segments are the same)
2. angle measures (remain the same)
3. parallelism (parallel lines remain parallel)
4. colinearity (points stay on the same lines)
5. midpoint (midpoints remain the same)
6. orientation (lettering order NOT preserved. Order is reversed.)

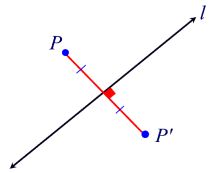
Definition: A reflection is an isometry where if ? is any line and P is any point not on ?, then r_?(P) = P’ where ? is the perpendicular bisector of \(\overline { P{ P }^{ ‘ } }\) and if P∈? then r_?(P) = P.

So what is this definition saying:
Part I (up to the word “and”): Here we see line ? and point P not on line ?. The reflection of point P in this line will be point P’. This is stated by r_?(P)=P’. The line ? will be the perpendicular bisector of the segment joining point P to point P’.
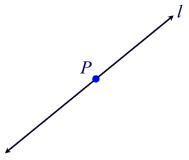
Part II (after the word “and”): The second part of the definition deals with point P being on line ?. P∈? says P is an element of line ?. If P in on the line, then it is its own reflection in line l. This is stated by r_?(P) = P.

Reflections in the Coordinate Plane:

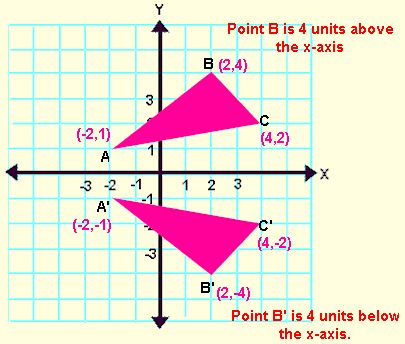
Reflecting over the x-axis: (the x-axis as the line of reflection)
When you reflect a point across the x-axis, the x-coordinate remains the same, but the y-coordinate is transformed into its opposite.
The reflection of the point (x, y) across the x-axis is the point (x, -y). P(x,y)→P'(x,-y) or r_x-axis(x,y) = (x,-y)
Hint: If you forget the rules for reflections when graphing, simply fold your graph paper along the line of reflection (in this example the x-axis) to see where your new figure will be located. Or you can measure how far your points are away from the line of reflection to locate your new image. Such processes will allow you to see what is happening to the coordinates and help you remember the rule.

Reflecting over the y-axis: (the y-axis as the line of reflection)
When you reflect a point across the y-axis, the y-coordinate remains the same, but the x-coordinate is transformed into its opposite.
The reflection of the point (x, y) across the y-axis is the point (-x, y). P(x,y)→P'(-x,y) or r_y-axis(x,y) = (-x,y)
Reflection in a Line 4

Reflecting over the line y = x or y = -x: (the lines y = x or y = -x as the lines of reflection)
When you reflect a point across the line y = x, the x-coordinate and the y-coordinate change places. When you reflect a point across the line y = -x, the x-coordinate and the y-coordinate change places and are negated (the signs are changed).
The reflection of the point (x, y) across the line y = x is the point (y, x). P(x,y)→P'(y,x) or r_y=x(x,y) = (y,x)
The reflection of the point (x, y) across the line y = -x is the point (-y, -x). P(x,y)→P'(-y,-x) or r_y=-x(x,y) = (-y,-x)

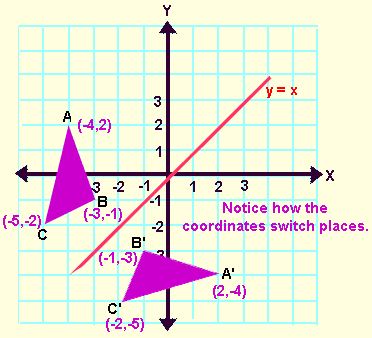
Reflecting over any line:
Each point of a reflected image is the same distance from the line of reflection as the corresponding point of the original figure. In other words, the line of reflection lies directly in the middle between the figure and its image — it is the perpendicular bisector of the segment joining any point to its image. Keep this idea in mind when working with lines of reflections that are neither the x-axis nor the y-axis.
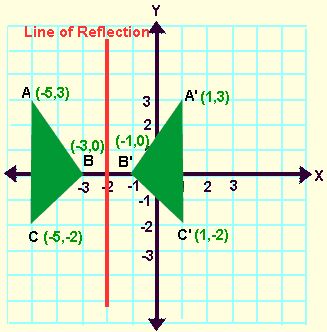Notice how each point of the original figure and its image are the same distance away from the line of reflection (which can be easily counted in this diagram since the line of reflection is vertical).