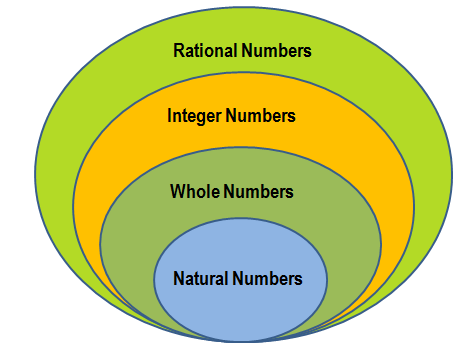
What is a Rational Number?

What is a Rational Number?

A rational number is a number which can be put in the form \(\frac { p }{ q }\), where p and q are both integers and q ≠ 0.
p is called numerator (N^r) and q is called denominator (D^r).

You can also go through https://www.aplustopper.com/ncert-solutions-for-class-10-maths-chapter-1/ for more solved exercises on Rational Numbers

• A rational number is either a terminating or non-terminating but recurring (repeating) decimal.
• A rational number may be positive, negative or zero.
  Examples:
  
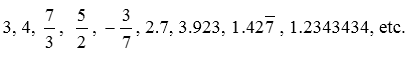
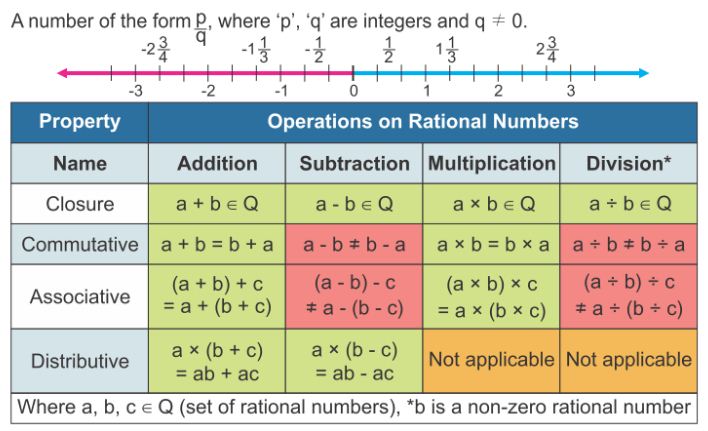
• The sum, difference and the product of two rational numbers is always a rational number.
• The quotient of a division of one rational number by a non-zero rational number is a rational number. Rational numbers satisfy the closure property under addition, subtraction, multiplication and division.

Results
Since every number is divisible by 1, we can say that :

1. Every natural number is a rational number, but every rational number need not be a natural number.
   For example, 3 = \(\frac { 3 }{ 1 }\) , 5 = \(\frac { 5 }{ 1 }\), 9 = \(\frac { 9 }{ 1 }\) and so on.
   but, \(\frac { 7 }{ 9 } ,\frac { 11 }{ 13 } ,\frac { 5 }{ 7 }\) are rational numbers but not natural numbers.
2. Zero is a rational number because \(\left( 0=\frac { 0 }{ 1 } =\frac { 0 }{ 2 } =…. \right)\).
3. Every integer is a rational number, but every rational number may not be an integer.
   For example \(\frac { -2 }{ 1 } ,\frac { -5 }{ 1 } ,\frac { 0 }{ 1 } ,\frac { 3 }{ 1 } ,\frac { 5 }{ 1 }\), etc. are all rationals, but rationals like \(\frac { 3 }{ 2 } ,\frac { -5 }{ 2 }\) etc. are not integers.
4. Rational numbers can be positive and negative.
   
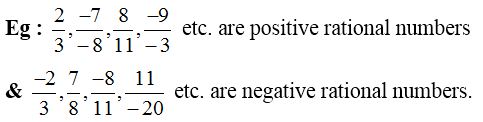
5. Every positive rational number is greater than zero.
6. Every negative rational number is less than zero.
7. Every positive rational number is greater than every negative rational number.
8. Every negative rational number is smaller than every positive rational number.

Equivalent Rational Numbers

∵  Rational no. can be written with different N^r and D^r.

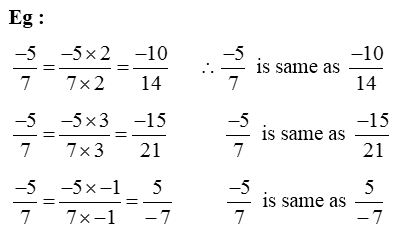
Such rational number that are equal to each other are said to be equivalent to each other.
Example: Write \(\frac { 2 }{ 5 }\) in an equivalent form so that the numerator is equal to –56.
Solution:
Multiplying both the numerator and denominator of \(\frac { 2 }{ 5 }\) by –28, we have

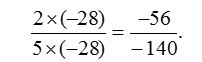
Lowest Form of a Rational Number

A rational number is said to be in lowest form if the numerator and the denominator have no common factor other than 1.

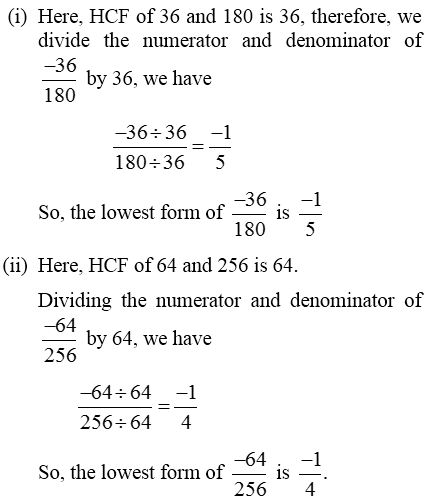
Example: Write the following rational numbers in the lowest form :

Solution:

Standard Form of a Rational Number

A rational number \(\frac { p }{ q }\) is said to be in its standard form if
(i) its denominator ‘q’ is positive
(ii) the numerator and denominator have no common factor other than 1.
For example : \(\frac { 3 }{ 2 } ,\frac { -5 }{ 2 } ,\frac { 1 }{ 7 }\), etc.

Example: Express the rational number \(\frac { 14 }{ -21 }\) in standard form.
Solution:
The given rational number is \(\frac { 14 }{ -21 }\).
1. Its denominator is negative. Multiply both the numerator and denominator by –1 to change it to positive, i.e.,

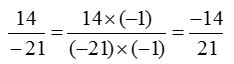
2. The greatest common divisor of 14 and 21 is 7. Dividing both numerator and denominator by 7, we have

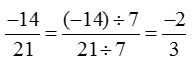
which is the required answer.

Equality of Rational Numbers

Method-1: If two or more rational numbers have the same standard form, we say that the given rational numbers are equal.
Example: Are the rational numbers \(\frac { 8 }{ -12 }\) and \(\frac { -50 }{ 75 }\) equal?
Solution: We first express these given rational numbers in the standard form.
The first rational number is \(\frac { 8 }{ -12 }\).
(i) Multiplying both the numerator and denominator by –1.

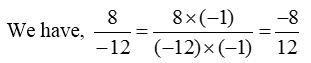
(ii) Dividing both the numerator and denominator by the greatest common divisor of 8 and 12, which is 4.

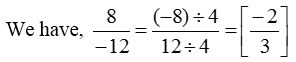
Again, the second rational number is \(\frac { -50 }{ 75 }\).
(i) The denominator is positive.
(ii) Dividing both numerator and denominator by the greatest common divisor of 50 and 75, which is 25.

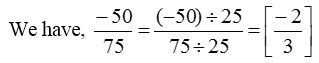
Clearly, both the rational numbers have the same standard form.
Therefore, \(\frac { 8 }{ -12 }\) = \(\frac { -50 }{ 75 }\)

Method-2: In this method, to test the equality of two rational numbers, say \(\frac { a }{ b }\) and \(\frac { c }{ d }\), we use cross multiplication in the following way : \(\frac { a }{ b } =\frac { c }{ d }\)
Then a × d = b × c
If a × d = b × c, we say that the two rational numbers \(\frac { a }{ b }\) and \(\frac { c }{ d }\) are equal.
Example: Check the equality of the rational numbers \(\frac { -7 }{ 21 }\) and \(\frac { 3 }{ -9 }\).
Solution:

Comparison of Rational Numbers

Comparing fraction. We compare two unequal fractions, each is written as another equal fraction so that both have the same denominators. Then the fraction with greater numerator is greater.
Example : To compare \(\frac { 7 }{ 6 }\) and \(\frac { 5 }{ 8 }\), find the L.C.M. of 6 and 8 (it is 24) and


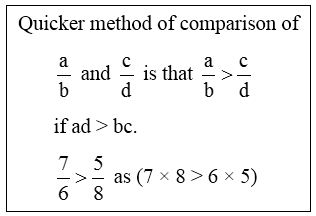
To compare two negative rational numbers, we compare them ignoring their negative signs and then reverse the order.

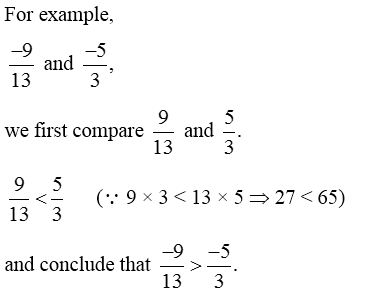
Note :
Every positive rational number is greater than negative rational number.

Representation of Rational Numbers on Number Line

We know that the natural numbers, whole numbers and integers can be represented on a number line. For representing an integer on a number line, we draw a line and choose a point O on it to represent ‘0’.
We can represent this point ‘O’ by any other alphabet also. Then we mark points on the number line at equal distances on both sides of O. Let A, B, C, D be the points on the right hand side and A’, B’, C’, D’ be the points on the left of O as shown in the figure.

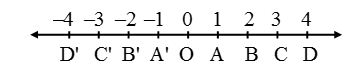
The points on the left side of O, i.e., A’, B’, C’, D’, etc. represent negative integers –1, –2, –3, –4 whereas, points on the right side of O, i.e., A, B, C, D represent positive integers 1, 2, 3, 4 etc. Clearly, the points A and A’ representing the integers 1 and –1 respectively are on opposite sides of O, but at equal distance from O. Same is true for B and B’ ; C and C’ and other points on the number line.
(1) Natural Numbers

(2) Whole Numbers

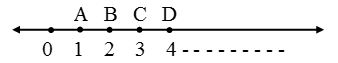
(3) Integers

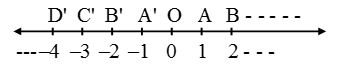
Negative numbers are in left side of zero (0) & positive numbers are in right side.
∵  negative numbers are less than positive numbers
∴  If we move on number line from right to left we are getting smaller numbers.
Also OA = distance of 1 from 0
OD’ = distance of –4 from 0
D’A = distance between –4 and 1. etc.
(4) Rational Numbers
(a) If N^r < D^r:
We divide line segment OA (i.e. distance between 0 & 1) in equal parts as denominator (Dr).

(b) If N^r > D^r:
Example: Represent \(\frac { 13 }{ 3 }\) and \(-\frac { 13 }{ 3 }\) on number line.
Solution:

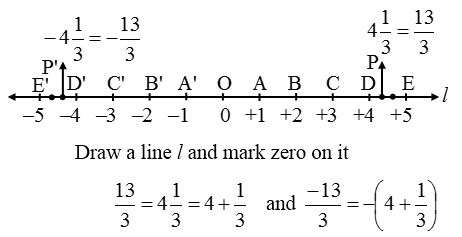
Therefore, from O mark OA, AB, BC, CD and DE to the right of O such that
OA = AB = BC = CD = DE = 1 unit.
Clearly,
Point A,B,C,D,E represents the Rational numbers 1, 2, 3, 4, 5 respectively.
Since we have to consider 4 complete units and a part of the fifth unit, therefore divide the fifth unit DE into 3 equal parts. Take 1 part out of these 3 parts. Then point P is the representation of number \(\frac { 13 }{ 3 }\) on the number line. Similarly, take 4 full unit lengths to the left of 0 and divide the fifth unit D’E’ into 3 equal parts. Take 1 part out of these three equal parts. Thus, P’ represents the rational number \(-\frac { 13 }{ 3 }\).

Rational Number Example Problems With Solutions

Example 1:    Is zero a rational number? can you write it in the form , where p and q are integers and q ≠ 0?
Solution:

Example 2:   Find five rational numbers between  3/5 and 4/5.
Solution:

Example 3:    Find six rational numbers between 3 and 4.
Solution:    

Example 4:    Find two rational & two irrational numbers between 4 and 5.
Solution:

Therefore the 3 rational numbers between 4 & 5 are \(\frac { 9 }{ 2 } \), \(\frac { 19 }{ 2 } \)

The 2 irrational numbers between 4 and 5 are as follows:
a = 4.101001000….
b = 4.20002000….

Maths