Radicals | Simplification, Properties, Addition and Multiplication of Radicals
A radical or the principal nth root of k:
k, the radicand, is a real number.
n, the index, is a positive integer greater than one.
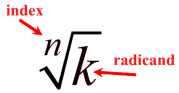
Properties of Radicals:

Simplifying Radicals:
Radicals that are simplified have:
– no fractions left under the radical.
– no perfect power factors in the radicand , k.
– no exponents in the radicand , k, greater than the index, n.
– no radicals appearing in the denominator
of a fractional answer.
Examples: (The following examples demonstrate various solution methods.)
Simplify: Factor the radicand to isolate the perfect power factor(s), which will allow them to be removed from under the radical. You will need to remember your rules for working with exponents in order to isolate the perfect powers.
perfect squares
4, 9, 16, 25, 36, …
x2, x4, x6, x8, …
x2y2, x2y4, 16x6y8, …
powers are “even”
perfect cubes 
8, 27, 64, 125, …
x3, x6, x9, x12, …
x3y3, x3y6, 27x6y9, …
powers are “multiples of 3”

To add radicals
simplify first if possible, and add “like” radicals.

To multiply radicals
![]()

