Prisms
Prisms are three-dimensional closed surfaces.
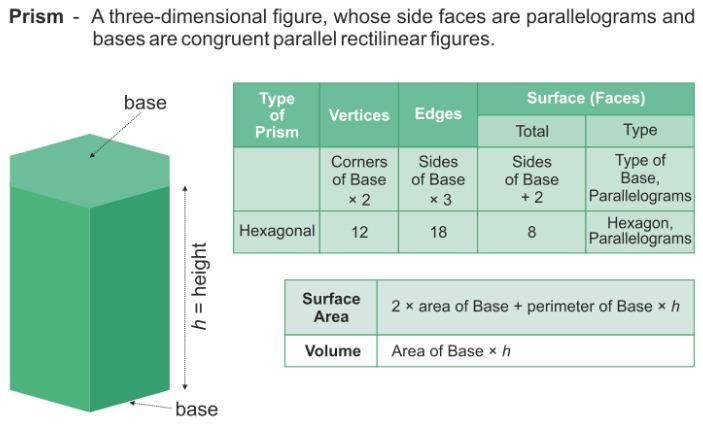
A prism has two parallel faces, called bases, that are congruent polygons. The lateral faces are rectangles in a right prism, or parallelograms in an oblique prism. In a right prism, the joining edges and faces are perpendicular to the base faces.
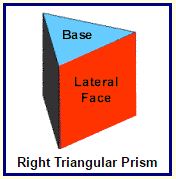Prisms are also called polyhedra since their faces are polygons. A regular prism is a cube.
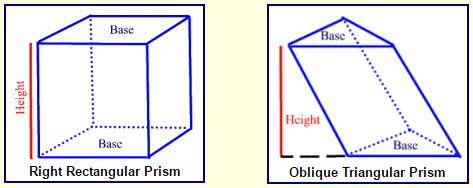
Parallelepiped
A prism which has a parallelogram as its base is called a parallelepiped. It is a polyhedron with 6 faces which are all parallelograms.
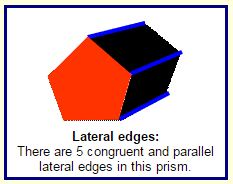
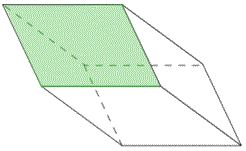The edges of the prism where the lateral faces intersect are called its lateral edges. The lateral edges in a prism are congruent and parallel.
- The volume of a prism is the product of the base area times the height of the prism.
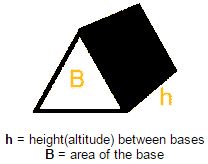V = Bh
(Volume of a prism: B = base area, h = height) - The surface area of a prism is the sum of the areas of the bases plus the areas of the lateral faces. This simply means the sum of the areas of all faces.
A net is a two-dimensional figure that can be cut out and folded up to make a three-dimensional solid.
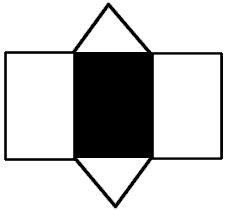
The surface area, S, of a right prism can be found using the formula S = 2B + ph.
B = area of base, p = perimeter of base, h = height.
Note: A cross section of a geometric solid is the intersection of a plane and the solid.
A prism has the same cross section (parallel to the base) all along its length.
Shown here are the cross sections (in the same plane) of two prisms of equal height. The cross section slices are indicated in red and are parallel to the bases.
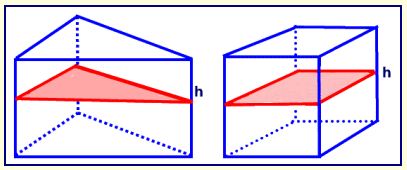If the areas of these two cross section slices are equal, the prisms will be equal in volume.
Seventeenth century mathematician, Bonaventura Cavalieri, generalized this concept for solids.
Cavalieri’s Principle: If, in two solids of equal height, the cross sections made by planes parallel to and at the same distance from their respective bases are always equal, then the volumes of the two solids are equal.
For Algebra 1 you should know a generalized statement of this principle:
“Two prisms will have equal volumes if their bases have equal area and their altitudes (heights) are equal.”
Reflective Prisms
In the study of optics, prisms are used to reflect light, such as occurs in binoculars. Prisms are also used to disperse light, or break light into its spectral colors of the rainbow. The most commonly used optic prism is a triangular prism, which has a triangular base and rectangular sides.
