Plus Two Physics Notes Chapter 5 Magnetism and Matter is part of Plus Two Physics Notes. Here we have given Plus Two Physics Notes Chapter 5 Magnetism and Matter.
| Board | SCERT, Kerala |
| Text Book | NCERT Based |
| Class | Plus Two |
| Subject | Physics Notes |
| Chapter | Chapter 5 |
| Chapter Name | Magnetism and Matter |
| Category | Plus Two Kerala |
Kerala Plus Two Physics Notes Chapter 5 Magnetism and Matter
Introduction
The word magnet is derived from the name of an island in Greece called magnesia where magnetic ore deposits were found.
Properties of a magnet
- When a bar magnet is freely suspended, it points in the north-south direction.
- There is a repulsive force when north poles (or south poles) are brought close together.
- We cannot isolate the north or south pole of a magnet.
- It is possible to make magnets out of iron and its alloys.
Note: The earth behaves as a magnet with the magnetic field pointing approximately from the geographic south to the north.
The Bar Magnet
A magnet has two poles. One pole is North pole and the other South pole.
Magnetic poles:
These two points near the ends of a magnet at which the power of attraction of the magnet is mostly concentrated are called its magnetic poles.
Note: A current carrying solenoid behaves like a bar magnet.
1. The magnetic field lines:
Properties of magnetic field lines
- The magnetic field lines of a magnet form continuous closed loops.
- The tangent to the field line at a given point represents the direction of magnetic field at that point.
- Flux density of magnetic field represents the strength of magnetic field.
- The magnetic field lines do not intersect
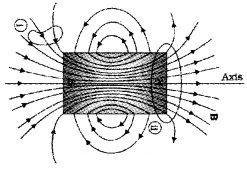
Field lines of bar magnet:
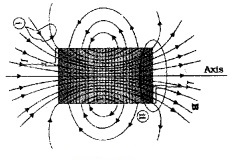
Field lines of current-carrying solenoid:
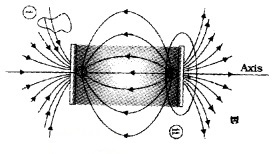
Field lines of electric dipole:
2. Bar magnet as an equivalent solenoid:
Magnetic field along the axis of a solenoid or bar magnet
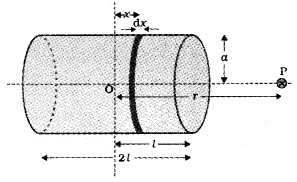
Consider a solenoid of radius ‘a’ and numberof turns per unit length‘n’. Let 2l be the length and I be the current flowing through the solenoid. Consider a point P at a distance ‘r’ from the centre of solenoid. To find magnetic field at P, we take a circular element of thickness dx at a distance x from the centre of solenoid.
The magnetic field at P due to this small element,
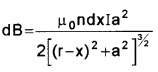
where ndx = N
(total number of turns in a circular element of thickness dx.)
Integrating from x = – l to x = + l, we get
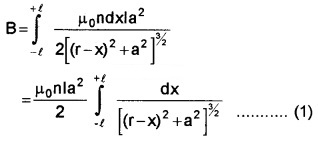
If the point lies at large distance from the solenoid, we can take,
[(r – x)2 + a2]3/2 ≈ r3
r>>a, r>>x
Hence eq.(1) can be written as
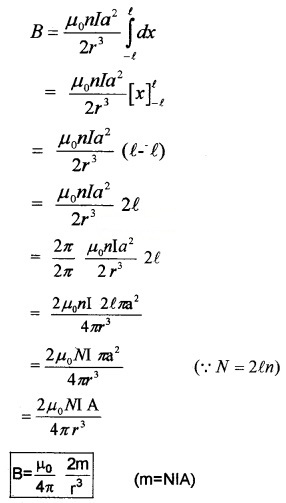
Where m is called magnetic moment of the solenoid.
3. The dipole in a uniform magnetic field:
Torque acting on a magnetic dipole:
Consider a magnetic dipole of dipole moment ‘m’ placed in a uniform magnetic field B. If this dipole is rotated to an angle θ, a restoring torque will act on the needle. ie τ = -mBSinθ.
But we know rotational torque τ = la, where I is the moment of inertia of the magnetic dipole and α is the angular acceleration,
lα = -mBSinθ
(Restoring torque and rotational torque are equal in magnitude but opposite in direction)
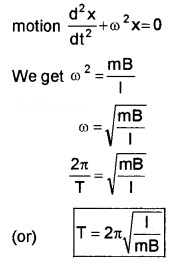
But α = \(\frac{d^{2} \theta}{d t^{2}}\), for small rotations sinθ ≈ θ.
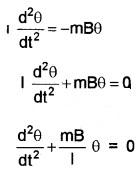
This equation represents that, the oscillation of this magnetic needle is simple harmonic. When we compare the above equation with standard harmonic.
Potential energy of a magnetic dipole:
The work done in rotating a magnet in a magnetic field is stored in it as its potential energy. If dipole is rotated through an angle (dθ) in a uniform magnetic field B, work done for this rotation,
dw = τdθ
dw = mBsinθdθ
If this magnetic needle is rotated from θ1 to θ2, total work done
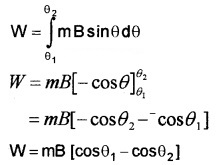
If dipole is rotated from stable equilibrium (θ1 = π/2) to θ2 = 0 we get,
W = -mBcosθ
![]()
This work done is stored as magnetic potential energy, ie.
![]()
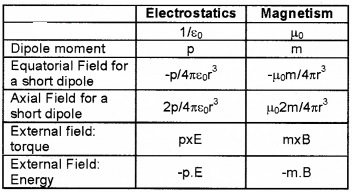
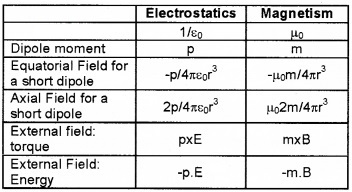
4. The electrostatic analog:
Permanent Magnets And Electromagnets
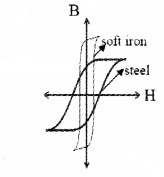
Substances that retain their ferromagnetic property at room temperature for a long time, even after the magnetizing field has been removed are called permanent magnets.
The hysteresis curve helps us to select such materials. They should have high retentivity so that the magnet is strong and high coercivity so that the magnetization is not lost by strong magnetic fields. The material should have a wide hysteresis loop. Steel, Alnico, cobalt-steel and nickel are examples.
Electromagnets are usually ferromagnetic materials with low retentivity, low coercivity and high permeability. The hysteresis curve should be narrow so that the energy liberated as heat is small.
The hysteresis curves of both these materials are shown in the above figure.

Magnetism And Gauss’s Law
Gauss’s law in magnetism: The net magnetic flux through any closed surface is zero.
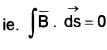
Explanation:
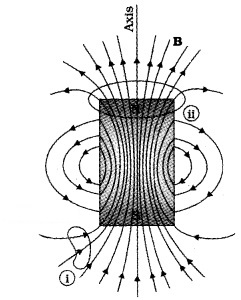
Consider Gaussian surfaces represented by I and II. Both cases demonstrates that the number of magnetic field lines leaving the surface is balanced by the number of lines entering it. This is true for any closed surface.
The Earth’s Magnetism
Earth’s magnetic field rise due to electrical currents produced by motion of metallic fluids in the outer core of the earth. This is known as the dynamo effect.
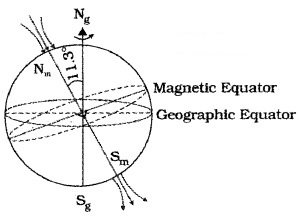
The magnetic field of the earth behaves as magnetic dipole located at the centre of the earth. The axis of the dipole does not coincide with the axis of rotation of the earth. The axis of dipole is titled by 11.3° with axis of rotation of the earth.
The pole near the geographic north pole of the earth is called north magnetic pole (Nm). Likewise, the pole near the geographic south pole is called the south magnetic pole. (Sm).
Note: The north magnetic pole (Nm) behaves like the south pole of a bar magnet (inside the earth). Similarly, the south magnetic pole (Sm) behaves like the north pole of a bar magnet.
(i) Magnetic declination and dip:
The elements of earth’s magnetic field:
The earth’s magnetic field at a place can be completely specified in terms of three quantities. They are
- Declination
- Dip
- Horizontal intensity
Magnetic meridian:
Magnetic meridian at a place is the vertical plane passing through the earth’s magnetic poles.
Geographic meridian:
Geographic meridian at a place is the vertical plane passing through the geographic poles.
1. Magnetic Declination (I):
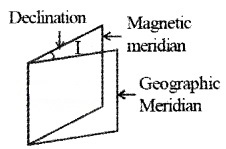
Declination at a place is the angle between the geographic meridian and magnetic meridian at that place.
2. Dip or Inclination (θ):
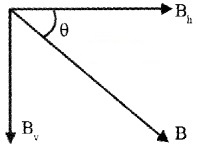
The angle between the earth’s magnetic field and the horizontal component of the earth’s magnetic field at a place is called dip. Dip angle changes from place to place. On the equator, the dip is zero and at the poles, the dip is 90°.
3. Horizontal Intensity Bh:
The horizontal intensity at a place is the horizontal components of the earths field.
Relation between Dip, Horizontal intensity and Earth’s magnetic field:
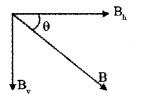
Let B be the Earth’s magnetic field and θ be the angle of dip. Let Bh be the horizontal intensity and Bvthe vertical intensity of the earth’s magnetic field. Then from figure, we get
Bh = B cos θ
The vertical component, Bv = B sin θ
∴ Tanθ = \(\frac{B_{v}}{B_{h}}\)
and resultant field, B = \(\sqrt{\mathbf{B}_{\mathrm{h}}^{2}+\mathbf{B}_{\mathrm{v}}^{2}}\)
Magnetization And Magnetic Intensity
The magnetic properties of a substance can be studied by defining some parameter such as
- Intensity of magnetization (M)
- Magnetic intensity vector (H)
- Susceptibility
- Permeability
1. Intensity of magnetisation (M):
It is defined as the magnetic moment per unit volume. It is the measure of the extent to which a specimen is magnetized.

2. Magnetic Intensity Vector (Magnetising field):
It is defined as the magnetic field which produces an induced magnetism in a magnetic substance. If H is the magnetising field and B the induced magnetic field in the material.
ie. H = \(\frac{B}{\mu}\)
where µ is the constant called the magnetic permeability of the medium.
3. Magnetic susceptibility (χ):
Magnetic susceptibility of a specimen is the ratio of its magnetization to the magnetising field,
ie. χ = \(\frac{M}{H}\)
4. Magnetic permeability (µ):
It is the ratio of magnetic field inside a specimen to the magnetising field.
ie. µ = \(\frac{B}{H}\)
µ = µ0µr
µ0 – Permeability of free space
µr – Relative permeability of a medium.
Relation between permeability and susceptibility:
Let a magnetic material be kept in a solenoid. The specimen gets magnetized by induction. The resultant field inside the specimen is the sum of the field due to the current in the solenoid and the field due to the magnetization of the material.
Resultant field B = Field due to current B0 + Field due to magnetization Bm.
∴ B = B0 + Bm
But Bm = µ0M, B0 = µ0H
∴ B = µ0H + µ0M
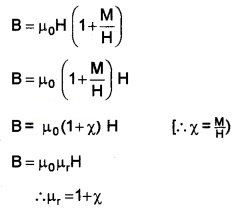
Magnetic Properties Of Materials
Materials can be classified as diamagnetic, paramagnetic or ferromagnetic in terms of the susceptibility χ. A material is diamagnetic if χ is negative, para-if χ is positive and small, and Ferro-if χ is large and positive.
1. Diamagnetism:
Diamagnetic substances are those which have tendency to move from stronger to the weaker part of the external magnetic field.
Diamagnetic material in external magnetic field:
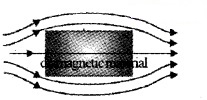
Figure shows a bar of diamagnetic material placed in an external magnetic field. The field lines are repelled and the field inside the material is reduced.
Explanation of diamagnetism:
Electrons in an atom orbit around nucleus. These orbiting electrons produce magnetic field. Hence atom possess magnetic moment.
Diamagnetic substances are the ones in which resultant magnetic moment in an atom is zero. When magnetic field is applied, those electrons having orbital magnetic moment in the same direction slow down and those in the opposite direction speed up.
Thus, the substance develops a net magnetic moment in direction opposite to that of the applied field and hence it repels external magnetic field.
Examples:
Some diamagnetic materials are bismuth, copper, lead, silicon, nitrogen (at STP), water and sodium chloride.
Meissner effect:
The phenomenon of perfect diamagnetism in superconductors is called the Meissner effect.
2. Paramagnetism:
Paramagnetic substances are those which get weakly magnetized in an external magnetic field. They get weakly attracted to a magnet.
Reason for paramagnetism:
The atoms of a paramagnetic material possess a permanent magnetic dipole moment. But these magnetic moments are arranged in all directions.
Due to this random arrangement net magnetic moment becomes zero. But in the presence of an external field B0, the atomic dipole moment can be made to align in the same direction of B0. Hence paramagnetic material shows magnetism in external magnetic field.
Paramagnetic material in external magnetic field:

Figure shows a bar of paramagnetic material placed in an external field. The field lines gets concentrated inside the material, and the field inside is increased.
Examples:
Some paramagnetic materials are aluminium, sodium, calcium, oxygen (at STP) and copper chloride.
Curie law of magnetism:
Curie law of magnetism states that the magnetisation of a paramagnetic material is inversely proportional to the absolute temperature T.
In the case of paramagnetic materials it can be shown that the magnetic susceptibility at temperature is given by
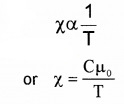
Where C is a constant called curie’s constant.
3. Ferromagnetism:
Ferromagnetic substances are those which gets strongly magnetized in an external magnetic field. They get strongly attracted to a magnet.
Ferro magnetic materials without external magnetic field:

The atoms in a ferromagnetic material possess a dipole moment. These dipoles align in a common direction over a macroscopic volume called domain. Each domain has a net magnetization. Domains are arranged randomly. Hence net magnetic moment of all domains is zero, so ferromagnetic substance does not show magnetism.
Ferro magnetic materials in external magnetic field:
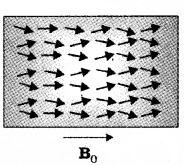
When we apply an external magnetic field B0, the domains arranged in the direction of B0 and grow in size. Thus, in a ferromagnetic material the field lines are highly concentrated.
Question 1.
What happens when the external field is removed?
Answer:
In some ferromagnetic materials the magnetisation persists even if external field is removed. Such materials are called hard ferromagnets. Such materials are used to make permanent magnets.
Eg: Alnico
There is a another class of ferromagnetic materials in which the magnetisation disappears on removal of the external field. Such materials are called soft ferromagnetic materials.
Eg: soft iron
Curie temperature:
The ferromagnetic property depends on temperature. At high temperature, a ferromagriet becomes a paramagnet. The domain structure disintegrates with temperature. This disappearance of magnetisation with temperature is gradual. The temperature of transition from ferromagnetic to paramagnetism is called the Curie temperature Tc.
Variation of B and H in paramagnetic materials:
Figure shows the plot of B versus H. As H is gradually increased from zero, B also increase from zero along OP.
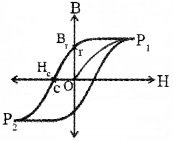
As H increases, more and more magnetic dipoles get aligned in the direction of the field. So M increases and hence B increases.
When all the dipoles get aligned in the direction of the field, the curve becomes almost flat. After this there is no increase of B with H.
When H is gradually decreased from P1 there is no corresponding decrease in the magnetization. The shifting of domains in the ferromagnetic materials is not completely reversible and some magnetization remains even when H is reduced to zero.
The value of the magnetic field when H is zero is called the remanent field Br (Retentivity). If the current in the solenoid is now reversed so that H is in the opposite direction, the magnetic field B can be gradually brought to zero at the point C. The value of H needed to reduce B to zero is called the coercive force He (Coercivity).
The remaining part of curve is obtained by applying H in reverse direction. From these variations it is clear that B always lags behind H. This phenomenon is known as magnetic hysteresis (hysteresis means to lag behind).
The area enclosed by the hysteresis curve gives the loss of energy in the form of heat during the magnetisation – demagnetization cycle.
Permanent Magnets And Electromagnets
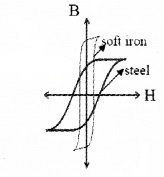
Substances which retain their ferromagnetic property at room temperature for a longtime, even after the magnetizing field has been removed are called permanent magnets.
The hysteresis curve helps us to select such materials. They should have high retentivity so that the magnet is strong and high coercivity so that the magnetization is not lost by strong magnetic fields. The material should have a wide hysteresis loop. Steel, Alnico, cobalt-steel and nickel are examples.
Electromagnets are usually ferromagnetic materials with low retentivity, low coercivity and high permeability. The hysteresis curve should be narrow so that the energy liberated as heat is small.
The hysteresis curves of both these materials are shown in the above figure.

We hope the Plus Two Physics Notes Chapter 5 Magnetism and Matter help you. If you have any query regarding Plus Two Physics Notes Chapter 5 Magnetism and Matter, drop a comment below and we will get back to you at the earliest.
