Kerala Plus Two Physics Model Question Paper 1 with Answers
| Board | SCERT |
| Class | Plus Two |
| Subject | Physics |
| Category | Plus Two Previous Year Question Papers |
Time: 2 Hours
Cool off time : 15 Minutes
Maximum : 60 Score
General Instructions to candidates:
- There is a ‘cool off time’ of 15 minutes in addition to the writing time of 2 hrs.
- You are not allowed to write your answers nor to discuss anything with others during the ‘cool off time’.
- Use the ‘cool off time’ to get familiar with the questions and to plan your answers.
- Read questions carefully before you answering.
- All questions are compulsory and only internal choice is allowed.
- When you select a question, all the sub-questions must be answered from the same question itself.
- Calculations, figures and graphs should be shown in the answer sheet itself.
- Malayalam version of the questions is also provided.
- Give equations wherever necessary.
- Electronic devices except non programmable calculators are not allowed in the Examination Hall.
Questions 1 – 7 Carry 1 Score each. Answer any six questions. (6 × 1 = 6)
Question 1.
Write the expression for intensity of electric field near to the surface of a charged conductor with uniform charge density σ.
Answer:
E = σε0
Question 2.
The unit of electrical resistivity is ……….
Answer:
Ωm (ohm metre)
Question 3.
E.m.f can be induced in a coil placed in an external magnetic field by:
a) Changing the intensity of magnetic field
b) Changing the area of coil
c) Changing the orientation of the coil
d) All of the above
Answer:
d) All of the above
Question 4.
Write the energy and momentum associated with a moving photon.
Answer:
E = hν, P = mc
Question 5.
The value of relative permeability of a diamagnetic material is;
a) μr > 1
b) μr = 1
c) μr < 1
d) μr = 1
Answer:
c) μr < 1
Question 6.
The electric field amplitude of an electromagnetic wave is 15V/m. Find the magnetic field amplitude of the wave.
Answer:
B = EC=153×108 = 5 × 10-8T
Question 7.
A convex lens is placed in a medium having refractive index greater than that of the lens. The lens now behaves as;
a) Converging lens
b) Diverging lens
c) Plane glass plate
d) None of the above
Answer:
b) Diverging lens
Questions 8 to 15 carry 2 score each. Answer any 7 questions. (7 × 2 = 14)
Question 8.
a) ‘Electrostatic field is always normal to the surface of a charged conductor’. Justify the statement.
b) The value of elelctric potential at the surface of a charged conductor is 10V. Find the vlaue of intensity of elecric field and potential at a point interior to it.
Answer:
a) The surface of charged conductor is an equi- potential surface. In equipotential surface, potential is same and hence electric field is always normal to surface of a charged conductor.
b) E = 0
Question 9.
A galvanometer with a coil of resistance 12Ω shows a full scale deflection for a current of 2.5 mA. How can it be convereted into an ammeter of range 7.5 A?
Answer:
G= 12Ω, Ig= 2.5 mA = 2.5 × 10-3A, I = 7.5 A
S = Ig×GI−Ig=2.5×10−3×127.5−2.5×10−3=4×10−3Ω
Question 10.
The curves shown in figure are drawn for different magnetic materials. Among the three curves, name the curve that,
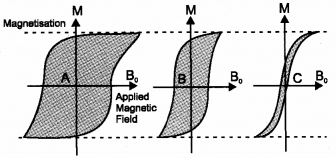
a) Represent the material usually used for making permanent magnets.
b) Represent the material usually used for making electromagnetis.
Answer:
a) A
b) C
Question 11.
A capacitor C, a variable resistance R and a bulb B are connected in series to a.c. mains in the circuit as shown. The bulb glows with some brightness. How the glow of the bulb change if.
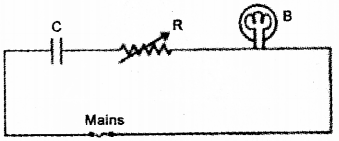
a) A dielectric slab is introduced between the plates of the capacitor.
b) The resistance R is increased keeping the same capacitance.
Answer:
a) The brightness of bulb increases.
(When dielectric slab is introduced, capacitance increases hence capacitive reactance decreases, then bulb glows with more brightness.)
b) The brightness of bulb decreases.
Question 12.
James Clerk Maxwell modified Ampere’s circuital theorem by introducing the concept of displacement current.
a) What do you mean by displacement current?
b) Write down the equation for displacement current.
Answer:
a) The current due to time varying electric field is called displacement current.
b) id = ε0dφdt
Question 13.
An object AB is kept in front of a concave mirror as shown in figure.
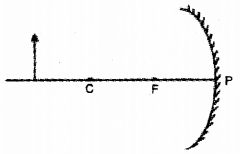
a) Complete the ray diagram showing the image formation of object.
b) How will the position and intensity of image be affected if the lower half of the mirror’s reflecting surface is painted black?
Answer:
a)
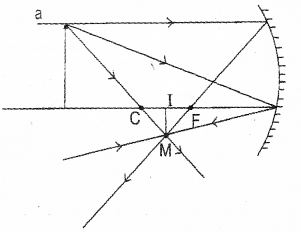
b) The position of image does not charge. But the intensity decreases.
Question 14.
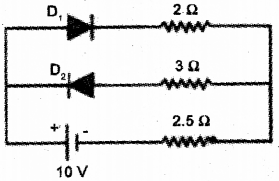
Assuming that the two diodes D1 and D2 used in the electric circuit as shown in figure are ideal. Find out the value of current flowing through 2.5Ω resistor.
Answer:
Since D2 is reverse biased, no current flows through 3Ω. We need to consider only the other two resistance 3Ω and 2.5Ω, which are connected in series. Total resistance, R = 3Ω + 2.5Ω = 5.5Ω
v = 10V
I = VR=105.5 = 1.81 A
Question 15.
a) Mention the function of the following used in the communication system
(i) Transducer
(ii) Transmitter
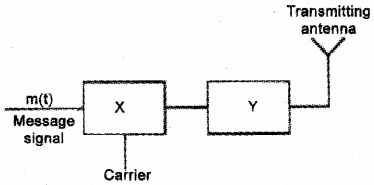
b) Figure shows the block diagram of a AM transmitter. Identify the boxes X and Y.
Answer:
a) i) Transducer: The device that converts one form of energy into another is called tranducer.
ii) Transmitter – Atransmittertransmits the information
b) x → Modulator
y → Power Amplifier
Questions 16 to 22 carry 3 scores each. Answer any 6 questions. (6 × 3 = 18)
Question 16.
Three capacitors of capacitances 2µF, 3µF and 4µF are connected in series.
a) Find the equivalent capacitance of the combination
b) The plates of a parallel plate capacitor have an area 20 cm2 each are separated by a distance of 2.5 mm. The capacitor is charged by connecting it to a 400V supply. How much electrostatic energy is stored in the capacitor?
Answer:
a) C1 = 2μF = 2 × 10-6F
C2 = 3μF = 3 × 10-6F
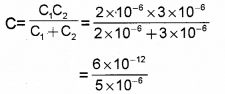
b) A = 20cm2 = 20 × 10-4m2
d = 2.5 mm = 2.5 × 10-3m
ε0 = 8.85 × 10-12C2/Nm2
v = 400v
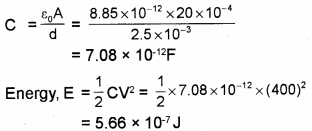
Question 17.
A circuit using potentiometer and a battery of negligible internal resistance is set up as shown to develop a constant potential gradient along the wire PQ. Two cells of e.m.fs E1 and E2 are connected in sereis as in figure in combination 1 and 2. The balance points are obtained respectively at 400 cm and 240 cm from point P. Find
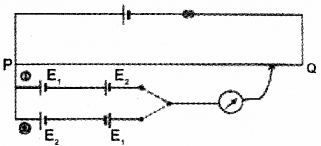
a) The ratio between E1 and E2
b) Balancing length for the cell with emf E1 only.
Answer:
a) From the connection (1), we get
E1 + E2 = k × 400 ………(1)
k = constant
From the connection (2), we get
E2 – E1 = 240 K ……….(2)
Adding (1) and (2), we get
2E2 = 640 K
E2 = 320 K
Subtituting for E2 in equation (1)
E1 + 320 K = 400 K
E1 = 80 K
The ratio E1E2=14
b) l1 be the balancing length of E1
E1 = kl1
80k ∝ kl1
l1 = 80 cm
Question 18.
A conducting rod PQ of length ‘l’ connected to a resistance ‘R’ is moved at a uniform speed ‘V’, normal to a uniform magnetic field ‘B’.
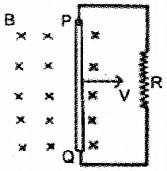
a) Deduce the expresssion for e.m.f induced in the conductor.
b) Find the magnitude and direction of current through the conductor.
Answer:
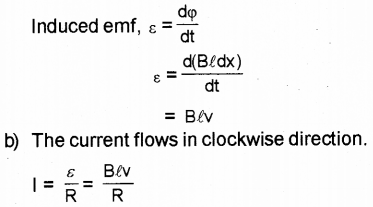
a) flux, Φ = BA
Here, Area A = ldx
dx is small displacement rod in time Δ T
Φ = B × l × dx
Question 19.
In tuner circuits, we use the phenomenon of resonance.
a) Write the condition of resonance in series LCR circuit.
b) A series LCR circuit uses L = 0.1 H, C = 10μF and R = 100Ω. Find the value of frequency at which the amplitude of current is maximum.
Answer:
a) Lω = 1Cω
b) L = 0.1 H, C = 10μF = 10 × 10-6F
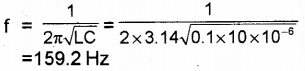
Question 20.
The focal length of a lens has dependence on its radii of curvatures and refractive index. Derive Lens maker’s formula.
Question 21.
a) Write the expression for the de Broglie wavelength associated with a charged particle having charge ‘q’ and mass ‘m’, when it is accelerated by a protential of ‘V’ volts.
b) A proton and an electron have same kinetic energy. Which one has greater value of de Broglie wavelength and why?
Answer:
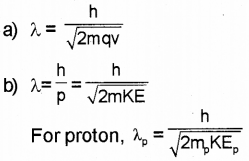
For electron, λe = h√2meKEe
But KEp = KEe and mp > Me, hence λe > λp
Electron has greater de Broglie wavelength.
Question 22.
Find the binding energy per nucleon of 4020Ca nucleus.
Given m4020Ca = 39.962589u.
mp = 1.00783u, mn = 1.00867u.
Take 1 amu = 931 MeV/c2
Answer:
Mass detect, Δm = Zmp + (A – Z) mn – M
Δm = 20 mp + 20 mn – 39.962589
= 20 × 1.00783 + 20 × 1.00867 – 39.962589
= 0.367411 u
Binding energy = 0.367411 × 931 = 342.06 MeV
Questions from 23 – 26 carry 4 scores. Answer any 3 questions. (3 × 4 = 12)
Question 23.
Gauss’s theorem is useful for finding the intensity of elelctric field.
a) Write the Gauss’s law in its mathematical form
b) Using the law, prove that intensity of electric field at a point due to a uniformly charged infinite plane sheet is independent of the distance from it.
Answer:
ϕ=∮→E⋅d→s=qε0
Question 24.
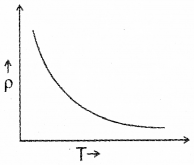
a) Express Ohm’s law in terms of current density, electrical resistivity and intensity of electric field.
b) Explain the variation of resistance of a semiconductor with temperature. Also draw the graph showing the variation of resistivity of silicon with temperature.
Answer:
a) J = σE
b) As temperature increases, the number density of electrons (η) of semiconductor increases.
ρ = mhe2τ
Hence resistivity decreases with increase in temperature.
Question 25.
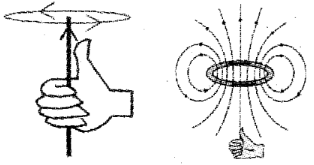
The relation between magnetic field and current is given by Biot-Savart’s law.
a) Write the expression for the magnetic field at point along the axis of a circuit loop of radius ‘R’ carrying a current ‘I’.
b) From the above expression. Find the value of magnetic field at the centre of the loop.
c) Sketch the magnetic field lines for current carrying circular loop
Answer:
a) B = μ0NIR22(x2+R2)3/2
I → Current
N → Number of turns
X → distance tot he point from the center of loop
b) B = μ0NI2a
c)
Question 26.
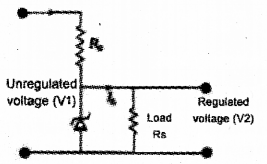
a) Identify the diode in the circuit and write the use of the resistance Rs.
b) Explain how the diode helps as to stabilize the output voltage of the circuit.
c) Name the type of biasing used in this diode
Answer:
a) Zenerdiode, Voltage regulator
b) Solar Cell: Solar cell is junction diode used to convert solar energy into electrical energy.
Circuit details:
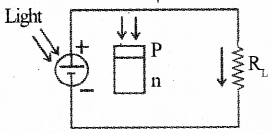
Its p-region is thin and transparent and is called emitter. The n-region is thick and is called base. Output is taken across RL.
Working: When light falls on this layer, electrons from the n-region cross to the p-region and holes in the p-region cross into the n-region. Thus a voltage is developed across RL.
Solar cells are used to charge storage batteries during daytime.
c) Reverse bias
Questions 27 to 29 carry 5 scores. Answer any 2 questions. (2 x 5 = 10)
Question 27.
In the figure, PQ is the incident ray on the equilateral glass prism ABC.
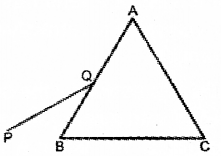
a) Complete the ray diagram showing the passage of light and mark the angle of deviation.
b) Derive an expression for the refractive index of the material of the prism.
Answer:
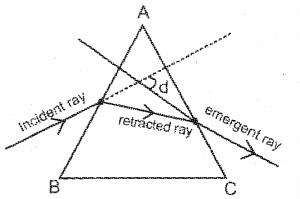
b)
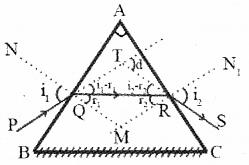
ABC is a section of a prism. AB and AC are the refracting faces, BC is the base of the prism. ∠A is the angle of prism.
A ray PQ incidents on the face AB at an angle i1. QR is the refracted ray inside the prism, which makes two angles r1 and r2 (inside the prism). RS is the emergent ray at angle ‘i2’
The angle between the emergent ray and incident ray is the deviation ‘d’.
In the quadrilateral AQMR,
∠Q + ∠R = 180°
[since N1M and NM are normall
i.e. ∠A + ∠M = 18O° ………(1)
In the ΔQMR,
∴ r1 + r2 + ∠M = 180°……..(2)
Comparing eq (1) and eq (2)
r1 + r2 = ∠A ……….(3)
From the ΔQRT,
(i1 – r1) + (i2 – r2) = d
[since exterior angle equal sum of the opposite interior angles]
(i1 + i2) – (r1 + r2) = d
but, r1 + r2 = A
∴ (i1 + i2) – A = d
(i1 + i2) = d + A ……..(4)
It is found that for a particular angle of incidence, the deviation is found to be minimum value ‘D.
At the minimum deviation position,
i1 = i2 =i, r1 = r2 = r and d=D
Hence eq (3) can be written as,
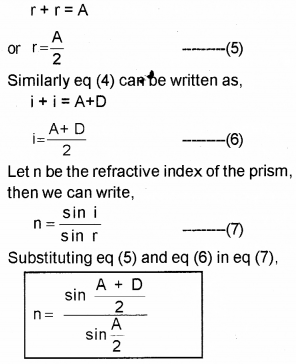
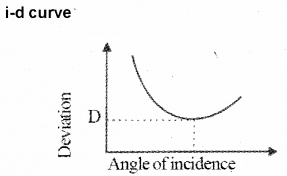
It is found that when the angle of incidence increases deviation (d) decreases and reaches a minimum value and then increases. This minimum value of the angle of deviation is called the angle of minimum deviation.
Question 28.
According to Christian Huygens wave theory, light emanating from a source as wave fronts.
a) What is the shape of wave front emerging form a linear source?
b) Derive the mathematical expression for the bandwidth of interference bands obtained in young’s double slit experiment with the help of suitable diagram.
Answer:
a) Cylinder
b)
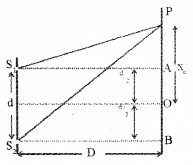
S1 and S2 are two coherent sources having wave length A. Let ‘d’ be the distance between two coherent sources. A screen is placed at a distance D from sources. ‘O’ is a point on the screen equidistant from S1 and S2.
Hence the path difference, S1O – S2O = 0
So at ‘O’ maximum brightness is obtained.
Let ‘P’ be the position of nm bright band at a distance xn from O. Draw S1A and S2B as shown in figure.
From the right angle ΔS1AP
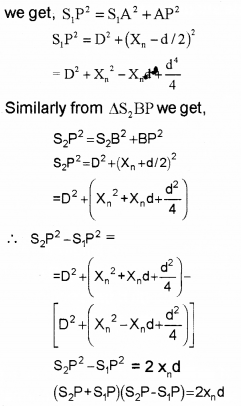

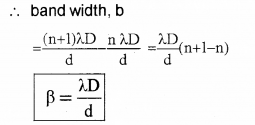
This is the width of the bright band. It is the same for the dark band also.
Question 29.
a) The radius of nth stationary orbit of hydrogen atom is:
rn = n2 h2ε0πme2
Using Bohr postulates, obtain an expression for the energy of electron in the stationary states of H-atom.
b) Draw the energy level diagram showing how the spectrallines corresponding to Balmerseries occur due to transition between the energy levels.
Answer:
a) Consider hydrogen atom which has one electron revolving around nucleus having one proton. The centripetal force for electron is provided by electrostatic force between electron and proton
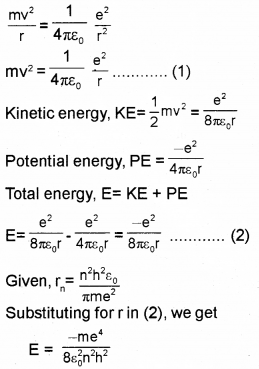
b) Energy level diagram of hydrogen atom
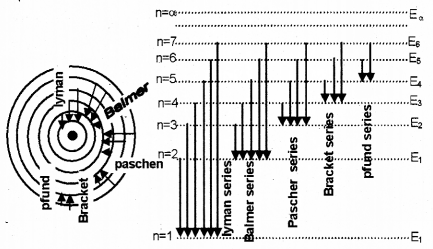
Note: An electron can have any total energy above E=0ev. In such situations electron is free. Thus there is a continuum of energy states above E = 0ev.
