Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity is part of Plus Two Physics Chapter Wise Questions and Answers. Here we have given Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity.
| Board | SCERT, Kerala |
| Text Book | NCERT Based |
| Class | Plus Two |
| Subject | Physics Chapter Wise Questions |
| Chapter | Chapter 3 |
| Chapter Name | Current Electricity |
| Category | Kerala Plus Two |
Kerala Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity
Plus Two Physics Current Electricity NCERT Text Book Questions and Answers
Question 1.
A storage battery of a car has an e.m.f. of 12V. If the internal resistance of the battery is 0.4W, what is the maximum current that can be drawn from the battery?
Answer:
E = 12 V, r = 0.4Ω, I = ?
Since I = Er=120.4 = 30A.
Question 2.
A battery of e.m.f. 10V and internal resistance 3W is connected to a resistor. If the current in the circuit is 0.5A, what is the resistance of the resistor? What is the terminal voltage of the battery when the circuit is closed?
Answer:
Given E = 10V, r=3?, I = 0.5, R = ?
Since V = E – Ir
or V= 10 – 0.5 × 3
or V = 8.5 volt
And R = VI=8.50.5 = 17A.
Question 3.
- Three resistors 1W, 2W and 3W are combined in series. What is the total resistance of the combination?
- If the combination is connected to a battery of e.m.f. 12V and negligible internal resistance, obtain the potential drop across each resistor:
Answer:
Given
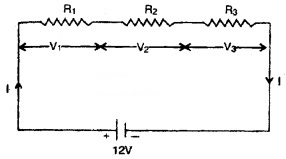
R1 = 1Ω, R2 = 2Ω , R3= 3Ω
1. Total resistance of series combination
R = R1 + R2 + R3
or R – 1 + 2 + 3 = 6Ω.
2. Since V=IR
I = VR=126
∴ V1 = IR1 = 2 × 1 = 2V
V2 = IR2 = 2 × 2 = 4V
V3 = IR3 = 2 × 3 = 6V.
Question 4.
A silver wire has a resistance of 2.1W at 27.5°c, and a resistance of 2.7W at 100°C. Determine the temperature coefficient of resistivity of silver.
Answer:
Rt1 = R27.5 = 2.1Ω and
Rt2 = R100 = 2.7Ω
Applying the relation
![]()
a = 0.0039°C-1
Question 5.
The number density of free electrons in a copper conductor estimated is 8.5 × 1028m-3. How long does an electron take in drifting from one end of a wire 3.0m long to its other end? The area of cross-section of the wire is 2.0 × 10-6m2 and it is carrying a current of 3.0A.
Answer:
n = 8.5 × 1028m-3; A = 2 × 10-6m2
I – 3.0A; I = 3m
I = vdneA
3t=38.5×10×1.6×10×2×10
t = 8.5 × 1128 × 1 .6 × 10-19 × 2 × 10-6
= 27.2 × 10-3sec.
Question 6.
Choose the correct alternative:
- Alloys of metals usually have (greater/ less) resistivity than that of their constituent metals.
- Alloys usually have much (lower/higher) temperature coefficients of resistance than pure metals.
- The resistivity of alloy manganin (is nearly indepent of/ increases rapidly) with increase of temperature.
- The resistivity of a typical insulator (e.g. amber) is greater than that of a metal by a factor of the order of (1022/1023).
Answer:
- Greater
- lower
- nearly independent of
- 1022.
Plus Two Physics Current Electricity One Mark Questions and Answers
Question 1.
n equal resistors are first connected in series and then connected in parallel. What is the ratio of the maximum to the minimum resistance?
(a) n
(b) 1/n2
(c) n2
(d) 1/ n
Answer:
(c) n2
Explanation: In series Rs = nR
In parallel 1Rp = 1R + 1R ….n terms, Rp = Rn
∴ Rs/Rp = n2/1.
Question 2.
A car battery of emf 12 V and internal resistance 5 × 10-2Ω, receives a current of 60 amp, from external source, then potential difference of battery is.
(a) 12V
(b) 9V
(c) 15V
(d) 20 V
Answer:
(c) 15V
When cell is changed by an external source, terminal voltage, V = E + Ir
V= 12 + 60 × 5 × 10-2
=15.
Question 3.
A flowing of 107 electron persecond in a conducting wire constitutes a current of……….A
(a) 1.6 × 10-12
(b) 1.6 × 1026
(c) 1.6 × 10-26
(d) 1.6 × 1012
Answer:
(a) 1.6 × 10-12
Flow of electrons nt = 107/sec.
There fore, Current (I) = qt = net = nt × e
= 107 × (1.6 × 10-19)
= 1.6 × 10-12 A.
Question 4.
Copper and silicon is cooled from 300K to 60K, the specific resistance.
(a) decrease in copper but increase in silicon
(b) increase in copper but decrease in silicon
(c) increase in both
(d) decrease in both
Answer:
(a) decrease in copper but increase in silicon.
Question 5.
State the potentiometer principle.
Answer:
Potential difference between two points of a current carrying conductor is directly proportional to the length of the wire between two points.
Question 6.
Find the current following through the network shown in the figure
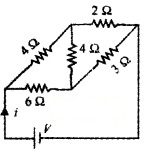
Answer:
Since given circuit is in the form of Wheatstone bridge,
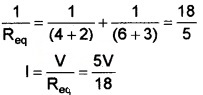
Question 7.
Kirchhoff’s first and second laws of electrical circuits are consequences of……(1)….and…..(2).. respectively
Answer:
- Conservation of electric charge
- Energy respectively
Question 8.
Pick the odd one out of the following.
(a) Ohm’s law
(b) Lenz’slaw
(c) Coulomb’s law
(d) Gauss’s law
(e) Energy conservation law
Answer:
(a) Ohm’s law (It is not a universal law).
Question 9.
“Ohms law is not a fundamental law” Comment on this.
Answer:
Ohms law is not a universal law because metals do not obey this law at high temperatures. Moreover, certain materials (diode and transistors, etc.) does not obey ohms law.
Plus Two Physics Current Electricity Two Mark Questions and Answers
Question 1.
Figure below shows a diagram of a water circuit. In many ways, it behaves like an electric circuit. Draw an equivalent electric circuit. (Hint – water-wheel can be replaced by motor)
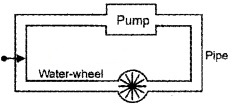
Answer:
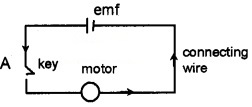
Question 2.
A, B, C, and D are four rings on a carbon resistor. A = yellow, B = violet, C = Yellow, D, Silver
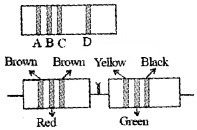
1. What is the value of resistance of above resistor?
2. The combined resistance of the above two resistor is
- 120Ω
- 45Ω
- 165Ω
- 35Ω
Answer:
1. 47 × 104 ± 10%.
2. 12 × 101 + 45 × 10°
120 + 45 = 165Ω.
Question 3.
A = Brown B = Black C= Red D= Gold
- What is the value of resistance without considering variation?
- If the fourth ring is silver coloured what will be the change in accuracy?
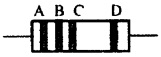
Answer:
- 1000Ω ± 5%
- 10%
Question 4.
A junction of an electrical circuit is given below. Analyze the figure and answer the following
- What is the value of I1 and I2?
- State the law that can be applied to find I1 and I2?
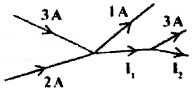
Answer:
1. According Kirchoff first rule
3 + 2 = I + I1
I1 = 4A
I1 = 3 +I2
4 = 3 + I2
I2 = 1A
2. Total current meeting at any junction is zero.
Question 5.
The following question consists of two statements each, printed as assertion and reason. While answering these questions you should choose any one of the following responses.
Assertion: In a simple battery circuit, the point at the lowest potential is positive terminal of the battery. Reasons: The electrons flows from higher potential to lower potential.
(a) Both assertion and reason are true and the reason is a correct explanation of the assertion.
(b) Both assertion and reason are true but the reason is not a correct explanation of the assertion.
(c) Assertion is true but reason is false.
(d) Both assertion and reason are false.
Answer:
(d) Both assertion and reason are false.
Plus Two Physics Current Electricity Three Mark Questions and Answers
Question 1.
Match the following.
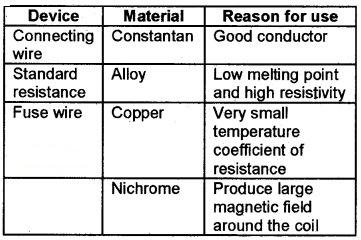
Answer:
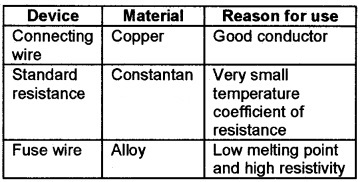
Question 2.
Match the following.
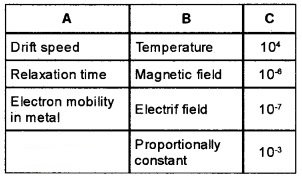
Answer:
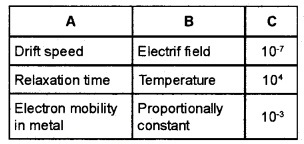
Question 3.
“When electric current is passed through a resistance wire, it get heated up”.
- Name the law associated with this phenomenon.
- What happens to the heat energy developed, if the current through the wire is doubled.
Answer:
- Joules law of heating
- Heat developed in the conductor H = 12R If current is doubled, Heat developed becomes 4 times.
Question 4.
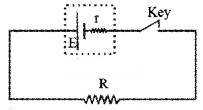
1. Name the pd between terminals of the cell when
- key K is open
- K is closed
2. What is the reason for the difference in potential in the above two cases?
Answer:
1. key K is open
- e.m.f
- voltage.
2. Emf in a circuit
E = Ir + V
When key is open, I = 0
∴ E = V
When key is closed I ? 0
∴ V = E – Ir.
Question 5.
The variation of resistivity (ρ) with temperature (T) of a conductor, semiconductor and super conductor are given in the figure.
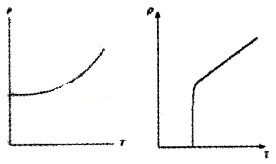
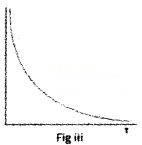
- Identify them from the graph. (1)
- Identify the figure in which the temperature coefficient of resistance of the material is positive. (1)
- Write the equation connecting resistivity of the material with relaxation time. (1)
Answer:
1. From the graph:
- Fig (i) – Conductor
- Fig (ii) – Superconductor
- Fig (iii) – Semiconductor
2. Fig(i)
3. We have resistivity (ρ) from equation.
ρ = mne2τ, where t is the relaxation time, m mass of electron, n number density of electron and e charge of electron.
Question 6.
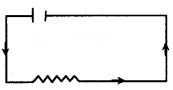
When an animal touches an ‘electric-fence’ the animal gets a shock by completing an electrical circuit.
- Draw an equivalent circuit using symbols, which shows the completed electrical circuit. (Assume that the animal has a resistance)
- How much charge passes through the animal, if it receives a current pulse of 20mA for 0.1 seconds.
- When a bird perches on the electric fence, the bird will not get a shock. Why?
Answer:
1.
2. 1 = Q/t
Q = 1 × t = 20 × 10-3 × 0.1 = 20 × 10-4C
3. Since the bird does not come in contact with the earth, its body is at same potential. So there is no potential difference and current. Hence the bird will not get a shock.
Question 7.
1. Pd between terminals when k is open is called
- emf
- lost voltage
- terminal voltage
- induced voltage
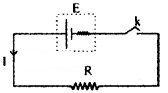
2. Give an equation connecting R, r, I and E What is the value of E for open circuit and ideal cell.
3. What happens to terminal voltage if current increases
- for an ideal cell
- for an ordinary cell.
Answer:
1. e.m.f
2. E = I(r+R).
3. For ideal cell, internal resistance is zero. Hence E = v. ie. terminal voltage does not change with the current.
For ordinary cell, when current increases, V decreases.
Question 8.
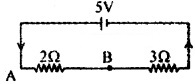
- What is the current through this circuit?
- What is p.d. across 2Ω and 3Ω?
- What is potential at A?
- What is potential at B?
Answer:
1. Current I = total voltage total resistance
I = 52+3 = 1A
2. Voltage across 2Ω
V = 1 × 2
V2 = 2 V
Voltage across 3Ω
V = 1 × 3 = 3V
3. 5V
4. 3V
Question 9.
The relation between Voltage V (across the conductor) and current I through the conductor is given in the graph.
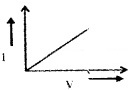
- Which law establishes the relation between voltage and current?
- A metal wire of resistivity 6.4 × 10-5 ohm-cm and length 1.98 m has a resistance of 7Ω. Find radius of wire
Answer:
1. Ohms law
2. Resistance of wire R = \frac{ρl}{A}
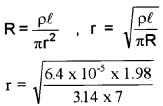
= 2 × 10-3m.
Question 10.
A circuit diagram of an instrument is given below.
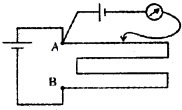
- Identify the instrument and state the principle of this instrument
- Modify the circuit diagram to compare the emf of two cells.
- How can increase the sensitivity of this instrument?
Answer:
1. Potentio meter
2. Comparison of e.m.f of two cells using potentiometer
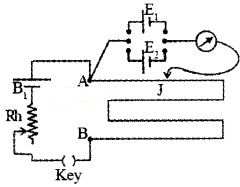
a. Principle:
Potential difference between two points of a current-carrying conductor (having uniform thickness) is directly proportional to the length of the wire between two points.
b. Circuit details :
A battery (B1), Rheostat and key are connected in between A and B. This circuit is called primary circuit. Positive end of E1 and E2 are connected to A and other ends are connected to a two way key. Jockey is connected to a two key through galvanometer. This circuit is called secondary circuit.
c. Working and theory :
Key in primary circuit is closed and then E1 is put into the circuit and balancing length l1 is found out.
Then E1∝ l1 …………(1)
Similarly E2 is put into the circuit and balancing length (l2) is found out.
Then, E2 ∝ l2 (2)
Dividing Eq(1)byEq(2),
\frac{E_{1}}{E_{2}}=\frac{l_{1}}{l_{2}}………..(3)
3. Increase the length of potentio meter wire.
Question 11.
A long resistance wire is stretched between two iron nails. A battery of 2V is applied across the wire. One end of a torch bulb is connected to nail and other end is made in contact as shown in figure.
1. If this wire slides over the resistance wire from nail 1 to nail 2, what happens to the brightness of the bulb.
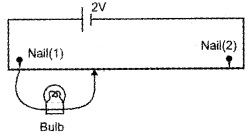
- Increases
- Decreases
- Remains constant
- First increases then decreases.
2. How this principle is used to determine internal resistance of cell.
3. What happens to the reading, if we change 2V with 3V during the time of reading.
Answer:
1. Increases.
2. Measurement of internal resistance using potentiometer Principle:
[Same as before]
a. Circuit details :
Battery B1 Rheostat and key K1 are connected in between A and B. This circuit is called primary.
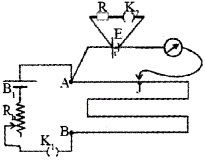
In the secondary circuit a battery E having internal resistance ‘r’ is connected. A resistance box (R) is connected across the battery through a key (K2). Jockey is connected to battery through galvanometer.
b. Working and theory :
The key (K1) in the primary circuit is closed and the key is the secondary (K2) is open. Jockey is moved to get zero deflection in galvanometer. The balancing length l1, (from A) is found out.
Then we can write.
E1∝ l1 ____(1)
Key K2 is put in the circuit, corresponding balancing length (l2) is found out. Let V be the applied voltage, then
V1 ∝ l1 ____(2)
‘V’ is the voltage across resistance box. Current through resistance box ie, voltage across resistance,
V = \frac{E R}{(R+r)} _____(3)
Substituting eq (3) in eq (2),
\frac{E R}{(R+r)} ∝ l2 ______(4)
Dividing eq (1) by eq (4),
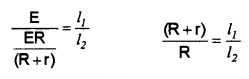
r = \frac{\mathrm{R}\left(l_{1}-l_{2}\right)}{l_{2}}
3. Primary Voltage should not change while doing experiment. When we use 3v instead of 2v, potential gradient will change. Hence balancing length will change.
Question 12.
“Electric current has both direction and magnitude”
- What is meant electric current
- What is the conventional direction of electric current
- Even though current has both magnitude and direction it is not a vector quantity. Why?
- Thermal Motion of electrons in a conductor cannot constitute an electric current. Why?
Answer:
- Rate of flow of charge is called current I = dq/dt
- Direction of motion of positive charges
- It does not obey vector law of addition
- Average velocity of thermal motion is zero. Hence thermal motion does not produce current.
Question 13.
Match the following
| A | B |
| Metals | Cooper pairs |
| Semiconductors | electrons and holes |
| superconductors | positrons |
| electrons |
Answer:
- Metals – electrons
- Semiconductors – electrons and holes
- Superconductors – cooper pairs
Plus Two Physics Current Electricity Four Mark Questions and Answers
Question 1.
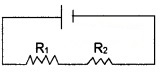
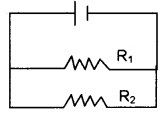
A cell arid two resistors R1 and R2 are provided to you.
- Draw different combinations of resistors using R1, R2, and the cell.
- Derive an expression for the effective resistance of the circuit in which current is the same in both resistors
- If R1= 4Ω and R2 = 6Ω, in which combination effective resistance is minimum? Find its value?
Answer:
1.
2. Derive an expression for effective resistance in series:
Consider three resistors R1, R2 and R3 connected in series and a pd of V is applied across it.
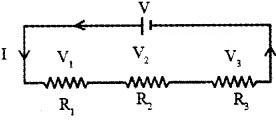
In the circuit shown above the rate of flow of charge through each resistor will be same i.e. in series combination current through each resistor will be the same. However, the pd across each resistor are different and can be obtained using ohms law.
pd across the first resistor V1 = I R1
pd across the second resistor V2 = I R2
pd across the third resistor V3 = I R3
If V is the effective potential drop and R is the effective resistance then effective pd across the combination is
V = IR
Total pd across the combination = the sum pd across each resistor, V = V1 + V2 + V3
Substituting the values of pds we get IR = IR1 + IR2 + IR3
Eliminating I from all the terms on both sides we get
R = R1 + R2 + R3 ………(1)
Thus the effective resistance of series combination of a number of resistors is equal to the sum of resistances of individual resistors.
3. The effective resistance becomes minimum in parallel connection.
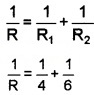
R = 2.4Ω.
Question 2.
1. State whether the following statement is true or false “The value of resistance of a metal increase with the rise of temperature”.
2. Explain the reason.
3. With the help of the graph, match the following
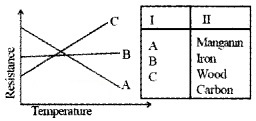
4. Alloys like manganin, eureka, constantan, etc. are used in making standard resistance coils. Why?
Answer:
1. True.
2. When temperature increases, the amplitude of vibration of atom increases. Hence relaxation time decreases. Hence resistivity of metal increases according to the equation
![]()
3.
A_____Carbon
B_____Manganin
C_____Iron.
4. The temperature coefficient of resistance of manganin, eureka and constantan, etc are zero. Hence they are used in making standard resistance coils.
Question 3.
1. Identify the above device and give the principle behind it.
2. Obtain the mathematical condition for the galvanometer current to be zero.
3. If the balancing length T obtained fora resistance wire in the arrangement is 40cm. Find the new balancing length if the same resistance wire is folded to half its length and connected to the same gap.
Answer:
1. Meter bridge.
2. We get galvanometer current as zero, when P/Q = R/S. For derivation of P/Q=R/S
Wheatstone’s Bridge:
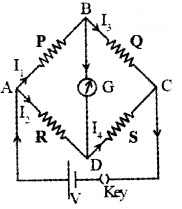
Four resistances P, Q, R, and S are connected as shown in figure. Voltage ‘V’ is applied in between A and C. Let I1, I2, I3 and I4 be the four currents passing through P, R, Q, and S respectively.
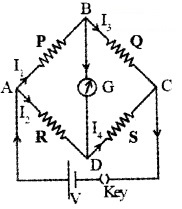
Working:
The voltage across R
When key is closed, current flows in different branches as shown in figure. Under this situation
The voltage across P, VAB = I1P
The voltage across Q, VBC = I3Q ……(1)
The voltage across R, VAD = I2R
The voltage across S, VDC = I1S
The value of R is adjusted to get zero deflection in galvanometer. Under this condition,
I1 = I3 and I2 = I4……(2)
Using Kirchoff’s second law in loopABDA and BCDB, weget
VAB = VAD ……….(3)
and VBC = VDC …….. (4)
Substituting the values from eq(1) into (3) and (4), we get
I1P = I2R……….(5)
and I3Q = I4S……..(6)
Dividing Eq(5) by Eq(6)
\frac{I_{1} P}{I_{3} Q}=\frac{I_{2} R}{I_{4} S}
\frac{P}{Q}=\frac{R}{S} [since I1 = I3 and I2 = I4]
This is called Wheatstone condition.
3. When we apply this condition in meter bridge, we get
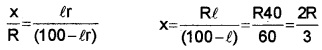
If wire is folded, New resistance x1 = \frac{x}{2}
Substituting this in P/Q = R/S we get
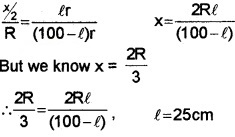
Question 4.
1. Potentiometer is better than voltmeter for measuring emf because
- It is cheap
- Easy to handle
- Its measurement uses null method
2. Give the basic principle of potentiometer,
3.
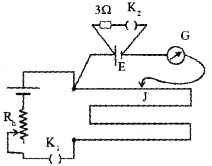
If K2 is open balancing length is 600cm, if K2 is closed 350cm is balancing length. Calculate the internal resistance.
Answer:
1. Its measurement uses null method.
2. Principle:
Potential difference between two points of a current-carrying conductor (having uniform thickness) is directly proportional to the length of the wire between two points.
3. I = 600cm, I = 350 cm
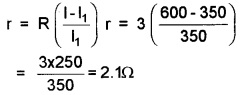
Question 5.
Under an external electric field electrons drift slowly inside the conductor.
1. The velocity of drift is
- 1 mm/s
- 105 m/s
- 3 × 108 m/s
- 3 × 109 m/s
2. What is meant by relaxation time?
3. Write an expression for drift velocity in terms of relaxation time.
4. When temperature increases what happens to drift velocity?
Answer:
1. 1mm/s.
2. The average time between two successive collision is called relaxation time.
3. Vd = \frac{\mathrm{eE}}{\mathrm{m}} t
4. We know drift velocity
Vd = \frac{\mathrm{eE}}{\mathrm{m}} t
When temperature increases, relaxation time decreases. As a result drift velocity decreases.
Question 6.
1. State whether the following statement is correct. “If the current through the cell is from its positve to negative, against the direction of emf, then the potential drop across the internal resistance aids the emf of the cell. (1)
2. You are given two cells. Group them so that they give more voltage. Arrive at the expression for effective emf and internal resistance. (3)
Answer:
1. True. Internal resistance and lost volt always opposes current flow through the cell”.
2.
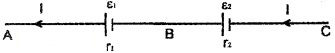
Considertwo cells in series. Let ε1, r1 be the emf and internal resistance of first cell. Similarly ε2, r2 be the emf and internal resistance of second cell. Let I be the current in this circuit.
From the figure, the P.d between A and B
VA – VB = ε1 – 1 ………(1)
Similarly P.d between B and C
VB – VC = ε2 – 1 ……..(2)
Hence, P.d between the terminals Aand C
VAC = VA – VC = VA – VB + VB – VC
VAC = [VA – VB] + [VB – VC]
when we substitute eqn. (1) and (2) in the above equation.
VAC = ε1 – Ir1 + ε2 – Ir2
VAC = (ε1 – ε2) – I(r1 + r2)
VAC = εeq – Ireq
where εeq = ε1 + ε2, and req = r1 + r2
Question 7.
The resistance value of a conductor depends on its physical dimensions.
1. Give the expression for resistance of a conductor in terms of its physical dimension.
2.

(l length and d diameter) A potential V is applied between the ends of two-conductor of same material shown in the figure.
- Express the resistance of the second conductor in terms of the resistance of the first conductor. (1)
- Find the ratio of electric field across the two conductors.
Answer:
1. R = ρ \frac{1}{A}
l – length of the conductor and
A – Area of cross-section
2.
a.
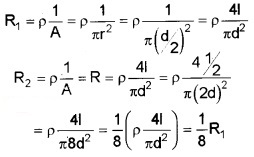
b.
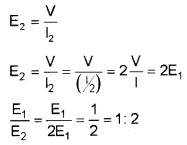
Question 8.
Three resistors R1, R2, R3 are to be combined as shown in the figure.
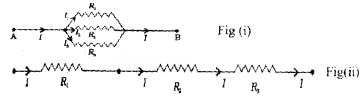
- Identify the series and parallel combinations. (1)
- Which combination has lowest resistance. Arrive at the expression for the effective resistance of this combination. (3)
Answer:
1. Fig(i) – parallel Fig. (ii) – series
2. Fig. (i)
Let V be the potential between A and B.
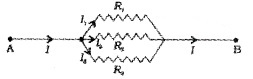
I = I1 + I2 + I3
and applying Ohm’s law to R1, R2 and R3 we get,
V= I1R1,V=I2R2,V=I3R3
So that
I = I1 + I2 + I3 = V\left(\frac{1}{R_{1}}+\frac{1}{R_{2}}+\frac{1}{R_{3}}\right)
An equivalent resistance Req that replace the combination, and hence
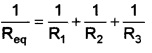
Question 9.
An electric circuit is given in Figure.
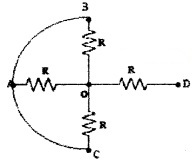
The potential difference between A and D is 40V.
- Find the effective resistance between A and D in terms of R. (1)
- Calculate the potential difference between A and O.
Answer:
1.
![]()
2. VAD = VAO + VOD
But VOD = 3VAO
∴ VAD = VAO + 3VAO
40 = 4VAO
VAO = \frac{40}{4} = 10V.
Plus Two Physics Current Electricity Five Mark Questions and Answers
Question 1.
Resistance are used to reduce the current flow in a circuit.
1. A carbon resistor has coloured strip and shown in the figure. What is its resistance?

2. Resistance can be connected in series and parallel to obtain the required value of resistance. Derive an expression for the effective resistance when three resistors are connected in parallel.
3. Kirchhoff’s rules are used to analyses the electric circuit. Use it to analyze the Wheatstone Bridge and arrive at Wheatstone’s condition for balancing the bridge.
Answer:
1. blue, Gray, yellow, gold
1st – Blue – 6
IInd – Gray – 8
IIIrd – Yellow – 104
Iv – gold – 5%
68 × 104 ± 5%.
2. Derive an expression for effective resistance in series:
Consider three resistors R1, R2 and R3 connected in series and a pd of V is applied across it.
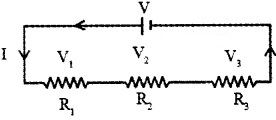
In the circuit shown above the rate of flow of charge through each resistor will be same i.e. in series combination current through each resistor will be the same. However, the pd across each resistor are different and can be obtained using ohms law.
pd across the first resistor V1 = I R1
pd across the second resistor V2 = I R2
pd across the third resistor V3 = I R3
If V is the effective potential drop and R is the effective resistance then effective pd across the combination is
V = IR
Total pd across the combination = the sum pd across each resistor, V = V1 + V2 + V3
Substituting the values of pds we get IR = IR1 + IR2 + IR3
Eliminating I from all the terms on both sides we get
R = R1 + R2 + R3 ………(1)
Thus the effective resistance of series combination of a number of resistors is equal to the sum of resistances of individual resistors.
3. Wheatstone’s Bridge:
Four resistances P,Q,R and S are connected as shown in figure. Voltage ‘V’ is applied in between A and C. Let I1, I2, I3 and I4 be the four currents passing through P,R,Q and S respectively.
Working:
The voltage across R
When key is closed, current flows in different branches as shown in figure. Under this situation
The voltage across P, VAB = I1P
The voltage across Q, VBC = I3Q ……(1)
The voltage across R, VAD = I2R
The voltage across S, VDC = I1S
The value of R is adjusted to get zero deflection in galvanometer. Under this condition,
I1 = I3 and I2 = I4……(2)
Using Kirchoff’s second law in loopABDA and BCDB, weget
VAB = VAD ……….(3)
and VBC = VDC …….. (4)
Substituting the values from eq(1) into (3) and (4), we get
I1P = I2R……….(5)
and I3Q = I4S……..(6)
Dividing Eq(5) by Eq(6)
\frac{I_{1} P}{I_{3} Q}=\frac{I_{2} R}{I_{4} S}
\frac{P}{Q}=\frac{R}{S} [since I1 = I3 and I2 = I4]
This is called a Wheatstone condition.
Question 2.
A rectangular conductor of length I and area of cross-section A and electron density n; is shown below.

- When the face Y is given positive potential and X negative potential what will happen to the electrons inside the block
- What is meant by drift velocity? How is it related to the field inside the metal?
- Deduce an expression connecting intensity of electric field and drift velocity.
- Under the application of an electric field do all the electrons move in a same direction? Explain
Answer:
1. Electrons will be accelerated towards the side Y.
2. Drift Velocity (vd):
The average velocity acquired by an electron under the applied electric field is called drift velocity.
Explanation :
When a voltage is applied across a conductor, an electric filed is developed. Due to this electric field electrons are accelerated. But while moving they collide with atoms, lose their energy and are slowed down. This acceleration and collision are repeated through the motion. Hence electrons move with a constant average velocity. This constant average velocity is called drift velocity.
3. Expression for drift velocity :
Let ‘V’ be the potential difference across the ends of a conductor. This potential difference makes an electric field E. Under the influence of electric field E, each free electron experiences a Coulomb force.
F = -eE
or ma = -eE
a = \frac{-e E}{m} ……….(1)
Due to this acceleration, the free electron acquires an additional velocity. A metal contains a large number of electrons.
For first electron, additional velocity acquired in a time τ,
v1 = u1 + aτ1
where u1 is the thermal velocity and τ is the relaxation time.
Similarly the net velocity of second, third……electron
v2 = u2 + aτ2
v3 = u3 + aτ3
vn = un + aτn
∴ Average velocity of all the ‘n’ electrons will be
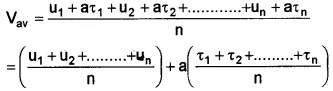
Vav = 0+ aτ (∴ average thermal velocity of electron is zero)
where
![]()
where Vav is the average velocity of electron under an external field. This average velocity is called drift velocity.
ie. drift velocity Vd = aτ……(2)
Substitute eq (1) in eq (2)
Vd = \frac{-e E}{m} τ
4. Electrons will continues its random thermal motion even in the presence of electric field.
Question 3.
To study the relation between potential difference and current in an electrical circuit, a student is provided with a resistance wire, a cell, and a key.
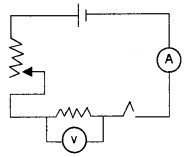
1. Draw a circuit which allows current flow through the resistance wire.
2. Modify the circuit by introducing an ammeter, Voltmeter and a rheostat for varying the potential difference across the resistance and to measure that potential difference and the corresponding current.
3. Let in the above experiment the student obtained the following data.
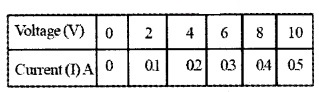
Draw a graph connecting V and I using above data. Then establish the relation between V and I as a law.
4. Instead of the resistance wire if the student uses a p-n junction diode in the forward biased condition how the relation between V and I changes? Justify.
Answer:
1.
2.
3.
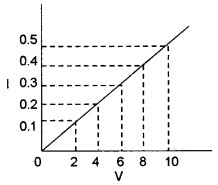
The above graph shows that current flowing through a conductor is directly proportional to potential difference across it ends.
4. The relation between V and I becomes nonlinear. Because Pn diode does not obey ohms law.
Question 4.
In all metallic conductors, electric conduction is due to drifting of free electrons. But the resistivity of different metals are different.
- Write the expression for resistivity of a conductor in terms of its dimensions.
- Name the factors on which resistivity of a metal depends.
- Arrive at an expression for electrical resistivity of a metal in terms of relaxation time.
- Using the above expression explain the variation of resistivity with temperature.
Answer:
1. ρ = \frac{RA}{l}.
2. Temperature and Nature of metal
3. We know current density
J = nvde
But Vd = \frac{e E}{m} τ
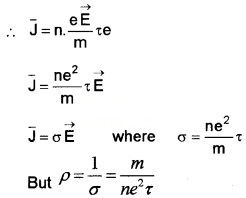
4. When temperature increases, the amplitude of oscillation of atom increases. This will decrease the relaxation time and hence resistivity increases.
Question 5.
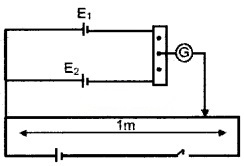
You are supplied with a 1m long uniform resistance wire of resistance 3Ω and a cell of emf 1.5v.
- Can you construct a potentiometer using 1m wire? If no, give reason; if yes, what is the least count of the arrangement?
- Draw the connection diagram to compare the emf of Leclanche cell and Daniel cell using this arrangement.
- How can you modify above arrangement to measure a p.d. in the range 0-1 mV with a least count of 0.15m V.
Answer:
1. Yes, LC is 1.5mV
2.
3. To get least count of 0.15mV, 10m wire has to be used.
Question 6.
A circuit diagram is given below. Analyze the figure and answer the following questions.
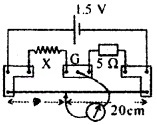
- The above circuit is a modification of……..
- What is the value of balancing length?
- If 1.5 V cell is replaced by a 3V cell what will be the balancing length?
- Calculate the value of X.
Answer:
1. Wheatstone bridge.
2. 100-20=80cm
3. No change in balancing length
4. P/Q = R/S
ie X/5 = 80/20
x = 80/20 × 5 = 20Ω.
Question 7.
- Define electric power. What is its SI unit? (2)
- Two bulbs of 50W, 220V and 100W, 220V are given. How will you connect the bulbs so that 50W, 220V bulb will glow brighter than 100W, 220V bulb. (1)
- When the bulb 50W, 220V is connected to a 110V supply calculate the power generated. (2)
Answer:
1. The energy dissipated per unit time is power.
P = \frac{\Delta \mathrm{W}}{\Delta \mathrm{t}}
P = IV =I2R = \frac{V^{2}}{R}
Unit is watt.
2. In series
![]()
3.
![]()
Question 8.
The figure shows the diagram of a potentiometer.
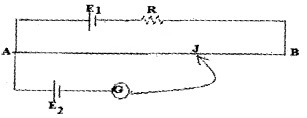
1. Give the principle of a potentiometer. (1)
2. The length of AB is 3m and resistance per unit length of the potentiometer wire is 4Ω/m. If E1 = 4V, R = 20Ω and E2 = 1V find the length of the potentiometer wire that balance E2. (3)
3. If E2>E1 can we get the null deflection in galvanometer. Give reason. (1)
Answer:
1. When a constant current is flowing through a wire having uniform area of cross section and uniform composition the potential difference across any length of the wire is directly proportional to its length.
V ∝ l.
2. The resistance of potentiometer
Rp = 4 × 3 = 12Ω
Current through the potentiometer
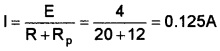
Potential across potentiometer wire
VAB = IRp = 0.125 × 12 = 1.5V
Potential gradient k = \frac{V_{A B}}{I}=\frac{1.5}{3} = 0.5 V/m
Balancing length for cell E2 is given from equation
E2 = kI2 = 0.5I2
![]()
3. If E2 > E1, we will not get null deflection. The potential difference across the potentiometer wire AB should be higher than the emf of E2.
Question 9.
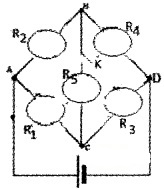
- When the switch is closed will all the bulbs glow? Give reason. (2)
- Identify the underlying principle. Deduce the principle for a resistance network. (3)
Answer:
1. No. Since all the bulbs are identical the bridge is balanced. So potential B and C is same and no current flows through the bulb connected between B and C. So all other bulbs except R5 will glow.
2. Four resistances P, Q, R, and S are connected as shown in figure. Voltage ‘V’ is applied in between A and C. Let I1, I2, I3, and I4 be the four currents passing through P, R, Q, and S respectively.
We hope the Kerala Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity help you. If you have any query regarding Kerala Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity, drop a comment below and we will get back to you at the earliest.
