Plus Two Physics Chapter Wise Previous Questions Chapter 3 Current Electricity is part of Kerala Plus Two Physics Chapter Wise Previous Questions and Answers Kerala. Here we have given Plus Two Physics Chapter Wise Questions and Answers Chapter 3 Current Electricity.
Kerala Plus Two Physics Chapter Wise Previous Questions Chapter 3 Current Electricity
Question 1.
A potentiometer wire of length 100 cm has a resistance of 10 ohms. It is connected in series with a resistance R and a cell of emf 2V and negligible internal resistance. The circuit is as shown below. (March – 2009)
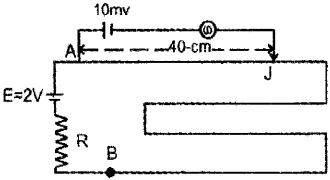
p) What is the principle of a potentiornèter?
q) Why is it prefered over a voltmeter?
r) What is the resistance of 40 cm length of the potentiometer wire?
s) If a source of emf 10 millivolts is balanced by a length of 40cm of the potentiometer wire, find the value of the external resistance
t) While performing an experiment on potentiometer what precautions (at least tow) one must observe in the laboratory?
Answer:
p) The potential difference between two points of a current-carrying conductor is directly proportional to the length of wire between two points.
q) Potentiometer measures emf without drawing current from the cell (null method)
r) Resistance per unit length = 10Ω/100cm = 0.1Ω/cm Resistance of 40cm wire = 40 x 1 = 4Ω
s) The current through wire, i=2(10+R)i=2(10+R) Potential drop across 40cm wire =8(10+R)=8(10+R)
Hence 8/10 + R = 10 mv ∴ R = 790Ω
t) Primary circuit should not be changed during the measurement, or The primary emf should be greater than emf of celt to be measured.
Question 2.
Copper is one of the suitable material to make connecting wires due to its low resistivity. (March -2010)
a) What do you mean by resistivity?
b) A copper wire is in the form of a cylinder and has a resistance R. It is stretched till its thickness reduces by half of its initial size. Find its new resistance in terms of R.
Answer:
a) Resistivity is the resistance of a conductor having unit length and unit area cross-section.
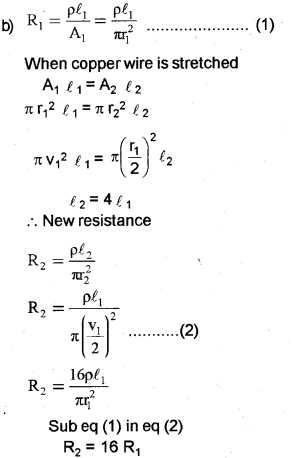
Question 3.
a) A carbon resistor of 58 kΩ is marked with rings of different colours for identifying it. What will be the sequence of colours on this carbon resistor? (Say – 2010)
b) Suppose two wires – one made of copper and another made of Germanium are given to you. If you heat these wires, what happens to their resistivity? Give reasons (Assuming there is no change in dimension of wires)
c) Five equal resistances each of value ‘R’ Ω are connected as a network shown below:
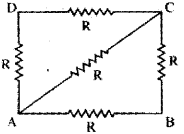
Find the equivalent resistance between point marked ‘A’ and ‘B’.
d) State and explain Kirchoff’s junction rule of an electrical network.
Answer:
a) Green, Gray, Orange
b) Resistivity of copper increases with temperature. But for germanium, it decreases.
Explanation: When temperature increase, the relaxation time decreases for metals. As a result resistivity increases.
More and more electrons become free in germanium with temperature. Hence resistivity decreases with temperature in the semiconductor.
c) The resistance RAD and RDC are in series R = R + R = 2R
This is in parallel with RAC

d) First law (Junction rule)
The total current entering the junction is equal to the total current leaving the junction.
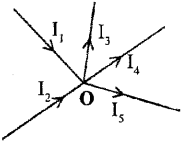
Consider a junction ‘O’ Let l1 and l2 be the incoming currents and l3, l4 and l5 be the outgoing currents.
According to Kirchoff’s first law, l1 + l2 = l3 + l4 + l5
Question 4.
Remya makes the following circuit to measure the emf of a cell. (March – 2011)
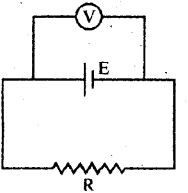
She says that the voltmeter reading will give the emf of the cell.
a) The Physics teacher says that it is not possible to measure the emf of the cell in this way. Justify this statement.
b) Explain with a cupuit diagram the method to measure the emf of a cell with a potentiometer if you are given another standard cell. (Whose e.m.f. is known)
OR
The thermal velocity of electron is about 106 m/s and drift velocity is about 10-3m/s.
a) What do you mean by drift velocity?
b) Obtain a relation between drift velocity and electric current.
Answer:
a) In this circuit, voltmeter draws current. Hence we do not get emf of the cell,
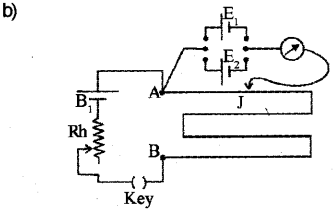
Construct a circuit as shown in the figure. Key in primary circuit is closed and then E., is put into the circuit and balancing length A, is found out.
Then, E1 α l1 _________ (1)
Similarly E2 is put into the circuit and balancing length (l2) is found out.
Then, E2 αl2 _________ (2)
Dividing Eq (1) by Eq (2),
E1E2=l1l2E1E2=l1l2 _________ (3)
OR
a) The average velocity gained by electrons in a conductor when an electric field is applied is called drift velocity.
b) Relaxation time is the average of the time between two successive collisions of the free electrons with atoms.
c) Consider a conductor of cross-sectional area A. Let n be the number of electrons per unit volume. When a voltage is applied across a conductor, an electric filed is developed: Let vd be the drift veloc-ity of electron dye to this electric field.
Total volume passed in unit time = Avd
Total number of electrons in this volume = Avdn
Total charge flowing in unit time = Avdne
But charge flowing per unit time is called current I ie. current, I = Avdne I = neAvd
Question 5.
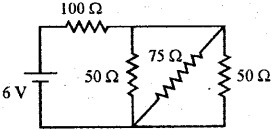
a) Find the equivalent resistance of the above circuit.
b) Determine the current through the 75 W resistance.
Answer:
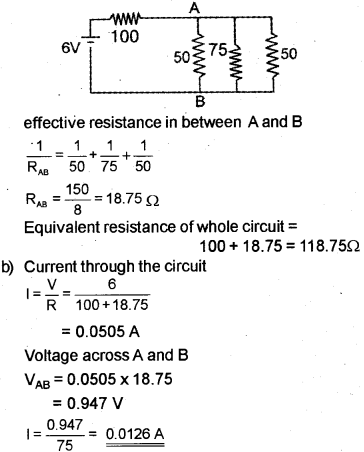
a) The circuit can be redrawn like this
Question 6.
Colour code of the carbon resistor is shown in the figure. What is the value of this resistance with tolerance? (Say – 2011)
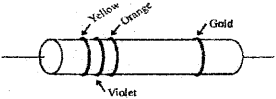
Answer:
47×103Ω±5%47×103Ω±5%
Question 7.
In the circuit shown below what would be the amme ter and voltmeter readings if the key K is dosed?
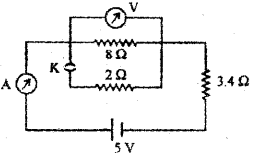
Answer:
Effective resistance of 8Ω and 2Ω is
Reft =R1R2R1+R2=8×28+2=1610=1.6ΩReft =R1R2R1+R2=8×28+2=1610=1.6Ω
∴ Total resistance t9 the circuit = 1.6Ω + 3.4Ω = 5Ω
Current in the circuit, I=VR=55=1 AI=VR=55=1 A
Voltage across combination of 8Ω, 2Ω is V = IR = I x 1.6 = 1.6V
Ammeter shows IA and voltmeter shows 1.6V.
Question 8.
A cell consists of electrodes and electrolyte. When it is connected to an external circuit it sends a current through it.
a) What is meant by internal resistance of a cell?
b) What happens to the terminal voltage of the cell when it sends a current through the external circuit?
c) Write down the relation fro the determination of internal resistance of a cell using a potentiom¬eter and explain the symbols.
Answer:
a) Internal resistance is the-resistance offered by electrolyte to current flow, b) When a cell sends current through external current, terminal voltage will be less than e.m.f. V = ∈ – Ir
c) r=R(ℓ1−ℓ2)ℓ2r=R(ℓ1−ℓ2)ℓ2
R → external resistance l1 and l2 are balancing length without or with external resistance.
Question 9.
a) The resistance ‘R’ of a conductor depends on its length T, area of cross-section ‘A’ and the resistivity of the material ‘r’. The correct expression connecting R, I, A and ‘r’ is (March – 2012)
1)R=ρAℓii)R=Aℓρiii)R=ρℓAiv)R=ρAℓ1)R=ρAℓii)R=Aℓρiii)R=ρℓAiv)R=ρAℓ
b) The voltage-current graphs for two resistors of the same material and same radii with lengths L1 and L2 are shown inihe figure. If L1 > L2 state with reason, which of these graphs represents voltage-current change for L1?
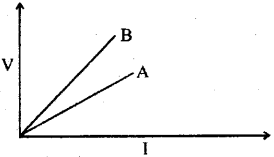
Answer:
a) R=ρℓAR=ρℓA
b) Slope of V – I graph gives the resistance of the material. In this graph slope of B is greater than A. ie. RB > RA. Hence the graph B represents voltage current change for L1.
Question 10.
In the potentiometer circuit shown, the balance point is at X.
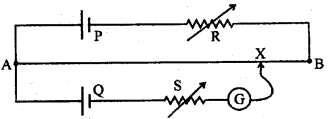
State with reason, where the balance point will be shifted, when
a) Resistance R is increased, keeping all param eters unchanged.
b) Resistance S is increased, keeping R constant.
c) Cell P is replaced by another cell whose e.m.f. is lower than that of cell Q.
d) You are asked to compare the e.m.f of two cells using a potentiometer. Draw the circuit diagram for this and explain how you will determine the ratio of e.m.f.
Answer:
a) Balance point will be shifted towards B. When R increases, current through wire (AB) decreases. Hence potential gradient of wire AB decreases. Hence more length is required to bal ance the cell Q.
b) No change in balancing length. Variation ¡n resis tance s does not change potential gradient of wire AB.
c) Balance point will be shifted towards A. When we decrease the value of secondary emf, corresponding balancing length of the wire decreases because Eαl.
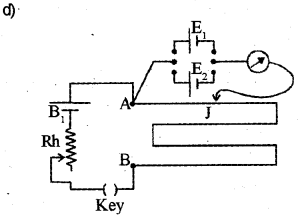
Construct a circuit as shown in the figure. Key in primary circuit is closed and then E1 is put into the circuit and balancing length I is found out. Then,E1 α l1 ………………… (1)
Similarly E2 is put into the circuit and balancing length (l2) is found out.
Then, E2 α l2 ………………… (2)
Dividing Eq (1) by Eq (2),
E1E2=l1l2E1E2=l1l2 ………………… (3)
Question 11.
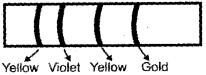
a) To construct an, electronic circuit, you want to select a 470 kW resistor with 5% tolerance. Draw a schematic diagram indicating the colour combinations that you will select. (March – 2013)
b) As the temperature of a metallic resistor is increased; the product of its resistivity and conductivity …………….
i) Increase
ii) Decreases
iii) Remains constant
iv) May increase or decrease
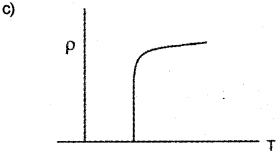
c) Draw a graph showing the relation between resistivity and temperature of a superconductor.
d) State Kirchhoff’s rules for the analysis of electrical circuits.
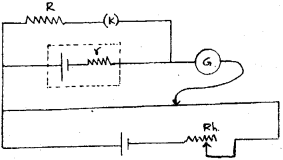
e) The circuit diagram of a potentiometer for the determination of internal resistance of a cell is shown below. Calculate the value of the internal resistance (r) of the cell.
Given R = 100Ω, balancing length when key (K)
open = 60 cm. Balancing length when key (K) closed = 58 cm.
Answer:
a) Yellow, Violet, Yellow, Gold
b) Remains constant
d) 1) First law (Junction rule)
The total current entering the junction is equal to the total current leaving the junction.
2) Second law (loop rule)
In any closed circuit the algebraic sum of the product of the current and resistance in each branch of the circuit is equal to the net emf in that branch.
OR
Total em! in a closed circuit is equal to sum of voltage drops.
e) Internal resistance r=R(ℓ2−ℓ1)ℓ1r=R(ℓ2−ℓ1)ℓ1
=100(65−58)58=3.45Ω=100(65−58)58=3.45Ω
Question 12.
The resistance ‘R’ of a conductor depends on its length T, area of cross-section ‘A’ and the resistivity ‘p’ of the material. (Say – 2013)
a) Write an expression connecting R, I, A and p.
b) What happens to the resistivity of the material if the conductor is stretched to double its length?
c) The voltage-current variation of two metallic wires ‘X’ and ‘Y’ at constant temperature are as shown in fig.
Assuming that the wires have the same length and the same diameter, explain which of the wires will have larger resistivity.

d) Three resistances are connected to a battery as shown in the diagram. Find the value of current in the circuit.
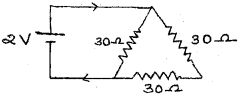
Answer:
a) R=ρLAR=ρLA
b) Resistivity does not change with dimension of material.
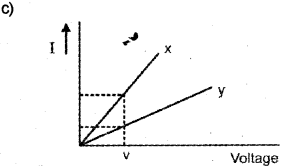
For a given voltage X gives more current. Hence resistance of X is smaller than Y. ie, Resistance of Y is greater than that of X. So resistivity of Y is greaterthanthatofX.
d) The given circuit can be redrawn as
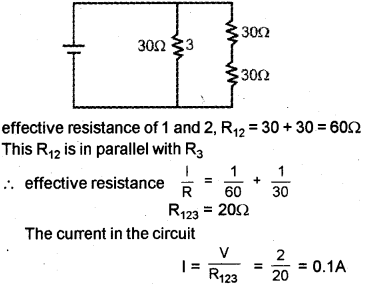
effective resistance of 1 and 2, R12 = 30 + 30 = 60Ω This R12 is in parallel with R3
Question 13.
Kirchhoff’s rules are very useful for the analysis of complicated electric circuits.
a) State Kirchhoff’s junction rule and loop rule.
b) Draw circuit diagram of Wheatstone bridge.
a) Kirchhoff’s first rule
The total current entering the junction is equal to the total current leaving the junction.
Kirchhoff’s second law
Total emf in a closed circuit is equal to sum of voltage drops.
b) Refer Section 3.14
Question 1.
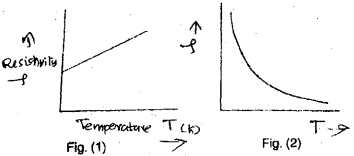
a) From the above figures which one corresponds to a typical semiconductor. (March – 2014)
b) Dimension of temperature coefficient a is
c) Steady current flows in a metallic conductor of the nonuniform cross-section. Which of the following quantities is a constant along the conductor?
A) Current
B) Current density
C) Electric field
D) Drift speed
Answer:
a) Fig. (2)
b) K-1 or θ-1
c) Current (or A)
d) Because current is independent of area. All other quantities depend on area of cross-section.
Question 14.
Resistors are sometimes joined together and they have several applications in electronics.
a) Draw a series combination of three resistors R1, R2 and R3.
b) Obtain the expression forthe effective resistance in the combination of two resistors R1 and R2 in parallel. Sketch the combination also.
c) Inspite of the validity of Ohm’s law, it has some limitations. Give one limitation of Ohm’s law.
Answer:
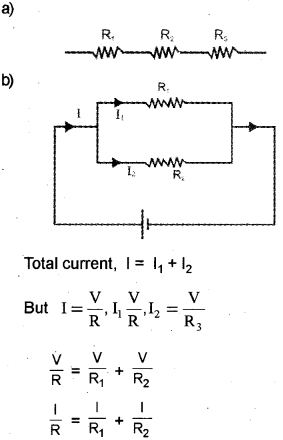
c) Limitations of Ohm’s law
- For certain materials, is not proportional to I.
- The relation between V and I depends on the sign of I.
- There will be more than one value of Vforsame current.
Question 15.
In an electrical circuit resistances can be connected in series or parallel or combination of both. (Say – 2014)
a) If R1 and R2 are two resistors connected in series what is their effective resistance.
b) What is the effective resistance when they are connected in parallel.
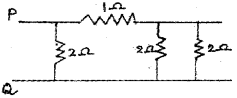
c) Calculate the effective resistance between the terminals P and Q in the above diagram
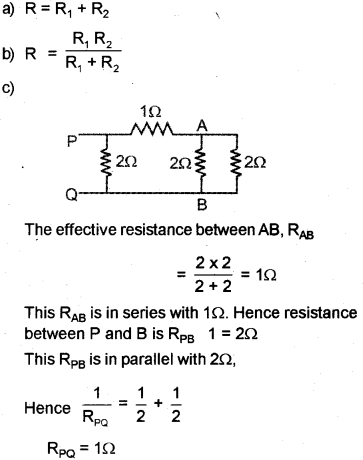
Answer:
Question 16.
You are given a cell whose internal resistance is to be calculated.
a) Which instrument do you use?
b) Draw the circuit diagram for measuring the inter¬nal resistance using this instrument.
c) Obtain an expression for calculating the internal resistance.
Answer:
a) Potentiometer
b) Refer March 2015, Q.No. 1(c)
c) Refer March 2015, Q.No. 1(c)
Question 17.
a) Potentiometer measures the potential difference more accurately than a voltmeter because of the potentiometer. (March – 2015)
a) does not draw current from external circuit.
b) has a wire of high resistance.
c) draws a heavy current from external circuit.
d) has a wire of low resistance.
b) With the help of a diagram explain the principle of a potentiometer.
c) Using a potentiometer how do you determine the internal resistance of a cell?
Answer:
a) a
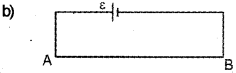
AB is a resistance wire. Which is connected to a cell as shown in the figure. If current through AB is constant, potential difference between any two points of wire AB will be proportional to length between two points.
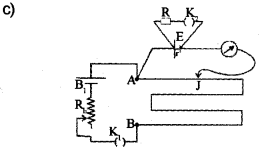
Construct a circuit as shown in the figure. The key (K1) is the secondary (K2) is open. Jockey is moved to get zero deflection in galvanometer. The balancing lengh (l1) is found out.
Then we can write.
E1 α l1 …………………….. (1)
Key K2 is put in the circuit, corresponding balancing length (l2) is found out. Let V be the applied voltage, then
Vαl1 ………………………….. (2)
‘V’ is the voltage across resistance box.
Current through resistance box I=ER+rI=ER+r
ie, voltage across resistance,
V=ER(R+r)V=ER(R+r) …………………….. (3)
Substituting eq (3) in eq (2),
ER(R+r)αl2ER(R+r)αl2
Dividing eq (1) by eq (4),
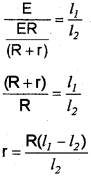
Question 18.
Kirchhoff’s rules are very useful for analysis of electrical circuits.
a) State Kirchhoff’sjunction rule.
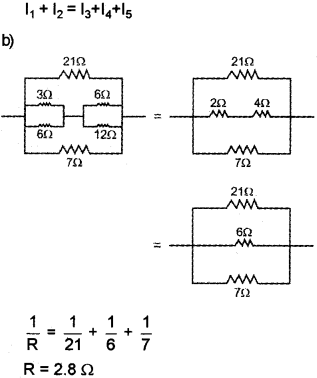
b) Find the effective resistance of the circuit given below:
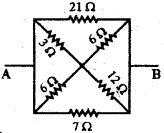
Answer:
1) First law (Junction rule)
The total current entering the junction is equal to the total current leaving the junction.
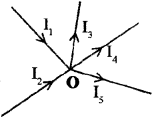
Consider a junction ‘O’ Let l1 and l2 be the incoming currents and l3, l4 and l5 be the outgoing currents.
According to Kirchoff’s first law,
Question 19.
The following question has a choice: (March – 2016)
a) Which of the following obeys Ohm’s law?
i) Transistor
ii) Nichrome
iii) Diode
iv) Liquid electrolyte
b) A wire has a resistance of 10Ω. It is stretched by 10% of its original length, what will be the new resistance?
i) 10Ω
ii) 11Ω
iii) 9Ω
iv) 12.1Ω
c) With the help of a circuit diagram describes the method to find the value of an unknown resistance using meter bridge arrangement.
OR
a) Which of the following material is used to make wire wound standard resistors?
i) Manganin
ii) Germanium
iii) Copper
iv) Carbon
b) A bread toaster and a bulb are connected parallel in a circuit. The toaster produces more heat than the bulb. Which of the following statements is true?
i) Resistance of toaster is greater than resis¬tance of bulb.
ii) Resistance of bulb is same as the resistance of toaster.
iii) Resistance of bulb is greater than resistance of toaster.
iv) Cannot predict.
c) With the help of a circuit diagram describe the method to find the internal resistance of a cell using potentiometer.
Answer:
a) (ii) Nichrome
b) (iv) 12.1Ω
c) Working : A suitable resistance R is taken in the box. The position of jockey is adjusted to get zero deflection.
If T is the balancing length from A, using Wheatstone’s condition,
XR=lr(100−l)r Or X=Rl(100−l)XR=lr(100−l)r Or X=Rl(100−l)
knowing R and /, we can find X (resistance of wire)
OR
a) Manganin
b) iii) Resistance of the bulb is greater than the resistance of toaster.
c) Refer March 2015, Q.No. 1c
Question 20.
Vector form of a lawman be written as ˉJ¯J = aˉE¯E (Say – 2016)
The above equation is an equivalent form of a famous law. Name the law.
i) Biot-Savart’s law
ii) Ohm’s law
iii) Coulomb’s law
iv) Gauss’s law
Answer:
ii) Ohm’s law
Question 21.
a) Table given below shows the current (I) voltage (V) relationship of a device.
| Voltage (V) | Current (I) |
| 1 | 20 |
| 2 | 30 |
| 3 | 35 |
| 4 | 50 |
| 5 | 55 |
| 6 | 68 |
Draw V-l graph. With the help of the graph explain whether the device is showing ohmic or non-ohmic behaviour.
b) Name two devices which do not obey Ohm’s law.
Answer:
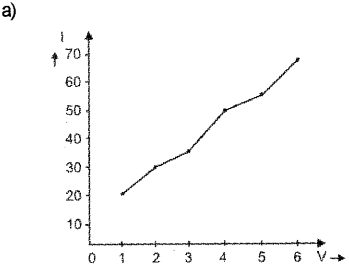
This material shows non-ohmic behaviour,
b) Diode, transistor
Question 22.
a) Resistivity of a conductor depends upon (March – 2017)
i) its material
ii) its cross-sectional area
iii) its length
iv) All of the above
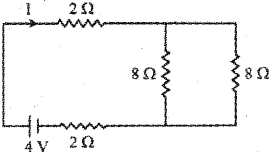
b) Calculate the current flowing through the following circuit:
c) A potentiometer is a device to measure emf of a cell. Explain how the emfs of two cells can be compared using a potentiometer.
Answer:
a) i) Its material
b) Effective resistance,
R=2Ω+2Ω+(8×88+8)Ω=2+2+4=8Ω
Current, 1=VR=48=12=0.5A
c) Refer section 3.16
Question 23.
A resistance R is connected to a cell of EMF E and has an internal resistance r as shown in the figure. The voltage across R is measured using and ideal voltmeter and circuit current is measured by an ammeter. A rheostat of negligible resistance is used to vary the current in the circuit. (SAy – 2017)
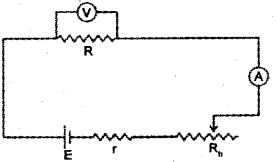
a) Write down the expression for current in the circuit and voltage across R at any instant.
b) Draw a graph connecting current in the circuit along X-axis and voltage across R along Y-axis.
c) The quantity obtained from the slope of the graph is ……………………….. .
Answer:
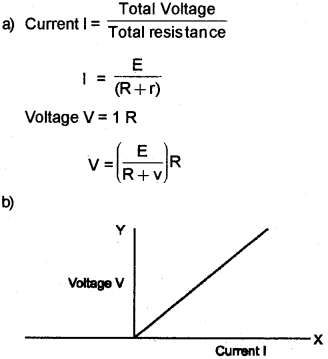
c) Slope of this graph gives resistance R.
We hope the Kerala Plus Two Chemistry Chapter Wise Questions and Answers Chapter 3 Current Electricity help you. If you have any query regarding Kerala Plus Two Chemistry Chapter Wise Questions and Answers Chapter 3 Current Electricity, drop a comment below and we will get back to you at the earliest.
