Plus Two Microeconomics Notes Chapter 3 Production and Costs

Kerala Plus Two Microeconomics Notes Chapter 3 Production and Costs

Production Function

Production function explains the technological relationship between input and output. It is a process by which the inputs are transformed into output. The factors used in production process are known as factors of production. Land, labour, capital and organization are the major factors of production. Every unit of production uses various inputs and tries to maximize the output in the production. The production is closely associated with technology. When the technology is very modern, the firm can produce higher levels of output even with the same level of input. Therefore, production function can be defined as the maximum output that can be produced by combining various inputs with the given level of technology.

ഉല്പാദന ധർമ്മം: നിവേശങ്ങളും, ഉല്പാദനവും തമ്മിലുള്ള ബന്ധ ത്ത യാണ് ഉല്പാദന ധർമ്മം എന്ന് വിളിക്കുന്നത്. (Production function) അതായത് നിവേശങ്ങളെ ഉല്പന്നങ്ങ ളാക്കി മാറ്റുന്ന പ്രക്രിയയെയാണ് ഉല്പാദനം എന്ന് വിളിക്കുന്ന ത്. ഉല്പാദന പ്രക്രിയയിൽ ഉപയോഗിക്കുന്ന നിവേശങ്ങളെ ഉല്പാ ദന ഘടകങ്ങൾ (Factors of Production) എന്ന് വിളിക്കുന്നു. ഉദാഹരണമായി ഭൂമി, തൊഴിൽ, മൂലധനം, സംഘാടനം എന്നിവ യാണ് ഉല്പ്പാദന ഘടകങ്ങൾ. ഒരു നിശ്ചിത സാങ്കേതികവിദ്യ ഉപ യോഗിച്ച് പരമാവധി ഉല്പാദനം നടത്താൻ കഴിയുന്ന നിവേശ ങ്ങളുടെ സംയോഗത്തെ ഉല്പാദന ധർമ്മം എന്ന് വിളിക്കുന്നു.

Isoquant

Isoquant or iso-product curve is a curve joining all the input combinations that gives equal and maximum level of output. Isoquants are also known as production indifference curve. A collection of isoquants are known as isoquant map. Isoquants are normally slopes downwards from left to right.

സമ ഉല്പന്ന വക്രം: തുല്യവും, പരമാവധി ഉല്പാദനം ലഭിക്കു ന്നതുമായ രണ്ട് നിവേശങ്ങളുടെ വ്യത്യസ്ത സംയോഗ ബിന്ദുക്കൾ യോജിപ്പിച്ചുകൊണ്ട് വരയ്ക്കുന്ന വകത്തെ സമ ഉല്പ്പന്ന വക്രം (isoquant) എന്ന് പറയുന്നു. ഒരു സമ ഉല്പന്ന വകത്തിലെ എല്ലാ ബിന്ദുക്കളിലെയും ഉല്പന്നത്തിന്റെ അളവ് ഒന്നായിരിക്കും. ഉയർന്ന സമ ഉല്പന്ന വക്രം കാണിക്കുന്നത് ഉയർന്ന ഉല്പാദന ത്തെയും, താഴ്സ്. സമ ഉല്പന്ന വകം കാണിക്കുന്നത് താഴ ഉല്പാദനത്തെയും ആണ്. സമ ഉല്പന്ന വക്രം സാധാരണയായി മുകളിൽനിന്ന് താഴോട്ട് ചെരിഞ്ഞായിരിക്കും.

Short run and the long run

The time span used for production can be classified into short run and long run. In short run a producer changes the level of output only by varying one input and keeping other inputs fixed. Long run is a period of time in which the firm can vary all the inputs for a change in output. Short run is a period when at least one of the factors of production is fixed. Long run is a period when all factors of production become variable.

ഹ്രസ്വകാലയളവും ദീർഘകാലയളവും: ഉല്പാദകന് ഉല്പാദന പ്രക്രിയയിൽ ഉപയോഗിക്കുന്ന ഉല്പാദന ഘടകങ്ങളിൽ മാറ്റം വരുത്താൻ സാധിക്കുന്ന സമയക്രമത്തിന്റെ അടിസ്ഥാനത്തിൽ സമയ ദൈർഘ്യത്തെ രണ്ടായി തിരിക്കാം.

1. ഹ്രസ്വ കാലയളവ് (Short run)
2. ദീർഘ കാലയളവ് (Long run)

ഉല്പാദനത്തിന്റെ അളവിൽ മാറ്റം വരുത്താൻ ഒരു ഉല്പാദകന് എല്ലാ നിവേശങ്ങളിലും മാറ്റം വരുത്താൻ കഴിയാത്ത കാലയള വിനെ ഹ്രസ്വകാലയളവ് എന്നു പറയുന്നു. അതായത് ഹസ്വ കാലയളവിൽ ചില നിവേശങ്ങളുടെ അളവിൽ മാറ്റം വരുത്താൻ ഒരു ഉല്പാദന യൂണിറ്റിന് കഴിയുകയില്ല. അങ്ങനെ അളവിൽ മാറ്റമുണ്ടാക്കാൻ കഴിയാത്ത നിവേശങ്ങളെ സ്ഥിര നിവേശങ്ങൾ എന്ന് വിളിക്കുന്നു.

ഉല്പന്നത്തിന്റെ അളവിൽ മാറ്റം വരുത്താൻ ഉല്പാദകന് എല്ലാ നിവേശങ്ങളിലും മാറ്റം വരുത്താൻ കഴിയുന്ന കാലയളവിനെ ദീർഘ കാലയളവ് എന്ന് പറയുന്നു. ദീർഘ കാലയളവിൽ നിവേ ശങ്ങളുടെ അളവിൽ മാറ്റം വരുത്തി ഉല്പാദനത്തിന്റെ അളവിൽ മാറ്റമുണ്ടാക്കാൻ ഉല്പാദകന് കഴിയും. അങ്ങനെ അളവിൽ മാറ്റ മുണ്ടാക്കാൻ കഴിയുന്ന നിവേശങ്ങളെ വിഭേദക നിവേശങ്ങൾ (Variable inputs) എന്ന് വിളിക്കുന്നു.

Total Product, Average Product and Marginal Product

Total Product:
Consider a short run production function, where one input is variable keeping all the other inputs constant. Total Product (TP) refers to the total output when one input is varied keeping all other inputs fixed. Total Product is also known as Total Physical Product (TPP).

Average Product:
Average product (AP) is the output per unit of variable input. Average Product is derived by dividing the number of variable inputs used. Average Product (AP) = TP/X , where TP = Total Product and X = Number of variable inputs used.

Marginal Product:
Marginal Product (MP) refers to the change in total product when we change the variable input by one unit keeping all the other inputs fixed. Marginal Product shows the change in Total Product.
MP = \(\frac{\Delta \mathrm{TP}}{\Delta \mathrm{X}}\)

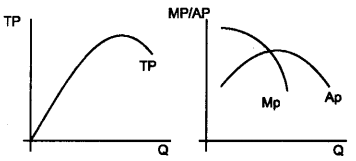
TP, AP and MP. Tp increases at an increasing rate initially, then reaches the maximum thereafter starts falling.

മൊത്തം ഉല്പന്നം ആദ്യം വർധിച്ച തോതിൽ വർധിക്കുകയും ക്രമേണ വർധനവിന്റെ നിരക്ക് കുറയുകയും പരമാവധി എത്തു കയും അതിനുശേഷം കുറയുകയും ചെയ്യുന്നു.

Both Ap and Mp are inverse u shape. Mp will always pass through the maximum point of Ap.

Apയും Mpയും റ ആകൃതിയിലാണ്. Mp എപ്പോഴും കടന്നു പോകുന്നത് Apയുടെ ഏറ്റവും ഉയർന്ന പോയിന്റിലൂടെയാണ്.

മൊത്തം ഉല്പന്നം, ശരാശരി ഉല്പന്നം, സീമാന്ത ഉല്പന്നം

മൊത്തം ഉല്പന്നം (TP): മറ്റെല്ലാ നിവേശങ്ങളും സ്ഥിരമാക്കി നിർത്തിക്കൊണ്ട് ഒരു വിഭേദക നിവേശത്തിന്റെ അളവിൽ മാത്രം മാറ്റം വരുത്തുമ്പോൾ ഉണ്ടാകുന്ന ആകെ ഉല്പാദനത്തിനാണ് മൊത്തം ഉല്പന്നം എന്ന് പറയുന്നത്.

ശരാശരി ഉല്പന്നം (AP) : വിഭേദക നിവേശത്തിന്റെ ഒരു യൂണി റ്റിൽ നിന്ന് ലഭിക്കുന്ന ഉല്പന്നത്തയാണ് ശരാശരി ഉല്പന്നം എന്ന് പറയുന്നത്.
അതായത് AP = \(\frac{\mathrm{TP}}{\mathrm{X}}\)
TP = മൊത്തം ഉല്പന്നം
x = വിഭേദക നിവേശത്തിന്റെ അളവ്

സീമാന്ത ഉല്പന്നം (MP): ഒരു വിഭേദക നിവേശത്തിന്റെ അളവ് ഒരു യൂണിറ്റ് കൂടുതലായി ഉപയോഗപ്പെടുത്തുമ്പോൾ അധിക മായി ഉല്പന്നത്തിലുണ്ടാകുന്ന വർദ്ധനവിനെ സീമാന്ത ഉല്പന്നം എന്ന് പറയുന്നു.
അതായത്, MP = \(\frac{\Delta T P}{\Delta X}\)

The Law of Variable Proportions

When more and more units of a variable input are added with the fixed input, the marginal product would increase only upto a certain point. Thereafter, the marginal product declines. This phenomenon is known as the Law of Variable Proportions. It is also known as returns to a factor.

The shape of TP, AP and MP suggests that they are . specifically passing through three phases. They are:
First phase: In the first stage, both AP and MP increase. As a result TP also increases at an increasing rate. This stage is known as the stage of increasing return to a factor. AP reaches the maximum level in this stage.

Second phase: Both AP and MP decrease at this stage. The TP increases at a decreasing rate. More importantly, TP reaches maximum and MP touches zero. This stage is also known as the stage of diminishing returns to a factor.

Third phase : At this stage, the MP becomes negative. As a result, TP also starts declining. The decline of AP is continuous. In the graph, when TP reaches maximum and MP touches zero. When MP becomes negative, TP starts declining. This stage is known as the stage of negative returns to a factor.

വിഭാദകാനുപാത നിയമവും അപചയ സീമാന്ത ഉല്പന്ന നിയമവും:
ഹസ്വകാലത്തിൽ ചില ഉല്പാദന ഘടകങ്ങൾ സ്ഥിരമായിരിക്കും ഒരു വിഭേദക നിവേശം കൂടുതലായി ഉപയോഗിക്കുകയും മറ്റു നിവേശങ്ങൾ സ്ഥിരമായി നിൽക്കുകയും ചെയ്യുമ്പോൾ നിവേശ ങ്ങളുടെ അനുപാതം മാറുന്നു. ഇതിനെ വിഭേദകാനുപാതം എന്ന് പറയുന്നു. മറ്റു നിവേശങ്ങൾ സ്ഥിരമാക്കി നിർത്തി ഒരു നിവേശ ത്തിന്റെ അളവിൽ മാത്രം മാറ്റം വരുത്തിക്കൊണ്ടിരുന്നാൽ സീമാന്ത ഉല്പന്നം ഒരു ഘട്ടം വരെ വർദ്ധിക്കും. അതിനുശേഷം സീമാന്ത ഉല്പന്നം കുറയും. ഇതിനെയാണ് വിദേദകാനുപാത നിയമം എന്ന് പറയുന്നത്.

മറ്റ് നിവേശങ്ങൾ സ്ഥിരമാക്കി നിർത്തി ഒരു നിവേശത്തിന്റെ അള വിൽ മാത്രം മാറ്റം വരുത്തിക്കൊണ്ടിരുന്നാൽ ഒരു പ്രത്യേക ഘട്ടം കഴിയുമ്പോൾ മൊത്തം ഉല്പന്നത്തിലെ മാറ്റത്തിന്റെ അളവ് (Marginal Product) കുറയുവാൻ തുടങ്ങും . ഇതിനെയാണ് അപചയ സീമാന്ത ഉല്പന്ന നിയമം എന്ന് പറയുന്നത്.

മൊത്തം ഉല്പന്നം, ശരാശരി ഉല്പന്നം, സീമാന്ത ഉല്പന്നം എന്നിവ വ്യക്തമായ മൂന്നു ഘട്ടങ്ങളിലൂടെയാണ് കടന്നുപോകു ന്നത്.

ഒന്നാം ഘട്ടം: ഒന്നാം ഘട്ടത്തിൽ ശരാശരി ഉല്പന്നവും (AP) സീമാന്ത ഉല്പന്നവും (MP) വർദ്ധിക്കുന്നു. അതിന്റെ ഫലമായി മൊത്തം ഉല്പന്നം (TP) വളരെ കൂടിയ നിരക്കിൽ വർദ്ധിക്കുന്നു. ഈ ഘട്ടത്തെ വർദ്ധമാന പ്രത്യായഘട്ടം (Increasing returns) എന്ന് വിളിക്കുന്നു. ഈ ഘട്ടത്തിൽ ശരാശരി ഉല്പന്നം (AP) പര മാവധിയിൽ എത്തുന്നു.

രണ്ടാം ഘട്ടം: ശരാശരി ഉല്പന്നവും സീമാന്ത ഉല്പന്നവും കുറ യുന്ന ഈ ഘട്ടത്തിൽ മൊത്തം ഉല്പന്നം കുറഞ്ഞ നിരക്കിൽ വർദ്ധിച്ചുകൊണ്ടിരിക്കുന്നു. മൊത്തം ഉല്പന്നം അതിന്റെ പരമാ വധിയിലെത്തുന്ന ഈ ഘട്ടത്തിൽ സമാന്ത ഉല്പന്നം പൂജ്യത്തി ലെത്തുന്നു. AP യും MP യും കുറയുന്ന ഈ ഘട്ടം അപചയ പ്രത്യായ ഘട്ടം (Diminishing returns) എന്നറയിപ്പെടുന്നു.

മൂന്നാം ഘട്ടം: സീമാന്ത ഉല്പന്നം ഋണാത്മകമാകുന്നു. (നെഗ റ്റീവ്). അതിന്റെ ഫലമായി മൊത്തം ഉല്പന്നം കുറയുവാൻ തുട ക്കുന്നു. ശരാശരി ഉല്പന്നം കുറയുന്നത് തുടരുന്നു. TP വകം പരമാവധിയിലെത്തുന്ന ബിന്ദുവിൽ MP പൂജ്യമാവുന്നു. MP വകം നെഗറ്റീവ് ആകുമ്പോൾ (പൂജ്യത്തിന് താഴേക്ക്) TP കുറ യുവാൻ തുടങ്ങുന്നു. ഈ ഘട്ടത്ത ഋണാത്മക പ്രത്യായ ഘട്ടം
(Negative returns) എന്ന് വിളിക്കുന്നു.

Returns to scale

As stated earlier, all the factor inputs become variable in the long run and thereby no distinction can be made between fixed inputs and variable inputs. Return to scale is associated with long run production function. Returns to scale refers to the change in output when all inputs are variable and the proportion between inputs remains constant. When all the inputs vary in the same proportion, the output (TP) behaves in different manner, which can be clubbed into three categories.

1) Increasing Returns to Scale (IRS): When a proportionate change in all the inputs leads to more than proportionate change in output, it is known as the stage of increasing returns to scale. For instance, a 10 percentage change in inputs results in more than 10 percentage change in
output.

2) Constant Returns to Scale (CRS): When a proportionate change in all the inputs leads to change in output in the same proportion is known as the stage of constant returns to scale. It indicates that a 10 percentage change in inputs leads to exactly 10 percentage change in output.

3) Decreasing Returns to Scale (DRS): When a proportionate change in all the inputs leads to less than proportionate change in output is known as the stage of decreasing returns to scale. It indicates that a 10 percentage change in inputs leads to less than 10 percentage change in output.

തോതനുസരിച്ചുള്ള പ്രത്യായം: എല്ലാ നിവേശങ്ങളും മാറുകയും, എന്നാൽ നിവേശങ്ങൾ തമ്മിലുള്ള അംശബന്ധം സ്ഥിരമായിരി ക്കുകയും ചെയ്താൽ മൊത്തം ഉല്പന്നത്തിലുണ്ടാകുന്ന മാറ്റ ത്തെയാണ് തോതനുസരിച്ചുള്ള പ്രത്യായം (Returns to Scale) എന്നു വിളിക്കുന്നത്. ഒരേ അനുപാതത്തിൽ നിവേശങ്ങൾ മാറി യാൽ ഉല്പന്നങ്ങൾ മൂന്നു ഘട്ടങ്ങളിലൂടെ കടന്നുപോകുന്നു.

i) തോതനുസരിച്ചുള്ള വർദ്ധമാന പ്രത്യായം (IRS): നിവേശങ്ങ ളിലുള്ള ആനുപാതിക വർദ്ധനവിനേക്കാൾ കൂടുതൽ ആനു പാതിക വർദ്ധനവ് ഉൽപന്നത്തിന് ഉണ്ടാകുന്നതിനെയാണ്
തോതനുസരിച്ചുള്ള വർദ്ധമാന പ്രത്യായം എന്നു പറയുന്നത്.

ii) തോതനുസരിച്ചുള്ള സ്ഥിര പ്രത്യായം (CRS): നിവേശങ്ങളി ലുള്ള ആനുപാതിക വർദ്ധനവും ഉല്പന്നത്തിലുള്ള ആനു പാതിക വർദ്ധനവും ഒരേ തോതിലാണെങ്കിൽ അതിനെ തോതനുസരിച്ചുള്ള സ്ഥിര പ്രത്യായം എന്നു വിളിക്കുന്നു.

iii) തോതനുസരിച്ചുള്ള അപചയ പ്രത്യായം (DRS): നിവേശങ്ങൾ ഒരേ അനുപാതത്തിൽ വർദ്ധിക്കുമ്പോൾ ഉല്പന്നത്തിൽ കുറഞ്ഞ അനുപാതത്തിൽ ഉണ്ടാകുന്ന വർദ്ധനവാണിത്. അതായത് ചുരുക്കത്തിൽ ദീർഘകാല ഉല്പ്പാദന ധർമ്മത്തിൽ നിവേശങ്ങളെല്ലാം ഇരട്ടിയാകുമ്പോൾ ഉല്പന്നം ഇരട്ടിയിൽ കൂടുതലാണെങ്കിൽ IRS എന്നും, ഉല്പന്നം ഇരട്ടിയാണ ങ്കിൽ CRS എന്നും, ഉല്പന്നം ഇരട്ടിയിൽ കുറവാണെങ്കിൽ DRS എന്നും വിളിക്കുന്നു.

Costs

The expense incurred for purchasing inputs are known as costs. The cost-output relationship is called cost function.

ചെലവ്: സാധനങ്ങളും സേവനങ്ങളും ഉല്പാദിപ്പിക്കാൻ വേണ്ടി ഒരു ഉല്പാദക യൂണിറ്റിന് ഉണ്ടാകുന്ന വ്യയത്തെയാണ് ചെലവ് എന്ന് പറയുന്നത്. ഉല്പ്പന്നവും ചെലവും തമ്മിലുള്ള ബന്ധത്തെ ചെലവ് ധർമ്മം എന്ന് വിളിക്കുന്നു.

Short-run costs

Costs of some of the factors of production cannot be varied in the short run are known as short run costs. Short run costs are given below.
i) There are costs that incurred by the firms even though the output is zero. The fixed costs will be positive when the level of output is zero.

ii) There will not be any variable cost if the output of the firm is zero.

iii) Average Cost (AC): It is the cost per unit. The average cost curve is U shaped.

ശരാശരി ചെലവ്: ഒരു യൂണിറ്റ് ഉല്പാദിപ്പിക്കാനാവശ്യമായ ചെലവാണ് ശരാശരി ചെലവ്. AC = TC/Q

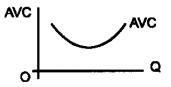
iv) Average Variable Cost (AVC): It is the variable cost per unit. It is also U shaped.
ശരാശരി വിഭേദക ചെലവ്: ഒരു യൂണിറ്റ് ഉല്പാദിപ്പിക്കാനാ വശ്യമായ വിഭേദക ചെലവ്. ഇതും U ആകൃതിയിലാണ്.
AVC = TVC/Q

v) Average Fixed Cost (AFC): It is the fixed cost per unit. It is a rectangular hyperbola. This is the only cost that is always decreasing. But it never becomes zero.

ഒരു യൂണിറ്റ് ഉല്പാദിപ്പിക്കാനാവശ്യമായ ശരാശരി സ്ഥിരപ്പെ ലവ്. ഇതിന് ഒരു ദീർഘചതുര ഹൈപ്പർബോളയുടെ ആക തിയാണ്. ഇത് എപ്പോഴും കുറഞ്ഞുകൊണ്ടിരിക്കുന്ന ചെല വാണ്. ഇതൊരിക്കലും പൂജ്യം ആകുന്നില്ല.

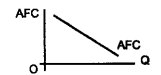
vi) Marginal Cost (MC): It is the cost of producing an extra unit of output. If the outputs are in continuous numbers MC is also U shaped.
MC = \(\frac{\Delta T C}{\Delta Q}\) or TC₂ – TC₁
ഒരു യൂണിറ്റ് കൂടുതലായി ഉല്പാദിപ്പിക്കാൻ വരുന്ന ചെല വാണ് സീമാന്ത ചെലവ്. MC യും U ആകൃതിയിലാണ്.

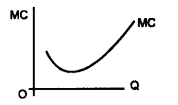
Relation between AC, MC and AVC
The diagram below shows the relation between AC, MC and AVC.

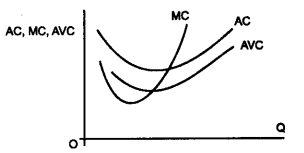
MC will always pass through the minimum of AVC and AC. As the output increases the gap between AC and AVC reduces.

AC, MC, AVC എന്നിവ തമ്മിലുള്ള ബന്ധം ഡയഗ്രത്തിൽ AC, MC, AVC എന്നിവ തമ്മിലുള്ള ബന്ധം കാണിച്ചിരിക്കുന്നു.

• M എപ്പോഴും AC, AVC എന്നിവയുടെ മിനിമം പോയിന്റി ലുടെ മാത്രമേ കടന്നുപോകു.
• ഉല്പാദനം വർധിക്കുന്നതിനനുസരിച്ച് AC, AVC എന്നിവ തമ്മിലുള്ള അകലം കുറഞ്ഞുവരുന്നു.

Cobb Douglas Production Function

Paul Douglas and Charles Cobb developed Cobb Douglas Production function in 1927. The production function is in the form
q = \(x_{1}^{\alpha} x_{2}^{\beta}\)
Where a and B are constants. The firm produces q amount of output using x₁ amount of factor 1 and x₂ amount of factor 2. This is a long run when α + β = 1, the production function exhibits constant returns to scale.

When α + β > 1, the production function exhibits increasing returns to scale.
When α + β < 1, the production function exhibits decreasing returns to scale.

കോബ് ഡഗ്ലാസ് ഉല്പാദന ധർമ്മം പോൾ ഡഗ്ലാസ് ചാൾസ് കോബ് എന്നിവർ 1927 – ൽ വികസിപ്പി ച്ചതാണ് കോബ് ഡഗ്ലാസ് ഉല്പ്പാദന ധർമ്മം. ഈ ഉല്പാദന ധർമ്മം താഴെ കൊടുത്തിരിക്കുന്ന രൂപത്തിലാണുള്ളത്.
q = \(x_{1}^{\alpha} x_{2}^{\beta}\)
α + β എന്നിവ സ്ഥിരമാണ്. ഫേം X₁ എന്ന അളവിൽ ഒന്നാമത്തെ ഘടകവും X₂ എന്ന അളവിൽ രണ്ടാമത്തെ ഘടകവും ഉപയോ ഗിച്ച് q എന്ന അളവിൽ ഉല്പന്നം ഉല്പാദിപ്പിക്കുന്നു. ഇത് ഒരു ദീർഘകാല ഉല്പാദന ധർമ്മമാണ്.

α + β = 1 ആണെങ്കിൽ ഇത് തോതനുസരിച്ചുള്ള സ്ഥിര പ്രത്യായം എന്ന് അറിയപ്പെടുന്നു.
α + β > 1 ആണെങ്കിൽ ഇത് തോതനുസരിച്ചുള്ള വർധമാന പ്രത്യായം എന്ന് അറിയപ്പെടുന്നു.
α + β < 1 ആണെങ്കിൽ ഇത് തോതനുസരിച്ചുള്ള അപചയ | പ്രത്യായം എന്ന് അറിയപ്പെടുന്നു.

Plus Two Economics Notes