Kerala Plus Two Maths Previous Year Question Paper Say 2018 with Answers
| Board | SCERT |
| Class | Plus Two |
| Subject | Maths |
| Category | Plus Two Previous Year Question Papers |
Time : 2½ Hours
Cool off time : 15 Minutes
Maximum : 80 Score
General Instructions to Candidates :
- There is a ‘Cool off time’ of 15 minutes in addition to the writing time.
- Use the ‘Cool off time’ to get familiar with questions and to plan your answers.
- Read questions carefully before you answering.
- Read the instructions carefully.
- When you select a question, all the sub-questions must be answered from the same question itself.
- Calculations, figures and graphs should be shown in the answer sheet itself.
- Malayalam version of the questions is also provided.
- Give equations wherever necessary.
- Electronic devices except non programmable calculators are not allowed in the Examination Hall.
Question 1 to 7 carry 3 scores each. Answer any 7 questions.
Question 1.
a) Construct a 2 × 2 matrix whose elements are given aij = 2i + j
b) Find A2.
Answer:
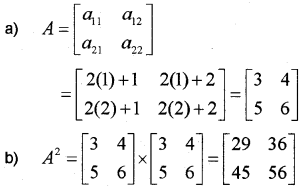
Question 2.
a) If \(\int \frac{f(x)}{x^{2}+1} d x\) = log | x2 + 1 | + C, then f(x) = …………
b) Find ∫ xex dx
Answer:
a) f(x) = 2x
b) ∫ xex dx = x∫ex dx – ∫1 × ex dx
= xex – ex + c = ex (x – 1) + c
Question 3.
Form the differential equation of the family of all circles touching the y-axis at origin.
Answer:
Equation of the family of circle which touches the y-axis at origin is of the form.
(x – a)2 + y2 = a2 ……… (1)
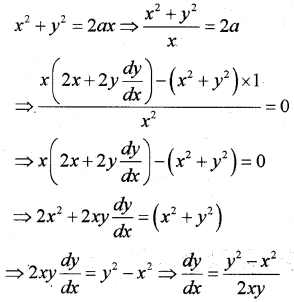
Question 4.
Consider the relation in the set N of Natural numbers defined as R = { (a,b): ab is a factor of 6}. Determine whether the relation is reflexive, symmetric or transitive.
Answer:
(2, 2) ∉ R, Not reflexive
(x, y) ∈ R ⇒ (y, x) ∈ R ⇒ xy = yx, Symmetric
(3, 2) ∈ R, (2, 3) ∈ R ⇒ (3, 3) ∉ R,
Since 3 × 3 = 9, not transitive.
Question 5.
Find the area bounded by the curve y= cos x and x axis between x = 0 and x = π.
Answer:
Area = 2 \(\int_{0}^{\pi / 2}\) cos xdx
= 2 \([\sin x]_{0}^{\pi / 2}\) = 2[1 + 0] = 2
Question 6.
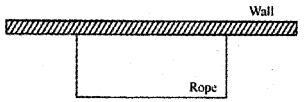
A rectangular plot is to be fenced using a rope of length 20 meters with one of its sides is a wall as shown in the figure. Find the maximum area of such rectangle.
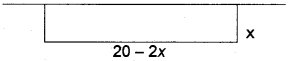
Length = 20 – 2x, breadth = x
A = x(20 – 2x) = 20x – 2x2
A'(x) = 20 – 4x ⇒ A'(x) = 0 ⇒ x = 5
A”(x) = -4 < 0
Hence A is maximum at x = 5
Maximum area = 5 × 10 = 50
Question 7.
A manufacture produces nuts and bolts. The time required to produce one packet of nuts and one packet of bolts on machines A and B is given in the following table
| Machine A | Machine B | |
| Nuts (1 packet) | 2 hours | 3 hours |
| Bolts (1 packet) | 3 hours | 1 hour |
He earns a profit of Rs. 25 per packet of nuts and Rs. 12 per packet of bolts. He operates his machines for almost 15 hours a day. Formulate a linear programming problem to maximise his profit.
Answer:
Maximise: Z = 25x + 12y
Subject to
2x + 3y ≤ l5; 3x + y ≤ 5; x, y ≥ 0
Questions 8 to 17 carry 4 scores each. Answer any 8.
Question 8.
Consider the curve y = x3 + 8x + 3
a) Find the point on the curve at which the slope of the tangent is 20.
b) Does there exist a tangent to the curve with negative slope? Justify your answer.
Answer:
a) \(\frac{d y}{d x}\) = 3x2 + 8
Slope is given as 20
20 = 3x2 + 8 ⇒ x = ±2
Therefore points (2, 27), (-2, -21)
b) No. 3x2 + 8 ≥ 0 (Always positive for any value of x.)
Question 9.
a) Which of the following functions is not continuous at zero?
i) f(x) = sin x
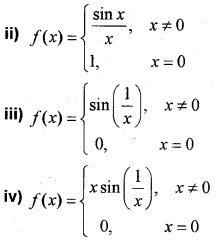
b) Find the Values of a and b such that the function defined by
f(x) = \(\left\{\begin{array}{cc}
10, & x \leq 3 \\
a x+b, & 3<x<4 \\
20, & x \geq 4
\end{array}\right.\)
[Here f(x) is oscillating between -1 and 1 as x approaches to 0. In other cases the limit value and function value are same. So continuous.]
b) \(\lim _{x \rightarrow 3^{+}}\)f(x) = 3a + b ⇒ 3a + b = 10
\(\lim _{x \rightarrow 4^{-}}\) f(x) = 4a + b ⇒ 4a + b = 20
Solving both equations we get a = 10, b = -20
Question 10.
Consider the plane 2x – 3y + z = 5
a) Find the equation of the plane passing through the point (1, 1, 3) and parallel to the above plane.
b) Find the distance between above planes
Answer:
a) Equation of a plane parallel to the
2x – 3y + z = 5 is of the form 2x – 3y + z = k .
Since it passes through the point (1, 1, 3),
we have 2 – 3 + 3 = k ⇒ k = 2
⇒ 2x – 3y + z = 2
b) Distance between the planes
= \(\left|\frac{5-2}{\sqrt{4+9+1}}\right|=\frac{3}{\sqrt{14}}\)
Question 11.
Consider the vectors
\(\vec{a}\) = 2i + j + 3k; \(\vec{b}\) = i + 4j – k
a) Find the projection of \(\vec{a}\) on \(\vec{b}\)
b) If \(\vec{a}\) is perpendicular to a vector \(\vec{c}\) then projection of \(\vec{a}\) on \(\vec{c}\)
c) Write a vector \(\vec{d}\) such that the projection of \(\vec{a}\) on \(\vec{d}\) = |\(\vec{a}\)|
Answer:
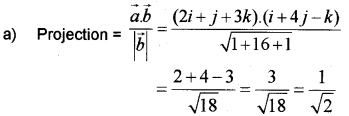
b) Projection will be zero.
c) Projection of \(\vec{a}\) on \(\vec{d}\) = |\(\vec{a}\)|, means the angle between \(\vec{a}\) and \(\vec{d}\) is zero. Hence both are parallel. So any vector parallel to \(\vec{a}\) is \(\vec{d}\).
Question 12.
a)
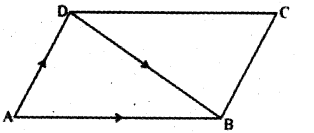
In the figure ABCD is a Parallelogram. If
\(\overrightarrow{A B}\) = 3i – j + 2k; \(\overrightarrow{A D}\) = i + j + 2k, find \(\overrightarrow{A C}\) and \(\overrightarrow{D B}\)
b) If \(\vec{a}\) and \(\vec{b}\) are adjacent sides of any parallelogram \(\vec{c}\) and \(\vec{d}\) are diagonals,
then show that |\(\vec{c}\) × \(\vec{d}\)| = 2|\(\vec{a}\) × \(\vec{b}\)|
Answer:
a) \(\overrightarrow{A C}\) = \(\overrightarrow{A B}\) + \(\overrightarrow{A D}\) = 4i + 4j
\(\overrightarrow{B D}\) = \(\overrightarrow{A B}\) – \(\overrightarrow{A D}\) = 2i – 2j
b) Let \(\vec{c}\) = \(\vec{a}\) + \(\vec{b}\) and d = \(\vec{a}\) – \(\vec{b}\)
\(\vec{c}\) × \(\vec{d}\) = (\(\vec{a}\) + \(\vec{b}\)) × (\(\vec{a}\) – \(\vec{b}\))
= \(\vec{a}\) × \(\vec{a}\) – \(\vec{a}\) × \(\vec{b}\) + \(\vec{b}\) × \(\vec{a}\) – \(\vec{b}\) × \(\vec{b}\)
= 0 – \(\vec{a}\) × \(\vec{b}\) – \(\vec{a}\) × \(\vec{b}\) – 0 = -2(\(\vec{a}\) × \(\vec{b}\))
Question 13.
Find ∫(4x + 7)\(\sqrt{x^{2}+4 x+13}\)dx
Answer:
4x + 7 = A(2x + 4) + B ⇒ A = 2, B = -1
I = 2∫\(\sqrt{x^{2}+4 x+13}\)dx – ∫\(\sqrt{x^{2}+4 x+13}\)dx
I = 2I1 – I2
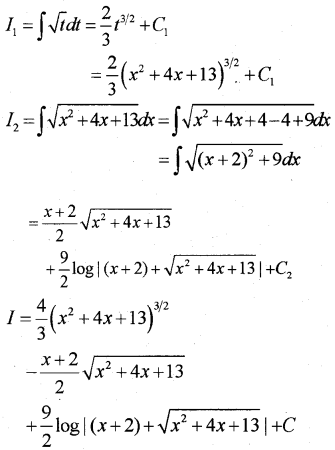
Question 14.
a) Write integrating factor of the linear differential equation \(\frac{d y}{d x}+\frac{y}{x}\) = sin x
b) Slope of the tangent to a curve at any point is twice the x coordinate of the point. If the curve passes through the point (1, 4), find its equation.
Answer:
IF = \(e^{\int P d x}\) = \(e^{\log x}\) = x
b) \(\frac{d y}{d x}\) = 2x ⇒ dy = 2x dx
Integrating we get;
∫dy = ∫ 2x dx + C ⇒ y = x2 + C
Since passes through (1, 4) we have;
4 = 1 + C ⇒ C = 3
Hence equation is y = x2 +3
Question 15.
Solve the linear programming problem graphically Maximise Z = 3x + 5y
Subject to the constraints
x + 3y ≤ 3
x + y ≤ 2
x, y ≥ 0
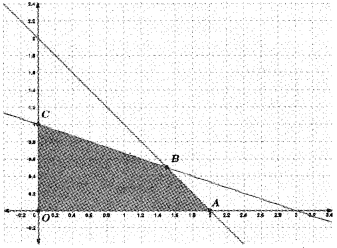
| Corner points | Z = 3x + 5y |
| O (0, 0) | 0 |
| A (2, 0) | 6 |
| B\( \left(\frac{3}{2}, \frac{1}{2}\right)\) | 7 |
| C (0, 1) | 5 |
Maximum 7 at B = \(\left(\frac{3}{2}, \frac{1}{2}\right)\)
Question 16.
a) If cos-1 \(\frac{12}{13}\) = tan-1 x then find x.
b) Show that cos-1 \(\frac{4}{5}\) + cos-1 \(\frac{12}{13}\) = tan-1 \(\frac{14}{33}\)
Answer:
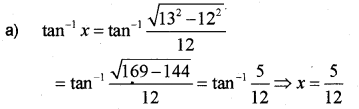
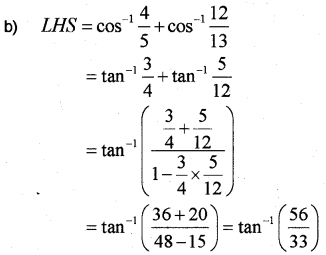
Question 17.
Consider the binary operation * on the set R of real numbers, defined by a*b = \(\frac{a b}{4}\)
a) Show that * is commutative and associative.
b) Find the identity element for * o R.
c) Find the inverse of 5.
Answer:
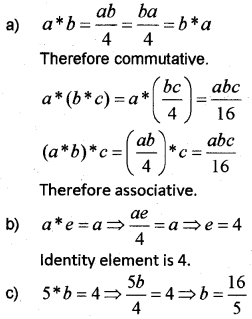
Questions from 18 to 24 carry 6 scores each. Answer any 5.
Question 18.
Consider the matrix A = \(\left[\begin{array}{lll}
1 & 0 & 2 \\
0 & 1 & 2 \\
0 & 4 & 9
\end{array}\right]\)
a) Find A-1 using elementary row operations.
b) Find the solution of the system of equations given below:
(A-1 obtained above may be used)
x + 2z + 2; y + 2z + 1; 4y + 9z = 3
Answer:
a) A = IA
\(\left[\begin{array}{lll}
1 & 0 & 2 \\
0 & 1 & 2 \\
0 & 4 & 9
\end{array}\right]=\left[\begin{array}{lll}
1 & 0 & 0 \\
0 & 1 & 0 \\
0 & 0 & 1
\end{array}\right] A\)
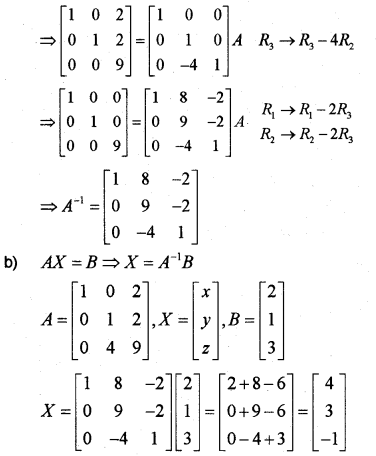
Question 19.
a) Show that
\(\left|\begin{array}{lll}
1 & a & b c \\
1 & b & a c \\
1 & c & a b
\end{array}\right|\) = (a – b)(b – c)(c – a)
b) If A = \(\left[\begin{array}{cc}
2 & 3 \\
4 & -1
\end{array}\right]\) verift that A × adj A = |A|I
Answer:
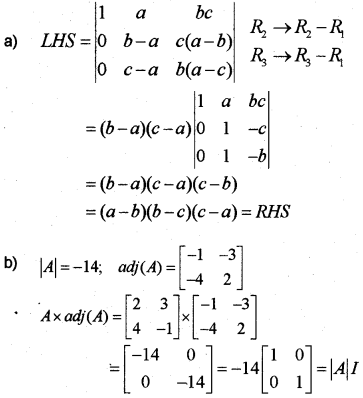
Question 20.
a) If f is a function such that f(-x) = f(x), then \(\int_{-a}^{a}\) f(x) dx = ………
b) Evaluate \(\int_{-\pi / 2}^{\pi / 2}\) cos x dx
c) Evaluate \(\int_{0}^{1}\) (x2 + 1)dx as the limit of a sum.
Answer:
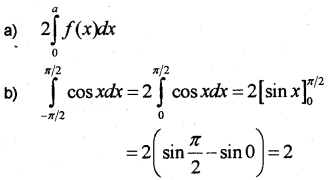
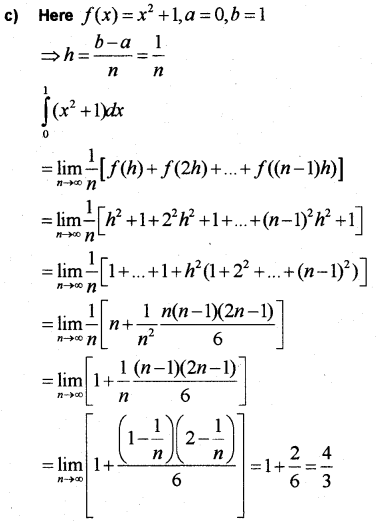
Question 21.
a) Verify mean value theorem for the function f(x) = x2 – 4x – 3 in the interval [1, 4].
b) Consider the function
f(x) = sin-1 2x \(\sqrt{1-x^{2}}\), \(\frac{-1}{\sqrt{2}} \leq x \leq \frac{1}{\sqrt{2}}\)
i) Show that f(x) = 2sin-1 x
ii) Find f'(x)
Answer:
a) f(x) is a continuous function in [1, 4], since it is a polynomial.
f'(x) = 2x – 4, f is differentiable in (1, 4).
f(4) = 16 – 16 – 3 = -3, f(1) = 1 – 4 – 3 = -6
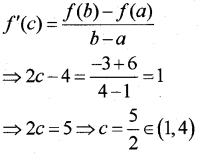
Hence mean value theorem is verified.
b) i) Put x = sinθ
f(x) = sin-1 (2 sin θ\(\sqrt{1-\sin ^{2} \theta}\))
= sin-1 (2 sin θ cos θ)
= sin-1 (sin 2θ) = 2θ = 2 sin-1 x
ii) f'(x) = \(\frac{2}{\sqrt{1-x^{2}}}\)
Question 22.
a) Show that the lines
\(\frac{x-2}{1}=\frac{y+1}{2}=\frac{z-3}{1} ; \frac{x-3}{2}=\frac{y-1}{1}=\frac{z-4}{2}\) are coplanar.
b) Find the equation of the plane that contains the above lines.
c) Show that the above lines intersect at the point (3, 1, 4).
Answer:
a) Points (2, -1, 3) and (3, 1, 4)
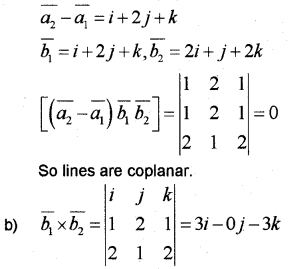
Normal direction ratios are 3, 0, -3.
Therefore equation of the plane is
3(x – 2) -3 (z – 3) = 0
3x – 6 – 3z + 9 = 0
x – z + 1 = 0
c) (3, 1, 4) is a point on the second line. Substitute the point in the first line
\(\frac{3-2}{1}=\frac{1+1}{2}=\frac{4-3}{1} \Rightarrow \frac{1}{1}=\frac{2}{2}=\frac{1}{1}\)
Therefore the point (3, 1, 4) satisfies the first line. Hence both interest at (3, 1, 4).
Question 23.
a) A coin is tossed 3 times. Find the probability distribution of the number of heads.
b) A bag contains 5 black and 6 white balls, 4 balls of the same colour (Black or white) are added to the bag, shuffled well and one ball is drawn. If the ball obtained is white. What is the probability that the balls added are black?
Answer:
a) Let X be the random variable denoting the
number of heads appears. Then X = {0, 1, 2, 3}
P(X = x) = nCx Pxqn-x; p = \(\frac{1}{2}\), q = \(\frac{1}{2}\), n = 3
| X | 0 | 1 | 2 | 3 |
| P(X) | \(\frac{1}{8}\) | \(\frac{3}{8}\) | \(\frac{3}{8}\) | \(\frac{1}{8}\) |
E1 = Balls added are black.
E2 = Balls added are white.
A = Ball drawn is white.
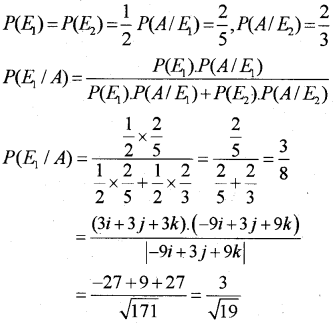
Question 24.
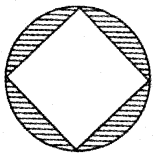
In a circle of radius 2 a square is inscribed as shown in the figure. Using integration, find the area of the shaded region (Area of a square may be calculated using any convenient method)
Answer:
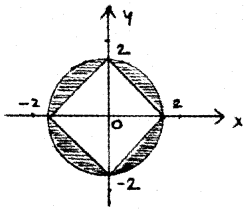
Equation of the circle is x2 + y2 = 4
Area of the sector in the first quadrant
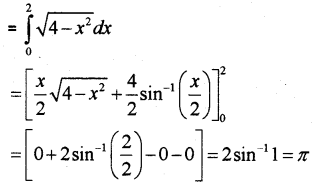
Area of the triangle = \(\frac{1}{2}\) (2)(2) = 2
Area of the shaded region in the first quadrant = π – 2
Hence the area of the required region = 4(π – 2) = 4π – 8
