Plus Two Maths Notes Chapter 4 Determinants is part of Plus Two Maths Notes. Here we have given Plus Two Maths Notes Chapter 4 Determinants.
| Board | SCERT, Kerala |
| Text Book | NCERT Based |
| Class | Plus Two |
| Subject | Maths Notes |
| Chapter | Chapter 4 |
| Chapter Name | Determinants |
| Category | Kerala Plus Two |
Kerala Plus Two Maths Notes Chapter 4 Determinants
Introduction
Determinants have wide applications in engineering, Science, Economics, Social Science, etc. In this chapter we study about the various properties of determinants, minors, cofactors, applications in finding the area of triangle, adjoint, and inverse of a square matrix, and consistency and in consistency of linear equations.
A. Basic Concepts
I. Determinant
Determinant is a real number associated with a square matrix. The determinant of matrix A is denoted by |A|. The value of a determinant is obtained by the sum of products of elements of a row (column) with corresponding cofactors.
- |AB| = |A||B|
- |An| = |A|n
Properties:
(i) The value of a determinant remains the same if its rows and columns are interchanged.
ie; |AT| = |A|.
(ii) If any two rows (or columns) of a determinant are interchanged, then sign of determinant changes.
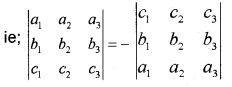
(iii) If any two rows (or columns) of a determinant is identical, then value of determinant is zero.
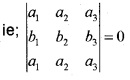
(iv) If each element of a row (or columns) of a determinant is multiplied by a constant k, then its value gets multiplied by k.
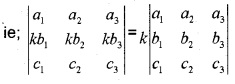
(a) If A is a square matrix of order n, then
|KA| = kn|A|
(v) If any two rows (or columns) of a determinant is proportional, then value of determinant is
zero.
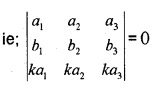
(vi) If some or all elements of a row or column of a determinant are expressed as sum of two (or more) terms, then the determinant can be expressed as sum of two (or more) determinants.
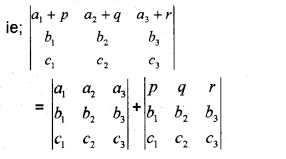
(vii) If, to each element of any row or column of a determinant, the equimultiples of corresponding elements of other row (or column) are added, then value of determinant remains the same, ie; the value of determinant remains same if we apply the operation Ri → Ri + kRj or Ci → Ci + kCj.
(viii) If the elements of a row or column are multiplied with cofactors of any other row or column, then their sum is zero.
ie; for example; a11C21 + a12C22 + a13C23 = 0.
If the elements of a row or column are multiplied with cofactors of the corresponding row or column, then their sum is |A|.
ie; for example; a11C11 + a12C12 + a13C13 = |A|.
1. Minor of an element:
The minor of an element aij is the determinant obtained by deleting the ith row and jth column, usually denoted by Mij.
2. Cofactor of an element:
A signed Minor is called cofactor, ie; Cij = (-1 )i + j Mij. The matrix obtained by replacing all elements by its cofactor is called cofactor matrix.
3. Adjoint Matrix:
The transpose of a cofactor matrix is. called Adjoint Matrix, usually denoted by adj(A)
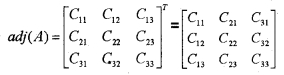
Properties:
- A × adj(A) = adj(A) × A = I|A|
- If A is a square matrix of order n, then |adj(A)| = |A|n – 1
- adj(AB) = adj(A)adj(B)
II. Inverse of a Matrix
A square matrix A is invertible if |A| ≠ 0 and A inverse is denoted by A-1 ie; A-1 = adj(A)|A|
Properties:
- (A-1)-1 = A
- (AB)-1 = B-1A-1
- (AT)-1 = (A-1)T
- If A is a square matrix of order n, then adj(adj(A)) = |A|n-2 × A.
III. Application of Determinants
1. Area of a triangle whose vertices are (x1, y1), (x2, y2), (x3, y3) is
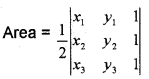
(i) If Area = 0 then the points are collinear.
2. Solving of system of linear equations using
matrix method:
Consider the system of linear equations
a1x + b1y + C1z = d1
a2x + b2y + c2z = d2
a3x + b3y + c3z = d3
Convert the linear equation into matrix form AX = B, where
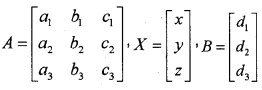
Then the solution is given by X = A-1B
- If |A| ≠ 0 the system is consistent and has unique solution.
- If |A| = 0 and adj(A) × B ≠ 0,the system is inconsistent and has no solution.
- If |A| = Oandadj(A) × B = 0 ,the system may be consistent and has infinitely many solutions.
In order to find these infinitely many solutions, replace one of the variable by k (say z = k) and solve any two of the given equations for x and y in terms of k
We hope the Kerala Plus Two Maths Notes Chapter 4 Determinants help you. If you have any query regarding Kerala Plus Two Maths Notes Chapter 4 Determinants, drop a comment below and we will get back to you at the earliest.
