Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions

Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions are part of Plus Two Maths Chapter Wise Questions and Answers. Here we have given Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions.

Kerala Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions

Plus Two Maths Inverse Trigonometric Functions Three Mark Questions and Answers

Question 1.
Prove the following:

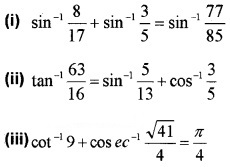
Answer:

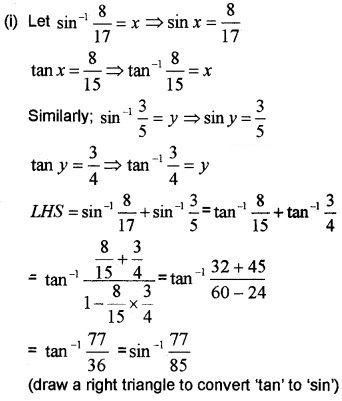
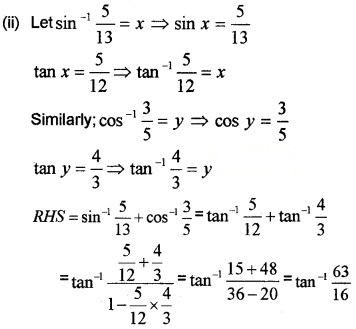
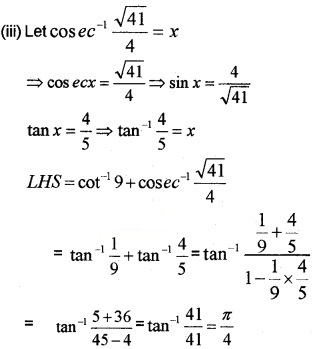
Question 2.
Find the value of

Answer:

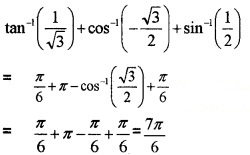
Question 3.
If tan^-1x + tan^-1y + tan^-1z = π, show that x + y + z = xyz
Answer:
Given;
tan^-1x + tan^-1y + tan^-1z = π
⇒ tan^-1x + tan^-1y = π – tan^-1z

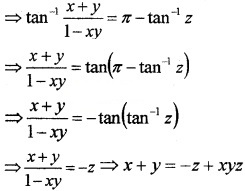
⇒ x + y + z = xyz.

Question 4.
Match the following

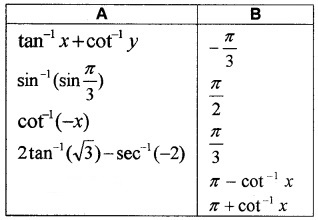
Answer:

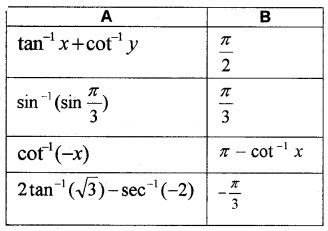
Question 5.
Solve 2 tan^-1(cos x) = tan^-1(2 cos x)
Answer:
2 tan^-1(cosx) = tan^-1(2cosx)
⇒ $\frac{2 \cos x}{1-\cos ^{2} x}$ = 2cosx
⇒ 1 = 1 – cos² x ⇒ 1 = sin²x
⇒ x = ±$\frac{\pi}{2}$.

Question 6.
Solve the following

1. 2tan^-1(cosx) = tan^-1(2cosecx)
2. tan^-12x + tan^-13x = $\frac{\pi}{4}$

Answer:
1. 2tan^-1(cosx) = tan^-1(2cosecx)

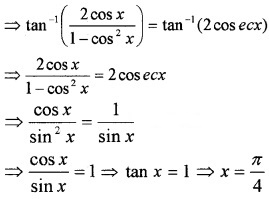
2. tan^-12x + tan^-13x = $\frac{\pi}{4}$

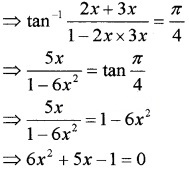
⇒ (6x – 1)(x + 1) = 0
⇒ x = $\frac{1}{6}$, x = – 1
Since x = – 1 does not satisfy the equation, as the LHS becomes negative. So x = $\frac{1}{6}$.

Question 7.
Solve 2 tan^-1(cos x) = tan^-1(2 cos x)
Answer:
2 tan^-1(cos x) = tan^-1(2 cos x)
⇒ $\frac{2 \cos x}{1-\cos ^{2} x}$ = 2cosx
⇒ 1 = 1 – cos² x
⇒ 1 = sin² x
⇒ x = ±$\frac{\pi}{2}$

Plus Two Maths Inverse Trigonometric Functions Four Mark Questions and Answers

Question 1.
Prove that $\sin ^{-1} \frac{12}{13}+\cos ^{-1} \frac{4}{5}+\tan ^{-1} \frac{63}{16}=\pi$
Answer:

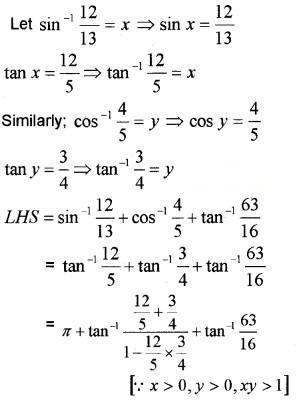
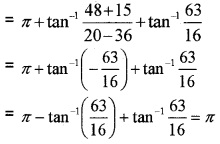
Question 2.

1. Find the principal value of sec^-1$\left(-\frac{2}{\sqrt{3}}\right)$ (1)
2. if sin$\left(\sin ^{-1}\left(\frac{1}{5}\right)+\cos ^{-1}(x)\right)=1$, then find the value of x. (3)

Answer:
1. principal value of:

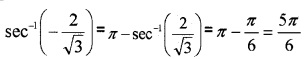
2. find the value of x:

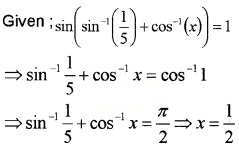
Question 3.
Solve the following

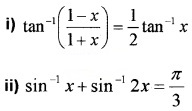
Answer:

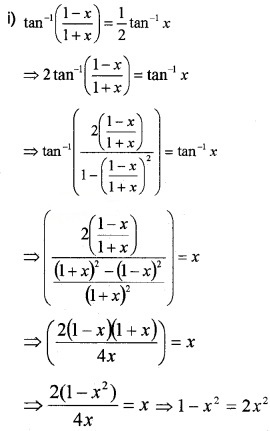
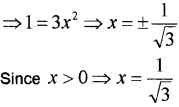
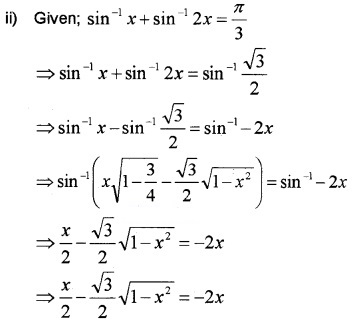
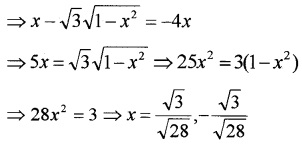
The value x = –$\frac{\sqrt{3}}{\sqrt{28}}$ makes the LHS negative, so rejected.

Question 4.
(i) Choose the correct answer from the bracket. cos(tan^-1 x), |x| < 1 is equal to (1)

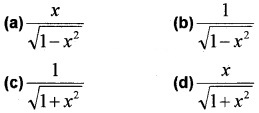
Answer:

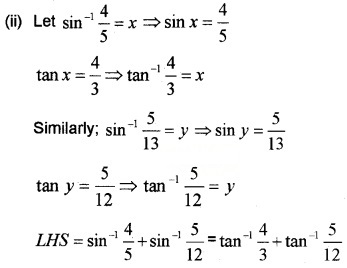
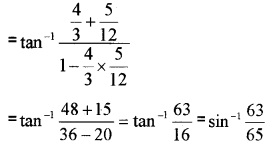
(draw a right triangle to convert ‘tan’ to ‘sin’).

Question 5.
(i) In which quadrants are the graph of cos^-1 (x) lies, x ∈ [-1,1 ] (1)
(ii) If cos^-1x + cos^-1y = $\frac{\pi}{3}$, then
sin^-1x + sin^-1y = ……… (3)
(a) $\frac{2 \pi}{3}$
(b) $\frac{\pi}{3}$
(c) $\frac{\pi}{6}$
(d) $\frac{\pi}$
(iii) If tan^-1x + tan^-1y = $\frac{\pi}{4}$ then prove that x + y + xy = 1 (2)
Answer:
(i) First and Second quadrant

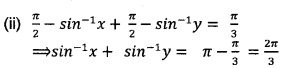
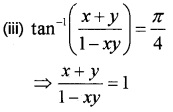
⇒ x + y = 1 – xy ⇒ x + y + xy = 1.

Question 6.
(i) sin(tan^-1(1)) is equal to

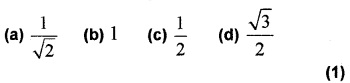
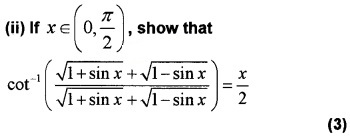
Answer:

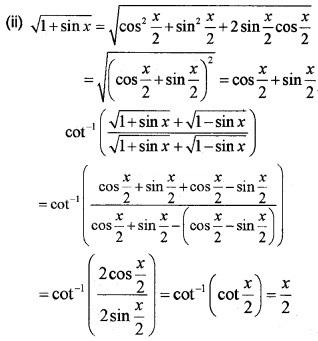
Plus Two Maths Inverse Trigonometric Functions Six Mark Questions and Answers

Question 1.
Show that sin^-1$\frac{3}{5}$ – sin^-1$\frac{8}{17}$ = cos^-1$\frac{84}{85}$.
Answer:

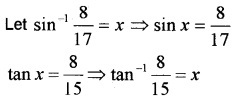
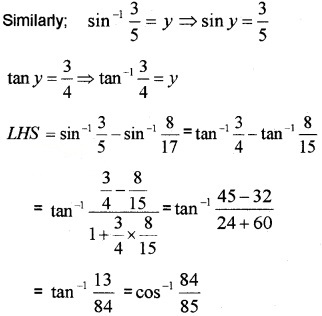
(draw a right triangle to convert ‘tan’ to ‘cos’).

Question 2.
(i) Choose the correct answer from the Bracket.
If cos^-1x = y, then y is equal to (1)
(a) π ≤ y ≤ π
(b) 0 ≤ y ≤ π
(c) $-\frac{\pi}{2}$ ≤ y ≤ $\frac{\pi}{2}$
(d) 0 ≤ y ≤ π
(ii) Find the value of cos^-1 cos$\left(\frac{7 \pi}{3}\right)$ (3)
(iii) Solve for x if, tan^-1$\left(\frac{1+x}{1-x}\right)$ = 2 tan^-1x (2)
Answer:
(i) Range of cos^-1x is [0, π] ⇒ 0 ≤ y ≤ π

(ii) Here $\left(\frac{7 \pi}{3}\right)$ lie outside the interval [0, π].
To make it in the interval proceed as follows.

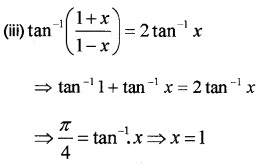
We hope the given Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions will help you. If you have any query regarding Plus Two Maths Chapter Wise Questions and Answers Chapter 2 Inverse Trigonometric Functions, drop a comment below and we will get back to you at the earliest.