Plus Two Maths Chapter Wise Previous Questions Chapter 8 Application of Integrals

Plus Two Maths Chapter Wise Previous Questions Chapter 8 Application of Integrals are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 8 Application of Integrals.

Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 8 Application of Integrals

Plus Two Maths Application of Integrals 4 Marks Important Questions

Question 1.
(i) The area bounded by the curve y = f(x) x-axis and the line x= a and x= b is……
(ii) Find the area enclosed between the Parabola y = x² and the straight line 2x – y + 3 = 0 (March – 2010)
Answer:

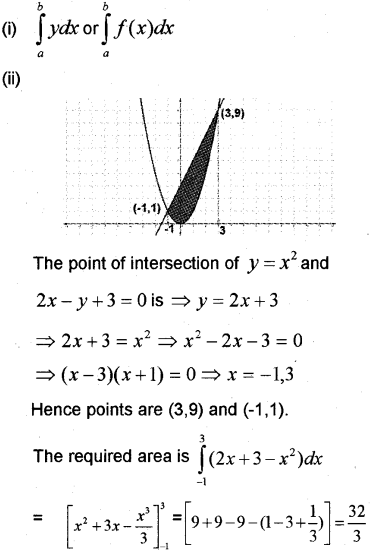
Question 2.
Find the area enclosed between the curve x² = 4y and the line x = 4y – 2 (March -2011)
Answer:

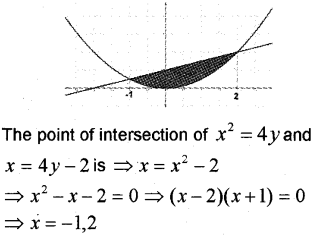
Question 3.
(i) Area of the shaded portion in the figure is equal to

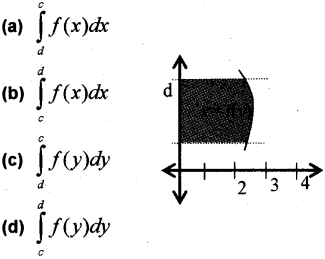
(ii) Consider the curves y = x², x = 0, y = 1, y = 4.
Draw a rough sketch and shade the region bounded by these curves, Find area of the shaded region.
Answer:

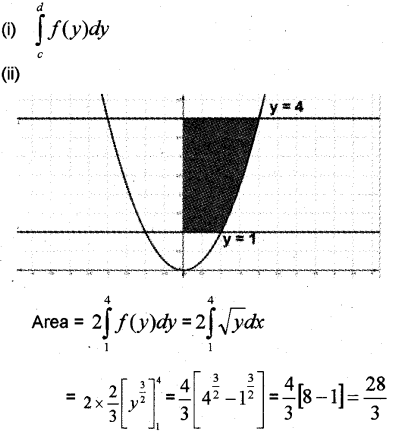
Question 4.
Consider the following figure:

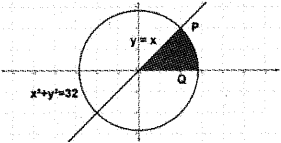
(i) Find the point of Intersection P of the circle x² + y² = 32 and the line y = x.
(ii) Find the area of the shaded region. (EDUCATE – 2017; March – 2013; March – 2014)
Answer:

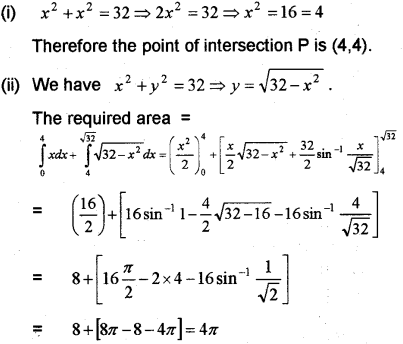
Question 5.
(a) The area bounded by the curve, above the x-axis, between x = a and x = b is

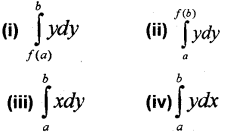
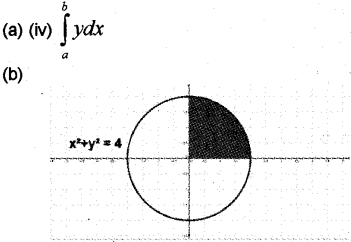
(b) Find the area of the circle x² + y² = 4 using integration. (March – 2016)
Answer:

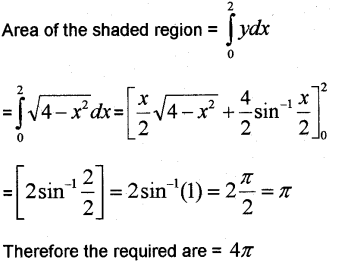
Question 6.
(i) The area bounded by y = 2cosx , the x-axis from x = 0 to x = \(\frac{\pi}{2}\) is
(a) 0
(b) 1
(c) 2
(d) -1
(ii) Find the area of the region bounded by the y² = 4ax and x² = 4ay, a > 0 (March – 2017)
Answer:

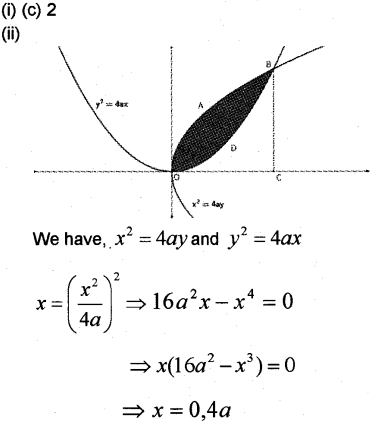
When x = 4a, y = 4a and x = 0, y = 0.
Therefore the point is (0, 0) and (4a, 4a).

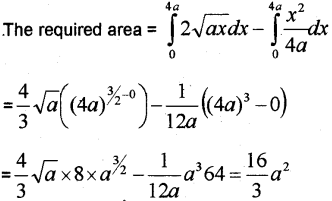
Plus Two Maths Application of Integrals 6 Marks Important Questions

Question 1.
Consider the circle x² + y² = 16 and the straight line \(y=\sqrt{3} x\) as shown ¡n the figure

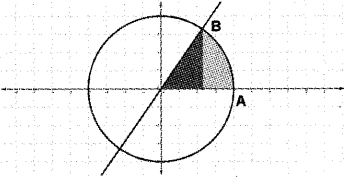
(i) Find the points A and B as shown in the figure.
(ii) Find the area of the shaded region in the figure using definite integral. (May -2010)
Answer:
(i) The point of intersection of x² + y² = l6 and

Question 2.
(i) Draw the rough sketch of \(\frac{x^{2}}{4}+\frac{y^{2}}{9}=1\)
(ii) Find the area bounded by the above curve using integration. (May – 2011)
Answer:
(i) The curve is an ellipse.

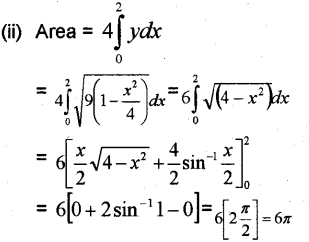
Question 3.
(i) Find the area enclosed between the curve y² = x, x = 1, x = 4 and x-axis.
(ii) Using ntegration, find the area of the region bounded by the triangle whose vertices are (1, 0), (2, 2) and (3, 1). (March-2012)
Answer:

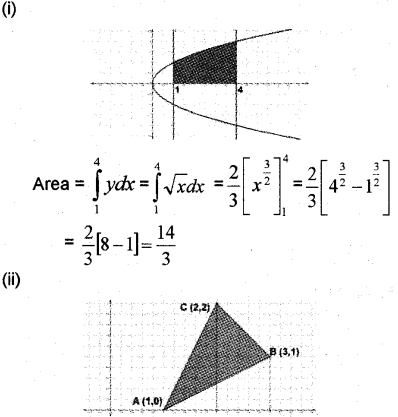
Required area ΔABC = Area ΔAB2
+ Area 2BC3 – Area ΔAC3
Equation AC is y = 2(x – 1)
Equation BC is y = 4 – x
Equation AB is y = 1/2 (x – 1)

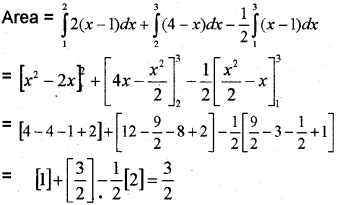
Question 4.

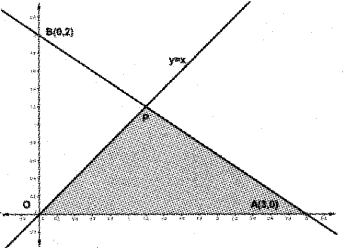
Using the above figure
Find the equation of AB.
Findthe point P.
Find the area of the shaded region by integration. (May – 2013)
Answer:
(i) The equation of a line passing through (0,2)

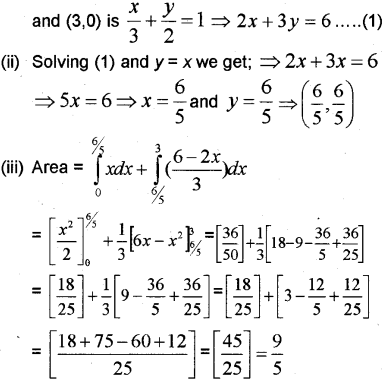
Question 5.
Consider the ellipse \(\frac{x^{2}}{9}+\frac{y^{2}}{4}=1\) and the line \(\frac{x}{3}+\frac{y}{2}=1\).
(a) Find the points where the line intersects the ellipse?
(b) Shade the smaller region bounded by the ellipse and the line.
(c) Find the area of the shaded region. (May – 2014)
Answer:

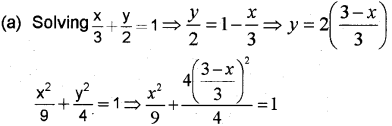
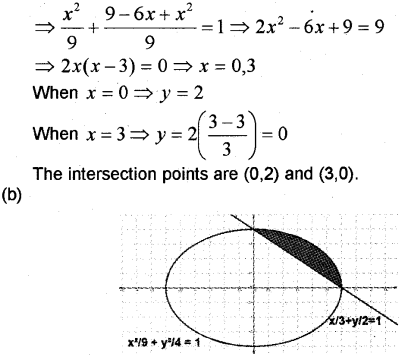
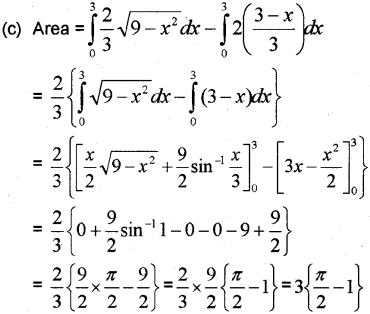
Question 6.
Consider the function f(x) = |x| + 1; g(x) = 1 – |x|
(a) Sketch the graph and shade the enclosed region between them.
(b) Find the area of the shaded region. (March – 2015)
Answer:

(b) The equation of the line through AB is

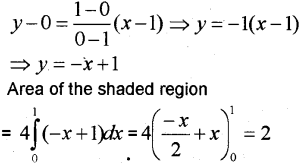
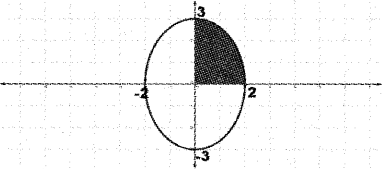
Question 7.
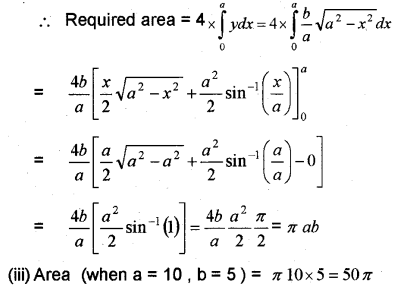
Using the given figure answer the following questions.

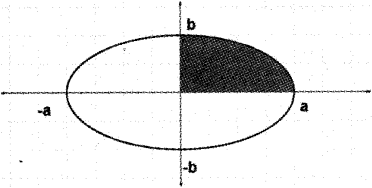
Define the equation of the given curve.
Find the area of the enclosed region.
Find the area when a = lo and b = 5. (March – 2011; May – 2015; March – 2017)
Answer:
(i) The figure represents an ellipse with equation

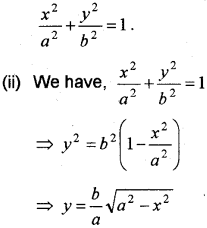
Ellipse is symmetric w.r.t coordinate axles. Therefore the area of the enclosed region is same as four times area enclosed by the curve in first quadrant.

We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 8 Application of Integrals help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 8 Application of Integrals, drop a comment below and we will get back to you at the earliest.