Plus Two Maths Chapter Wise Previous Questions Chapter 7 Integrals are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 7 Integrals.
Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 7 Integrals
Plus Two Maths Application of Derivatives 3 Marks Important Questions
Question 1.
Find the following integrals. (May -2011)
(i) ∫x2e2xdx (ii) ∫exsinxdx
Answer:
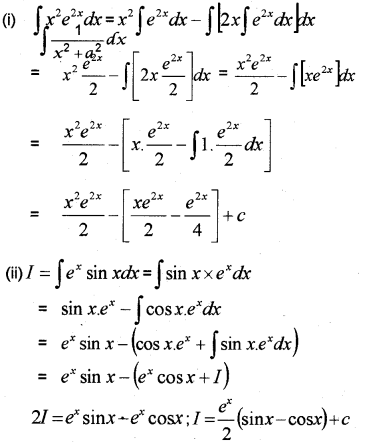
Question 2.
(i) ∫exsecx(1+tanx)dx=……
(a) ex cosx + c (b) ex sec x + c
(C) ex tanx + c (d) ex sin x + c
(ii) Find ∫sin2xcos3xdx (March – 2014)
Answer:
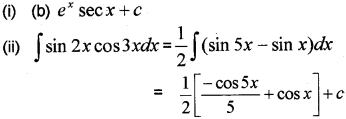
Question 3.
Find the following integrals.
(i) (i) ∫1(x+1)(x+2)dx (ii) ∫2x−1(x−1)(x+2)2dx (March – 2014)
Answer:
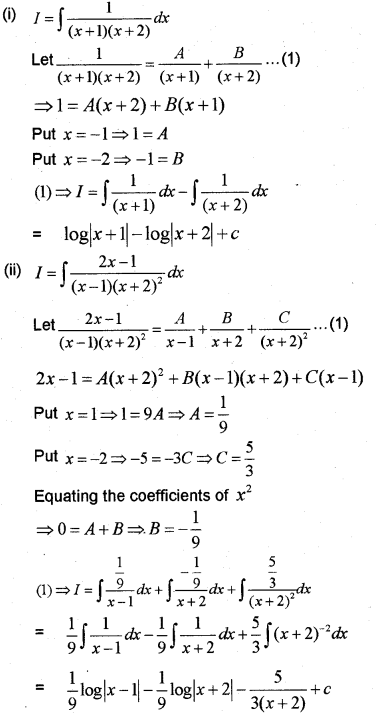
Plus Two Maths Application of Derivatives 4 Marks Important Questions
Question 1.
Consider the integral I=∫π0xsinx1+cos2xdx
(i) Express I=π2∫π0sinx1+cos2xdx
(ii) Show that I=π24 (March – 2012)
Answer:
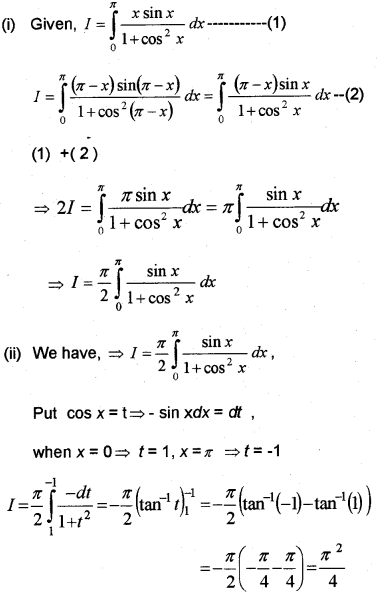
Question 2.
(i) Evaluate: ∫32xx2+1dx
(ii) Evaluate: ∫π0x1+sinxdx (March – 2014)
Answer:
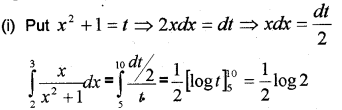
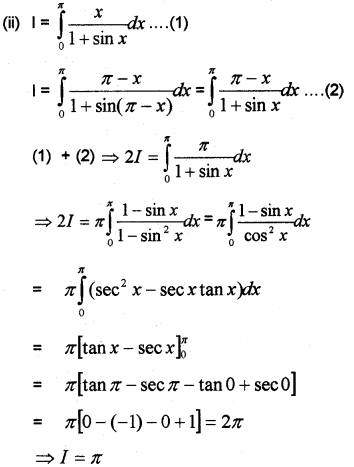
Question 3.
(a) What is the value of ∫10x(1−x)9dx If the
(i) 110 (ii) 111 (iii) 190 (iv) 1110
(b) Find ∫10(2x+3)dx of a sum. (March – 2015)
Answer:
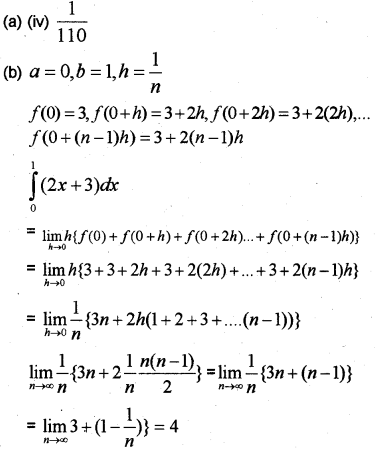
Question 4.
Evaluate ∫x0log(1+cosx)dx
Answer:
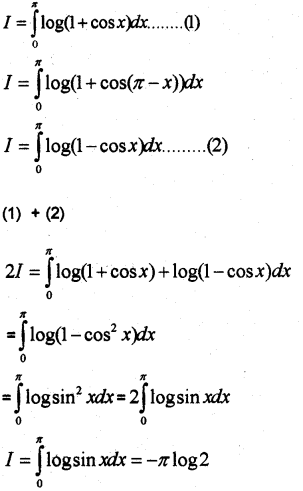
Question 5.
Find ∫50(x+1)dx as limit of a sum.
Answer:
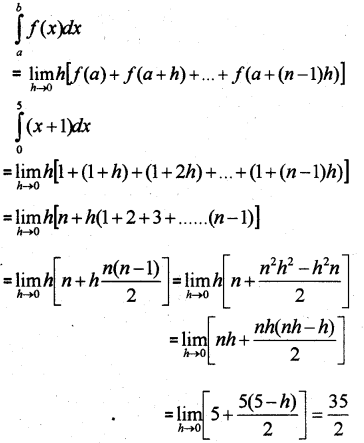
Question 6.
Evaluate ∫40x2dx as the limit of a sum. (March – 2017)
Answer:
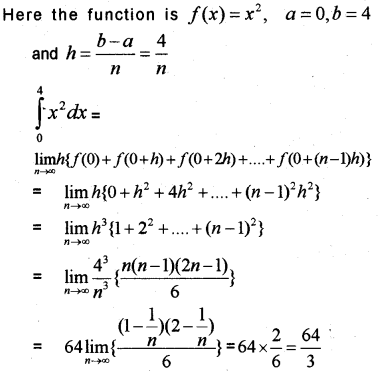
Plus Two Maths Application of Derivatives 6 Marks Important Questions
Question 1.
(i) Fill in the blanks; ∫1xdx=_____
(ii) Evaluate ∫5x+1x2−2x−35dx
(iii) Integrate with respect to x. √x2+4x+8 (March – 2010)
Answer:
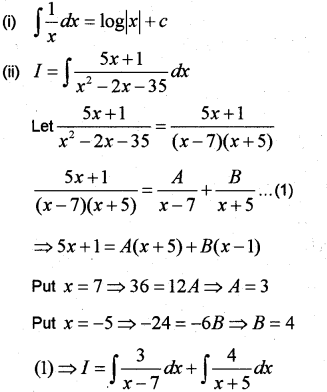
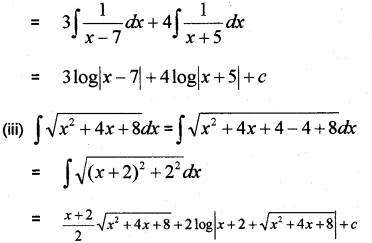
Question 2.
(i) Evaluate ∫−cosec2x√cot2x+9dx
(ii) Evaluate ∫(cos−1x)2dx (May -2010)
Answer:
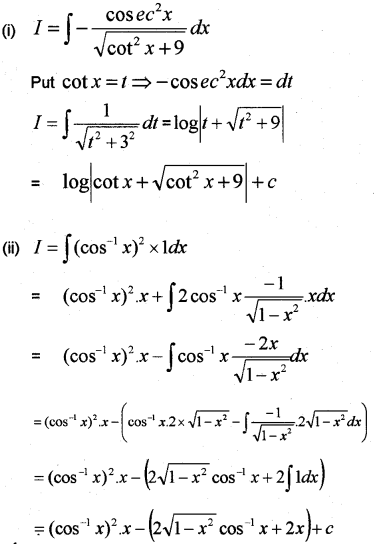
Question 3.
(i) Evaluate ∫π0xsinx1+cos2xdx
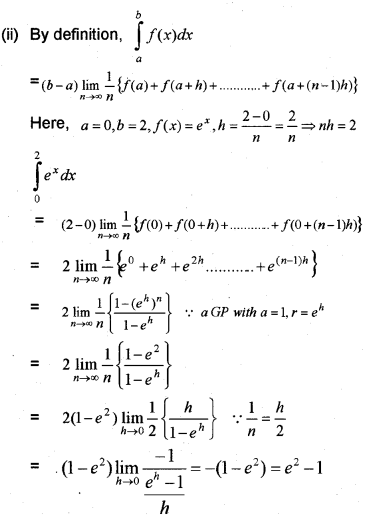
(ii) Evaluate ∫20exdx as limit of a sum. (May -2010)
Answer:
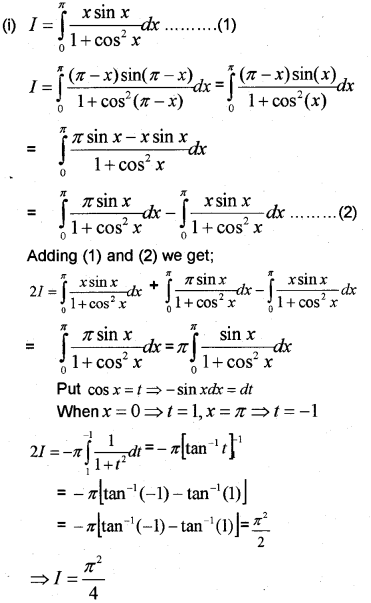
Question 4.
(i) Fill in the blanks ∫cotxdx=_____
(ii) Evaluate the integrals
(a) ∫sin2xcos4xdx (b) ∫x(x+1)(x+2)dx (March -2011)
Answer:
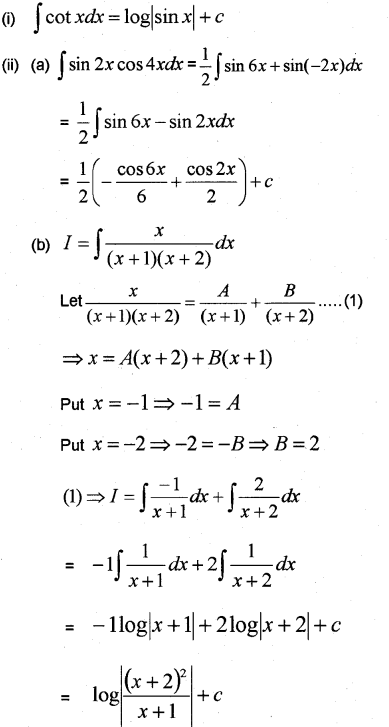
Question 5.
(i) Evaluate ∫10xdx as the limit of a sum.
(ii) Evaluate ∫10x(1−x)ndx (March – 2011)
Answer:
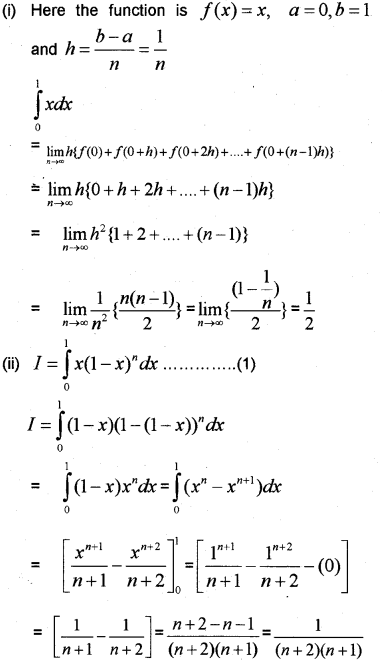
Question 6.
(i) Evaluate ∫211x(1+logx)2dx
(ii) Evaluate ∫30(2x2+3)dx as the limit of a sum. (May – 2011)
Answer:
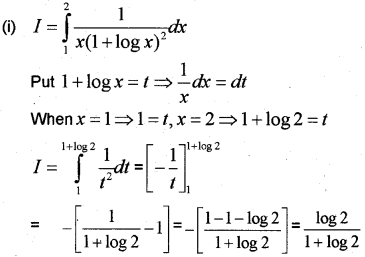
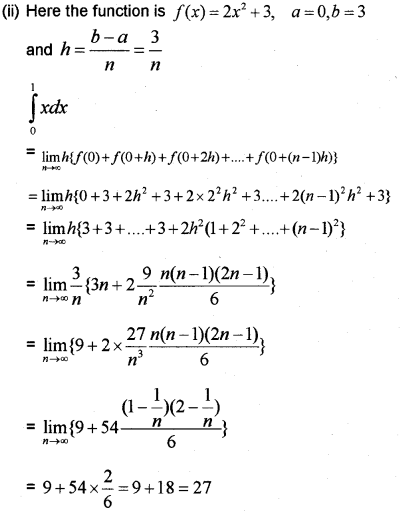
Question 7.
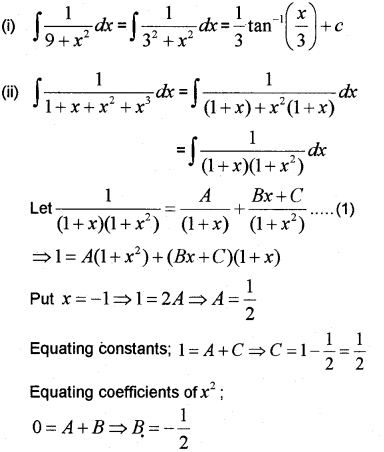
(i) What is ∫19+x2dx=?
(ii) Evaluate the integrals ∫11+x+x2+x3dx (May – 2012)
Answer:
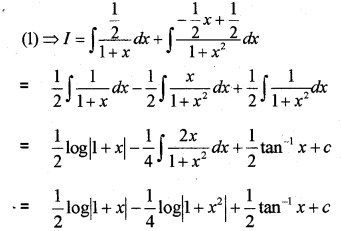
Question 8.
(i) Evaluate ∫30f(x)dx
where f(x)={x+3,0≤x≤23x,2≤x≤3
(ii) Prove that ∫10log(x1−x)dx=∫10log(1−xx)dx Find the value of ∫10log(x1−x)dx (May – 2012)
Answer:
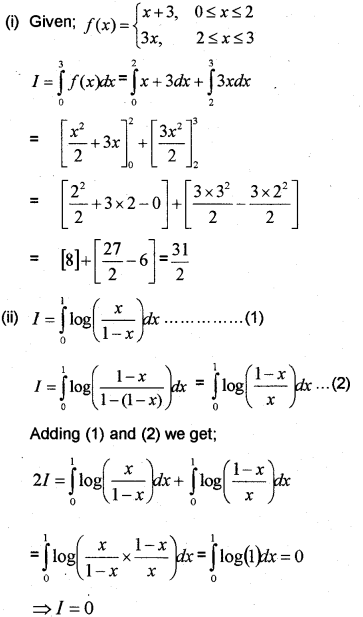
Question 9.
(i) Find ∫cotxdx=……
(ii) Find ∫xlogxdx
(iii) Find ∫x−1(x−2)(x−3)dx (March – 2013)
Answer:
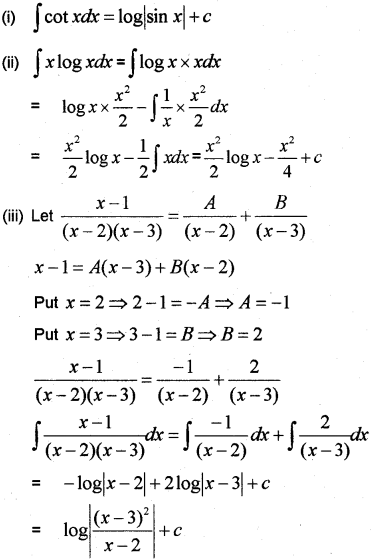
Question 10.
Evaluate
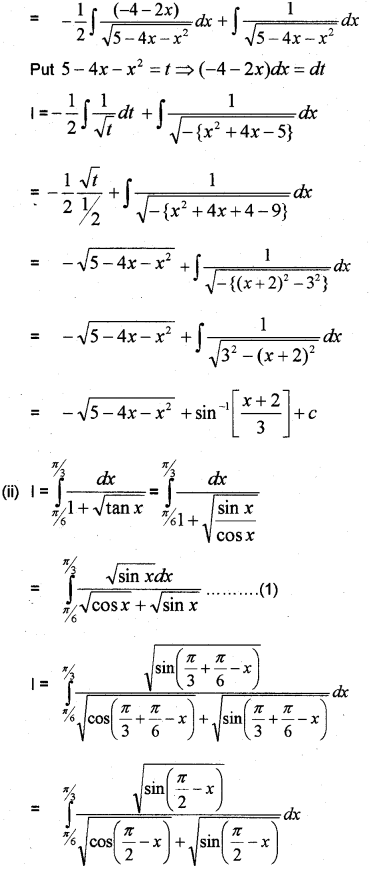
(i) ∫x+3√5−4x−x2dx
(ii) ∫π/3π/6dx1+√tanx (May – 2013)
Answer:
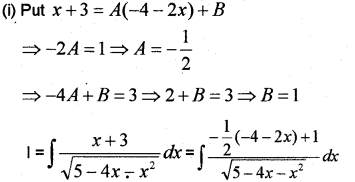
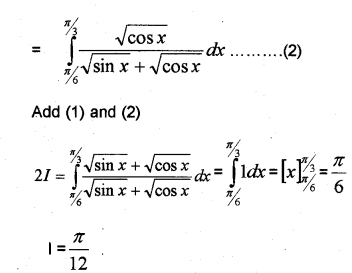
Question 11.
Evaluate
(i) ∫x2tan−1xdx (ii) ∫2−1x3−xdx (May – 2013)
Answer:
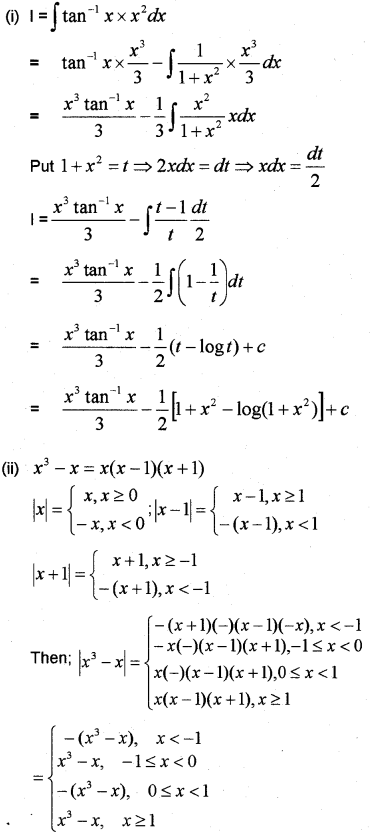
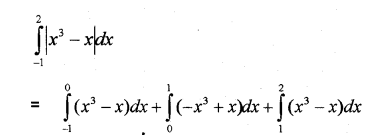
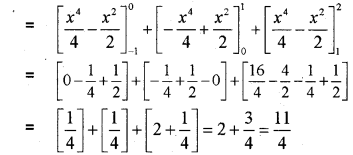
Question 12.
Evaluate ∫π/40log(1+tanx)dx (March – 2013)
Answer:
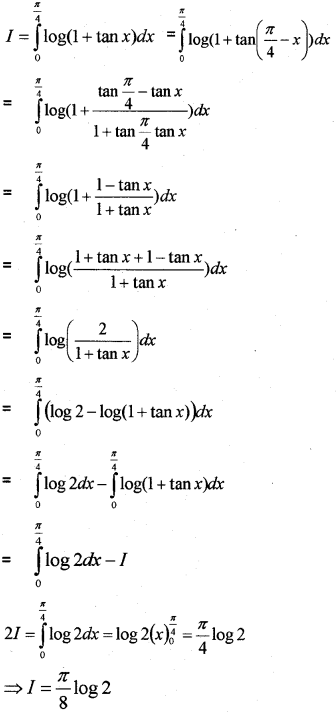
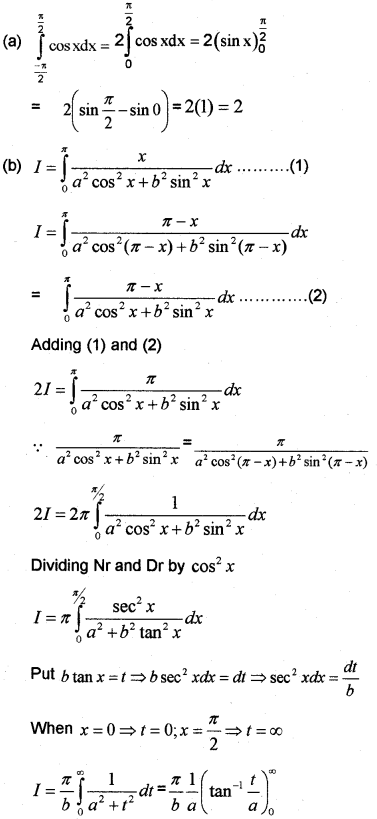
Question 13.
(a) The value of ∫π2−π2cosxdx (May – 2014)
(b) Prove that ∫π0xa2cos2x+b2sin2xdx=π22ab
Answer:
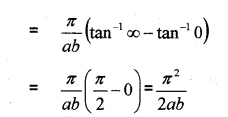
Question 14.
(a) ∫1x2+a2dx=
(b) Find ∫19x2+6x+5dx
(c) Find ∫x(x−1)2(x+2)dx (May – 2014)
Answer:
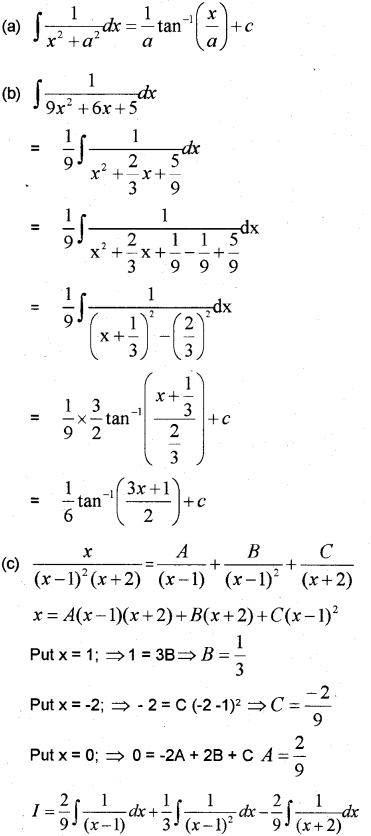
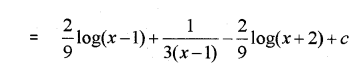
Question 15.
Integrate the following
(a) x−1x+1 (b) sinxsin(x−a) (c) 1√3−2x−x2 (March – 2015)
Answer:
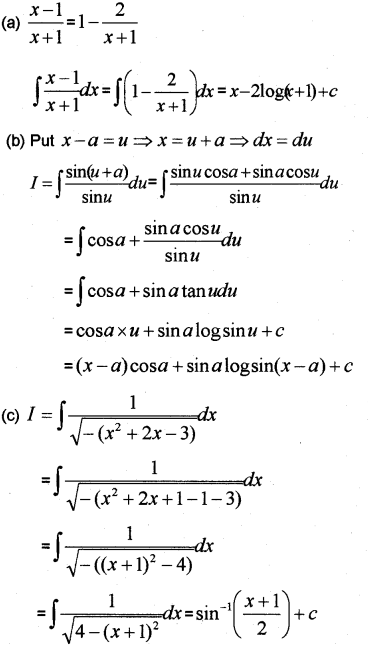
Question 16.
(a) Prove that ∫cos2xdx=x2+sin2x4+c
(b)Find ∫1√2x−x2dx
(c) Find ∫xcosxdx (May – 2015)
Answer:
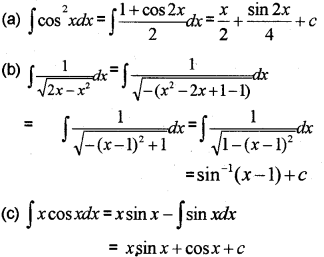
Question 17.
Find the following:
(i) ∫1x(x7+1)dx (ii) ∫41|x−2|dx
Answer:
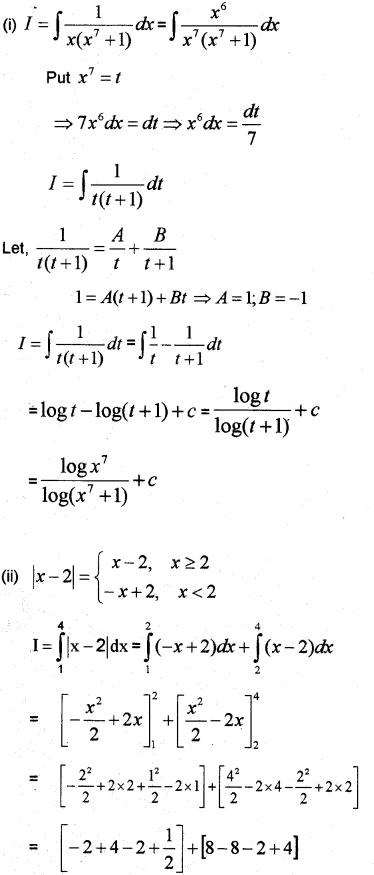
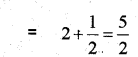
Question 18.
Find ∫π20logsinxdx
Answer:
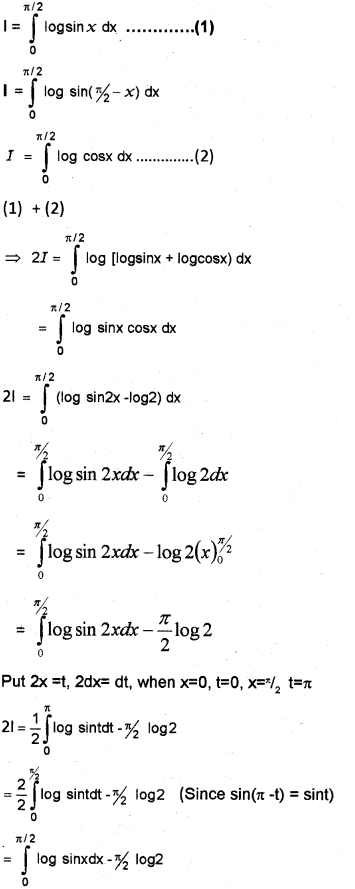
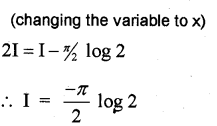
Question 19.
Find the following: (i) ∫cotxlog(sinx)dx (ii) ∫1x2+2x+2dx (iii) ∫xe9xdx (May – 2017)
Answer:
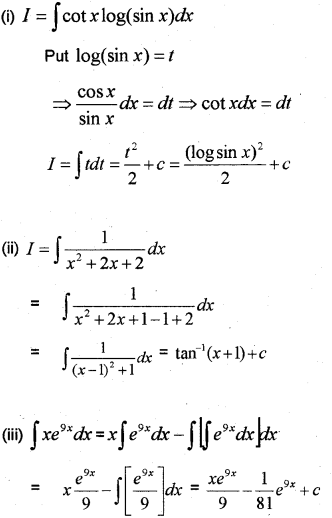
We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 7 Integrals help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 7 Integrals, drop a comment below and we will get back to you at the earliest.
