Plus Two Maths Chapter Wise Previous Questions Chapter 5 Continuity and Differentiability are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 5 Continuity and Differentiability.
Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 5 Continuity and Differentiability
Plus Two Maths Continuity and Differentiability 3 Marks Important Questions
Question 1.
Consider f(x)={x2−x−6x+2,x≠−2−5,x=−2
(i) Find f(-2)
(ii) Check whether the function f(x) is continuous at x= -2. (March – 2009)
Answer:
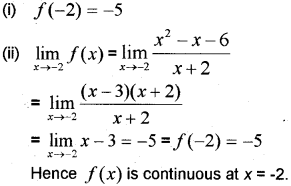
Question 2.
If f(x) = sin(Log x), prove that x2 y2 + xy1 + y = 0 (May -2009)
Answer:
Given; y sin(Iogx)
Differentiating with respect to X;
y1=cos(logx)1x⇒xy1=cos(logx)
Again differentiating with respect to x
⇒xy2+y1=−sin(logx)1x⇒x2y2+xy1=−y⇒x2y2+xy1+y=0
Question 3.
(i) Establish that g(x) =1 – x + |x| is continuous at origin.
(ii) Check whether h(x) = |l – x + |x|| is continuous at origin. (March – 2010)
Answer:
(i) Given; g(x) = 1 – x + |x| ⇒ g(x) (1 – x) + |x|
Here g(x) is the sum of two functions continuous functions hence continuous.
(ii) We have;
fog(x)=f(g(x))=f(1−x+|x|)=|1−x+|x∣=h(x)
The composition of two continuous functions is again continuous. Therefore h(x) continuous.
Question 4.
Find dydx of the following
(i) x=√asin44y=√acos−1t (ii) y=cos−1(1−x2)(1+x2),0<x<1 (iii) y=sin−12x√1−x2,y√2<x<y√2
Answer:
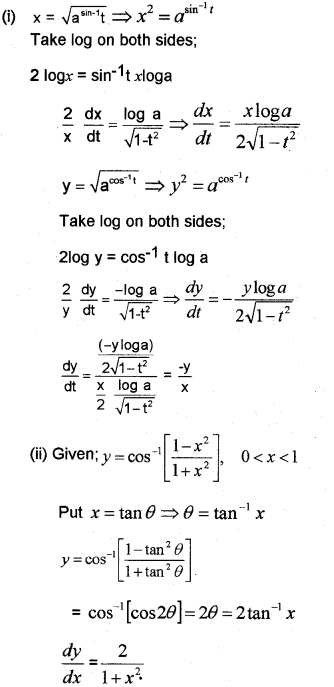
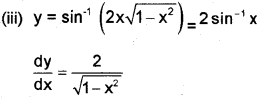
Question 5.
Find dydx if x3+2x2y+3xy2+4y3=5 (March – 2015)
Answer:
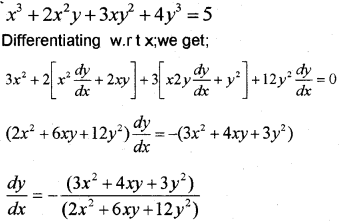
Question 6.
Find all points of discontinuity of f where f is defined by f(x)={2x+3,x≤22x−3,x>2 (March – 2016)
Answer:
In both the intervals x \(\leq[latex] 2 and x > 2 the function f(x) is a polynomial so continuous. So we havetocheckthe continuity at x = 2.
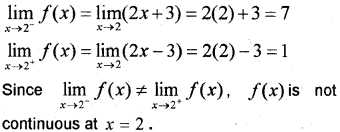
Question 7.
If ex-y = xy, then prove that [latex]\frac{d y}{d x}=\frac{\log x}{[\log \operatorname{ex}]}\) (May – 2014; March – 2016)
Answer:
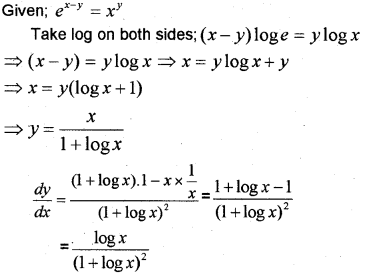
Plus Two Maths Continuity and Differentiability 4 Marks Important Questions
Question 1.
Find dydx of the following (March – 2009)
(i) y=sin−1(3x−4x3)+cos−1(4x3−3x) (ii) y=tan−1(√1−cosx1+cosx)
Answer:
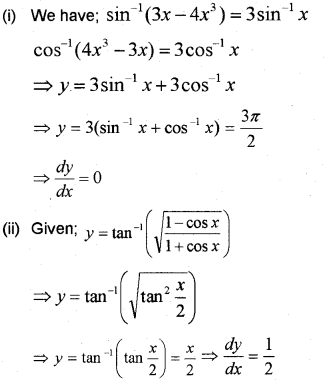
Question 2.
Consider the function f(x) = |x| x ∈ R
(i) Draw the graph of f(x) =|x|
(ii) Show that the function is continuous at x = 0. (March – 2010)
Answer:
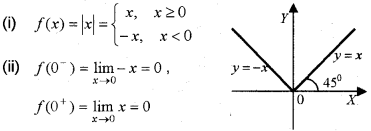
f(0–) f(0–) = 0. therefore continuous at x = 0.
AIso from the figure we can see that the graph does not have a break or jump.
Question 3.
(i) Find the derivative of y = xa + ax with respect to x.
(ii) If ey (x + 1) = 1 , showthat d2ydx2=(dydx)2 (May – 2011)
Answer:

Question 4.
(i) Check the continuity of the function given by f(x) f(x)={xsin1x,x≠01,x=0
(ii) Verify Mean Value Theorem for the function f(x) = x + 1/x in the interval [1,3]. (May – 2011)
Answer:
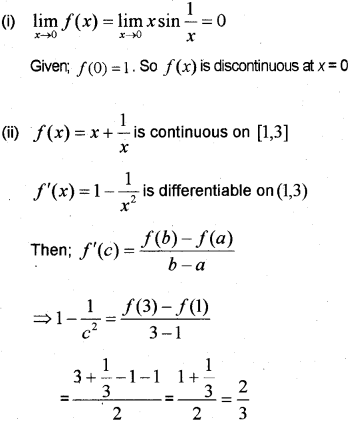
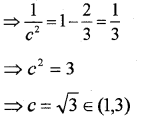
Hence Mean Value Theorem ¡s verified.
Question 5.
(i) Determine the value of k so that the function (May – 2012)
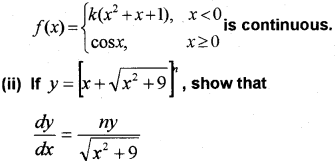
Answer:

Question 6.
Consider a fUnction f: R → R defined by
f(x)={a+x,x≤2b−x,x>2
(i) Find a relation between a and b if f is continuous at x = 2.
(ii) Find a and b, if f is continuous at x2 and a + b = 2. (May – 2013)
Answer:
(i) Since fis continuous at x = 2, we have;
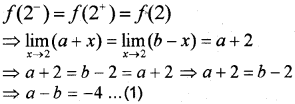
(ii) Given a = 2 …(2) Solving (1) and (2) we have;
⇒ 2a = – 2 ⇒ a = – 1
⇒ b = 2 – a = 2 + 1 = 3
Question 7.
(i) Find if x = a(t – sin t) y = a(1 + cos t)
(ii) Verify Rolles theorem for the function f(x) = x2 + 2 in the interval [-2, 2] (March – 2014)
Answer:
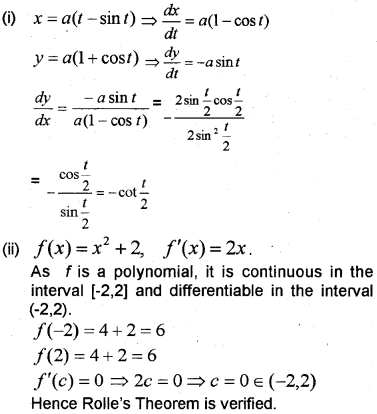
Question 8.
(a) Find the relationship between a and b so that the function f defined by
f(x)={ax2−1,x≤2bx+3,x>2 is continuous.
(b) Verify mean value theorem for the function f(x) = x2 – 4x -3 ¡n the interval [1, 4]. (May – 2014)
Answer:
(a) Since fis continuous
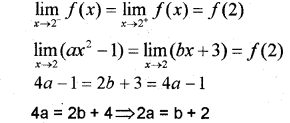
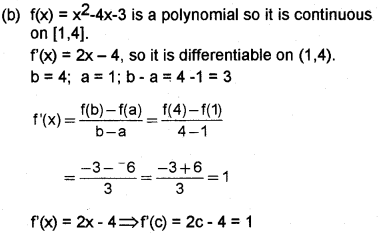
Hence mean value theorem satisfies for the funcion.
Question 9.
(a) Find ‘a’ and ‘b’ if the function
f(x)={sinxx,−2≤x≤0a×2x,0≤x≤1b+x,1<x≤2 is continous on [-2, 2]
(b) How many of the functions
f(x) = |x|, g(x) = |x|2, h(x) = |x|3 are not differentiable at x = 0?
(i) 0
(ii) 1
(iii) 2
(iv) 3 (March – 2015)
Answer:
(a) Since f(x) is continuous on [-2, 2]
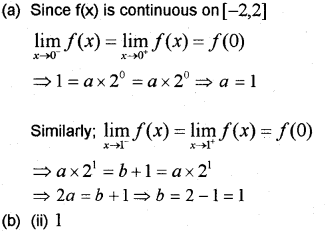
Question 10.
(a) Find the relation between ‘a’ and ‘b’ if the function f defined by
f(x)={ax+1,x≤3bx+3,x>3 is continuous.
lbx+3.x>3
(b) If ey (x + 1) = 1, show thats d2ydx2=(dydx)2 (May -2015)
Answer:
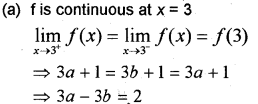
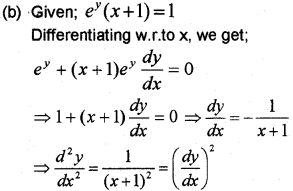
Question 11.
Find the value of a and b such that the function f(x)={5ax≤0asinx+cosx0<x<π2b−π2x≥π2 is continuous. (March – 2010)
Answer:
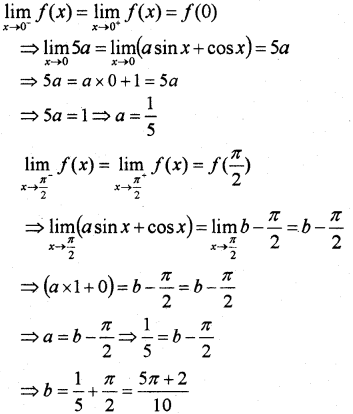
Question 12.
(i) Find dydx, if x=acos2θ;y=bsin2θ
(ii) Find the second derivative of the function y = ex sinx. (May – 2017)
Answer:
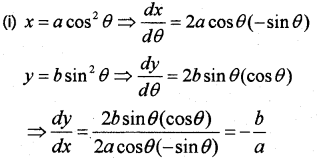
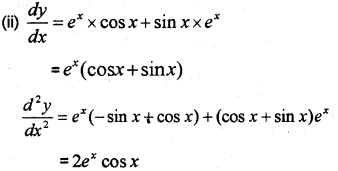
Question 13.
Find dydx of the following (4 score each)
(i) yx = xy (May – 2015)
(ii) (COSx)y = (cosy)x (March – 2017)
Answer:

Plus Two Maths Continuity and Differentiability 6 Marks Important Questions
Question 1.
Find dydx if
(i) sinx + cosy = xy
(ii) x = acos3t, y = asin3t
(iii) y = xx + (logx)x (May -2009; May -2011; March -2015)
Answer:
(i) Given; sinx + cosy = xy
Differentiating with respect to x;
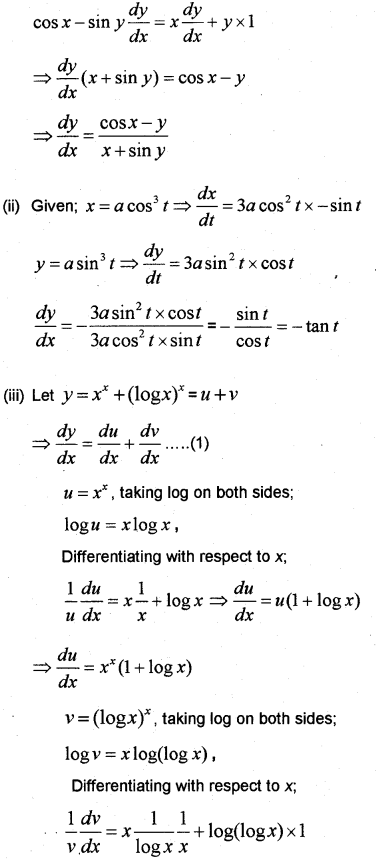
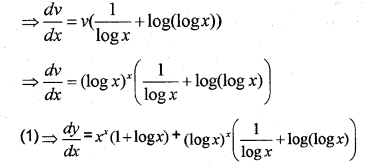
Question 2.
(i) Let y =3 cos(log x) + 4 sin (log x)
(a) Find dydx
(b) Prove that x2 y2 + xy1 + y = 0
(ii) (a) Find the derivative of y = e2x+logx
(b) Find dydx
if x = a (θ – sinθ), y = a(1 – cosθ) (March – 2009)
Answer:
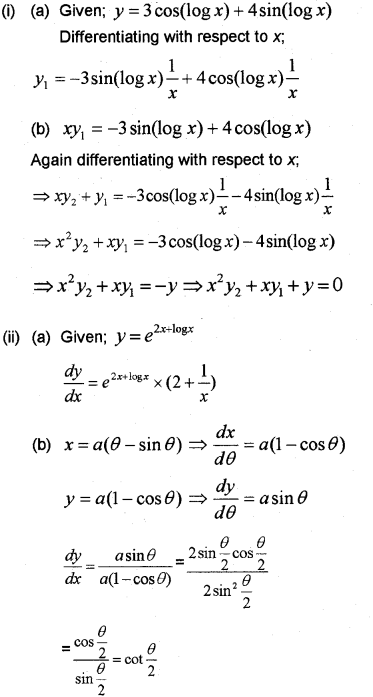
Question 3.
(i) Show that the function f (x) defined by f(x) = sin (cosx) is a continuous function.
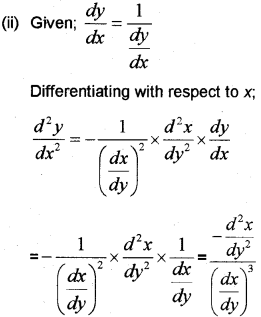
(ii) If dydx=1dxdy, Show that d2ydx2=−d2xdy2(dxdy)3 (May -2010)
Answer:
Given; f(x) = sin(cos x)
Let g(x) = sin(x) and h(x) = cos x
Both these function are trigonometric functions hence continuous.
goh(x) = g(h(x)) = g(cos x) = sin(cos x) = f(x)
Since f(x) is the composition of two continuous functions, hence continuous.
Question 4.
(i) Let y = xsin x + (sinx)x. Find dydx
(ii) Given; y=√tan−1x
(a) 2(1+x2)ydydx=1
(b) (1+x2)yd2ydx2+(1+x2)(dydx)2+2xydydx=0 (May – 2010; Onam – 2017)
Answer:
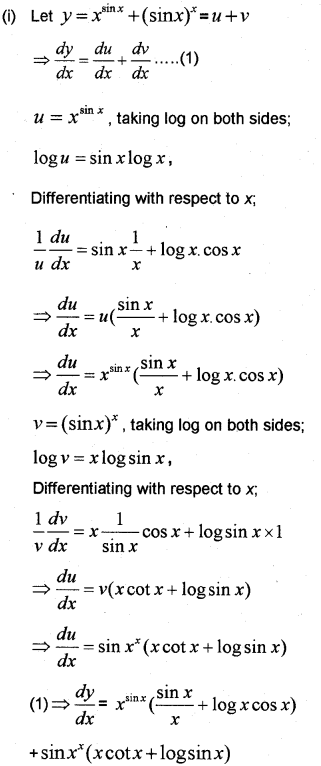
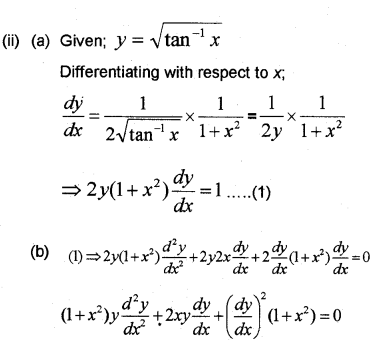
Question 5.
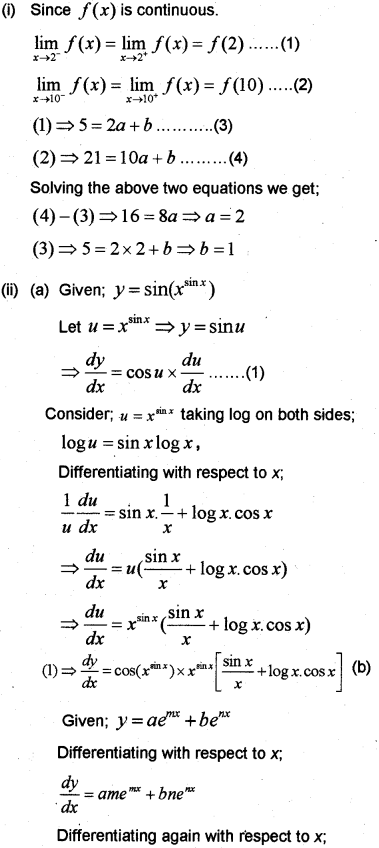
(i) The function f(x)={5,x≤2ax+b,2<x<10 is 21,x≥10 continuous. Find a and b
(ii) Find dydx (a) if y = Sin (xsinx)
(iii) If y = ae” + be’; show that d2ydx2−(m+n)dydx+mny=0 (March – 2011)
Answer:
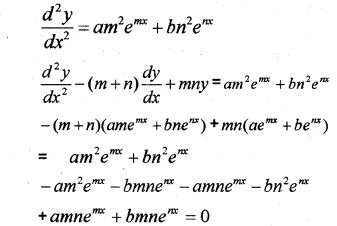
Question 6.
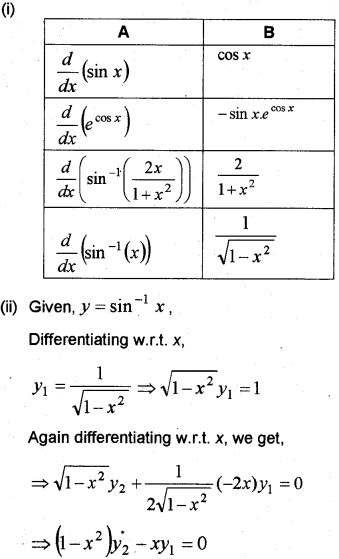
(i) Match the following.
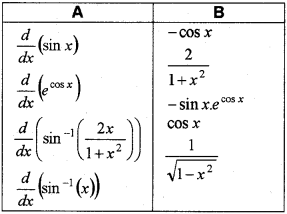
(ii) If y = sin-1 x, prove that (1 – x2) y2 – xy1 = 0 (March – 2012; May -2017)
Answer:
Question 7.
(i) Consider f(x)={3x−8,x≤52k,x>5 Find the value of k if f(x) is continuous at x = 5.
(ii) Find dydx, if y=(sinx)logx,sinx>0
(iii) If y = (sin-1 x)2, then show that (1−x2)d2ydx2−xdydx=2. (March -2013)
Answer:

Question 8.
(i) Find, if y = 1ogx, x>0
(ii) Is f(x) = |x| differentiable at x = 0?
(iii) Find if x = sin θ – cos θ and y= sinθ + cosθ (May – 2013)
Answer:
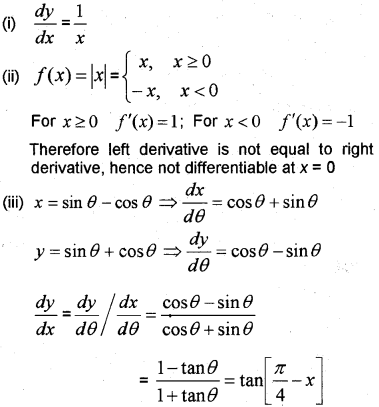
We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 5 Continuity and Differentiability help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 5 Continuity and Differentiability, drop a comment below and we will get back to you at the earliest.
