Plus Two Maths Chapter Wise Previous Questions Chapter 4 Determinants

Plus Two Maths Chapter Wise Previous Questions Chapter 4 Determinants are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 4 Determinants.

Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 4 Determinants

Plus Two Maths Determinants 3 Marks Important Questions

Question 1.
Prove that $\begin{array}{|lll|} 1 ! & 2 ! & 3 ! \\ 2 ! & 3 ! & 4 ! \\ 3 ! & 4 ! & 5 ! \end{array}$ (March – 2015)
Answer:

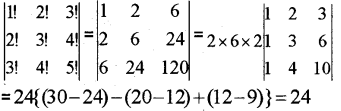
Question 2.
Using properties of determinants prove the following. (March – 2010; Christmas -2017)
Answer:

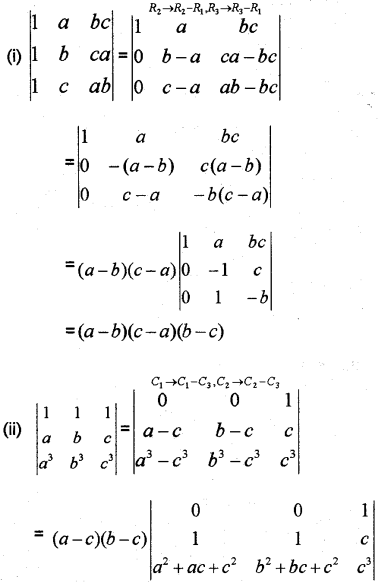
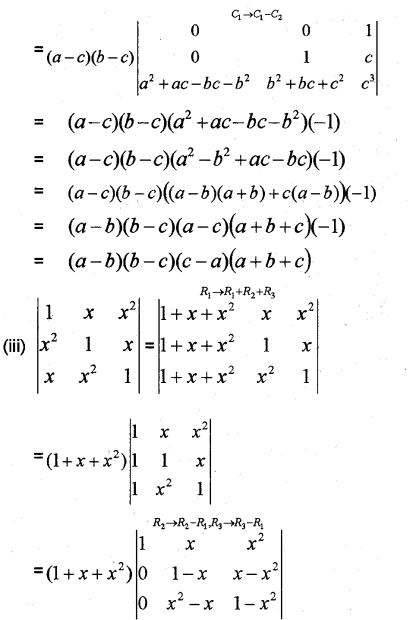
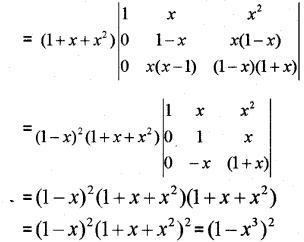
Plus Two Maths Determinants 4 Marks Important Questions

Question 1.
Consider the matrix $A=\left[\begin{array}{ll} 2 & 5 \\ 3 & 2 \end{array}\right]$

(i) Find adj (A)
(ii) Find A1
(iii) Using A^-1 solve the system of linear equations 2x + 5y = 13x + 2y = 7 (March – 2010)
Answer:

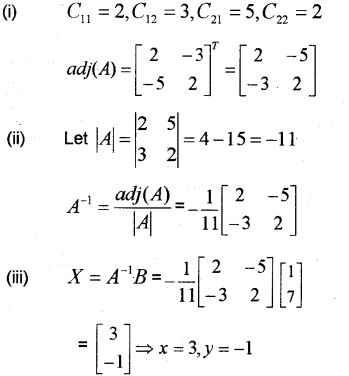
Plus Two Maths Determinants 6 Marks Important Questions

Question 1.
Consider the matrix $A=\left[\begin{array}{lll} a & b & c \\ b & c & a \\ c & a & b \end{array}\right]$

(i) Using the column operat,on
C₁ → C₁ + C₂ + C₃,
show that $|A|=(a+b+c)\left|\begin{array}{ccc} 1 & b & c \\ 1 & c & a \\ 1 & a & b \end{array}\right|$
(ii) Show that |A| = – (a3 + b3 + e3 — 3abc)
(iii) Find A x adj(A) if a = 1,b = 10,c = 100 (May – 2010)
Answer:

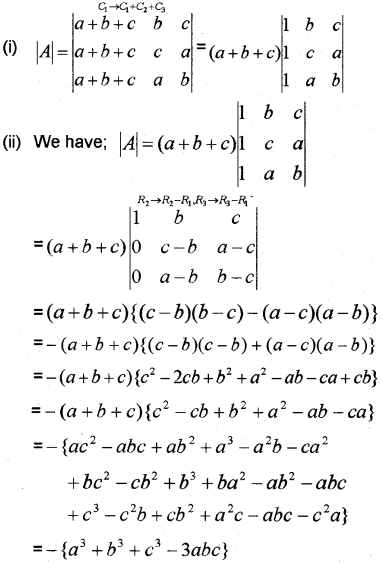
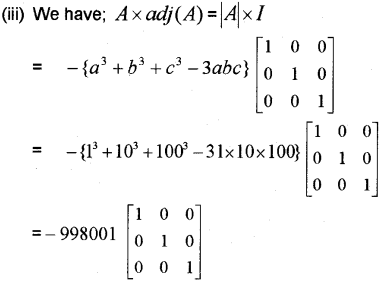
Question 2.
(i) (a) If $A=\left[\begin{array}{ccc} 1 & 1 & 5 \\ 0 & 1 & 3 \\ 0 & -1 & -2 \end{array}\right]$

What is the value of |3A|?
(b) Find the equation of the line joining the points (1,2) and (-3,-2) using determinants.
(ii) Show that $\left|\begin{array}{lll} 1 & a & a^{2} \\ 1 & b & b^{2} \\ 1 & c & c^{2} \end{array}\right|=(a-b)(b-c)(c-a)$
Answer:

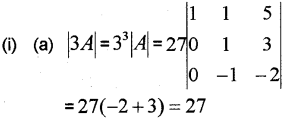
(b) Let (x,y) be the coordinate of any point on The line, then (1,2), (-3, -2) and (x, y) are collinear.

Hence the area formed will be zero.

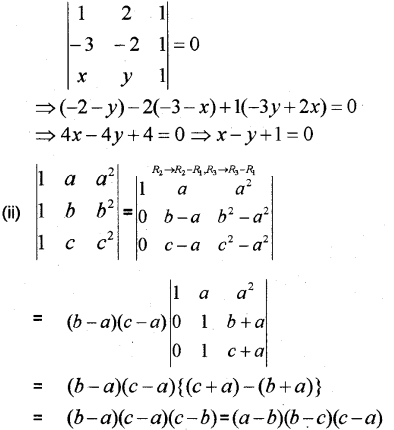
Question 3.
Consider the following system of linear equations; x + y + z = 6, x – y + z = 2, 2x + y + z = 1
(i) Express this system of equations in the Standard form AXB
(ii) Prove that A is non-singular.
(iii) Find the value of x, y and z satisfying the above equation. (May – 2011)
Answer:

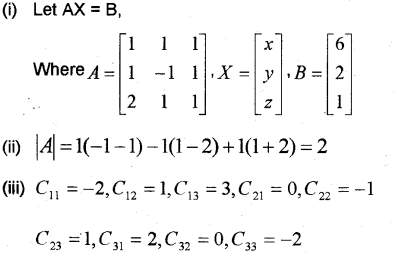
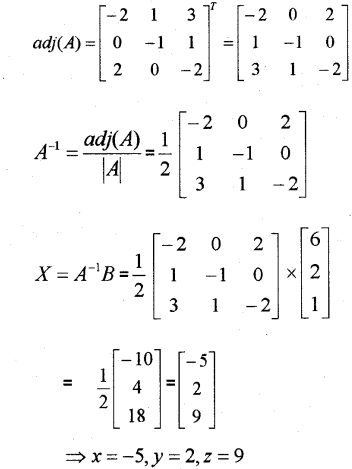
Question 4.
(i) lf $\left|\begin{array}{ll} x & 3 \\ 5 & 2 \end{array}\right|=5$, then x = ………..
(ii) Prove that
$\left|\begin{array}{ccc} y+k & y & y \\ y & y+k & y \\ y & y & y+k \end{array}\right|=k^{2}(3 y+k)$
(iii) Solve the following system of linear Equations, using matrix method; 5x + 2y = 3, 3x + 2y = 5 (March – 2012)
Answer:

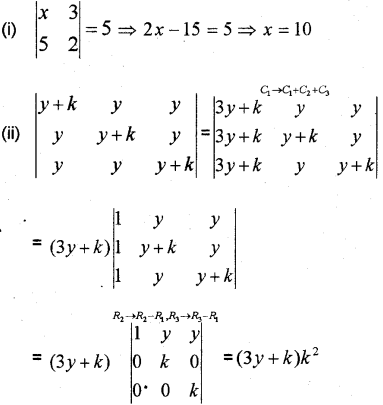
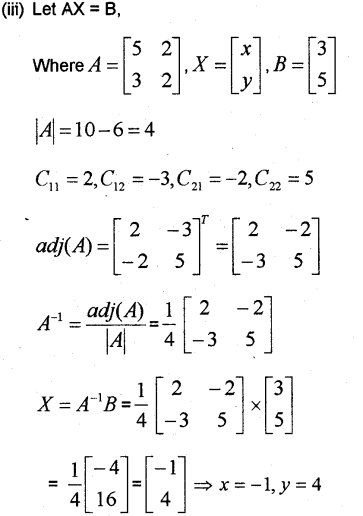
Question 5.
(i) Let B is a square matrix of order 5, then |kB| is equal to ………..
(a) |B|
(b) k|B|
(c) k⁵|B|
(d) 5|B|

(ii) Prove that $\left|\begin{array}{lll} 1 & x & x^{2} \\ 1 & y & y^{2} \\ 1 & z & z^{2} \end{array}\right|=(x-y)(y-z)(z-x)$
(iii) Check the consistency of the following equations; 2x + 3y + z = 6, x + 2y – z = 2, 7x + y + 2z =10 (May – 2012)
Answer:

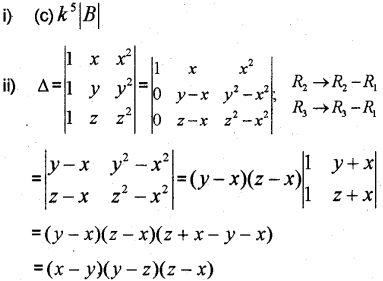
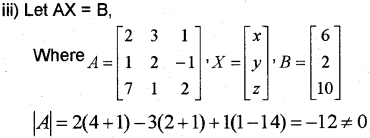
Therefore the system is consistent and has unique solutions.

Question 6.
(i) Find the values of x in which $\left|\begin{array}{ll} 3 & x \\ x & 1 \end{array}\right|=\left|\begin{array}{ll} 3 & 2 \\ 4 & 1 \end{array}\right|$

(ii) Using the property of determinants, show that the points A(a,b + c), B(b,c + a), C(c,a + b) are collinear.
(iii) Examine the consistency of system of following equations: 5x – 6y + 4z = 15, 7x + y – 3z = 19, 2x + y + 6z = 46 (EDUMATE – 2017; March – 2013)
Answer:

Since, the system is consistent and has unique solutions.

Question 7.
Consider a system of linear equations which is given below;
$\begin{array}{l} \frac{2}{x}+\frac{3}{y}+\frac{10}{z}=4 ; \frac{4}{x}-\frac{6}{y}+\frac{5}{z}=1 \\ \frac{6}{x}+\frac{9}{y}-\frac{20}{z}=2 \end{array}$

(i) Express the above equation in the matrix form AX = B.
(ii) Find A^-1, the inverse of A.
(iii) Find x,y and z. (May – 2013)
Answer:

Question 8.
Consider the matrices $A=\left[\begin{array}{ll} 2 & 3 \\ 4 & 5 \end{array}\right]$

(i) Find A² – 7A – 21 = 0
(ii) Hence find A-1
(iii) Solve the following system of equations using matrix method 2x + 3y = 4; 4x + 5y = 6 (March – 2014)
Answer:

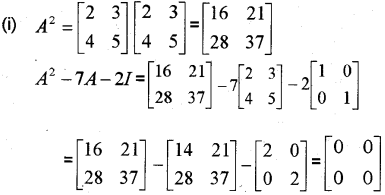
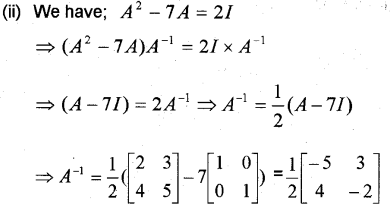
(iii) The given system of equations can be converted into matrix form AX = B

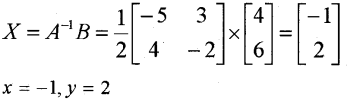
Question 9.
(i) Let A be a square matrix of order 2 x 2 then |KA| is equal to
(a) K|A|
(b) K²|A|
(c) K³|A|
(d) 2K|A|

(ii) Prove that
$\left|\begin{array}{ccc} \mathbf{a}-\mathbf{b}-\mathbf{c} & \mathbf{2 a} & 2 \mathbf{a} \\ 2 \mathrm{~b} & \mathrm{~b}-\mathrm{c}-\mathrm{a} & 2 \mathrm{~b} \\ 2 \mathrm{c} & 2 \mathrm{c} & \mathrm{c}-\mathrm{a}-\mathrm{b} \end{array}\right|=(\mathrm{a}+\mathrm{b}+\mathrm{c})^{3}$

(iii) Examine the consistency of the system of Equations. 5x + 3y = 5; 2x + 6y = 8 (May- 2014)
Answer:

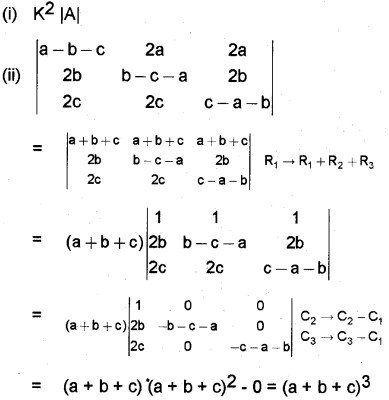
(iii) The given system of equation can be written in matrix form as

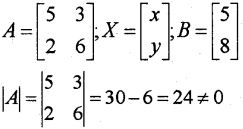
solution exist and hence it is consistent.

Question 10.
(a) Choose the correct statement related to the matnces $A=\left[\begin{array}{ll} 1 & 0 \\ 0 & 1 \end{array}\right], B=\left[\begin{array}{ll} 0 & 1 \\ 1 & 0 \end{array}\right]$
$\begin{array}{l} \text { (i) } A^{3}=A, B^{3} \neq B \\ \text { (ii) } A^{3} \neq A, B^{3}=B \\ \text { (iii) } A^{3}=A, B^{3}=B \\ \text { (iv) } A^{3} \neq A, B^{3} \neq B \end{array}$

(b) lf $M=\left[\begin{array}{ll} 7 & 5 \\ 2 & 3 \end{array}\right]$ then verity the equation M² – 10M + 11 I₂ = O

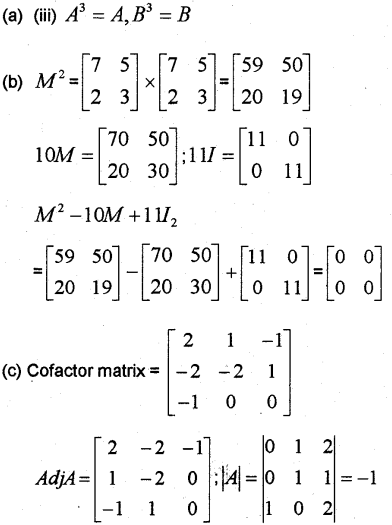
(c) Inverse of the matrix $\left[\begin{array}{lll} 0 & 1 & 2 \\ 0 & 1 & 1 \\ 1 & 0 & 2 \end{array}\right]$ (March – 2015)
Answer:

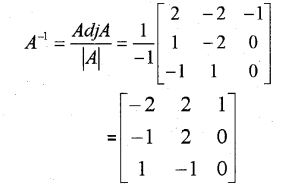
Question 11.
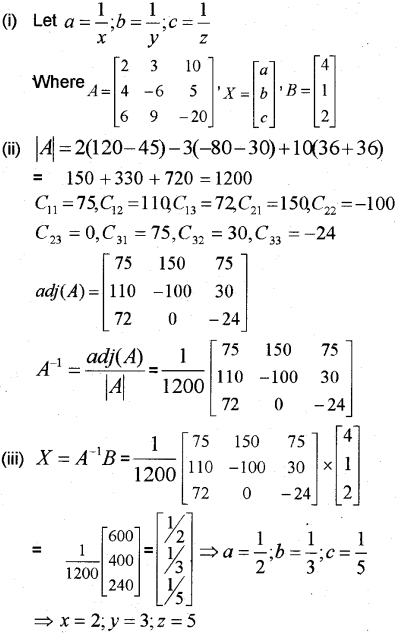
Solve the system of Linear equations x + 2y + z = 8; 2x + y – z = 1; x – y + z = 2 (March – 2015)
Answer:

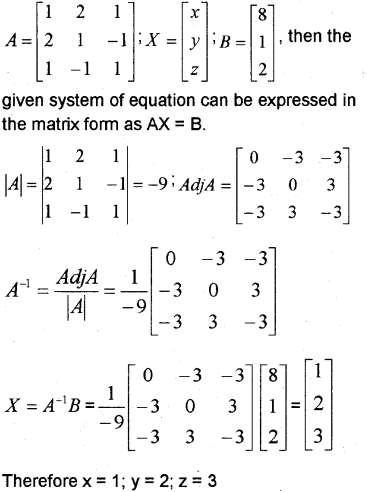
Question 12.
(a) If $\left|\begin{array}{ll} x & 1 \\ 1 & x \end{array}\right|=15$ then find the value of X.

(b)Solve the following system of equations 3x – 2y + 3z = ?, 2x + y – z = 1 4x – 3y + 2z = 4 (May – 2015)
Answer:

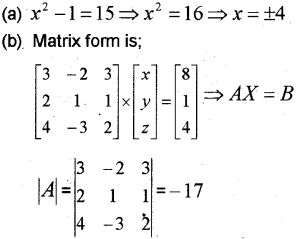
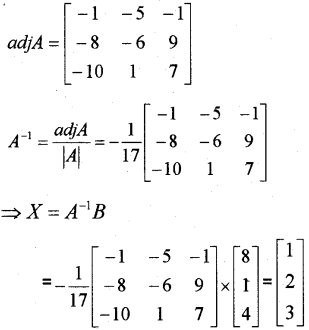
Question 13.
(i)The value of the determinant $\left|\begin{array}{ccc} 1 & 1 & 1 \\ 1 & -1 & -1 \\ 1 & 1 & -1 \end{array}\right|$ is
(a) -4
(b) 0
(c) 1
(d) 4

(ii) Using matrix method, solve the system of linear equations x + y + 2z = 4; 2x – y + 3z = 9; 3x – y – z = 2 (May – 2016)
Answer:
(i) (d) 4
(ii) Express the given equation in the matrix form as AX = B

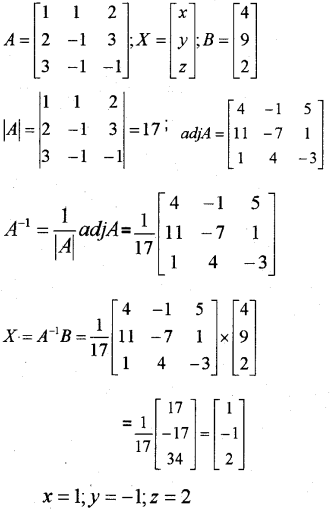
Question 14.
(i) If $A=\left[\begin{array}{ll} a & 1 \\ 1 & 0 \end{array}\right]$ is such that A² = I then a equals
(a) 1
(b) -1
(c) 0
(d) 2

(ii)Solve the system of equations x – y + z = 4; 2x + y – 3z = 0; x + y + z = 2 Using matrix method. (March – 2017)
Answer:

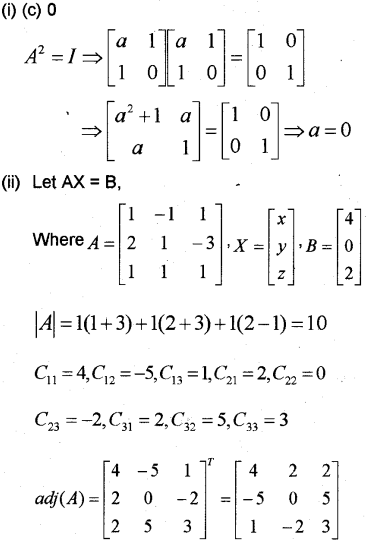
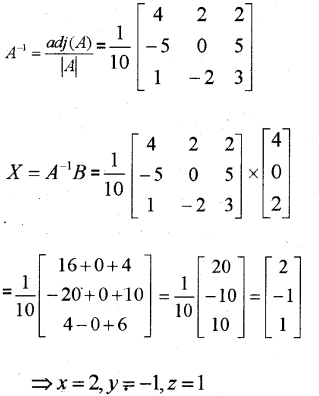
Question 15.
(i) IfA is a 2 x 2 matrix with |A| = 5, then |adjA| is
(a) 5
(b) 25
(c) 1/5
(d) 1/25

(ii) Solve the system of equations using matrix method.
x + y + z = 1; 2x + 3y – z = 6; x – y + z = -1 (May – 2017)
Answer:
(i) (a) 5
(ii) LetA X=B,

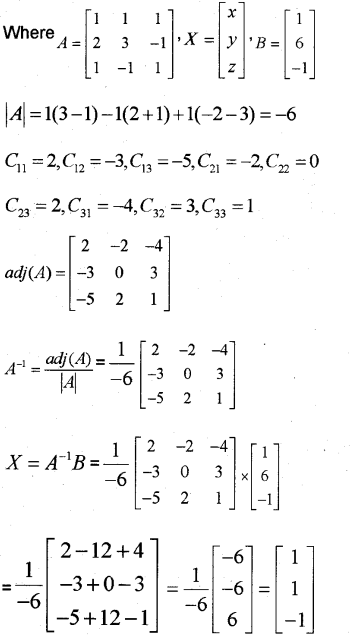
We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 4 Determinants help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 4 Determinants, drop a comment below and we will get back to you at the earliest.