Plus Two Maths Chapter Wise Previous Questions Chapter 13 Probability are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 13 Probability.
Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 13 Probability
Plus Two Maths Probability 3 Marks Important Questions
Question 1.
Suppose 10 cards numbered I to lo are placed in a box and shuffled and one card ¡s drawn at random.
(i) If A is the event that the number on the card is even, then write A.
(ii) If B is the event that the number on the card is more than 3, Find P(A/B). (May – 2010)
Answer:
A = {2,4,6,8,10}
B = {4, 5, 6, 7, 8, 9, 10}
A ∩ B = {4,6,8,10}
P(A/B)=P(A∩B)P(B)=410710=47
Question 2.
P(A)=512,P(B)=712,P(A∩B)=14
Find P(A/B) (March – 2010)
(ii) And B try independently to solve a problem.
The probability that A solves it 1/3 & that B is 3/5. Find the probability that the problem is solved.
Answer:
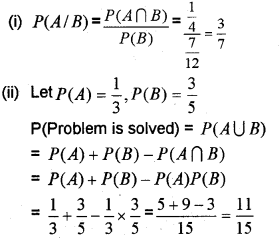
Question 3.
(i) If X is a random variable whose possible values x1, x2, …………….., xn are occur with probabilities p1, p2, ……… pn respectively, then E(X) =……..
(ii) A husband and wife appears for an Interview for 2 posts. The probability of husband selection is 1/7 and that of wife is 1/5. What is the probability that one is selected? (May – 2011)
Answer:
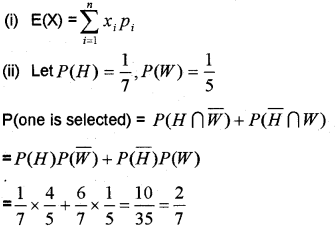
Question 4.
Two balls are drawn at random with replacement from a box containing 10 black aid 8 red balls. Find the probability that
(a) Both the balls are red.
(b)One of them is black and the other is red. (May – 2014)
Answer:
(a) P (both all red) =818×818=1681
(b) P (one of them is black and other red) = P(First ball black, second red) or P (First red, second black) =1018×818+818×1018=2081+2081=4081
Question 5.
(a) For two independent events A and B, which of the following pair of events need not be independent?
(i) A’, B’
(ii) A,B’
(iii) A’,B
(iv) A-B, B-A
(b) it P(A) = 0.6; P(B) = 0.7 and P(A U B) = 0.9 , then find P(A/B) and P(B/A) (March – 2015)
Answer:
(a) A – B, B – A
(b) P(A∩B) = P(A) + P(B) -P(A∪B)
= 0.6 + 0.7 – 0.9 = 0.4
P(A/B)=P(A∩B)P(B)=0.40.7=47
Plus Two Maths Probability 4 Marks Important Questions
Question 1.
The probability distribution of a random variable X is given below
(i) Find the value of k.
(ii) Find the mean and variance of the variable. (May – 2010)
Answer:
(i) We have sum of the probabilities ¡s 1.
k + 2k + 3k + 4k + 5k + 5k = 1 ⇒ k = 1/20
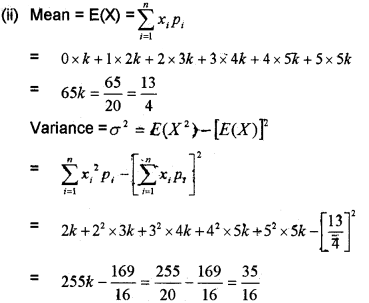
Question 2.
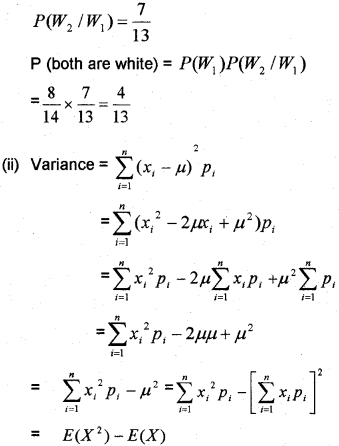
(i) An urn contains 8 white and 6 black balls. Two are drawn from the urn one after the other without replacement. What is the probability that both drawn balls arewhite?
(ii) Prove that Variance = E(X2) – [E(x)]2 (March – 2010)
Answer:
Describe the events as follows.
W1 : First ball is white.
W2 : Second ball is white.
P(W1)=814
Since the event is executed without replacement. The white ball number will be 7 and total will be 13.
Question 3.
(i) For any two events A and B, write the expression for P(A/B).
(ii) In a bulb factory, machine A, B and C manufacture60%, 30% and 10% bulbs respectively. 1%, 2% and 3%-of the bulbs produced byA, B and C respectively are defective. A bulb is drawn at randomfror the totaL “ production and found to b defective. Find the probability that-this has been produced from machine A. (May – 2011)
Answer:
(i) P(A/B)=P(A∩B)P(B)
(ii) Describe the events as follows.
D: Getting a defective bulb.
A: Machine A. B: Machine B. C: Machine C.
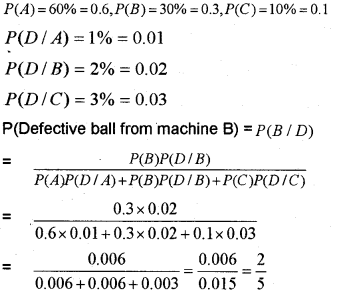
Question 4.
(i) Two balls are drawn with replacement from a box containing lo black and 8 red balls. Find the probability that one of them is black and other is red.
(ii) Find the probability of getting 5 exactly twice in 7 throw of a die. (March – 2012)
Answer:
Describe the events as follows.
B1, B2 : first, second black.
R1, R2 : first, second red.
P(one black and other red) = P(B1 R2) + P(R1 B2)
= P(B1) P(R2/B1) + P(R1 ) P(B2/R1)
=1018×818+818×1018=4081
(ii) Let X denotes the random variable of number of 5 in a throw of a die.
Clear X has a Binomial Distribution with n = 7
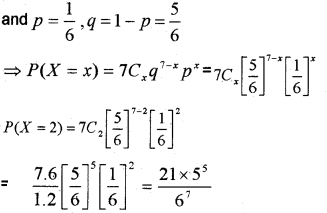
Question 5.
(i) Write the probability function of Binomial Distribution.
(ii) Five Defective bulbs are accidentally mixed with 20 good ones. It is not possible to just look at a bulb and tell whether or not it is defective. Find the probability distribution of the number of defective bulbs if 3 bulbs are drawn at random. (May – 2011)
Answer:
(i) P(X = x) = nCxqn-xpx
(ii) Let X denotes the random vanable of number of defective bulbs. Then X can take values 0, 1, 2, 3 D: Getting a defective bulb.
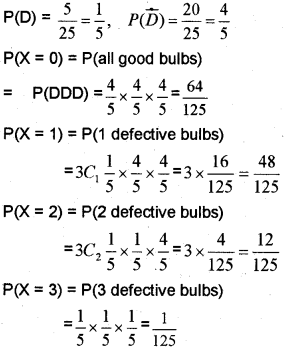
The required Probability Distribution is
| X | 0 | 1 | 2 | 3 |
| P (X) | 64/125 | 48/125 | 12/125 | 1/125 |
Question 6.
(i) Two balls are drawn with replacement from a box containing 10 black and 8 red balls. Find the probability that one of them is black and other ¡s red.
(ii) Find the probability of getting 5 exactly twice ¡n 7 throw of a die. (March – 2012)
Answer:
Describe the events as follows.
B1, B2: first,second black.
R1, R2: first, second red.
P(one black and other red) = P(B1R,)÷P(R1B2)
P(B1) P(R2/B1) + P(R1) P(B2/R1)
=1018×818+818×1018=4081
(ii) Let X denotes the random variable of number of 5 in athrowofa die.
Clearly X has a Binomial Distribution with n = 7
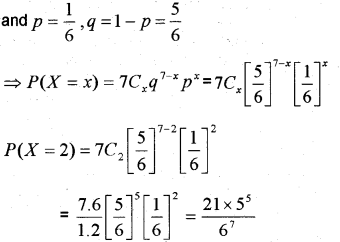
Question 7.
(i) A die is tossed thrice. Find the probability of getting an odd number at least once.
(ii)Bag I contains 3 red and 4 black balls while another Bag Il contains 5 red and 6 black balls. One ball is drawn at random from one of the Bag it is found to be red. Find the probability that it was drawn from Bag II. (March – 2012)
Answer:
(i) P(getting an odd number)
= 1 – P(an even number I all three tosses)
=1−12×12×12=78
(ii) Describe the events as follows.
A: getting a red ball
E1: Bagl. E2:Bagll.
P(E1) = P(E) = 1/2
P(A/E1) P (a red ball from Bag I) = 3/7
P(A/E2) P (a red ball from Bag II) = 5/11
P (a ball from Bag li, being given that it is red)
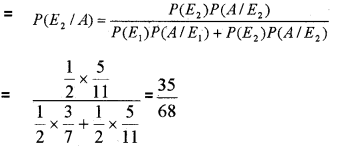
Question 8.
(i) If A and B are independent events, prove that ¯A and ¯B are independent
(ii) A box contains 30 defective bulbs and 30 non-defective bulbs. Two bulbs are drawn at random. The event A and B are defined as follows. A: first bulb is defective.’ B: ‘the second bulb is non-defective.’
Find probability of A and B. Prove that A and B are independent events. (May – 2012)
Answer:
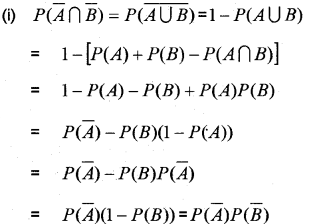
Hence ¯A and ¯B are independent.
(ii) Given, A:’first bulb is defective.’
B: the second bulb is non-defective.’
Let D: Defective bulb, ¯D: Non-defective bulb. Since the experiment is drawing 2btbs. The sample space will be S={DD,D¯D,¯DD,¯¯¯¯¯¯¯¯¯DD}
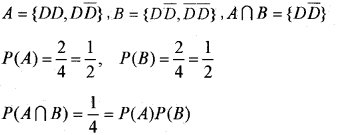
Hence independent events.
Question 9.
In a factory which manufactures bulbs, machine X,Y and Z manufactures respectively 25%, 35% and 40% of the bulbs. Of the outputs 1%, 2% and 3% are respectively defective bulbs. A bulb is drawn at random and found to be defective. What ¡s the probability that it is manufactured by machine Y? (May – 2012)
Answer:
Describe the events as follows.
D: Getting a defective bulb.
X: Machine X. Y: Machine Y. Z: Machine Z.
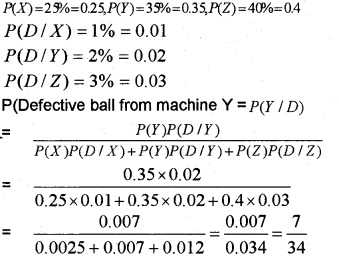
Question 10.
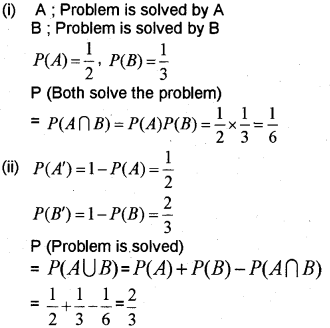
A and B try to solve a problem independently. Find probability that A solves the problem is and that of B solves the problem is. Find the probability that
(i) Both of them solve the problem.
(ii) The problem is solved. (March – 2013)
Answer:
Question 11.
If A and B are two independent events, then
(i) Prove that A and B’ are independent events.
(ii) Show that the probability of occurrence of at least one of A and B is I – P(A’)P (B’) (March – 2013)
Answer:
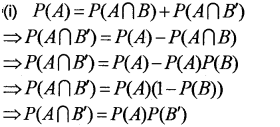
Hence A and B’ are independent events.
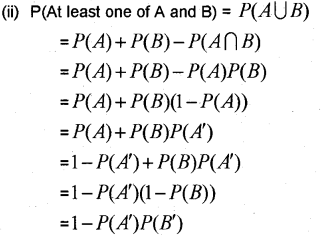
Question 12.
There are two identical boxes. Box I contains 5 red and 4 black balls, while Box II contains 3 red and 3 black balls. A person choose a box at random and takes out a ball.
(a) Find the probability that the ball drawn is red.
(b) If the ball drawn is black, what is the probability that it ¡s drawn from Box II. (May – 2014)
Answer:
(a) Let E1 be the event selecting box I and E2 be the event selecting box II.
P=(E1)=1/2,P=(E2)=1/2
Let A be the event selecting of a red ball then
P(A/E1)=12×59=518
P(A/E2)=12×36=312
P (taking a red ball) = 518+312=1936
b) Let B be the event selecting a black ball
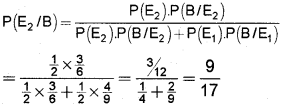
Question 13.
(a) If P(A) = O.8,P(B) = O.5,P(B/A) = 0.4 then find P(AUB)
(b) If a fair coin is tossed 10 times, then find the probability of getting exactly 6 heads. (May – 2015)
Answer:
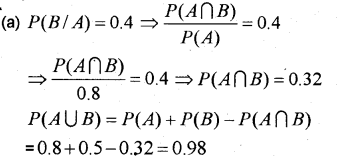
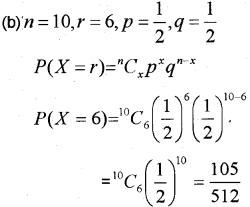
Question 14.
(a) If P(A) = 0.3, P(B) =0.4, then the value of where A and B are independent events
(i) 0.48
(ii) 0.51
(iii) 0.52
(iv) 0.58
(b) A card from a pack 0152 cards is lost. From the remaining cards of the packet, two cards are drawn and found to be diamonds. Find the probability of the lost card being a diamond. (March – 2016)
Answer:
(a) (iv) 0.58
(b) E1: lost card is a diamond.
E2: lost card is not a diamond.
A: Select 2 diamonds from the remaining cards.
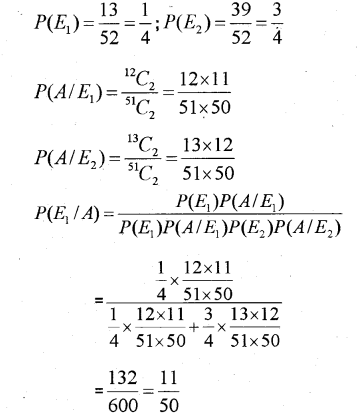
Question 15.
(a) A pair of dice is thrown 4 times. If getting a doublet is considered as a success.
(b) Find the probability of getting a doublet.
(c) Hence find the probability of getting two success. (March – 2016)
Answer:
(a) Probability of getting a doublet = 1/6
(b) Let X denotes the random variable of number of doublet in 4 throws of a die.
Clearly X has a Binomial Distribution with n = 4
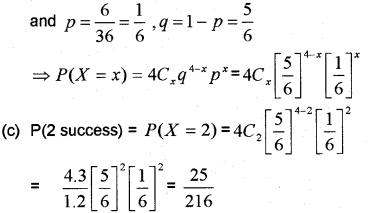
Plus Two Maths Probability 6 Marks Important Questions
Question 1.
(i) State and prove the theorem of total probability.
(ii) If a fair coin is tossed 10 times, what is the probability that the outcome is exactly 6 heads? (May – 2010)
Answer:
Theorem:
Let {E1,E2,….,En}be a partition of the sample space S, and suppose that each of the events E1, E2,….,En as nonzero probability of occurrence. Let A be any event associated with S, then
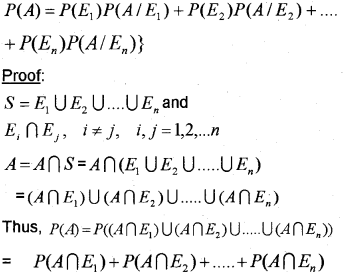
By multiplication rule of probability we have;
P(A)=P(E1)P(A/E1)+P(E2)P(A/E2)+…..+P(En)P(A/En)
(ii) Let X denotes the random variable of number of heads in an experiment of 10 trials. Clearly X has a Binomial Distribution with n = 10
Here n = 10, p = 1/2, q = 1 – p = 1/2
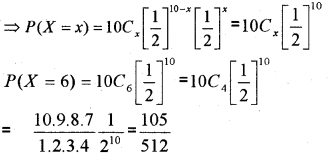
Question 2.
(i) 3 Coin are tossed and X be the number of heads turning up. Write probability distribution of X.
(ii) There are 5% defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item? (March – 2010)
Answer:
(1) S = { HHH , HHT ,HTH, THH, HTT , THT , HTH , TTT)
Let X denotes the random variable of getting a Head. Then X can take values 0,1 ,2,3.
P(X = 0) = P(no Heads)
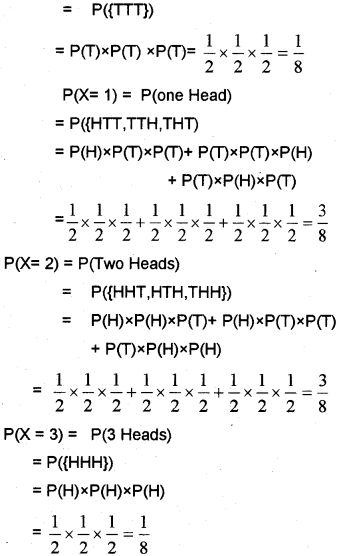
The required Probability Distribution is
| X | 0 | 1 | 2 | 3 |
| P(X) | 1/8 | 3/8 | 3/8 | 1/8 |
(ii) Let X denotes the random variable of number of defective items.
Clearly X has a Binomial Distribution with n = 10
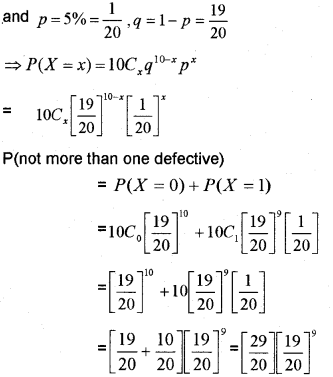
Question 3.
A class 15 students whose ages are 14, 17, 15, 14, 21, 17, 19, 20, 16, 18, 20, 17, 16, 19 and20 years. One student uis selected sucthat each has the same chance of being selected; the age X of the selected student is recorded.
(i) Write the probability Distribution of X.
(ii) Find E(X).
(iii) Find Var(X). (March – 2011)
Answer:
(i) Let X denotes the random vanable age of students.
Then X can take values 14, 15, 16, 17, 18, 19, 20, 21.
| X | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 |
| P(X) | 2/15 | 1/15 | 2/15 | 3/15 | 1/15 | 2/15 | 3/15 | 1/15 |
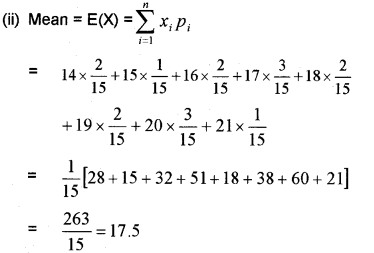
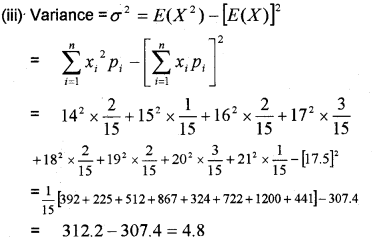
Question 4.
(i) An unbiased die is thrown twice. Let A be event ‘odd number on the first throw’ and B be the event ‘odd number on the second throw’. Check the independence of A and B.
(ii) If P(A) = O.8,P(B) = O.5,P(B/A) = 0.4,
Find
(a) P(A∩B)
(b) P(A/B)
(c) P(A∪B) (March – 2011)
Answer:

Question 5.
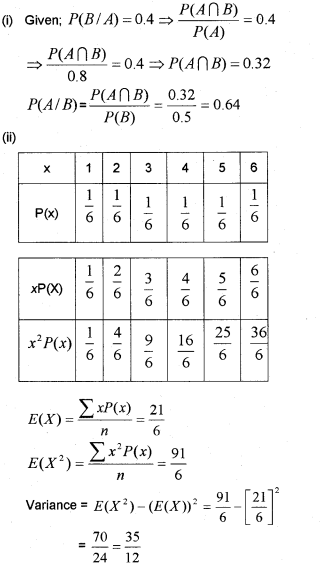
(i) A and B are two events such that P(A) = 0.8,P(B) = 0.5 and P(B/A) = 0.4, then find P(A/B)
(ii) Find the mean and variance of the number obtained on a throw of an unbiased die. (March – 2014)
Answer:
Question 6.
(i) Two events E and F are such that
P(E) = 0.6, P(F) = 0.2 and P(E∪F) O.68. Are E and F independent?
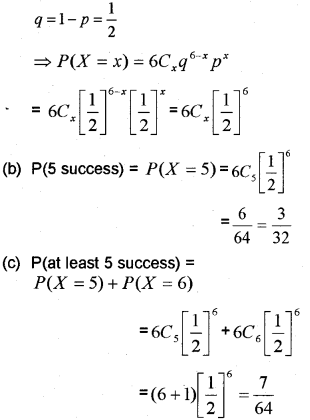
(ii) A die is thrown 6 times. If getting an odd number is a success, what is the probability of getting
(a) 5 successes?
(b) At least 5 successes?
(c) At most 5 successes? (March – 2014; May – 2016)
Answer:
(i) P(E∪F) = P(E) + P(F) – P(E∩F)
= 0.68 = 0.6 + 0.2 – P(E∩F)
= P(E∩F)=O.12
P(E) x P(F)= 0.2 x 0.6 = 0.12 = P(E∩F)
Hence E and F are independent events.
(ii) (a) Let X denotes the random variable of number of odd number in the throw of a die 6 times. Clearly X has a Binomial Distribution with n = 6 and p=36=12
Question 7.
The probability distribution of a random variable X is as given below.
| X | 1 | 2 | 3 | 4 | 5 |
| P(X) | 1/2 | 1/4 | 1/8 | 1/16 | P |
(a) Find the value of p.
(b) Find the mean of X.
(c) Find the variance of X. (March – 2015)
Answer:
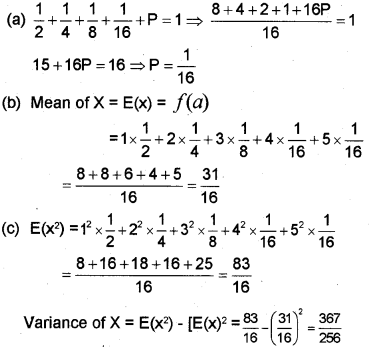
Question 8.
(a) A die is thrown thrice. Find the probability of getting an odd number at least once.
(b) Two cards are drawn successively with replacement from a pack of 52 cards. Find the probability distribution of the number of aces. (May – 2015)
Answer:
Let X denote the number of odds, X = 0, 1, 2, 3
The experiment follows Binomial distribution
n=3,p=12,q=12
The required probability = 1 – P(X = O)
=1−3C0(12)3(12)0=1−18=78
(b) P(Two cards are aces with replacement) = 4×452×52=1169
We know there are 4 aces in a deck of 52 cards.
Let X denote the number of aces. Then X can take values 0,1,2.
P(X0) = P(no ace and no ace)
= P(no ace) x P(no ace)
=4852×4852=144169
P(X=1)= P(ace and no ace or no ace and ace)
= P(ace and no ace ) + P(no ace and ace)
=452×4852+4852×452=24169
P(X =2) = P(ace & ace) = P(ace) x P(ace)
=452×452=1169
Therefore the distribution is as follows.
| X | 0 | 1 | 2 |
| P(X) | 144/169 | 24/169 | 1/169 |
We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 13 Probability help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 13 Probability, drop a comment below and we will get back to you at the earliest.
