Plus Two Maths Chapter Wise Previous Questions Chapter 11 Three Dimensional Geometry

Plus Two Maths Chapter Wise Previous Questions Chapter 11 Three Dimensional Geometry are part of Plus Two Maths Chapter Wise Previous Year Questions and Answers. Here we have given Plus Two Maths Chapter Wise Previous Chapter 11 Three Dimensional Geometry.

Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 11 Three Dimensional Geometry

Plus Two Maths Three Dimensional Geometry 3 Marks Important Questions

Question 1.
(i)Find the equation of the Plane with intercepts 2,3,4 on X,Y,Z axes respectively.^)
(ii) Find the distance of the point (- 1, – 2,3) from the Plane \(\bar{r}\)(2i – 3 j + 4k) = 4 (March – 2011)
Answer:
(i) The equation of the plane is \(\frac{x}{2}+\frac{y}{3}+\frac{z}{4}=1\)
(ii) The equation of the plane is 2x – 3y + 4z = 4
Hence the distance \(=\frac{2(-1)-3(-2)+4(3)-4}{\sqrt{4+9+16}}\)
\(=\frac{-2+6+12-4}{\sqrt{4+9+16}}=\frac{12}{\sqrt{29}}\)

Question 2.
Consider the points A(2,2, – 1), B(3,4,2), C(7,0,6)
(i) FindAB.
(ii) Find the Cartesian and vector equation the plane passing through these points. (March – 2011)
Answer:
(i) \(A B=\sqrt{1+4+9}=\sqrt{14}\)
(ii) Cartesian Equation of the plane is

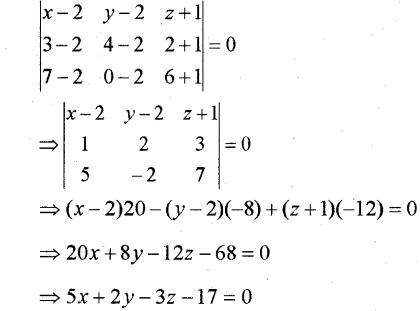
Vector equation is \(\bar{r}\)(5i + 2j – 3k) = 17

Question 3.
Consider the points A(3, – 4, – 5) and 5(2, – 3,1)
(i) Find the vector and Cartesian equation of the Line passing through the points A and B.
(ii) Find the point where the line crosses the XY Plane. (March – 2012)
Answer:
Let \(\bar{a}\) = 3i – 4j – 5k, b = 2i – 3j + k
(i) Vector Equation is \(\bar{r}\) = \(\bar{a}\) + λ(\(\bar{b}\) – \(\bar{a}\)) \(\bar{r}\) = 3i – 4j – 5k + λ( – i + j + 6k)
Cartesian Equation is \(\frac{x-3}{-1}=\frac{y+4}{1}=\frac{z+5}{6}\)

(ii) Let the point be (x, y, 0)
\(\begin{array}{l}
\Rightarrow \frac{x-3}{-1}=\frac{y+4}{1}=\frac{5}{6} \\
\Rightarrow x=\frac{13}{6}, y=\frac{-19}{6}
\end{array}\)
Then the point on the XY Plane is \(\left(\frac{13}{6}, \frac{-19}{6}, 0\right)\)

Question 4.
(i) Find the Cartesian equation of the plane passing through the point (1, 2, -3) perpendicular to the vector 2i – j + 2k.
(ii) Find the angle between the above \(\frac{x-1}{2}=\frac{y-3}{3}=\frac{z}{6}\) (March – 2012)
Answer:
(i) The equation of the Plane is
2(x – 1) – 1(y, -2) + 2(z+3) = 0
⇒ 2x – 2 – y + 2 + 2z + 6 = 0
⇒ 2x – y + 2z + 6 – 0

(ii) Angle between the line and the Plane is

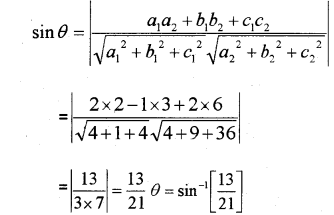
Question 5.
(i) Find the angle between the lines having direction ratios 1, 1, 2 and \(\sqrt{3}-1,-\sqrt{3}-1,4\)
(ii) If the lines \(\frac{x-1}{3}=\frac{y-1}{2 \lambda}=\frac{z-3}{2}\) and \(\frac{x-1}{3 \lambda}=\frac{y-1}{1}=\frac{z-6}{-5}\) are perpendicular, find the value of λ. (May – 2012)
Answer:

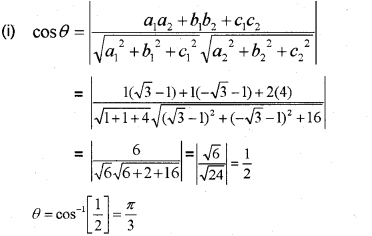
(ii) Since they are perpendicular
a₁a₂ + b₁b₂ + c₁c₂ = 0
⇒ 3 x 3λ + 2λ x 1 + 2x – 5 = 0
⇒ 9λ + 2λ – 10 = 0
⇒ 11λ + 10 ⇒ 2 = \(\frac{-10}{11}\)

Question 6.
(i) Find the equation of the Plane passing through the points (3, -1, 2), (5, 2, 4), ( -1, -1, 6)
(ii) Find the perpendicular distance from the point (6, 5, 9) to this plane. (March – 2013)
Answer:
(i) Cartesian Equation of the plane is

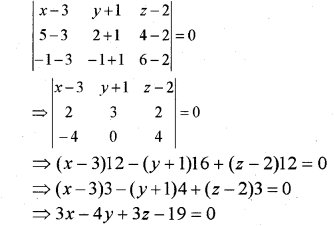
(ii) Perpendicular distance

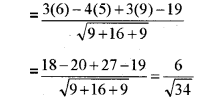
Question 7.
Consider the vector equation of the planes r.(2i + j+k) – 3 and r.(i – j – k) = 4
(i) Find the vector equation of any of the plane the intersection of the above two planes.
(ii) Find the vector equation of the planes through the intersection of the above two plane and the point (1, 2, -1). (May – 2013)
Answer:
(i) 2x + y + z – 3 + λ(x – y – z – 4) = 0
⇒ (2 + λ)x + (1 – λ)y + (1 – λ)z – 3 – 4λ = 0
Vector equation is
\(\bar{r}\).(2 + λ)i + (1 – λ)y + (1 – λ)k – (3 + 4)λ = 0

(ii) Since passing through (1,2, – 1) we have;
⇒ (2 + λ)1 + (1 – λ)2 + (1 – λ)( -1) – 3 – 4λ = 0
⇒ 2 + λ + 2 – 2λ – 1 + 1 – 3 – 4λ = 0
⇒ 0 – 4λ – 0
⇒ λ = 0

\(\bar{r}\).(2i + j + k) = 3 is required plane. Since the point (1, 2, -1) is a point on the first plane.

Question 8.
Consider the planes2x + y – 2z = 5 and 3x – 6y – 2z = 7
(a) Find their normal vectors.
(b) Find the angle between these two planes. (May – 2014)
Answer:
(a) Normal vectors are 2i + i – 2k; 3i – 6j – 2k
(b) Angle =

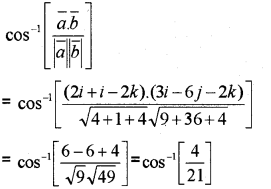
Question 9.
(a) If a1,b2, c1 and a2, b2, c2 are the direction ratios of two lines, then write the condition of its perpendicularity.
(b) Find the angle between the lines \(\frac{x+3}{3}=\frac{y-1}{5}=\frac{z+3}{4}\) and \(\frac{x+1}{1}=\frac{y-4}{1}=\frac{z-5}{2}\)
Answer:
(a) a₁a₂ + b₁b₂ + c₁c₂ = 0

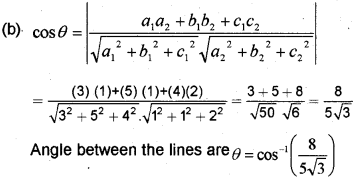
Question 10.
Find the shortest distance between the lines \(\bar{r}\) = i + j + λ(2i – j + k) and \(\bar{r}\) = 2i + j – k + µ(3i – 5 j + 2k) (March – 2016)
Answer:
From the given lines we have;

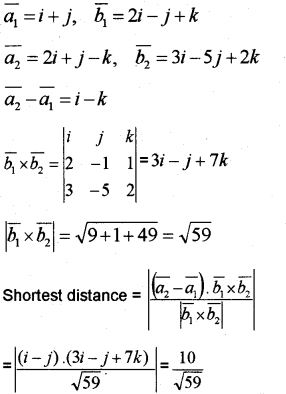
Question 11.
(i) The equation of the line which passes through the point(1,2,3) and parallel to the vector 3i + 2j – 2k is
(a) \(\bar{r}\) = 3i + 2j – 2k + λ(/ + 2j + 3k)
(b) \(\bar{r}\) = 2i – 5k + λ(3/ + 2j – 2k)
(c) \(\bar{r}\) = i + 2j + 3k + λ(-2i + 4j – 2k)
(d) \(\bar{r}\) = i + 2j + 3k + λ(3i + 2j – 2k)
(ii) Find the angle between the pair lines
\(\bar{r}\) = 2i – 5j + k + λ(3i + 2j + 6k) and \(\bar{r}\) = li – 6k + µ(i + 2j + 2k) (May – 2016)
Answer:
(i) (d) \(\bar{r}\) = i + 2j + 3k + λ(3i + 2j – 2k)
(ii) \(\bar{a}\) = 3i + 2j + 6k; \(\bar{b}\) = i + 2j + 2k
\(\cos \theta=\frac{\bar{a} \cdot \bar{b}}{|\bar{a} \| \bar{b}|}=\frac{19}{7 \times 3}=\frac{19}{21}\)

Plus Two Maths Three Dimensional Geometry 4 Marks Important Questions

Question 1.
Consider the lines \(\frac{x-3}{2}=\frac{y-1}{5}=\frac{z+3}{4}\) and \(\frac{x+5}{1}=\frac{y+2}{1}=\frac{z-3}{2}\)
(i) Find the angle between them.
(ii) Find the shortest distance between them. (March – 2011)
Answer:

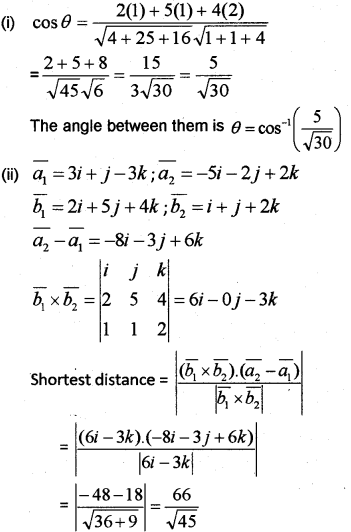
Question 2.
(i) Find the vector equation of the Plane Passing through the intersection of the Planes \(\bar{r}\).(i + j + k) = 6 and \(\bar{r}\)(2i + 3j + 4k) = – 5 and through the point (1, 1, 1).
(ii) Express the vector equation \(\bar{r}\).(5i + 3j + 4k) = 0 of a Plane in Cartesian form and hence find its perpendicular distance from the origin. (May – 2011)
Answer:
(i) The Cartesian equation of the given planes are x + y + z – 6 = 0 and 2x + 3 – y + 4z + 5 = 0
The family of such planes is x + y + z – 6 + λ(2x + 3y+ 4z + 5) = 0…..(1)
Since it passes through (1, 1, 1)

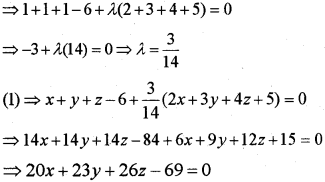
Vector Equation is \(\bar{r}\).(20i + 23j + 26k) = 69

(ii) Cartesian Equation is ⇒ 5x + 3y + 4z = 0 Perpendicular distance from origin is
\(=\left|\frac{5 \times 0+3 \times 0+4 \times 0}{\sqrt{25+9+16}}\right|=0\)

Question 3.
Given the Plane 5x – 2y + 4z – 9 = 0
(i) Find the foot of the perpendicular drawn from the origin to the Plane.
(ii) Write the vector and Cartesian equation of this perpendicular. (May – 2011)
Answer:
(i) The equation of the perpendicular line to the given plane 5x – 2y + 4z – 9 = 0 and passing through the origin is
\(\begin{array}{l}
\frac{x-0}{5}=\frac{y-0}{-2}=\frac{z-0}{4}=\lambda \\
\Rightarrow \frac{x}{5}=\frac{y}{-2}=\frac{z}{4}=\lambda
\end{array}\)
Hence any point on this line is (5λ, – 2λ, 4λ). Let this point be on the given plane then
⇒ 5(5λ) – 2( – 2λ) + 4(4λ) – 9 = 0
⇒ λ = 1/5
Then the foot of the perpendicular is
\(\left(5 \times \frac{1}{5},-2 \times \frac{1}{5}, 4 \times \frac{1}{5}\right) \Rightarrow\left(1,-\frac{2}{5}, \frac{4}{5}\right)\)
Since the line is perpendicular to the Plane and passes through the point \(\left(1,-\frac{2}{5}, \frac{4}{5}\right)\)
The Cartesian equation is \(\frac{x}{5}=\frac{y}{-2}=\frac{z}{4}\)
The Vector equation is \(\bar{r}\) = \(\bar{0}\) + λ(5i – 2j + 4k)

Question 4.
(i) The foot of the perpendicular from the origin to a Plane is P(4, – 2,5). Write \(\overline{O P}\)
(ii) Find the equation of the Plane in vector and Cartesian form. (May – 2012)
Answer:
(i) \(\overline{O P}\) = 4i – 2j + 5k
(ii) Then is perpendicular unit vector to the required plane is \(\frac{\overline{O P}}{\overline{O P} \mid}=\frac{4 i-2 j+5 k}{\sqrt{16+4+25}}=\frac{4 i-2 j+5 k}{\sqrt{45}}\)

The perpendicular distance from the origin is \(\sqrt{16+4+25}=\sqrt{45}\)
Vector equation of the Plane can be written as
\(\begin{array}{l}
\vec{r} \cdot \bar{m}=d \Rightarrow \bar{r} \cdot \frac{4 i-2 j+5 k}{\sqrt{45}}=\sqrt{45} \\
\Rightarrow \bar{r} .4 i-2 j+5 k=45
\end{array}\)
Cartesian from is 4x – 2y + 5z = 45

Question 5.
Consider the lines \(\frac{x-3}{3}=\frac{y-8}{-1}=\frac{z-3}{1}\) and \(\frac{x+3}{3}=\frac{y+7}{2}=\frac{z-6}{4}\)
(i) Express the equation to the lines into vector form.
(ii) Find the shortest distance between the lines. (March – 2013)
Answer:
(i) The vector equation;

Question 6.
Consider the Cartesian equation of a line
\(\frac{x-3}{2}=\frac{y+1}{3}=\frac{z-5}{-2}\)
(i) Find its vector equation.
(ii) Find the intersecting point with the plane 5x + 2y – 6z – 7 = 0 (May – 2013)
Answer:
(i) Vector equation of the line is
\(\bar{r}\) = (3i – 1j + 5k) + λ(2i + 3j – 2k)

(ii) Any point on the line is of the form

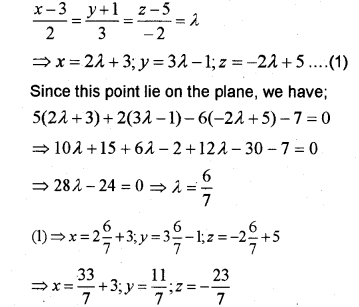
Question 7.
The foot of the perpendicular drawn from origin to a Plane is (4, -2, 5).
(i) How far is the plane from the origin?
(ii) Find a unit vector perpendicular to that Plane.
(iii) Obtain the equation of the Plane in general form. (March – 2014)
Answer:
(i) \(\overline{O P}\) = 4i – 2j + 5k
(ii) Then is perpendicular unit vector to the required plane is \(\frac{\overline{O P}}{\overline{O P} \mid}=\frac{4 i-2 j+5 k}{\sqrt{16+4+25}}=\frac{4 i-2 j+5 k}{\sqrt{45}}\)

The perpendicular distance from the origin is \(\sqrt{16+4+25}=\sqrt{45}\)
Vector equation of the Plane can be written as
\(\begin{array}{l}
\vec{r} \cdot \bar{m}=d \Rightarrow \bar{r} \cdot \frac{4 i-2 j+5 k}{\sqrt{45}}=\sqrt{45} \\
\Rightarrow \bar{r} .4 i-2 j+5 k=45
\end{array}\)
Cartesian from is 4x – 2y + 5z = 45

Question 8.
(a) Equation of the plane with intercepts 2, 3, 4 on the x, y z axis respectively is
(i) 2x + 3y + 4z = 1
(ii) 2x + 3y + 4z = 12
(iii) 6x + 4y + 3z = 1
(iv) 6x + 4y + 3z = 12

(b) Find the Cartesian equation of the plane passing through the points A(2, 5, -3), B(-2, -3, 5) and C(5, 3, -3). (March – 2016)
Answer:
(a) (iv) 6x + 4y + 3z = 12
(b) Equation of the plane is

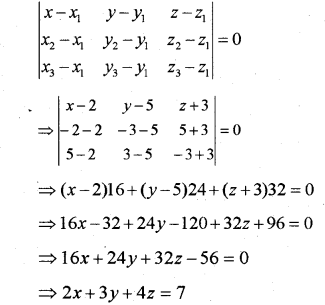
Question 9.
(i) The distance of the plane from the point(1, 1, 1)is
(a) 4 units
(b) \(\frac{1}{\sqrt{3}}\) units
(c) \(\frac{4}{\sqrt{3}}\) units
(d) \(\frac{1}{4 \sqrt{3}}\) units

(ii) Find the equation of the plane passing through (1, 0. -2) and perpendicular to each of the planes 2x + y – z = 2 and x – y – z = 3 (May – 2016)
Answer:
(i) (c) \(\frac{4}{\sqrt{3}}\) units
(ii) Equation of the plane passing through (1, 0, -2) is a(x – 1) + b(y – 0) + c(z + 2) = 0……………..(1)
Plane (1) is perpendicular to the given planes
2a + b – c = 0 ……………..(2)
a – b – c = 0 ……………..(3)
Solving (2) and (3) we get;

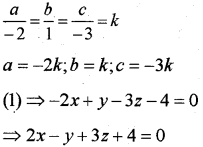
Question 10.
(i) The linesx – l = y = z is perpendicular to the line
\(\begin{array}{l}
\text { (a) } \frac{x-2}{1}=\frac{y-1}{2}=\frac{z}{-3} \\
\text { (b) } x-2=y-2=z \\
\text { (c) } \frac{x-2}{1}=\frac{y-1}{2}=\frac{z}{3} \\
\text { (d) } x=y=\frac{z}{2}
\end{array}\)

(ii) Find the shortest distance between the lines \(\bar{r}\) = i + 2j + 3k + λ(i + j + k) and \(\bar{r}\) – i + j + k + µ(i + j + k) (March – 2017)
Answer:

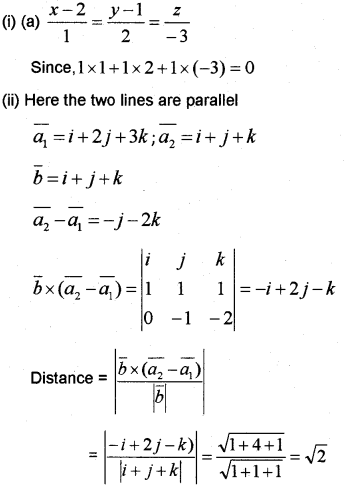
Plus Two Maths Three Dimensional Geometry 6 Marks Important Questions

Question 1.
(i) Find the shortest distance between the line \(\frac{x+1}{7}=\frac{y+1}{-6}=\frac{z+1}{1}\) and \(\frac{x-3}{1}=\frac{y-5}{-2}=\frac{z-7}{1}\)
(ii) Find the equation of the Plane passing through one point(-1, 3, 2) and ± r to the planes x + 2y + 3z = 5 and 3x + 3y + z = 0 (March – 2010)
Answer:

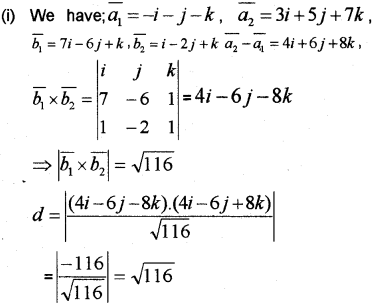
(ii) Let the equation of the plane be ax + by + cz + d = 0….(1)
Since (1) is perpendicular to 3x + 2y + 3z = 5, a + 2b + 3c = 0
Since (1) is perpendicular to 3x + 3y + z = 0
3a + 3b + c = 0
Using (2) and (3) we have;
\(\frac{a}{2-9}=\frac{-b}{1-9}=\frac{c}{3-6} \Rightarrow \frac{a}{-7}=\frac{b}{8}=\frac{c}{-3}\)
(1) = -7x + 8y – 3z + d = 0
Since (1) passes through (-1,3,2) we have;
⇒ -7 (-1) + 8(3) – 3(2) + d = 0
⇒ 7 + 24 – 6 + d = 0 = d = -25
Therefore the equation of the plane is
(1) ⇒ -7x + 8y – 3z – 25 = 0

Question 2.
(i) (a) A line makes equal angles with the coordinate axis. Find the direction cosines.
(b) Find the equation of the Plane Passing through (1, 1, -1),(2, 3, 5) an (1, 4, -5)
(ii) Find p and q, if the plariex+py+qz=0 is perpendicular to the plane 3x + 2y + z = 0 and the line \(\frac{x-3}{2}=\frac{y-2}{3}=\frac{z-1}{1}\) (March – 2010)
Answer:

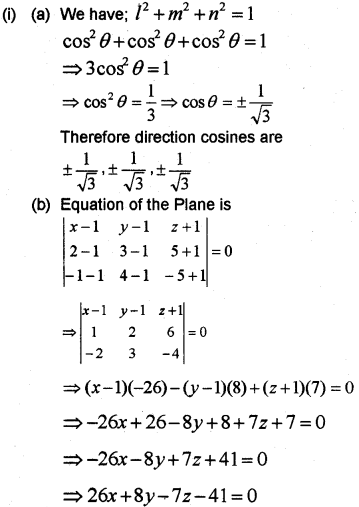
(ii) Since the Plane x + py + qz = 0 is perpendicular to the Line \(\frac{x-3}{2}=\frac{y-2}{3}=\frac{z-1}{1}\) we have their direction ratios proportional. Plane dr’s is 1, p, q and dr’s of Line is 2, 3, 1.
\(\Rightarrow \frac{1}{2}=\frac{p}{3}=\frac{q}{1} \Rightarrow p=\frac{3}{2}, q=\frac{1}{2}\)

Question 3.
Given the straight lines
\(\bar{r}[latex] = 3i + 2j – 4k + 2(i + 2j + 2k) and [latex]\bar{r}[latex] = 5i – 2k + µ(3i + 2j + 6k)
(i) Find the angle between the lines.
(ii) Obtain the unit vector perpendicular to both the lines.
(iii) From the equation of the line perpendicular to the given lines and passing through the point (1, 1, 1). (March – 2014)
Answer:

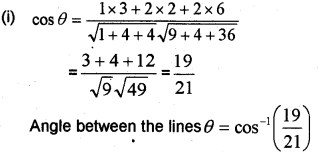
Question 4.
(a) Write the Cartesian equation of the straight line through the points (1,2,3) and along the vector 31 + j +2k
(b) Write a general point on this straight line.
(c) Find the point of intersection of this straight line with the plane 2x + 3y – z + 2 = 0
(d) Find the distance from (1,2,3) to the plane 2x + 3y – z + 2 = 0 (March – 2015)
Answer:
(a) Cartesian equation is [latex]\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\), \(\frac{x-1}{3}=\frac{y-2}{1}=\frac{z-3}{2}\)

(b) Let \(\frac{x-1}{3}=\frac{y-2}{1}=\frac{z-3}{2}=\lambda\)
x = 3λ + 1, y = λ + 2, z = 2λ + 3 are the general point of the line.

(c) Equation of the plane is 2x + 3y – z + 20….(1)
putting the values of x, y, z in (1) is
2(3λ + 1) – 3(λ + 2) – (2λ + 3) + 2 = 0
7λ + 7 = 0 ⇒ λ = -1
∴ Point of intersection is x = -3 + 1 = -2; y= -1 + 2 = 1, z= -2 + 3 = 1
ie; (-2, 1, 1)

(d) Distance = \(\frac{2(1)+3(2)-3+2}{\sqrt{4+9+1}}=\frac{7}{\sqrt{14}}\)

Question 5.
Consider a plane passing through the point (5, 2, -4) and perpendicular to the line
\(\bar{r}\) = (i + j) + λ(2i + 3j – k)
(a) Write the equation in cartesian form.
(b) Find its distance from the point (1,2, – 1).
(c) Find the angle made by it with line
\(\frac{x-1}{2}=\frac{y-2}{1}=\frac{z-3}{-2}\) (May – 2015)
Answer:
(a) The equation of a plane passing through the point (52,4) and perpendicular direction ratios 2, 3, -1 is

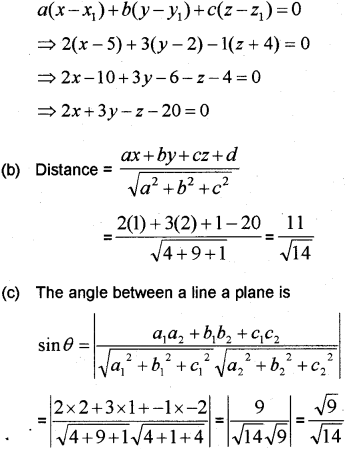
We hope the Plus Two Maths Chapter Wise Previous Questions Chapter 11 Three Dimensional Geometry help you. If you have any query regarding Kerala Plus Two Maths Chapter Wise Previous Questions Chapter 11 Three Dimensional Geometry, drop a comment below and we will get back to you at the earliest.