Kerala Plus One Physics Chapter Wise Previous Questions Chapter 8 Gravitation
Question 1.
Weight of a body depends directly on ‘g’. The value of ‘g’ depends on many factors like shape of earth, rotation of earth etc. (MARCH-2010)
a) Choose the correct alternative.
i) g increases/decreases with the increasing altitude.
ii) g is independent of mass of the earth/mass of the body.
iii) g is maximum/minimum at the poles.
iv) g increases/decreases with the increasing depth.
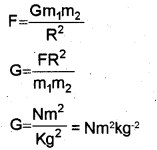
State Newton’s law of gravitation. Then write the unit of the gravitational constant (G).
Derive the expression for orbital and escape velocity.
Answer:
a) i) g decreases
ii) g is independent of mass of body
iii) g is maximum at poles
iv) g decreases with the increasing depth.
b)
c) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,
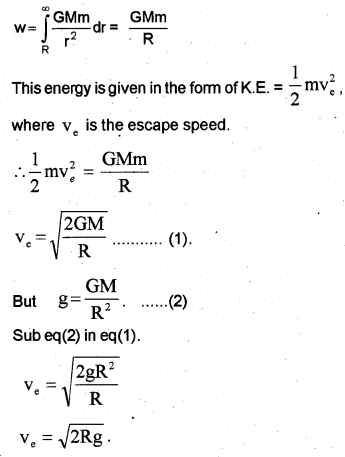
This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
Expression for orbital velocity
Consider a satellite of mass m revolving with orbital velocity ‘v’ around the earth at a height ‘ h’ from the surface of earth. Let M be the mass of earth and R be radius of earth.
The gravitational force of attraction between earth and satellite.
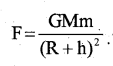
Centripetal force required forthe satellite
\(\mathrm{F}=\frac{\mathrm{mv}^{2}}{(\mathrm{R}+\mathrm{h})}\)
For stable rotation,
Centripetal force = Gravitational force
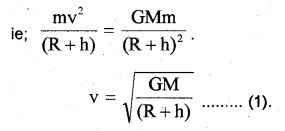
Question 2.
Weight of a body is the force of gravitational attraction experienced by it. It is equal to the product of mass of the body and acceleration due to gravity. (MAY-2010)
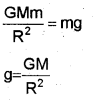
a) Obtain an expression for the acceleration due to gravity of a body in terms of the mass of the earth.
b) If you imagine the motion of a body from the centre of the earth to the surface of the moon, what changes will you observe in the weight of the body during that motion? (Neglect the effect of all other objects).
Answer:
a)
At the centre of earth g=0
Weight is zero.
Weight increases while moving from centre to surface. While moving above the surface weight de-creases. At neutral point weight becomes zero; while moving towards moon, g increases. Hence weight increases.
Question 3.
A person in an artificial satellite of the earth experiences weightlessness. The moon is a natural satellite of the earth.
a) Can a person on the moon experience weight? Why? (MARCH-2011)
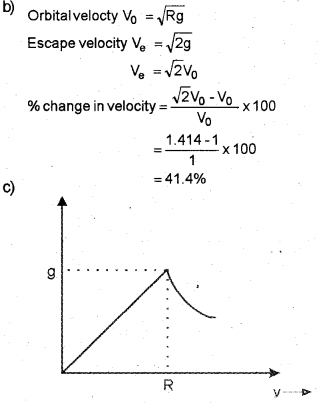
b) A satellite is revolving very close to the earth. What is the percentage increase in velocity needed to make it escape from the gravitational field of the earth?
c) Acceleration due to gravity ‘g’ depends on the distance T from the centre of the earth. Draw a graph showing the variation of ‘g’ with ‘r’.
Answer:
a) Yes. Moon attracts everybody towards its centre.
Question 4.
Imagine a point mass ‘m’ maintained at the centre of a shell of uniform density having mass ‘M’. If the radius of the shell is R, what will be the gravitational force exerted by the shell on the point mass? Explain. (MAY-2011)
Answer:
Zero. The point mass is attracted equally in all directions. Hence total force on point mass becomes zero.
Question 5.
The acceleration of a body due to the force exerted by earth on it is known as acceleration due to gravity. (MAY-2011)
a) Why does earth impart same acceleration on all bodies?
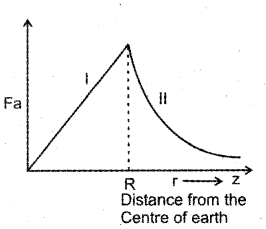
b) Show graphically the variation of gravitationl field strength with distance from the centre of the earth outwards.
Answer:
a) The acceleration due to gravity, g = \(\frac{\mathrm{GM}}{\mathrm{r}^{2}}\)
This equation shows that acceleration is indepent of mass of body that falls to the earth. Hence earth impart same acceleration on all bodies.
b)
Question 6.
a) The value of acceleration due to gravity is maximum at the (MARCH-2012)
i) Poles
ii) equator
iii) center of the earth
b) Find the height at which ‘g’ is reduced to g/2.
c) A rat and a horse are to be projected from earth into space. State whether the velocity is the same or different in projecting each animal. Justify.
Answer:
a) Poles
b)
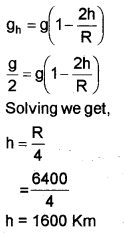
c) Velocity is same.
escape velocity is indepent of mass of body that projected in to space.
Question 7.
Nowadays we are familiar with satellites. (MAY-2012)
a) Name any two satellites.
b) Differentiate escape velocity from orbital velocity.
c) For an earth satellite show that, Escape Velocity = √2 x Orbital Velocity.
Answer:
a) INSAT, EDUSAT
b) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed.
Orbital velocity of a satellite is the velocity required for a satellite to revolve round the earth in a fixed orbit.
c) Orbital velocity is the velocity required for a satellite to revolve around earth in a fixed orbit. Escape velocity is the minimum velocity with which a body is projected so that it never returns to the earth.
Orbital velocity = √Rg ………(1)
Escape velocity = √2Rg ……….(2)
from eq.(1) and eq. (2)
Escape velocity = √2 orbital velocity
Question 8.
The escape speed for an object from the earth is 11.2 km/s. (MARCH-2013)
a) What is meant by escape speed?
b) Arrive at an expression for the escape speed from the earth.
c) Explain whether the escape speed depends on the mass of the object or not.
d) The earth contains an atmosphere while the moon does not give the reason.
Answer:
a) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
b) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
c) Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work was done in taking the body to infinity from surface of the earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
d) Escape velocity on moon is 2.3 km/s. The r.m.s. velocity of the gas molecules is greater than this value. Gas molecules easily escape from the surface of moon. Hence there is no atmosphere on moon.
Question 9.
The acceleration due to gravity (g) on the surface of the earth is 9.8 m/s². (MARCH-2013)
a) Define the acceleration due to gravity (g).
b) Derive an expression for the variation of g at a depth ‘d’ below the surface of earth.
c) At what height ‘h’ will the value of ‘g’ be half of that on the surface of the earth?
Answer:
a) Rate of change of velocity of body which falls towards earth is called acceleration due to gravity.
b)
If we assume the earth as a sphere of radius R with uniform density ρ,
mass of earth = volume x density

The above equation shows that, when depth increases g decreases.
c) Acceleration due to gravity g = \(\frac{\mathrm{GM}}{\mathrm{r}^{2}}\) ……..(1)
Let the value of g be \(\frac { g }{ 2 }\) at a height h above the ground
\(\frac { 1 }{ 2 }\) = \(\frac{\mathrm{GM}}{\mathrm{r}^{2}}\) ……(2)
(1)/(2) and solving we get
h = 0.414 R
Question 10.
For a particle to leave from the earth’s field, it should be projected with a minimum velocity. (MAY-2013)
a) Name the velocity.
b) Obtain the expression for the above velocity.
c) An elephant and an ant are to be projected from earth into space. Whether the velocities required for doing so are the same or different? Justify your answer.
d) Find the period of a simple pendulum, if this experiment is performed inside a satellite?
Answer:
a) Escape velocity
b) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
c) Escape velocity V = √2Rg
Escape velocity is independent of mass. Hence an elephant and an ant are to be projected with same escape velocity.
d) Period pendulum T = \(2 \pi \sqrt{1 / g}\)
Inside satellite g = 0
T = π \(2 \pi \sqrt{1 / 0}\) = ∞
Which means that pendulum does not oscillate inside a satellite.
Question 11.
The acceleration due to gravity (g) on the surface of the earth is 9.8 m/s². (MAY-2013)
a) Define acceleration due to gravity (g).
b) Derive an expression for the variation of g with height (h) above the surface of the earth.
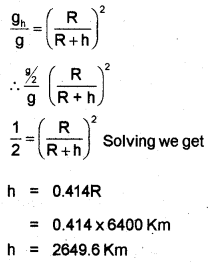
c) At what height ‘h’ the value of ‘g’ will be half of that on the surface of the earth?
Answer:
a) The uniform acceleration produced in a freely falling body due to gravitational pull of the earth is known as acceleration due to gravity.
b) The acceleration due to gravity on the surface of the earth,
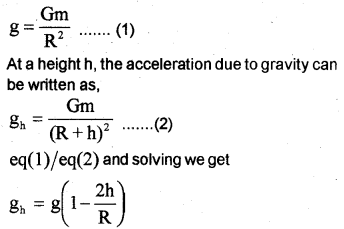
This equation shows that acceleration due to gravity decreases as height increases. The above equation is valid when h << R.
c) We know
Question 12.
Choose the correct alternative, (MARCH-2014)
a) Escape velocity is independent of the mass of the earth/the mass of the body.
Answer:
a) Escape velocity is independent of the mass of the body.
Question 13.
Earth satellites are objects which revolve around the earth. Consider a satellite at a height ‘h’ from the surface of the earth. (MARCH-2014)
a) Give an equation for its orbital velocity.
b) Obtain an equation for the period of the above satellite.
c) Distinguish between geostationary satellites and polar satellites.
OR
The value of acceleration due to gravity is maximum on the surface of the earth.
a) Write the relation between acceleration due to gravity and gravitational constant.
b) Obtain an equation for the variation of ‘g’ with height.
c) Draw a graph showing the variation of ‘g’ with depth and height from the surface of the earth. Assume that the density of the earth is constant.
Answer:
a) Orbital velocity,
\(V_{0}=\sqrt{\frac{G M}{(R+h)}}\)
b) Period of satellite is time taken by the satellite to revolve once around the planet in a fixed orbit.

c)

OR
a) \(\frac{\mathrm{GM}}{\mathrm{r}^{2}}\)
b) The acceleration due to gravity on the surface of earth,

This equation shows that acceleration due to gravity decreases as height increases. The above equation is valid when h << R.
c) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
Question 14.
The velocity of a satellite in its orbit is called orbital, velocity. (MAY-2014)
a) Find the relation between orbital velocity and escape velocity.
b) What is a geostationary satellite?
c) Moon has no atmosphere. Why?
Answer:
a)

b) Geostationary satellite is a satellite which appears to be stationary in the sky. It direction of rotation is from west to east.
Eg: INSAT
c) The escape velocity of moon is 2.3 km/s. The r.m.s. velocity of the gas molecule is greater than this value. Gas molecules easily escape from the surface of moon. Hence there is no atmosphere on moon.
Question 15.
Our earth has several artificial satellites. But the moon is the only natural satellite (MARCH-2015)
a) If acceleration due to gravity at the surface of earth is ‘g’, arrive at the expression for acceleration due to gravity at a depth ‘d’.
b) Distance to the moon from the earth is 3.84 x 108m and the time period of the moon’s revolution is 27.3 days. Obtain the mass of the earth.
(Gravitational constant G = 6.67 x 10-11 Nm2kg-2).
c) How do you explain weightlessness in an artificial satellite?
Answer:
a) The acceleration due to gravity on the surface of earth,

This equation shows that acceleration due to gravity decreases as height increases. The above equation is valid when h << R.
b)

c) Weight of rotating body in a satellite is used as centripetal force. Hence a body feel weightlessness in an artificial satellite.
Question 16.
For a body to leave from earth’s gravitational field, it should be projected with a minimum velocity. (MAY-2015)
a) i) Name the velocity.
ii) Derive an expression for this velocity.
b) The moon does not have an atmosphere around it? Give the reason.
Answer:
i) Escape velocity
ii) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
b) Escape velocity on moon is 2.3 km/s. The rms velocity of the gas molecules is greater than this value. Gas molecules easily escape from the surface of moon, hence there is no atmosphere on moon.
Question 17.
The acceleration due to gravity may vary with altitude and depth. (MARCH-2016)
a) Arrive at an expression for acceleration due to gravity at a height h. (h<<RE)
b) Why does satellite need no fuel to go around a planet in its fixed orbit.
c) Acceleration due to gravity is independent of (mass of earth/mass of body)
OR
For a particle to overcome the gravitational pull of the earth, it is projected with a minimum velocity.
a) Name the minimum velocity.
b) Obtain an expression for the above minimum velocity.
c) A ball bounces more on the surface of the moon than on the earth. Explain why.
Answer:
a) The acceleration due to gravity on the surface of earth,

This equation shows that acceleration due to gravity decreases as height increases. The above equation is valid when h << R.
b) According to law of conservation of angular momentum, angular momentum is constant if the torque on body is zero. For any planet, angular momentum is constant. No external torque is required by the planet by any means.
c) mass of body
OR
a) Escape velocity
b) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
c) The acceleration due to gravity (g) at the moon is 1/6th that on the earth.
Question 18.
a) State Kepler’s second law of planetary motion, (law of areas). (MAY-2016)
b) Derive an equation for the orbital velocity of a satellite.
c) Escape the velocity of a planet is 20km/s. Find the orbital velocity of the smallest possible orbit.
Answer:
a) The line joining any planet to the sun sweeps equal area in equal time interval, it aerial velocity is constant.
b) The minimum speed with which a body is projected so that it never returns to the earth is called escape speed or escape velocity.
Expression for escape speed
Force on a mass m at a distance rfrom the centre of earth = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
Work done in taking the body to infinity from surface of earth,

This escape velocity √2Rg is estimated to be 11.2 km/s on the earth.
c)
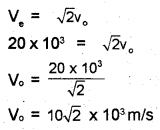
Question 19.
a) The escape speed from the surface of the earth is given by (MARCH-2017)

b) An artificial satellite circulating the earth is at a height 3400 km from the surface of the earth. If the radius of the earth is 6400 km and g = 9.8 m/s², calculate the orbital velocity of the satellite.
Answer:

