Plus One Physics Chapter Wise Previous Questions Chapter 10 Mechanical Properties of Fluids

Kerala Plus One Physics Chapter Wise Previous Questions Chapter 10 Mechanical Properties of Fluids

Question 1.
Washing with water doesn’t remove grease stains from clothes but addition of detergent removes the molecules of greasy substances. (MARCH-2010)
a) Which property of a liquid causes the above effect?
b) A single drop of liquid is split into 8 identical drops. What will be the excess pressure in each drop?
c) How can the coefficient of viscosity of a highly viscous liquid be determined by Stokes’ method?
Answer:
a) Surface tension
b) If R is the radius of drop;

From eq (1)and,(2) we get, the excess pressure inside the smaller drop increases two times,
c) If a body is moving through a fluid, its velocity is influenced by the viscosity of the medium. Consider a small sphere of radius‘a’falling undergravity in air: The viscocity of air opposes the motion of the sphere. This opposing force is called viscous force and can be written as.
F = 6 πaηv
This is called stokes, formula.
Where η is the coefficient of viscocity of the medium and v is the velocity of the body.

Question 2.
Water does not wet the feathers of ducks. A physical quantity called angle of contact determines whether a liquid will spread on the surface of a solid or it will form droplets on it.  (MAY-2010)
a) Define angle of contact.
b) A wire ring of internal radius 3 cm and external radius 3.2 cm is rested on the surface of a liquid and is then raised. An extra pulling force equivalent to the weight of 3.03 g is required before the film breaks than it is after. Calculate the surface tension of the liquid.
c) Derive an expression for the capillary height of a liquid in a narrow tube.
Answer:
a) Angle of contact is the angle between the solid surface and the tangent drawn to the liquid surface at the point of contact inside the liquid.

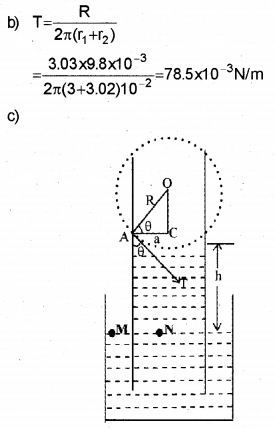
Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

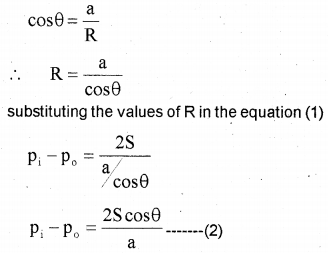
Considering two points M and N in the same horizontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg

Question 3.
——–
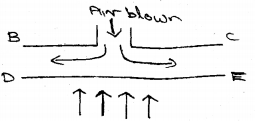
If air is blown through the space between two discs BC and DE shown in the figure, the lower disc DE instead of being blown off from BC, will move towards BC. (MAY-2010)
a) State the principle which is used to explain this phenomenon.
b) A garden hose having an internal diameter of 2 cm is connected to a lawn sprinkler that consists of an enclosure with 12 holes, each 0.2 cm in diameter. If the water in the hose has a speed 1.2 m/s at what speed does it leave the sprinkler holes ?
c) Show that the speed of liquid flowing out of a small hole in a tank filled with liquid will be √2gh where h is the height of liquid above the hole and g is the acceleration due to gravity.
Answer:
a) Bernoulli’s theorem
b)

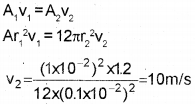
c)

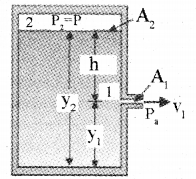
Consider a tank containing a liquid of density ρ with a small hole in its side. Let Y₁ be the height of the hole, and Y₂ be height of water in the tank. Applying Bemoullis equation at points (1) and (2) we get

(P₁ = P_a, the atmospheric pressure).
If the cross sectional area of the tank A₂ is much larger than that of the hole (ie; A₂>>A₁), we take

Question 4.
a) Hydraulic lift is a device used to lift heavy loads. State the principle behind the working of this device. (MARCH-2011)
b) The velocity of outflow of a liquid from an open tank is identical to that of a freely falling body.
i) Name this law and the principle behind the law.
ii) Derive this law based on this principle.
Answer:
a) Pascals law
Whenever external pressure is applied on any part of a fluid contained in a vessel, it is transmitted – undiminished and equally in all directions.
b) i) Torricellis law
Torricellis law may be stated as the velocity of afflux through a hole at a depth ‘h’ will be equal to the velocity gained by a freely falling body when it travels a distance‘h’.
ie; v = √2gh
ii)

Consider a tank containing a liquid of density ρ with a small hole in its side. Let Y₁ be the height of the hole, and Y₂ be height of water in the tank. Applying Bemoullis equation at points (1) and (2) we get

(P₁ = P_a, the atmospheric pressure).
If the cross sectional area of the tank A₂ is much larger than that of the hole (ie; A₂>>A₁), we take

Question 5.
a) A solid sphere falling through a viscous medium attains a constant velocity called terminal velocity after some time of its fall. What are the different forces acting on the sphere? Derive an expression for terminal velocity in terms of the coefficient of viscosity of the medium. (MARCH-2011)
b) Water kept in earthern pots gets cooled. Why?
Answer:
a) Viscous force, boyancy force and weight of the body
Expression for terminal velocity
Consider a sphere of radius ‘a’ density σ falling through a liquid of density a and viscocity η). The viscous force acting on the sphere can be written as
F = 6πaηv
Where v is the velocity of sphere This force is acting in upward direction. When the viscous force is equal to the weight of the body in the medium, the net force on the body is zero. It moves with a constant velocity called the terminal velocity.
The weight of a body in a medium,

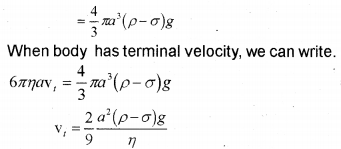
b) Due to evaporation.

Question 6.
The rise or fall of a liquid against gravitational force through fine tubes is known as capillarity. (MAY-2011)
a) Give an example for capillarity from practical life.
b) Derive an expression for the capillary rise through a capillary tube.
OR
When we blow between two light balls suspended near by they approach each other.
a) State the principle that can explain your observation.
b) Use this principle to arrive at Torricelli’s equation.
Answer:
a) 1) A blotting paper absorbs ink by capillary action.
2) It is by capillary action that water reaches every branch of a plant from the stem.
3) We use a towel to dry our body after bath.
4) Due to capillary action, water rises to the surface of the fields and gets evaporated. To avoid this loss of water, the fields are ploughed.
5) A sponge retains water,

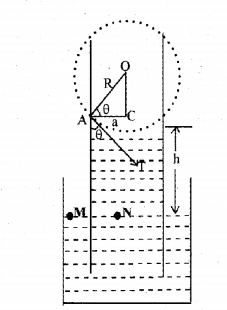
b) Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

Considering two points M and N in the same hori-zontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg

OR
a) Bernoullis principle
The total energy of an incompressible non-vis- cous liquid flowing from one place to another with-out friction is a constant.
b)

Consider a tank containing a liquid of density ρ with a small hole in its side. Let Y₁ be the height of the hole, and Y₂ be height of water in the tank. Applying Bemoullis equation at points (1) and (2) we get

(P₁ = P_a, the atmospheric pressure).
If the cross sectional area of the tank A₂ is much larger than that of the hole (ie; A₂>>A₁), we take

Question 7.
Say True or False : (MAY-2011)
A block of wood is floating in water its apparent weight is zero.
Answer:
True

Question 8.
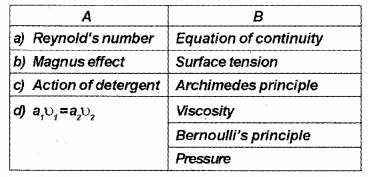
Match the following (MARCH-2012)

Answer:
1 a) Reynold’s number – viscosity
b) Magnus effect – Bernoullis principle
c) Action of detergent – Surface tension
d) \(a_{1} v_{1}=a_{2} v_{2}\) – Equation of continuity

Question 9.
Raindrops falling due to gravity do not acquire high velocity. (MARCH-2012)
a) Define the velocity of the raindrop when unbalanced force on it is zero.
b) Why do bubbles of air rise up through water?
c) The terminal velocity of a copper ball of radius 2.0 mm falling through a tank of oil at 20°C is 6.5 cm S^-1. Compute the viscosity of the oil at 20°C., Density of oil is 1.5 x 10³ kg m^-3, density of copper is 8.9 x 10³ kg m^-3.
d) Viscosity of gases ……… with temperature, Whereas viscosity of liquids ……… with temperature.
(Increases/decreases)
Answer:
a) Terminal velocity
b) Density of air bubble is less than water. Hence bubbles of air rise up through water.
c)

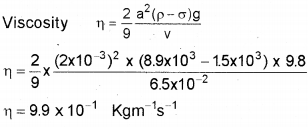
d) increases, decreases.

Question 10.
Surface tension changes with temperature. (MAY-2012)
a) Hot soup is tastier than cold one. Why.
b) What is the value of angle of contact for pure water?
c) Calculate the work done in breaking a water drop of radius 1 mm to 1000 droplets. Surface tension of water = 72 x 10^-3 N/m.
Answer:
a) Surface tension decreases with temperature. For hot soup surface tension is small. So easily spread on tasty buds.
b) For pure water with glass θ = 0°
c) R = 1 mm = 10^-3m
Since volume remains constant

Question 11.
a) Is pressure in a liquid, scalar or vector. (MAY-2012)
b) State the law associated with liquid pressure.
c) Briefly explain the working of hydraulic lift.
Answer:
a) Pressure is a scalar
b) Pascal’s law
The pressure in a fluid at rest is the same at all points if they are at the same height.
c)

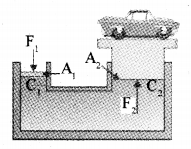
A hydraulic lift is used to lift heavy load. Consider a liquid enclosed in a vessel with two cylinders C₁ and C₂ attached as shown in the figure. The cylinders are provided with two pistons having areas A₁ and A₂ respectively.
If F₁ is the force exerted on the area A₁,


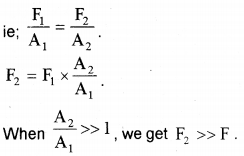
Using this method we can lift heavy load by applying small force.

Question 12.
A small water drop placed on a lotus leaf is spherical in shape. (MARCH-2013)
a) Define surface tension.
b) Why does the small water drop acquire a spherical shape?
Answer:
a) Liquids acquire a free surface when poured in a container. These surfaces possess some additional energy. This phenomenon is known as surface tension.
b) Due to surface tension, the liquid surface always tends to have the minimum surface area . For a given volume, a sphere has a minimum surface area. Hence, small drops and bubbles of a liquid assume spherical shape.

Question 13.
Three vessels of different shapes are filled with water to the same height ‘h’ and their bottom parts are connected to manometers measuring the pressure.The water levels in all the vessels remain the same. (MARCH-2013)

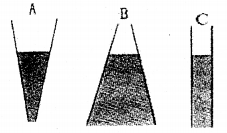
a) Identify the above phenomenon.
b) Predict the pressure level shown by the manometers.
c) Blood pressure in humans is greater at the feet
than at the brain. Explain why.
d) Pick the odd one out.
Dentist chair, hydraulic brake, hydraulic press, venturi meter.
Answer:
a) Capillary rise
b) Pressure readings of these three vessels are same, (hydrostatic paradox)
c) Blood inside the human heart produces pressure to human body.
Hence Blood pressure in human is greater at the feet than at the brain.
d) Venturi meter

Question 14.
The flow of an ideal fluid in a pipe of varying cross section is shown. (MARCH-2013)

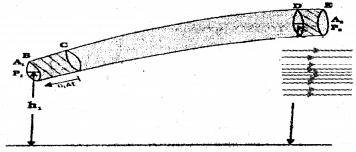
a) Differentiate between streamline flow and turbulent flow.
b) State and prove Bernoulli’s principle.
Answer:
a) Streamline flow
The flow of the fluid is said to be steady if at any given point, the velocity of each fluid particle remains constant in time.
The path taken by a fluid particle under a steady flow is a streamline.

Turbulent flow
As the speed of flow increase, at a certain speed the stream line flow stops and irregular flow starts. This irregular flow is called turbulent. The speed at which the turbulent flow starts is called critical speed.
b) As we move along a streamline the sum of the pressure (ρ), the kinetic energy per unit volume \(\frac { ρv² }{ 2 }\) and the potential energy per unit volume (ρgh) remains a constant
OR
The total energy of an incompressible non-viscous liquid flowing from one place to another with-out friction is a constant.
Mathematically Bernoulli’s theorem can be written as

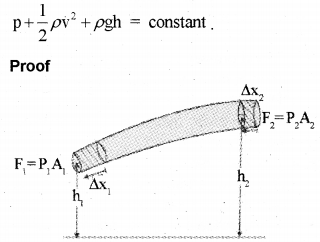
Consider an incompressible liquid flowing through a tube of non uniform cross section from region 1 to region 1. The corresponding values at the cross section and V₁ the speed of the flow at the region 1 . The corresponding values at region 2 are P₂, A₂ and V₁ respectively. Region 1 is at a height h₁ and region 2 is at a height h₂.
The workdone on the liquid in a time ∆t at the region 1 is given by
W₁ = P₁∆V₁
Similarly the workdone in a time ∆t at the region 2 is given by,
W₂=- P₂∆V₂ Net workdone
∆W₁ = P₁∆V – P₂∆V₂.
According the equation of continuity
∆V₁ = ∆V₂ = ∆V
∆W = P₁∆V – P₂∆V
∆W = (P₁ – P₂)∆V .
This work done changes the kinetic energy, pressure energy and potential energy of the fluid.
If ∆m is the mass of liquid passing through the pipe in a time ∆t. the change in Kinetic energy is given by
\(\Delta \mathrm{k} . E=\frac{1}{2} \Delta \mathrm{mV}_{2}^{2}-\frac{1}{2} \Delta \mathrm{mV}_{1}^{2}\) ……(2)
Change in gravitational potential energy is given by
∆P.E= ∆mgh₂ – ∆mgh₁ ………..(3)
According to work-energy theorem work done is equal to the change in kinetic energy plus the change in potential energy.
ie; ∆w =∆kE+∆PE ………(4)
Substituting eq. 1,2 and 3 in eq. 4, we get

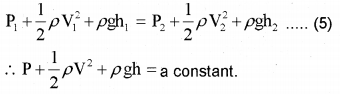
Question 15.
Pick the odd one out from the following (MAY-2013)
a) Atomiser
b) Hydraulic Lift
c) Venturimeter
d) Aerofoil
Answer:
Hydraulic lift
It is based on Pascals law. All others are related to Bernoulis principle.

Question 16.

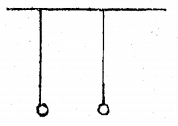
When a thin tube is dipped in water, water rises in the tube through a height ‘h’ (MAY-2013)
a) Name the phenomenon.
b) Arrive at an expression for the height of the water rise (h) in the tube.
c) Will water overflow, if the tube is cut at level A? Justify your answer.
Answer:
a) Capillary rise
b)
b) Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

Considering two points M and N in the same hori-zontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg


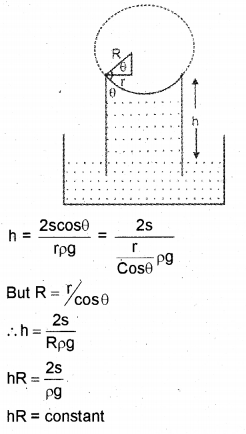
Where R is the radius of meniscus.
If the length of capillary tube is less than h, the liquid rises to a length l¹ and adjusts the radius
of its meniscus to R¹ such that l¹R¹ = hR
Thus it is clear that if the capillary tube is of ini sufficient length, the liquid rises upto the upper
end of the tube and adjusts the radius of curvature of meniscus. The liquid cannot emerge in the form of fountain.

Question 17.
Fill in the blanks: (MARCH-2014)
Venturimeter: Bernoulli’s theorem
Hydraulic lift: ………….
Answer:
Pascals Law

Question 18.
The viscous force exists when there is a relative motion between the layers of the fluid. (MARCH-2014)
a) State true or false: “The viscosity of gases decreases with an increase in temperature.”
b) Obtain an impression for the termilan velocity at
tained by an object falling through a viscous medium.
c) The speed time graph of a falling sky diver is shown below. During the fall he opens his parachute.

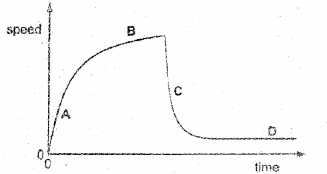
d) Which part of the graph shows the sky diver falling with terminal velocity?
OR
There is always an excess of pressure inside drops and bubbles.
a) State true or false: “A drop of liquid under no external forces is always spherical in shape.”
b) Obtain an expression for excess of pressure in-side a drop of radius r and surface tension S.
c) Two soap bubbles A and B are blown at the end of a tube, as shown below.

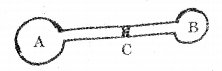
Choose the correct answre: When the block C is removed,………..
i) the size of A increases and that of B decreases.
ii) the size of B increases and that of A decreases
iii) no change occurs in their sizes
iv) their sizes become equal
Answer:
a) False
b) Viscous force, boyancy force and weight of the body
Expression for terminal velocity
Consider a sphere of radius ‘a’ density σ falling through a liquid of density a and viscocity η). The viscous force acting on the sphere can be written as
F = 6πaηv
Where v is the velocity of sphere This force is acting in upward direction. When the viscous force is equal to the weight of the body in the medium, the net force on the body is zero. It moves with a constant velocity called the terminal velocity.
The weight of a body in a medium,

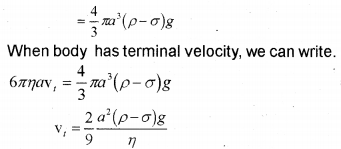
c) D
OR
a) True
b) The molecules near the surface of a drop experience a resultant pull inwards due to surface tension. Because of this inward pull, the pressure inside is greater than the outside. Due to the forces of surface tension, the drop tends to contract. But due to excess inside pressure, the drop tends to expand. When the drop is in equilibrium, these two forces will be equal and opposite.

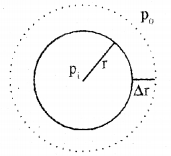
Consider a drop of liquid of radius r .Let p₁ and p₀ be the values of pressure inside and outside the drop. Let the radius of liquid of drop increases by a small amount ∆r under the pressure difference. The outward force acting on the surface of the drop,

c) i)

Question 19.

When air is blown in between the two balls, will they attract or repel?  (MAY-2013)
State the principle that explains your observation.
Using this principle, derive Torricelli’s equation [Speed of Efflux]
Answer:
a) ∆ttract each other
b) Bernoulli’s principle
(OR)
The total energy of an incompressible non-viscous liquid flowing from one place to another without friction is a constant
Speed of Afflux : Torricellis law
Torricellis law may be stated as the velocity of afflux through a hole at a depth ‘h’ will be equal to the velocity gained by a freely falling body when it travels a distance ‘h’. ie; v = √2gh
Proof:

Consider a tank containing a liquid of density ρ with a small hole in its side. Let Y₁ be the height of the hole, and Y₂ be height of water in the tank. Applying Bemoullis equation at points (1) and (2) we get

(P₁ = P_a, the atmospheric pressure).
If the cross sectional area of the tank A₂ is much larger than that of the hole (ie; A₂>>A₁), we take

Question 20.
A liquid surface behaves like a stretched elastic membrane. (MAY-2014)
a) Name the liquid property for the above behavior.
b) Define angle of contact. What is its value for pure water with glass?
c) Derive an expression for the rise of liquid in a capillary tube of radius r, having density p and surface tensions s.
Answer:
a) Surface tension
b) Angle of contact is the angle between the solid surface and tangent drawn to the liquid surface at point of contact inside the liquid.
For pure water angle of contact is zero.
c)
Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

Considering two points M and N in the same hori-zontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg

Question 21.
The pressure of the atmosphere at any point is the weight of the air column of a unit cross-sectional area. Its unit is bar. (MARCH-2015)
a) Identify the given diagram and write its uses.

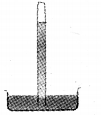
b) State Pascal’s law for transmission of fluid pressure and explain the principles of working of a hydraulic lift.
c) The above arrangement is placed in an elevator which is accelerating upwards. What happens to the possible height of the liquid column in the tube? Justify.
OR
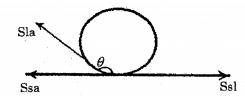
The antiseptics used for cuts and wounds in human flesh have low surface tension. Due to low surface tension they spread overthe wounds easily,
a) In the following figure, the angle of contact θ is ………..

b) Surface tension causes capillarity. Define capillarity and arrive at the expression for capillary rise in terms of surface tension.
c) In a capillary tube, water rises to a height. If the capillary tube is inclined at an angle 60°C with the vertical, what will be the length of the water column in the tube?

Answer:
a) Barometer
b) Pascal’s Law
Whenever external pressure is applied on any part of a fluid contained in a vessel, it is transmitted undiminished and equally in all directions.
Hydraulic lift: 

A hydraulic lift is used to lift heavy load. Consider a liquid enclosed in a vessel with two cylinders C₁ and C₂ attached as shown in the figure. The cylinders are provided with two pistons having areas A₁ and A₂ respectively.
If F₁ is the force exerted on the area A₁,


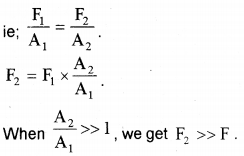
Using this method we can lift heavy load by applying small force.
c) Liquid level decreases. When this arrangement moves with an acceleration in upward direction weight of liquid column increases. Hence pressure inside the liquid column increases.
OR
a) Obtuse
b) Capillary rise
A tube of very fine core is called a capillary tube. When a capillary tube is dipped in a liquid, the liquid immediately rises in the tube. This phe-nomena is called capillarity or capillary rise.
In the case of mercury, capillary depression is observed.
Expression for capillary rise :

b) Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

Considering two points M and N in the same hori-zontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg

c) Let h be the initial height of liquid, h’is the height of liquid column.
From figure

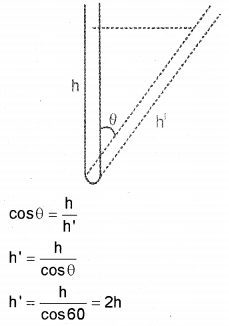
Question 22.
State and prove Bernoulli’s theorem. (MAY-2015)

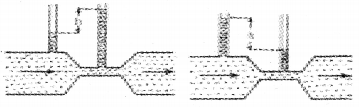
A steady flow of non viscous iiquids are shown fin figures 1 and 2. Which one of the figures is INCORRECT? Why?
a) What is the SI unit of pressure? Derive a mathematical expression for excess pressure inside a liquid drop.
b) What will happen to two soap bubbles of different radii, which are in contact with each other? Why?
Answer:
a) As we move along a streamline the sum of the pressure (ρ), the kinetic energy per unit volume \(\frac { ρv² }{ 2 }\) and the potential energy per unit volume (ρgh) remains a constant
OR
The total energy of an incompressible non-viscous liquid flowing from one place to another with-out friction is a constant.
Mathematically Bernoulli’s theorem can be written as

Consider an incompressible liquid flowing through a tube of non uniform cross section from region 1 to region 1. The corresponding values at the cross section and V₁ the speed of the flow at the region 1 . The corresponding values at region 2 are P₂, A₂ and V₁ respectively. Region 1 is at a height h₁ and region 2 is at a height h₂.
The workdone on the liquid in a time ∆t at the region 1 is given by
W₁ = P₁∆V₁
Similarly the workdone in a time ∆t at the region 2 is given by,
W₂=- P₂∆V₂ Net workdone
∆W₁ = P₁∆V – P₂∆V₂.
According the equation of continuity
∆V₁ = ∆V₂ = ∆V
∆W = P₁∆V – P₂∆V
∆W = (P₁ – P₂)∆V .
This work done changes the kinetic energy, pressure energy and potential energy of the fluid.
If ∆m is the mass of liquid passing through the pipe in a time ∆t. the change in Kinetic energy is given by
\(\Delta \mathrm{k} . E=\frac{1}{2} \Delta \mathrm{mV}_{2}^{2}-\frac{1}{2} \Delta \mathrm{mV}_{1}^{2}\) ……(2)
Change in gravitational potential energy is given by
∆P.E= ∆mgh₂ – ∆mgh₁ ………..(3)
According to work-energy theorem work done is equal to the change in kinetic energy plus the change in potential energy.
ie; ∆w =∆kE + ∆PE ………(4)
Substituting eq. 1,2 and 3 in eq. 4, we get

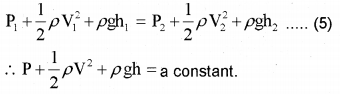
b) Figure 1 is incorrect. When area decreases velocity of liquid flow increase. Then pressure decreases. The pressure of liquid at narrow part of tube is less than the wider part. Therefore the liquid level above narrow part tube will be lower than that above wider part.
OR
a) The SI unit of pressure is Pascal (N/m²)
b) The molecules near the surface of a drop experience a resultant pull inwards due to surface tension. Because of this inward pull, the pressure inside is greater than the outside. Due to the forces of surface tension, the drop tends to contract. But due to excess inside pressure, the drop tends to expand. When the drop is in equilibrium, these two forces will be equal and opposite.

Consider a drop of liquid of radius r .Let p₁ and p₀ be the values of pressure inside and outside the drop. Let the radius of liquid of drop increases by a small amount ∆r under the pressure difference. The outward force acting on the surface of the drop,

c) i)

Question 23.
In case of fluids law of conservation of energy can be explained with Bernoulli’s principle. (MARCH-2016)
State and prove Bernoulli’s principle.
While travelling in aeroplane, it is advisable to remove inkform fountain pen. Why?
OR
Viscosity is the frictional force in fluids.
When a small metal ball is falling through a viscus medium, what are the various forces acting on it? Using this arrive at an expression for terminal velocity.
Raindrops falling under gravity do not acquire very high velocity. Why?
Answer:
As we move along a streamline the sum of the pressure (ρ), the kinetic energy per unit volume \(\frac { ρv² }{ 2 }\) and the potential energy per unit volume (ρgh) remains a constant
OR
The total energy of an incompressible non-viscous liquid flowing from one place to another with-out friction is a constant.
Mathematically Bernoulli’s theorem can be written as

Consider an incompressible liquid flowing through a tube of non uniform cross section from region 1 to region 1. The corresponding values at the cross section and V₁ the speed of the flow at the region 1 . The corresponding values at region 2 are P₂, A₂ and V₁ respectively. Region 1 is at a height h₁ and region 2 is at a height h₂.
The workdone on the liquid in a time ∆t at the region 1 is given by
W₁ = P₁∆V₁
Similarly the workdone in a time ∆t at the region 2 is given by,
W₂=- P₂∆V₂ Net workdone
∆W₁ = P₁∆V – P₂∆V₂.
According the equation of continuity
∆V₁ = ∆V₂ = ∆V
∆W = P₁∆V – P₂∆V
∆W = (P₁ – P₂)∆V .
This work done changes the kinetic energy, pressure energy and potential energy of the fluid.
If ∆m is the mass of liquid passing through the pipe in a time ∆t. the change in Kinetic energy is given by
\(\Delta \mathrm{k} . E=\frac{1}{2} \Delta \mathrm{mV}_{2}^{2}-\frac{1}{2} \Delta \mathrm{mV}_{1}^{2}\) ……(2)
Change in gravitational potential energy is given by
∆P.E= ∆mgh₂ – ∆mgh₁ ………..(3)
According to work-energy theorem work done is equal to the change in kinetic energy plus the change in potential energy.
ie; ∆w =∆kE+∆PE ………(4)
Substituting eq. 1,2 and 3 in eq. 4, we get

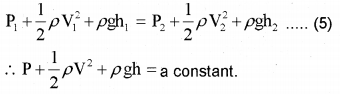
At higher altitude pressure is low. So ink may leak out from pen.
OR
a) Weight, viscous force
b) As rain drop falls under gravity through the air, it experience viscous force. ∆t a particular time, the net force on the raindrop becomes zero and then it moves with constant velocity (terminal velocity)
Viscous force, boyancy force and weight of the body
Expression for terminal velocity
Consider a sphere of radius ‘a’ density σ falling through a liquid of density a and viscocity η). The viscous force acting on the sphere can be written as
F = 6πaηv
Where v is the velocity of sphere This force is acting in upward direction. When the viscous force is equal to the weight of the body in the medium, the net force on the body is zero. It moves with a constant velocity called the terminal velocity.
The weight of a body in a medium,

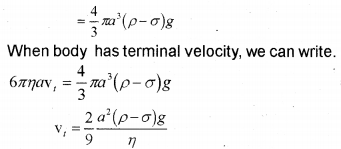
Question 24.
Consider the flow of a liquid through a pipe of varying cross section. (MAY-2016)
a) Write the equation of continuity of flow.
b) Draw a figure and derive Bernoulli’s equation.
c) A tank of 5m height is filled with water. Calculate the velocity of efflux through a hole, 3m below the surface of water.
OR
Surface tension is a property of liquids and it causes capillary rise in small tubes.
a) What do you mean by surface tension?
b) Draw a figure and derive an equation for capillary rise in a tube of radius ‘r’.
c) Excess pressure inside a liquid drop is 60N/m².
What will be the excess pressure inside a liquid bubble of the same radius formed by the same liquid?
Answer:
a) AV = constant or A₁V₁ =A₂V₂
b) As we move along a streamline the sum of the pressure (ρ), the kinetic energy per unit volume \(\frac { ρv² }{ 2 }\) and the potential energy per unit volume (ρgh) remains a constant
OR
The total energy of an incompressible non-viscous liquid flowing from one place to another with-out friction is a constant.
Mathematically Bernoulli’s theorem can be written as

Consider an incompressible liquid flowing through a tube of nonuniform cross-section from region 1 to region 1. The corresponding values at the cross-section and V₁ the speed of the flow at region 1 . The corresponding values at region 2 are P₂, A₂ and V₁ respectively. Region 1 is at a height h₁ and region 2 is at a height h₂.
The work done on the liquid in a time ∆t at the region 1 is given by
W₁ = P₁∆V₁
Similarly the workdone in a time ∆t at the region 2 is given by,
W₂=- P₂∆V₂ Net workdone
∆W₁ = P₁∆V – P₂∆V₂.
According the equation of continuity
∆V₁ = ∆V₂ = ∆V
∆W = P₁∆V – P₂∆V
∆W = (P₁ – P₂)∆V .
This work done changes the kinetic energy, pressure energy and potential energy of the fluid.
If ∆m is the mass of liquid passing through the pipe in a time ∆t. the change in Kinetic energy is given by
\(\Delta \mathrm{k} . E=\frac{1}{2} \Delta \mathrm{mV}_{2}^{2}-\frac{1}{2} \Delta \mathrm{mV}_{1}^{2}\) ……(2)
Change in gravitational potential energy is given by
∆P.E= ∆mgh₂ – ∆mgh₁ ………..(3)
According to work-energy theorem work done is equal to the change in kinetic energy plus the change in potential energy.
ie; ∆w =∆kE+∆PE ………(4)
Substituting eq. 1,2 and 3 in eq. 4, we get

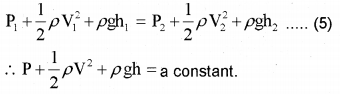
c) h = 3m
V = √2gh = \(\sqrt{2 \times 9.8 \times 3}\)
OR
a) Surface tension is surface energy per unit area. The liquid at free surface has some additional energy. This phenomenon is called surface tension.

b) Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle θ with the wall of the tube.
In the right angled triangle ACO

Considering two points M and N in the same horizontal level of a liquid at rest, pressure at N = pressure at M But pressure at M = ρ₁, the pressure over the concave meniscus and pressure at N = p₀ +hρg

c) A liquid bubble has air inside and outside it. Hence it has two surfaces.
Excess pressure inside liquid bubble =2x60N/m²
=120N/M²

Question 25.
Draw the schematic diagram of a hydraulic lift. Give its working principle. (MARCH-2017)
Answer:
c)

A hydraulic lift is used to lift heavy load. Consider a liquid enclosed in a vessel with two cylinders C₁ and C₂ attached as shown in the figure. The cylinders are provided with two pistons having areas A₁ and A₂ respectively.
If F₁ is the force exerted on the area A₁,


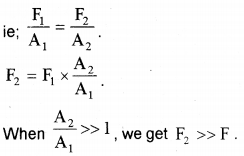
Using this method we can lift heavy load by applying small force.

Question 26.
A region of streamline flow of an incompressible fluid is shown in the figure. (MARCH-2017)

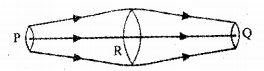
a) By considering mass conservation in the fluid flow, arrive at the ‘equation of continuity’.
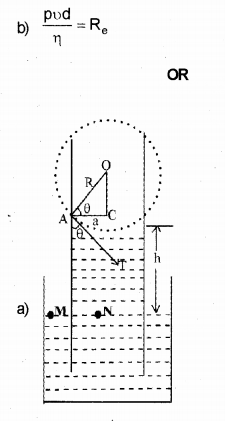
b) The onset of turbulence in a fluid is determined by ‘Reynold number’, given as….

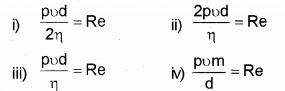
OR
Schematic diagram of capillary rise in a narrow tube is shown in the figure.

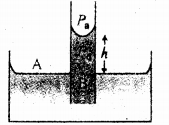
a) Arrive at an expression for capillary height ‘h’ in terms of the surface tension of the liquid.
b) On the surface of the moon, the liquid in a capillary tube will rise to the ………
i) same height as on earth.
ii) less height as on earth.
iii) more height than that on earth.
iv) infinite height.
a) Flow rate of an incompressible fluid is constant
ie Av = cont.
Where A is the area of cross section of flow and v is the velocity of flow. ‘Av’ is also called volume flux.
Proof
Consider a flow of incompressible liquid. Let P, R and Q be three points of a flow. Consider three planes perpendicular to direction of flow at P, R and Q.

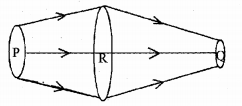
Let A_P, A_R and A_Q be the area of cross sections and V_P, V_R and V_Q be the corresponding speeds at P, R, Q respectively.
The mass of liquid passing through area A_P in a time ∆t, M_P = A_P V_P ρ_P ∆t
Similarly, M_R = A_R V_R ρ_R∆t
M_Q=A_QV_Q ρ_Q ∆t
But we know
ρ_P = ρ_R= ρ_Q= ρ
Hence we get
M_P = A_P V_P ρ ∆t
M_R=A_RV_Rρ∆t
M_Q = A_Q V_Q ρ ∆t
the mass of liquid flowing out = the mass flowing in ie;
M_P = M_R = M_Q
A_PV_P=A_RV_R=A_QV_Q
ie. AV = Constant

Consider a capillary tube of radius ‘a’ dipped in a liquid of density p and surface tension S. If the liquid has a concave meniscus it will rise in the capillary tube. Let h be the rise of the liquid in the tube. Let p, be the pressure on the concave side of the meniscus and p0, that on the other side .
The excess pressure on the concave side of the meniscus can be written as

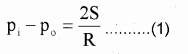
Where R is the radius of the concave meniscus. The tangent to the meniscus at the point A makes an angle 9 with the wall of the tube.
In the right angled triangle ACO

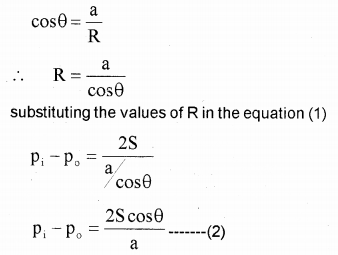
Considering two points M and N in the same horizontal level of a liquid at rest, pressure at N = pressure at M
But pressure at M = p₁ the pressure over the concave meniscus and pressure at N = p₀ +hρg

iii) more height than that on earth.

Plus One Physics Chapter Wise Previous Questions