Kerala Plus One Maths Previous Year Question Paper March 2018 with Answers
| Board | SCERT |
| Class | Plus One |
| Subject | Maths |
| Category | Plus One Previous Year Question Papers |
Time Allowed: 2 1/2 hours
Cool off time: 15 Minutes
Maximum Marks: 80
General Instructions to Candidates :
- There is a ‘cool off time’ of 15 minutes in addition to the writing time.
- Use the ‘cool off time’ to get familiar with the questions and to plan your answers.
- Read instructions carefully.
- Read questions carefully before you answering.
- Calculations, figures and graphs should be shown in the answer sheet itself.
- Malayalam version of the questions is also provided.
- Give equations wherever necessary.
- Electronic devices except non-programmable calculators are not allowed in the Examination Hall.
Answer any six questions from 1 to 7. Each carries 3 scores. (6 × 1 = 6)
Question 1.
Find the sum to n terms of the sequence 4 + 44 + 444 + ………
Answer:
Sn = 4 + 44 + 444 +
= 4(1 + 11 + 111 + ………)
= 49(9 + 99 + 999 + ………)
= 49(10 – 1 + 100 – 1 + 1000 – 1 + ……….. )
= 49(10 + 100 + 1000 + …. – 1 – 1 – 1 – ……)
= 49(10 + 102 +103 + …….. -n)
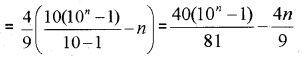
Question 2.
Solve: sin 2x – sin 4x + sin 6x = 0
Answer:
sin 2x + sin 6x – sin 4x = 0
⇒ 2 sin4x cos2x – sin4x = 0
⇒ sin 4x(2 cos2x -1) = 0
⇒ sin4x = 0 or (2 cos2x – 1) = 0
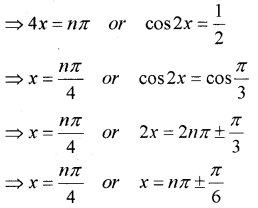
Question 3.
If A and B are events such that
P(A) = 14, P(B) = 12; P(A∩B) = 16
Then find
a) P(A or B)
b) P(not A and not B)
a) P(A or B)= P(A∪B)
= P(A) + P(B) – P(A∩B)
= 14+12−16=3+6−212=712
b) P(not A and not B) = P(A’∩B’)
= P((A∪B)’)
= 1 – P(A∪B) = 1 – 712=512
Question 4.
In a ΔABC , prove that tan(B−C2)=b−cb+ccotA2
Answer:
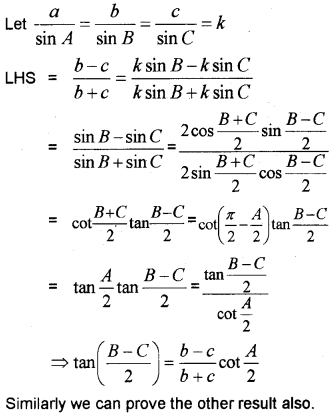
Question 5.
a) The maximum value of the function f(x) = sinx is ………..
(i) 1 (ii) √32 (iii) 12 (iv) 2
b) Prove that; (sin x + cos x)2 = 1 + sin 2x
c) Find the maximum value of sinx + cosx
Answer:
a) a) 1
b) (sinx + cosx)2 = sin2x + cos2x + 2sinx cosx
= 1 + sin 2x
c) The maximum value of sinx is 1, therefore the maximum value of sin2x is also 1.
(sin x + cos x)2 = 1 + sin 2x
sin x + cos x = √1+sin2x
Hence the maximum value of sinx + cosx iS
√1+1=√2
Question 6.
a) limx→2[x] =
(i) 2 (ii) 3 (iii) 0 (iv) does not exist
b) Evaluate : limx→2x3−4x2+4xx2−4
Answer:
a) iv) does not exist.
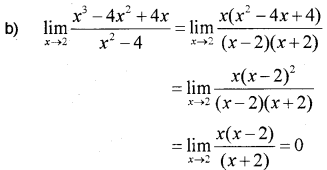
Question 7.
One card is drawn at random from a pack of 52 playing cards. Find the probability that,
a) the card drawn is black.
b) the card is a face card.
c) the card is a black face card.
Answer:

Answer any eight questions from 8 to 17. Each carries 4 scores each. (8 × 4 = 32)
Question 8.
a) If A = {a, b, c}, then write power set of P(A).
b) If the number of subsets with two elements of a set P is 10, then find the total number of elements in set P.
c) Find the number of elements in the power set of P.
Answer:
a) P(A) = {Φ, {a}, {b}, {c}, {a, b}, {a, c}, {b, c}, {a, b, c}}
b) nC2 = 10 ⇒ n(n−1)2 = 10
⇒ n2 – n – 20 = 0
⇒ (n – 5 )(n + 4) = 0 ⇒ n = 5, -4
Total number of elements in set P is 5
c) Number of elements in power set of P is 25 = 32
Question 9.
Consider the Venn diagram of the Universal set U = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13}
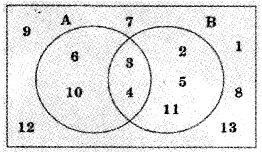
a) Write sets A,B in Roster form.
b) Verify (A∪B)’ = A’∩B’
c) Find n(A∩B)’
Answer:
a) A = {3, 4, 6, 10}; B = {2, 3, 4, 5, 11}
b) From the Venn diagram we can find all the sets.
(A∪B)’ = {1, 7, 8, 9, 12, 13}
A’ = {1, 2, 5, 7, 8, 9, 11, 12, 13}
B’ = {1, 6, 7, 8, 9, 10, 12, 13}
A’∩B’ = {1, 7, 8, 9, 12, 13}
Hence (A∪B)’ = A’∩B’
c) (A∩B)’ = {1, 2, 5, 6, 7, 8, 9, 10, 11, 12, 13}
n((A∩B)’) = 11
Question 10.
Consider the following graphs:
i)
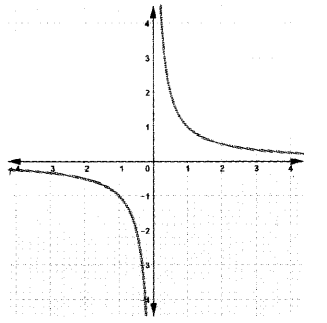
ii)
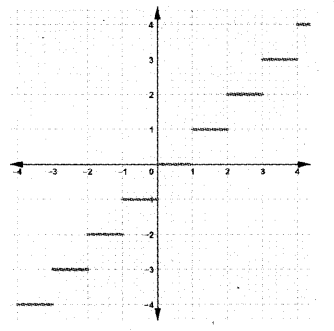
iii)
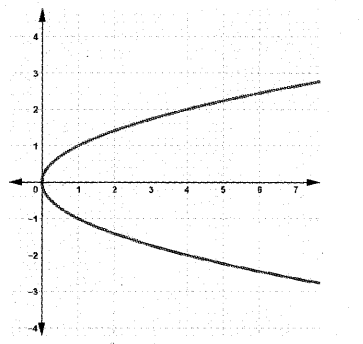
a) Which graph does not represent a function?
b) Identify the function f(x) = 1x from the above graph.
c) Draw the graph of the function f(x) = (x – 1)2
Answer:
a) There are two answers (ii) and (iii)
(vertical line interest at more than two points)
b) i)
c) The graph of f(x) = (x – 1)2 is obtained by shifting the graph of f(x) = x2 to right 1 units.
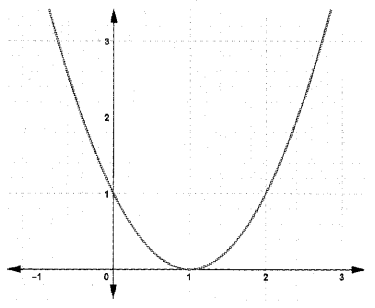
Question 11.
The figure shows the graph of a function f(x) which is a semi circle centered at origin.
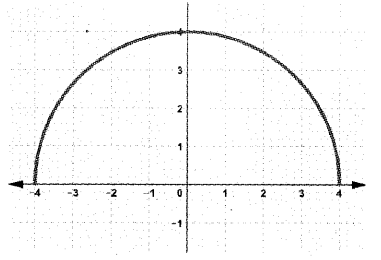
a) Write the domain and range of f(x).
b) Define the function f(x).
Answer:
a) From the graph it is clear that the domain = [-4, 4]; range = [0, 4]
b) The equation of the circle centered at origin with radius 4 is x2 + y2 =16
⇒ y = ±√16−x2
y = √16−x2 represent the upper semicircle and y = –√16−x2 represent the lower semicircle. Therefore the function is of the form f(x) = √16−x2
Question 12.
a) If 32n+2 – 8n – 9 is divisible by ‘k’ for all n∈N is true, then which one of the following is a value of ‘k’?
(i)8 (ii) 6 (iii) 3 (iv) 12
b) Prove by using the principal of Mathematical Induction P(n) = 1 + 3 + 32 + …….. + 3n-1 = 3n−12 is true for all n∈N
Answer:
a) i) 8 P(1) = 32+2 – 8 – 9 = 64

Question 13.
a) Solve the inequality 2x−13≥3x−24−2−x5
b) Represent the solution on a number line.
Answer:
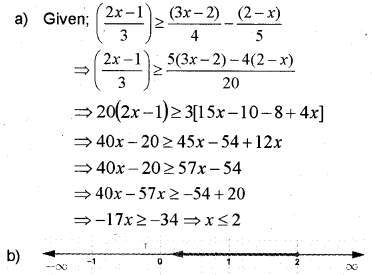
Question 14.
a) Find the nth term of the sequence 3, 5, 7, ………
b) Find the sum to n terms of the series 3 × 12 + 5 × 22 + 7 × 32 + ……….
Answer:
an = 3 + (n – 1)2 = 2n + 1
The series is the combination of two series 3, 5, 7,…….. and 1, 2, 3,……
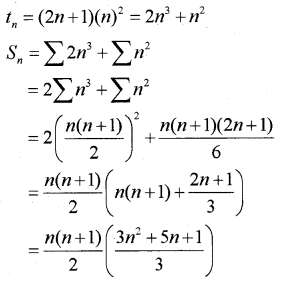
Question 15.
Find the equation of the circle passing through the points (4, 1) and (6, 5) and whose centre is on the line 4x + y = 16
Answer:
Let the equation of the circle be (x – h)2 + (y – k)2 = r2
Since the circle pass through the points (4, 1) and (6, 5)
(4 – h)2 + (1 – h)2 = r2 ……….(1)
(6 – h)2 + (5 – k)2 = r2 ……(2)
4x + y = 16 ……..(3)
Solving the three equations we get h = 3 and k = 4.
(4 – 3)2 + (1 – 4)2 = r2 ⇒ r2 = 10
Hence the equation of the required circle is (x – 3)2 + (y – 4)2 = 10
Question 16.
Consider a point A (4, 8, 10) in space
a) Find the distance of the point A from XY-Plane.
b) Find the distance of the point A from X-axis.
c) Find the ratio in which the line segment joining the point A and B (6, 10, -8) is divided by YZ-plane.
Answer:
a) 10 (z coordinate of the point)
b) The distance from x-axis
= √(8)2+(10)2=√164=2√41
c) When the line segment divides the YZ plane its x coordinate will be zero. Let the intersecting point divides the line segment in the ratio k : 1.
![]()
Therefore the ratio 2 : 3.
Question 17.
a) Which one of the following sentences is a Statement?
i) 275 is perfect square.
ii) Mathematics is a difficult subject.
iii) Answer this question.
iv) Today is a rainy day.
b) Verify by method of contradiction: ‘√2 is irrational’
Answer:
a) i) 275 is perfect square.
b) Assume that √2 is rational. Then √2 can be written in the form √2 = pq, where p and q are integers without common factors.
Squaring; 2 = p2q2 ⇒ 2q2 = p2
⇒ 2 divides p2 ⇒ 2 divides p
Therefore, p = 2k for some integer k.
⇒ p2 = 4k2 ⇒ 2q2 = 4k2 ⇒ q2 = 2k2
⇒ 2 divides q2 ⇒ 2 divides q
Hence p and q have common factor 2, which contradicts our assumption. Therefore, √2 is irrational.
Answer any 5 questions from 18 to 24. Each carries 6 scores. (5 × 6 = 30)
Question 18.
Consider the quadratic equation x2 + x + 1 = 0
a) Solve the quadratic equation.
b) Write the polar form of one of the roots.
c) If the two roots of the given quadratic are α and β. Show that α2 = β
Answer:
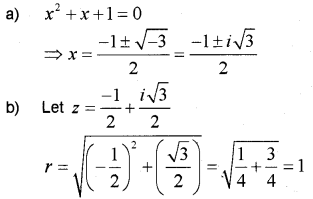
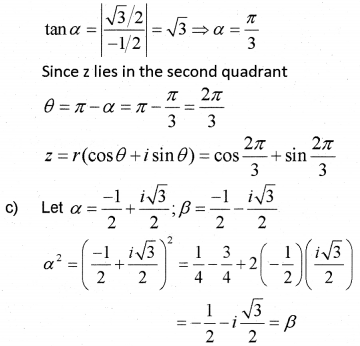
Question 19.
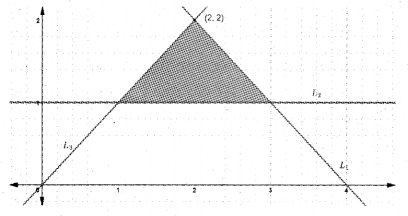
The graphical solution of a system of linear inequalities is shown in the figure.
a) Find the equation of the lines L1, L2, L3
b) Find the inequalities representing the solution region.
Answer:
a) L1 line passes through (2, 2) and (4, 0)
Slope = 2−42−0 = -1
Equation is (y – 0) = -1(x – 4) ⇒ x + y = 4
L2 is parallel to the x-axis and passess through (0, 1). Hence the equation is y =1
L3 passes through (0, 0) and (2, 2). Hence the equation is y = x
b) The inequalities that form the shaded region are x + y ≤ 4; y ≥ 1; y ≤ x
Question 20.
a) Which of the following has its middle term independent of x?
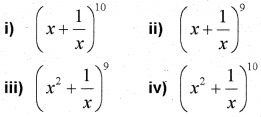
b) Write the expansion of (x2+3x)4
c) Determine whether the expansion of (x2+2x)18 will contain a term containing x10.
Answer:
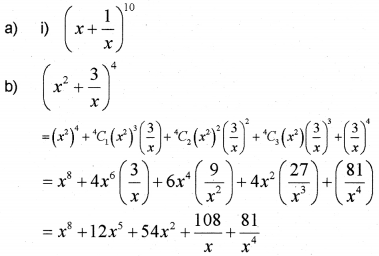
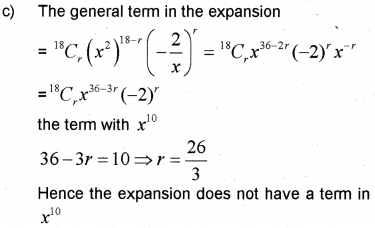
Question 21.
The figure shows an ellipse x225+y29 = 1 and a line L.
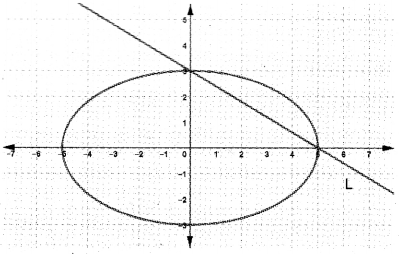
Find the eccentricity and focus of the ellipse.
Find the equation of the line L.
Find the equation of the line parallel to the line L and passing through any one of the foci.
Answer:
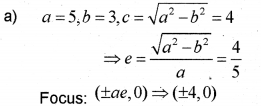

Question 22.
a) Find the derivative of y = sin x from the first principal.
b) Find dydx, if y = x5−cosxsinx
Answer:
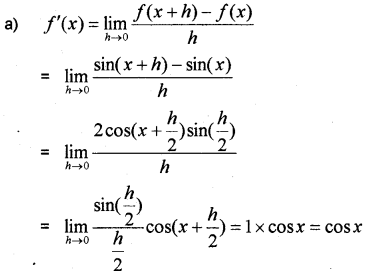
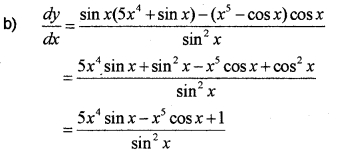
Question 23.
a) Find n, if 12 × (n-1)P3 = 5 × (n+1)P3
b) If nPr=840, nCr=35 , find r.
c) English alphabet has 5 vowels and 21 consonants. How many 4 letter words with two different vowels and two different consonants can be formed without repetition of letters?
Answer:
a) Given; 12(n – 1)P3 = 5(n + 1)P3
⇒ 12(n -1 )(n – 2)(n – 3) = 5 (n + 1)(n)(n – 1)
⇒ 12(n – 2)(n – 3) = 5(n + 1)(n)
⇒ 12(n2 – 5n + 6) = 5n2 + 5n
⇒ 12n2 – 60n + 72 = 5n2 +5n
⇒ 7n2 – 65n + 72 = 0
⇒ 7n2 – 56n – 9n + 72 = 0
⇒ 7n(n – 8) -9 (n – 8) = 0
⇒ (7n – 9)(n – 8) = 0 ⇒ n = 97; 8
Therefore the acceptable value of n = 8
b) nPr = r! × nCr ⇒ 840 = r! × 35 ⇒ r! = 24 ⇒ r = 4
c) Two different vowels can be selected in 5C2.
Two different consonants can be selected in 21C2.
Therefore total numbers of words
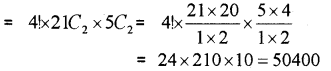
Question 24.
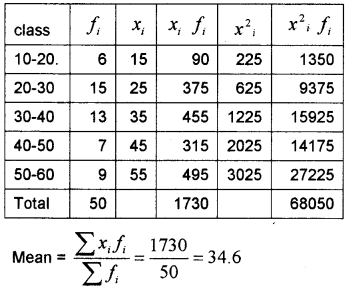
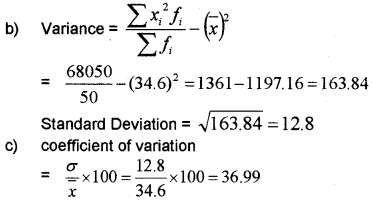
Consider the following distribution:

a) Calculate the mean of the distribution.
b) Find the standard deviation of the distribution.
c) Find the coefficient of variation of the distribution.
Answer: