Plus One Maths Notes Chapter 7 Permutation and Combinations is part of Plus One Maths Notes. Here we have given Kerala Plus One Maths Notes Chapter 7 Permutation and Combinations.
| Board | SCERT, Kerala |
| Text Book | NCERT Based |
| Class | Plus One |
| Subject | Maths Notes |
| Chapter | Chapter 7 |
| Chapter Name | Permutation and Combinations |
| Category | Plus One Kerala |
Kerala Plus One Maths Notes Chapter 7 Permutation and Combinations
I. Fundamental Principle of Counting
If an event can occur in ‘m’ different ways, following which another event can occur in ‘n’ different ways, then the total number of occurrences of the events in the given order is m × n.
II. Permutation
A permutation is the arrangement of some or all of a number of different objects.
Factorial notation: The notation n! represents the product of first n natural numbers,
ie; n! = n(n – 1 )(n – 2) ….. 3.2.1
1. 1! = 1
2. 0! = 1
The number of permutation of ‘n’ different objects taken ‘r’ at a time, where the objects do not repeat is n(n – 1)(n – 2)……(n – r + 1) which is denoted by nPr.
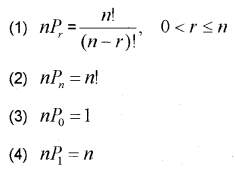
The number of permutation of ‘n’ different objects taken ‘r’ at a time, where repetition is allowed is nr.
Permutation when all the objects are not distinct.
1. The number of permutations of ‘n’ objects, where ‘p’ objects are of the same kind and rest all different =
2. The number of permutations of ‘n’ objects, where ‘p1’ objects are of one kind, ‘p2’ objects are of the second kind, …….., ‘pk‘ objects are of a kth kind and rest all different =
III. Combinations
A combination is a selection of some or all of a number of different objects (the order of selection is not important). The number of selection of ‘n’ things taken ‘r’ at a time is nCr.
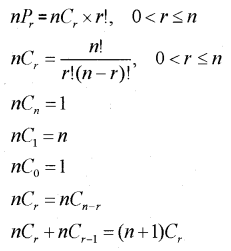
We hope the Plus One Maths Notes Chapter 7 Permutation and Combinations help you. If you have any query regarding Kerala Plus One Maths Notes Chapter 7 Permutation and Combinations, drop a comment below and we will get back to you at the earliest.
