Plus One Maths Notes Chapter 2 Relations and Functions is part of Plus One Maths Notes. Here we have given Kerala Plus One Maths Notes Chapter 2 Relations and Functions.
| Board | SCERT, Kerala |
| Text Book | NCERT Based |
| Class | Plus One |
| Subject | Maths Notes |
| Chapter | Chapter 2 |
| Chapter Name | Relations and Functions |
| Category | Plus One Kerala |
Kerala Plus One Maths Notes Chapter 2 Relations and Functions
I. Cartesian Product or Cross Product:
The Cartesian product between two sets A and B is denoted by A × B is the set of all ordered pairs of elements from A and B.
ie; A × B = {(a, b): a ∈ A, b ∈ B}
Properties:
- In general A × B ≠ B × A, but if A = B, A × B = B × A.
- n(A × B) = n(A) × n(B)
- n(A × B) = n(B × A)
II. Relations:
A relation R from a non-empty set A to a non-empty set B is a subset of the Cartesian product A × B.
Representation of a relation:
- Roster form
- Set builder form
- Arrow diagram.
Universal relation from A to B is A × B.
Empty relation from A to B is empty set φ.
A relation in A is a subset of A × A.
The number of relation that can be written from A to B if n(A) = p, n(B) = q is 2pq.
Domain: It is the set of all first elements of the ordered pairs in a relation.
Range: It is the set of all second elements of the ordered pairs in a relation.
If R: A → B, then R(R) ⊆ B.
Co-domain: If R: A → B, then Co-domain of R = B.
III. Functions:
A relation f from A to B (f : A → B) is said to be a function if every element of set A has one and only one image in set B.
If f : A → B is a function defined by f(x) = y.
- The image of x = y
- The pre-image of y = x
- Domain of f = {x ∈ A : f(x) ∈ B}
- Range of f = {f(x) : x ∈ D(f)}
- If f : A → B, then n(f) = n(B)n(A)
IV. Some Important Functions
Identity function: A function f : R → R defined by f(x) = x. Here D(f) = R, R(f) = R.
The graph of the above function is a straight line passing through the origin which makes 45 degrees with the positive direction of the x-axis.
Constant function: A function f : R → R defined by f(x) = c, where c is a constant.
Here D(f ) = R, R(f) = {c}.
The graph of the above function is a straight line parallel to the x-axis.
Polynomial function: A function f : R → R defined by
f(x) = a0 + a1x + ….. + anxn, where n is a no-negative integer and a0, a1, …., an ∈ R.
Rational function: A function f: R → R defined by f(x)=p(x)q(x), where p(x), q(x) are functions of x defined in a domain, where q(x) ≠ 0
Modulus function: A function f: R → R
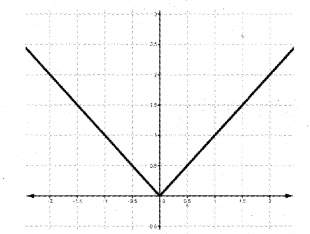
Here D(f) = R, R(f) = [0, ∞).
The graph of the above function is ‘V’ shaped with a corner at the origin.
Signum function: A function f: R → R
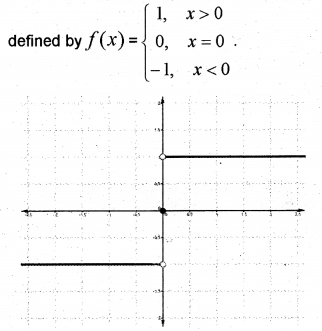
Here D(f) = R, R(f) = {-1, 0, 1}.
The graph of the above function has a break at x = 0.
Greatest integer function f: R → R defined by
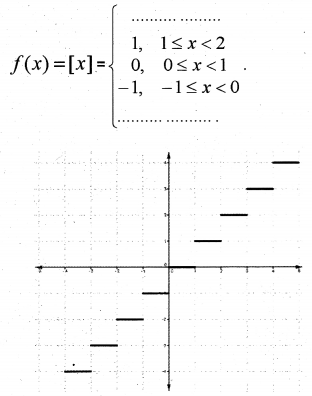
Here D(f) = R, R(f) = Z.
The graph of the above function has broken at all integral points.
V. Algebra of Functions
Let f : X → R and g : X → R be any two real functions, where X ⊂ R. Then, we define (f + g) : X → R by (f + g)(x) = f(x) + g(x) for all x ∈ X
Let f : X → R and g : X → R be any two real functions, where X ⊂ R. Then, we define (f – g) : X → R by (f – g)(x) = f(x) – g(x) for all x ∈ X
Let f : X → R be a real-valued function and k be a scalar. Then, the product kf : X → R by (kf)(x) = kf (x) for all x ∈ X
Let f : X → R and g : X → R be any two real functions, where X ⊂ R . Then, we define fg : X → R by fg(x) = f(x) × g(x) for all x ∈ X
Let f : X → R and g : X → R be any two real functions, where X ⊂ R. Then, we define fg : X → R by
(fg)(x)=f(x)g(x) for all x ∈ X
We hope the Plus One Maths Notes Chapter 2 Relations and Functions help you. If you have any query regarding Kerala Plus One Maths Notes Chapter 2 Relations and Functions, drop a comment below and we will get back to you at the earliest.
