Kerala Plus One Maths Chapter Wise Previous Questions Chapter 6 Linear Inequalities
Plus One Maths Linear Inequalities 4 Marks Important Questions
Question 1.
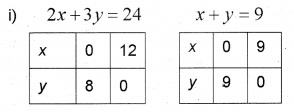
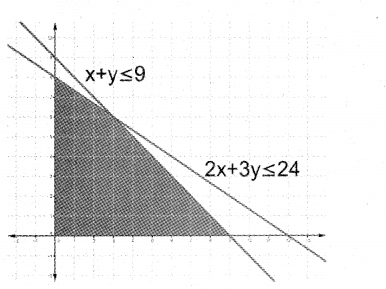
i) Draw the graphs of 2x + 3y = 24 and x + y = 9 (IMP-2011)
ii) Solve the following system of inequalities graphically;
2x + 3y ≤ 24,
x + y ≤ 9, x, y ≥ 0
Answer:
Question 2.
i) Solve 4x – 5 < 7, when x is a real number. (IMP-2012)
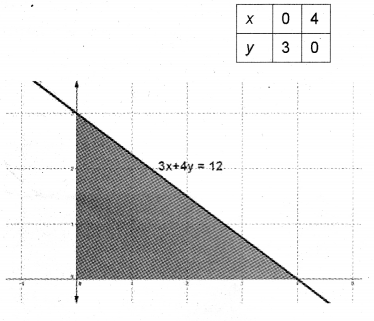
ii) Solve the following system of inequalities
graphically. 3x + 4y ≤ 12, x ≥ 0, y ≥ 0
Answer:
i) 4x – 5 < 7 => 4x < 12 => x < 3
ii) 3x + 4y ≤ 12, x ≥ 0, y ≥ 0
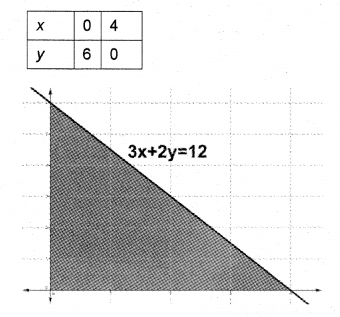
Question 3.
Solve: 4x + 3 < 3x + 7 represent the solution on the real line. (MARCH-2013)
ii) Solve the following system of inequalities graphically.
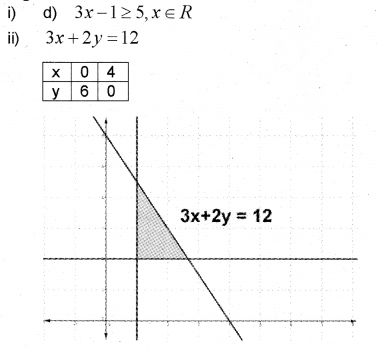
3x + 2y ≤ 12;
x,y ≥ 0
Answer:
i) 4x + 3 < 3x + 7 => 4x – 3x < 7 – 3 => x < 4
ii)
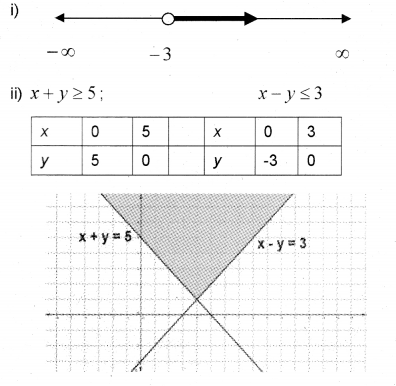
Question 4.
i) Represent the inequality x ≥ – 3 on a number line. (IMP-2014)
ii) Solve the following inequalities graphically:
x + y≥5;
x – y≤ 3
Answer:
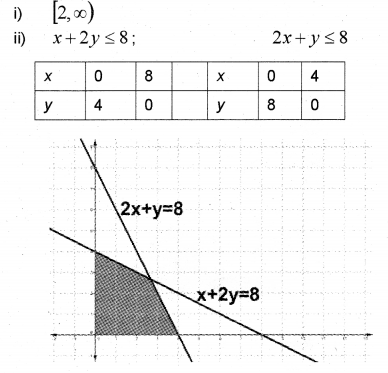
Question 5.
The interval representing the solution of the inequality 3x-1 ≥ 5, x∈R is (MARCH-2015)
a) [5,∞) b) [2, ∞)
c) [3,∞) d) (— ∞, ∞)
ii) Solve the system of inequality graphically
x + 2y ≤ 8,2x + y ≤ 8,
x ≥ 0,y ≥ 0
Answer:
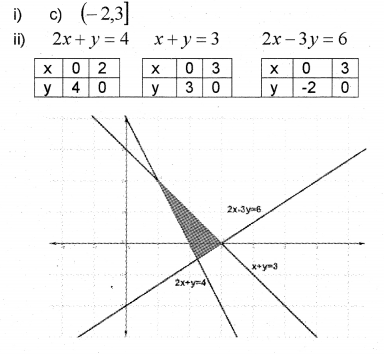
Question 6.
i) Which among the following is the interval corresponding to the inequality – 2 < x ≤ 3 . (MARCH-2016)
(a) [- 2,3]
(b) [- 2,3)
(c) (- 2,3]
(d) (- 2,3)
ii) Solve the following equation.
2x + y ≥ 4;
x + y ≤ 3;
2x – 3y ≤ 6.
Answer:
Question 7.
i) Which among the following inequality represents the intervals [2,∞)
a) x – 3 ≥ 5, x∈R
b) 3x – 3 ≥5, x∈R
c) 3x – 1≥ 3, x∈R
d) 3x – 1 ≥ 5, x∈R
ii) Solve the following system of inequalities graphically. 3x + 2y ≤ 12; x ≥ 1; y ≥ 2
Answer:
Plus One Maths Linear Inequalities 6 Marks Important Questions
Question 1.
i) Solve the inequality 3(x – 1) ≤ 2(x – 3) (MARCH-2010)
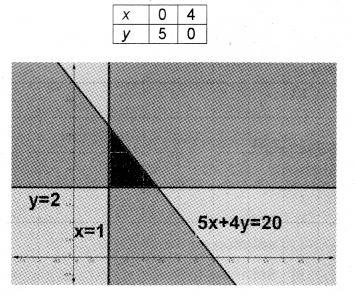
ii) Solve the following system of inequalities graphically. 5x + 4y ≤ 20; x ≥ 1, y ≥ 2
Answer:
i) 3(x – 1) ≤ 2(x – 3) =>3x – 3 ≤2x – 6
=>3x – 2x ≤ 3 – 6
=> x ≤ – 3
ii) 5x + 4y = 20
Question 2.
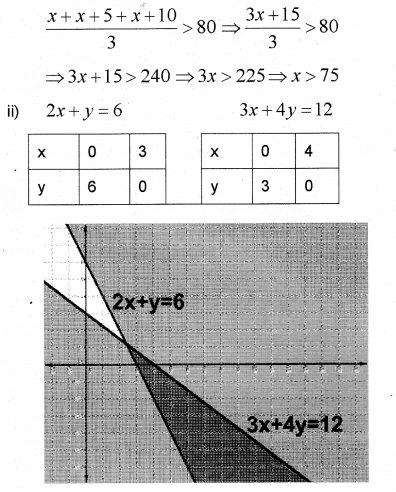
i) Arathi took 3 examinations in an year. The marks obtained by her in the second and third examinations are more than 5 and 10 respectively than in the first examination. If her average mark is at least 80 find the minimum mark that she should get in the final examinations? (IMP-2010)
ii) Solve the following system of inequalities graphically 2x + y ≥6; 3x + 4y ≤12
Answer:
Let x denote the marks of arathi in first examination. then mark in second exam and third exam are x +5 and x + 10 respectively. Given average in three examinations is atleast 80.
Question 3.
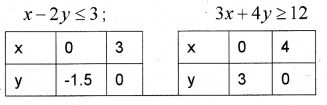
i) Solve the inequality
2(2x + 3) – 10 < 6(x – 2) (MARCH-2011)
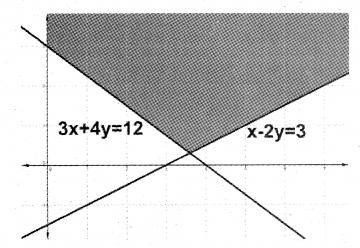
ii) Solve the following inequalities graphically. system of
x – 2y < 3;
3x + 4y ≥ 12; x,y ≥ 0
Answer:
i) 2(2x + 3) – 10 < 6(x – 2)
=> 4x + 6 – 10 ≤ 6x – 12
=> – 2x ≤ -12 + 4
=> – 2x ≤ – 8
=> x ≥ 4
ii)
Question 4.
i) Find all pairs of consecutive odd natural numbers, both of which are smaller than 10, such that their sum is more than 11. (IMP-2012)
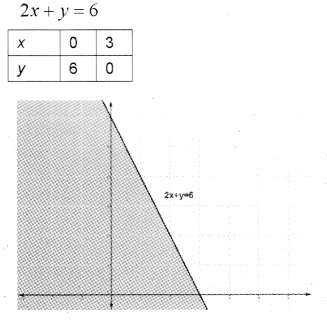
ii) Solve 2x + y ≤ 6 graphically.
Answer:
i) Consecutive odd natural numbers be x and x+2. Then,
x + x + 2 > 11;
x + 2 < 10
=> 2x > 11 – 2;
x < 10 – 2
=> x > 9/5 = 4.5;
x < 8
5 ≤ x < 8 Therefore x can take values 5,7.
Hence the pairs are (5,7),(7,9)
ii)
Question 5.
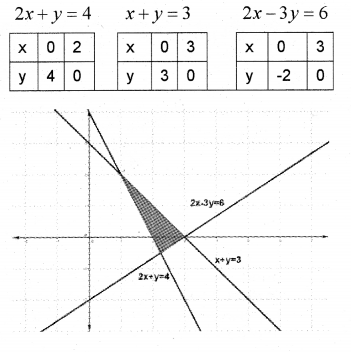
i) Solve the inequality: 3(2 – x) ≥ 2(1 – x) (MARCH-2013)
ii) Solve the following system of inequalities graphically.
2x + y ≥ 4;
x + y ≤ 3;
2x – 3 ≤ 6
Answer:
i) 3(2 – x) ≥ 2(1 – x)
=> 6 – 3x ≥ 2 — 2x
– 3x + 2x ≥ 2 – 6
=>- x ≥ – 4
=> x ≤ 4
ii)
Question 6.
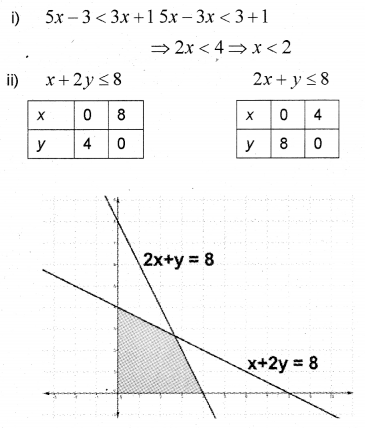
i) Solve: 5x – 3 < 3x + l (MARCH-2014)
ii) Solve the following inequalities graphically.
x + 2y ≤ 8;
2x + y ≤ 8;
x,y ≥ 0
Answer:
Question 7.
i) Raju obtained 70 and 60 marks in first two examinations. Find the minimum mark he should get in the third examination to have an average of atleast 50 marks. (IMP-2013)
ii) Solve the following system of inequalities graphically.
3x + 2y ≤ 12;
x ≥ 1;
y ≥ 2
Answer:
i) Let x be the mark obtained by Raju in third exam. Then,
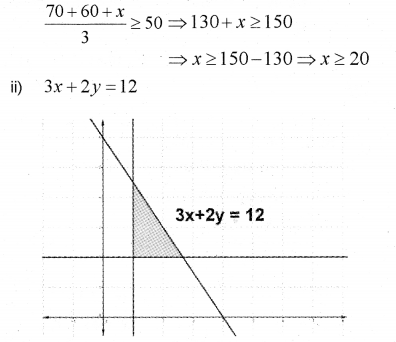
Question 8.
i) Solve: 5x + 3 < 2x + 7 represent the solution on the real line. (MARCH-2014)
ii) Solve the following system of inequalities graphically.
x + 2y ≤ 8;
2x + y ≤ 8;
x, y ≥ 0
Answer:
Question 9.
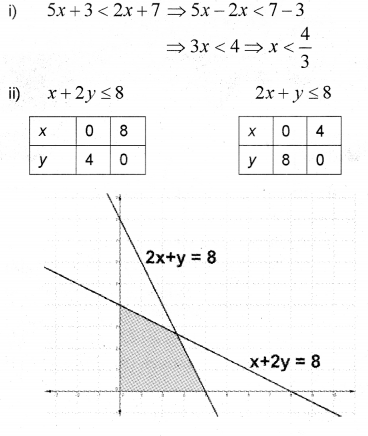
i) Solve: 7x + 3 < 5x + 9 represent the solution on the real line. (MARCH-2014)
ii) Solve the following system of inequalities graphically.
x + 2y ≤ 8;
2x + y ≤ 8;
x, y ≥ 0
Answer:
i)
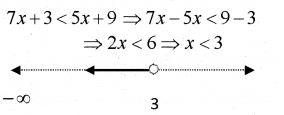
ii)
15
Question 10.
i) Solve 10x – 23 < 3x + 5
ii) Solve the following system of inequalities graphically: 3x + 5y ≤ 15; 5x + 2y ≤ 10; x,y ≥ 0 (IMP-2014)
Answer:
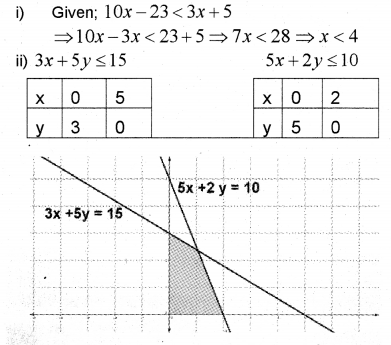
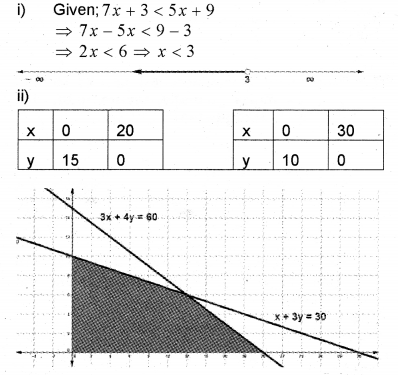
Question 11.
i) Solve; 7x + 3 ≤ 5x + 9; x∈R . Express the solution on a number line. (IMP-2015)
ii) Solve graphically; 3x + 4y ≤ 60;
x + 3y ≤ 30;
x,y ≥ 0.
Answer:
Question 12.
Solve the inequality x3>x2+1x3>x2+1 (MARCH-2017)
Solve the system of inequality graphically
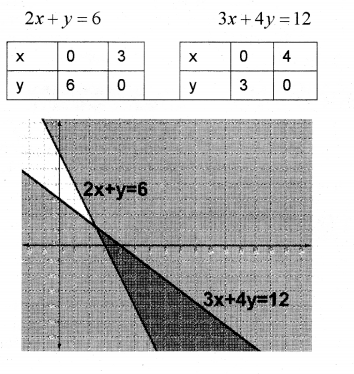
2x + y > 6,3x + 4y ≤ 12
Answer:
i) 2x > 3x + 6
=> – x > 6
=> x < – 6
ii)