Plus One Maths Chapter Wise Previous Questions Chapter 16 Probability

Kerala Plus One Maths Chapter Wise Previous Questions Chapter 16 Probability

Plus One Maths Probability 3 Marks Important Questions

Question 1.
(i) If \(\frac{2}{11} \) is the probability of an event A, then what is the probability of the event ‘not A’? (MARCH-2011)
(ii) If P(A) = \(\frac{3}{5} \) and P(B) = \(\frac{1}{5} \) , then find P(A∪B), if A and B are mutually exclusive events.
(iii) A coin is tossed twice. What is the probability that atleast one tail occurs?
Answer:

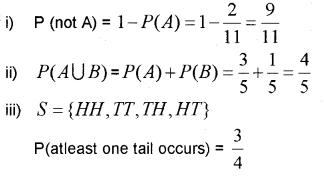
Question 2.
A bag contains 9 balls of which 4 are red, 3 are blue and 2 are yellow. The balls are similar in shape and size. A ball is drawn at random from the bag. Calculate the probability that the ball drawn will be (MARCH-2013)
(i) Red.
(ii) Not yellow.
(iii) Either red or yellow.
Answer:
(i) P (Red) = \(\frac{4}{9} \)
(ii) P(No yellow) \(\frac{7}{9} \)
(iii) P(Either red or yellow) = \(\frac{4+2}{9}=\frac{6}{9}=\frac{2}{3}\)

Question 3.
A and B are two events in a random experiment such that \(P(A)=\frac{1}{3} ; P(B)=\frac{1}{5} ; P(A \cup B)=\frac{7}{15}\) (IMP-2014)
(i) Find P(A∩B)
(ii) Find P(A’)
Answer:

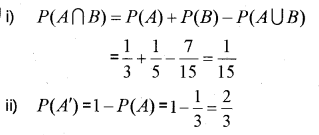
Question 4.
(i) The probability of a sure event is ……… (IMP-2014)
(ii) Two dice are thrown together. What is the probability that the sum of the numbers on the two faces is 8?
Answer:
(i) 1
(ii) A = {(2,6),(3,5),(4,4),(5,3),(6,2)}
\(P(A)=\frac{5}{36}\)

Question 5.
If A and B are two events such that P(A) = 0.42, P(B) = 0.48 andP(yinS) = 0.16 then, find: (IMP-2014)
(i) P(not A)
(ii) P(not B)
(iii) P(A∪B)
Answer:
(i) P(not A) = P(A’) = 1-P(A) = 1-0.42=0.58
(ii) P(not B) = P(B’) -1 -P(B) = 1 -0.48=0.52
(iii) P(A∪B) = P(A) + P(B)-P(A∩B)
= 0.42 + 0.48-0.16 = 0.74

Plus One Maths Probability 4 Marks Important Questions

Question 1.
Two students A and B appeared in an examination. The probability that A passes the examination is 0.25 and that B passes is 0.45. Also the probability that both will pass is 0.1. Find the probability that: (MARCH-2010)
i) Both will not pass.
ii) Only one of them will pass.
Answer:

Question 2.
If M and N are events such that: (IMP-2010)

Find
i) P(M or N)
ii) P(not M and not N)
(Imp (Science) – 2010)
Answer:

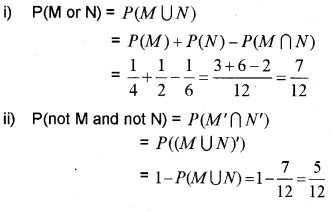
Question 3.
A and B are two events associated with (MARCH-2013)
i) a random experiment such that P(A) = 0.3, P(B) = 0.4 and P(A∪B) = 0.5
a) Find P(A∩B)
b) Find P(A’∪B’)
ii) A coin is tossed twice. What is the probability that at least one tail occurs?
Answer:

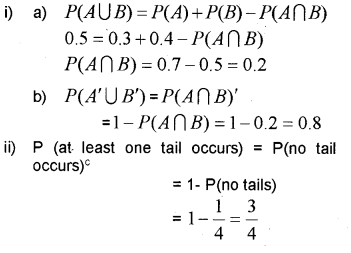
Question 4.
If A and B are two events in a random (IMP-2013)
experiment, then P(A) + P(B)-P(A∩B) = ……….
ii) Given P(A) = 0.5, P(B) = 0.6 and P(A∩B)=03. Find P(A∪B) and P(A’)
iii) Two dice are thrown simultaneously.
Find the probability of getting a doublet.
Answer:

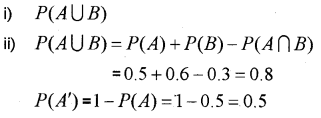
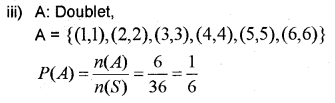
Question 5.
The probability that Ramu pass the examination in both Mathematics and Physics is 0.5, the probability of passing neither Mathematics nor Physics is 0.1, the probability of passing Mathematics is 0.75 (MARCH-2014)
i) What is the probability of passing Mathematics or Physics?
ii) What is probability of passing Physics?
Answer:

Question 6.
If A and B are two events such that (MARCH-2014)

then find;
4 2 8
i) P(A’)
ii) P(A∪B)
iii) P(A∩B)
Answer:

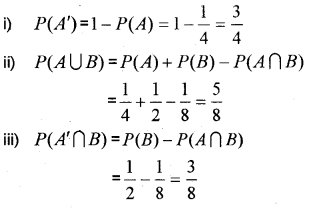
Question 7.
The number of outcomes in the sample space of the random experiment of throwing two dice is… (MARCH-2015)
a) 6³
b)6
c) 6²
d)12
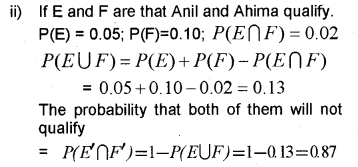
ii) Two students, Anil and Ashima appeared in an examination. The probability that Anil will qualify the examination is 0.05
Answer:
i) 6²

Question 8.
i) If A and B are mutually exclusive and exhaustive events then
P(A) + P(B) = ……….. (IMP-2015)
a) 0
b) 1
c) 1/2
d) 2
ii) Two students A and B appeared in an examination. The probability that A will qualify the examination is 0.25 and B will qualify is 0.45 and both will qualify the examination is 0.1. Find the probability that: (IMP-2015)
a) Both A and B will not qualify the examination.
b) One of them will qualify the examination.
Answer:
i) b) 1

Question 9.
i) In a random experiment, 6 coins are tossed simultaneously. Write the number of sample points in the sample space. (MARCH-2016)
(a) 2² 
(b) 2⁴
(c) 2⁶
(d) 2⁵
ii) Given that P(A) = 0.5, P(B) = 0.6,
P(A∩B) = 0.3
Find P(A’),P(A∪B),P(A’∩B’) and P(A’∪B’)
Answer:

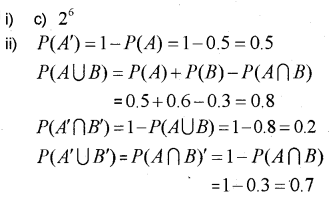
Question 10.
i) If P(A’) = 0.8 .write the value of P(A). (SAY-2017)
ii) In a class of 60 students; 30 selected for NCC, 32 selected for NSS and 24 selected for both NCC and NSS. If one of these students is selected at random, find the probability that:
a) the students selected for NCC or NSS.
b) the students has selected neither NCC nor NSS.
Answer:

Plus One Maths Probability 6 Marks Important Questions

Question 1.
Two dice are thrown. Let A be an event to get an even number on first die and B be an event to get sum of the numbers obtained on two dice is 8. (IMP-2011)
i) Write the sample space.
ii) Write the outcomes favorable to the event A, the event B.
iii) Find P(A or B).
Answer:

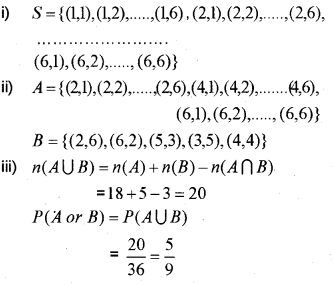
Question 2.
A box contains 6 red, 5 blue and 4 green balls. 3 balls are drawn from the box. Find the probability that (IMP-2011)
i) All are blue.
ii) All balls are either red or blue.
iii) Atleast one green ball.
Answer:

Question 3.
i) A coin is drawn repeatedly until a tail comes up. What is the sample space (IMP-2012)
a) no head
b) exactly one head.
c) atleast one head.
d) atleast two heads.
Answer:

Question 4.
If E and F are two events such that (IMP-2012)

find
i) P(E);P(F)
ii) P(E or F)
iii) P(not E and not F)
Answer:

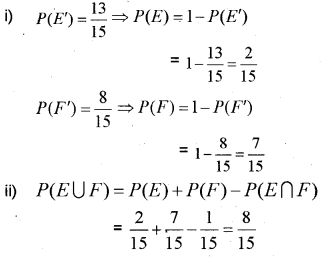
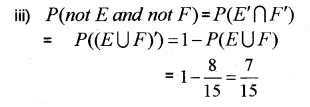
Question 5.
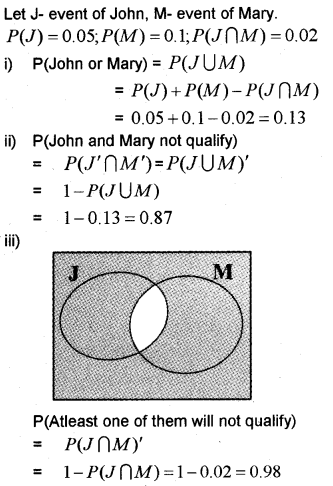
John and Mary appeared in an examination. The probability that John will qualify the examination is 0.05 and that Mary will qualify the examination is 0.10. The probability that both will qualify is 0.02. Find the probability that (MARCH – 2012)
i) John or Mary qualifies the examination.
ii) Both John and Mary will not qualify the examination.
iii) Atleast one of them will not qualify the examination.
Answer:

Question 6.
In a class of 60 students, 30 opted for NCC, 32 opted for NSS and 24 opted for both NCC and NSS. If one of these students is selected at random, find: (MARCH – 2012)
i) The probability that the student opted for NCC or NSS.
ii) The probability that the student has opted for exactly one of NCC or NSS.
Answer:
i) Let the events be defined as A – NCC and B – NSS

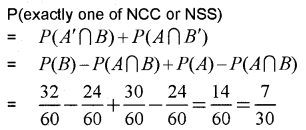
Question 7.
i) A coin is tossed repeatedly until a head comes up. Write the sample space,
ii) If A and B are two events in a random experiment, then (MARCH – 2014)
P(A∪B) = P(A) + P{B) – …………
iii)

Find P(not A and not B).
iv) A bag contains 9 discs of which 4 are red, 3 are blue and 2 are yellow. A disc is drawn at random from the bag. Calculate the probability that it will be
a) Red.
b) Not yellow.

Answer:

Question 8.
Match the following: (MARCH-2017)
i)

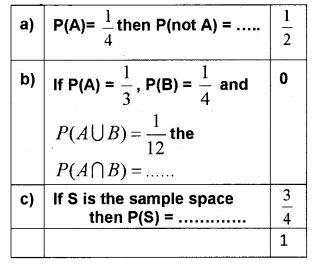
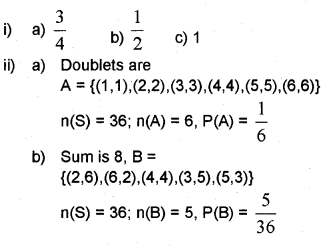
ii) Two dice are thrown at random. Find the probability of
a) Getting a doublet.
b) Getting sum of the numbers on the
dice 8.
Answer:

Plus One Maths Chapter Wise Previous Questions and Answer