Plus One Maths Chapter Wise Previous Questions Chapter 10 Straight Lines

Kerala Plus One Maths Chapter Wise Previous Questions Chapter 10 Straight Lines

Plus One Maths Straight Lines 3 Marks Important Questions

Question 1.
i) Find the slope of the line joining (- 2,6) and (4,8). (MARCH-2010)
ii) Find the value of x if the above line is perpendicular to the line joining (8,12) and (x,24).
Answer:
i)

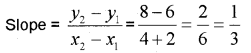
ii) Slope of line through (8,12)and (x,24)

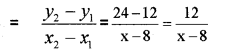
Since both are perpendicular to each other

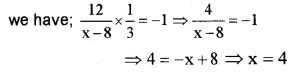
Question 2.
i) Write the equation of y-axis. (IMP-2010)
ii) Find the distance between the lines
8x + 15y – 5 = 0 and 8x + 15y + 12 = 0
(Imp (Science) – 2010)
Answer:
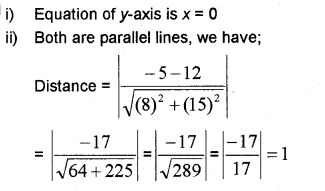
i) Equation of y-axis is x = 0
ii) Both are parallel lines, we have;

Question 3.
The vertices of ∆ ABCare A(2,1), B(- 3,5) and C(4,5). (IMP-2012)
i) Write the coordinates of the midpoint of AC.
ii) Find the equation of the median through the vertex B.
Answer:
i)

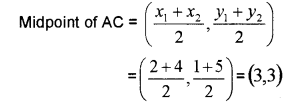
ii) Equation of the median through the vertex B is the equation of a line passing through the midpoint of AC and the vertex B. ie; through (3,3)and (-3,5)

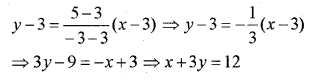
Question 4.
Find the slope of the straight lines √3x + y = 1, x + √3y = 1 (IMP-2012)
Also find the angles between them.
(Imp (Science) – 2012)
Answer:

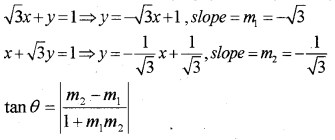
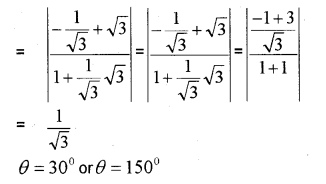
Question 5.
The vertices of ∆ABC are A(-2,3), B(2,-3) and C(4,5). (MARCH-2012)
i) Find the slope of BC.
ii) Find the equation of the altitude of ∆ABC passing through A.
Answer:

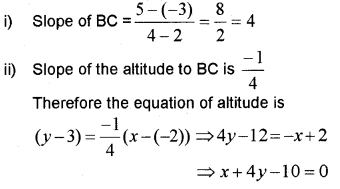
Question 6.
i) Find the slope of the line joining the points (2,2) and (5,3). (MARCH-2013)
ii) Find the equation of the line joining the points (2,2) and (5,3).
Answer:

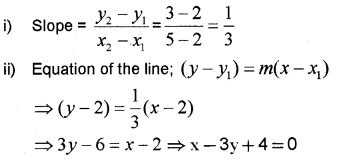
Question 7.
i) If two lines are perpendicular, then the product of their slopes is ______. (MARCH-2013)
ii) Find the equation of a line perpendicular to the line x – 2y + 3 = 0 and passing through the points (1 ,- 2).
Answer:

Question 8.
Consider the line joining the points P(- 4,1) and Q(0,5) (IMP-2013)
i) Write the coordinate of the line passing through the midpoint of PQ .
ii) Find the equation of the line passing through the midpoint of PQ and parallel to the line 3x – 4y + 2 = 0
Answer:
i) Midpoint of PQ =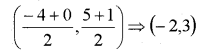
ii) The equation of the parallel line is of the form 3x – 4y + k = 0. Since it passes through (- 2,3) we have;
3(- 2) – 4 + k = 0
=> – 6 – 12 + A: = 0
=>k = 18
Hence the equation is 3x – 4y + 18 = 0

Question 9.
i) Find the slope of the line y = 2x – 3. (MARCH-2013)
ii) Find the equation of the line which makes intercepts – 3 and 2 on the X and Y axes respectively. Find its slope.
Answer:

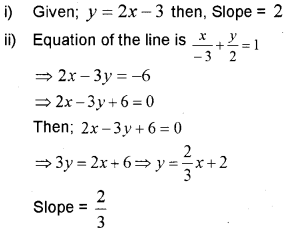
Question 10.
Consider the lines 2x – 3y + 9 = 0 and 2x – 3y + 7 = 0
i) Find the distance from the origin to these two lines.
ii) Find the distance between these two lines.
Answer:

Plus One Maths Straight Lines 4 Marks Important Questions

Question 1.
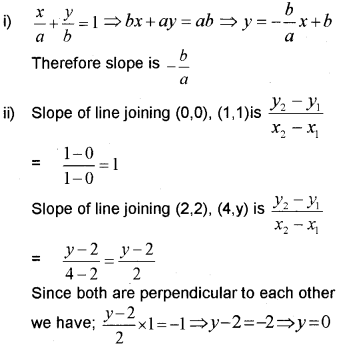
i) Find the slope of the line \(\frac{x}{a}+\frac{y}{b}=1\) (IMP-2010)
ii) If the lines joining the points and are perpendicular (0,0), (1,1) and (2,2), (4,y) find y.
Answer:

Question 2.
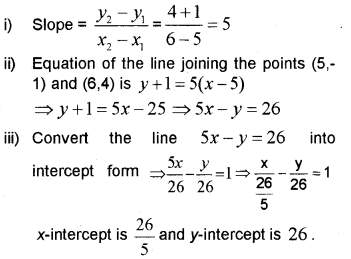
Find slope of the line through the points (5,-1) and (6,4). (IMP-2011)
ii) Find the equation of the line through (5,-1) and (6,4).
iii) Find x intercept and y intercept of this line.
Answer:

Question 3.
i) Find the slope of the line joining the points (3,- 1) and (4,- 2). (IMP-2012)
ii) Find the angle between the positive x-axis and the line joining the points (3,- 1) and (4,- 2).
iii) Find the equation of the line joining the points (3,- 1) and (4,- 2).
Answer:
i)

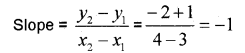
ii) Slope=- 1
=>tanθ = – 1
=>θ = 135°
iii) Equation of the line joining the points (3,1) and (4,- 2) is y +1 = – 1(x – 3)
=>y + 1 = – x + 3
=> x + y = 2

Question 4.
i) Find the point of intersection of the lines 2x + y – 3 = 0,3x – y – 2 = 0.(MARCH-2012)
ii) Find the equation of the line passing through the above point of intersection and parallel to the linex + y + 1 = 0
Answer:
i)
2x + y = 3 ……..(1)
3x-y = 2 …………(2)
(1) + (2)
=> 5x = 5
=>x = 1
(1)=> y = 3 – 2 =1
Intersection point is (1,1)
ii) Equation of the parallel line x + y + k = 0 Since it passes through (1,1) we have;
1 + 1 + A = 0
=>k = -2
Equation is x + y- 2 = 0

Question 5.
Consider the line x + 3y – 7 = 0 (IMP-2013)
i) The slope of the line is ……….
ii) Find the image of the point (3,8) with respect to the given line.
Answer:
i) We have; x + 3>’-7 = 0
\(y=-\frac{1}{3} x+\frac{7}{3}\)
Hence the slope = – \(\frac { 1 }{ 3 }\)

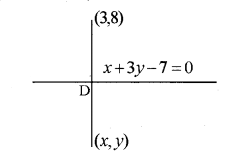
ii) The equation of the perpendicular to the given and passing through (3,8) is
(y – 8) = 3(x – 3)
=>y – 8 = 3x – 9
=> 3x – y = 1
Solving
=> 3x – y = 1 and x + 3y = 7
we get the coordinate of D, which is the midpoint of the points (3,8) and (x,y).
3x – y = 1
3x + 9y = 21
– 10y = – 20
=> y – 2;
x + 3y = 7
=> x = – 3 + 7 = 1
Hence midpoint is (1,2)
Therefore the coordinate of the image is
\(\left(\frac{x+3}{2}, \frac{y+8}{2}\right)=(1,2)\)
=>x + 3 = 2
=>x = – 1
=>y + 8 = 4
=>y = – 4
Hence (- 1,- 4)

Question 6.
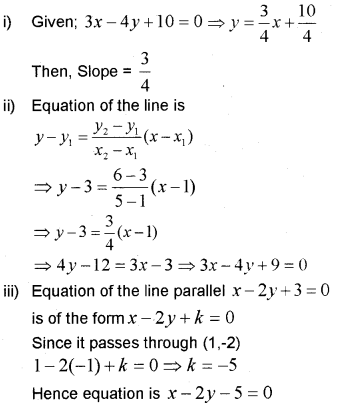
Find the slope of the line 3x – 4y + 10 = 0 (MARCH-2014)
ii) Find the equation of the line passing through the points (1,3) and (5,6).
iii) Find the equation of the line parallel to x – 2y + 3 = 0 and passing through the point (1,- 2).
Answer:

Question 7.
i) Find the slope of the line passing through the points (3,- 2) and (- 1,4). (MARCH-2014)
ii) Find the distance of the point (3,- 5) from the line 3x – 4y – 26 = 0
iii) Consider the equation of the line 3x – 4y + 10 = 0
Find its
a) Slope.
b) x and y intercepts.
Answer:

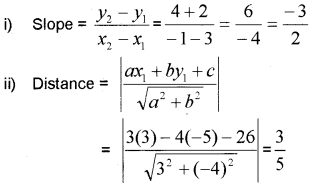
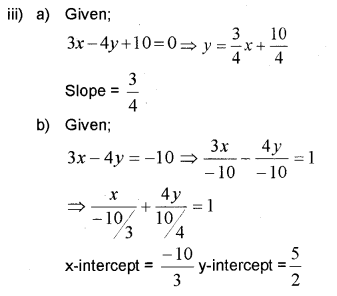
Question 8.
i) Find the equation of the line passing through (4,2) with a slope 2. (IMP-2014)
ii) Convert the above equation into intercept form. Find x and y intercepts.
Answer:
i) The equation of the line is
y – 2 = 2(x – 4)
=> y – 2 = 2x-8
=> 2x – y – 6 = 0
ii) Given
=> 2x – y – 6 = 0

x – intercept = 3;
y – intercept = – 6

Question 9.
i) Find the equation of the line passing through the two points (1,-1) and (3,5). (IMP-2014)
ii) Find the angles between the lines
y – √3x – 5 = 0 and √3y – x + 6 = 0
Answer:

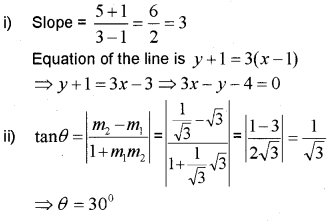
Question 10.
Slope of the line L : 2x + 3y + 5 = 0 is. (IMP-2015)
a) \(\frac { 2 }{ 3 }\)
b) – \(\frac { 2 }{ 3 }\)
c) – \(\frac { 3 }{ 2 }\)
d) \(\frac { 3 }{ 2 }\)
ii) Find the equation of the line L’ parallel to L and passing through (2, 2). Find the distance of the lines L and L’
from the origin. Also find the distance between the lines L and L’.
Answer:

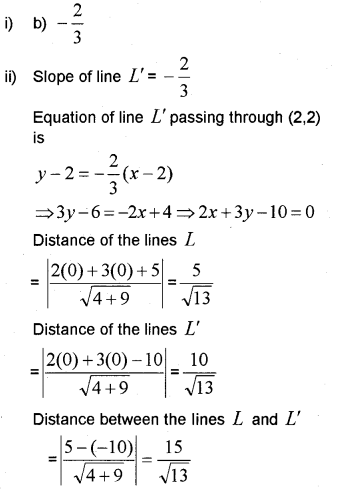
Question 11.
The slope of the line passing through the points (3,-2) and (7,-2) is (MARCH-2017)
(a) – 1
(b) 2
(c) 0
(d) 1
ii) Reduce the equation 6x + 3y – 5 = 0 into slope intercept form and hence find it slope and y-intercept.
iii) Find the point on the x-axis which equidistant from the points (7,6) and (3,4).
Answer:

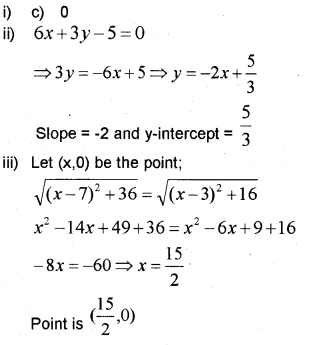
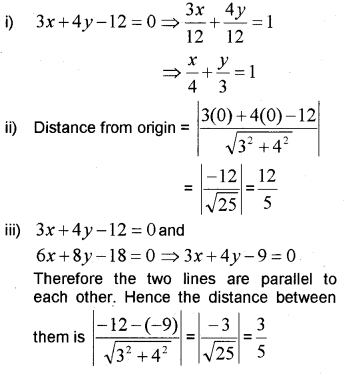
Plus One Maths Straight Lines 6 Marks Important Questions

Question 1.
Reduce the equation3x + 4y – 12 = 0 into intercept form. (MARCH-2010)
Find the distance of it from the origin. Find the distance of the above line from the line 6x + 8y – 18 = 0
Answer:

Question 2.
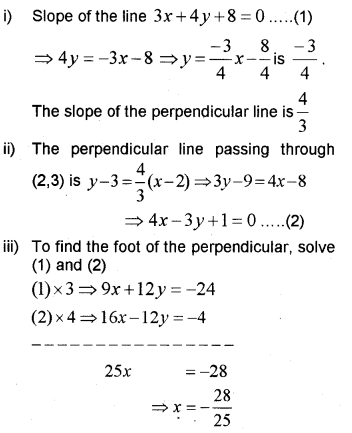
Consider the straight line 3x + 4y + 8 = 0 (MARCH-2011)
i) What is the slope of the line which is perpendicular to the given line?
ii) If the perpendicular line passes through (2,3) from its equation.
iii) Find the foot of the perpendicular drawn from (2,3) to the given line.
Answer:

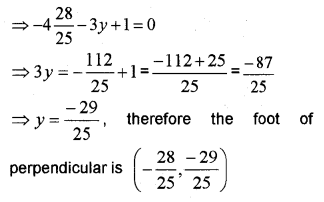
Question 3.
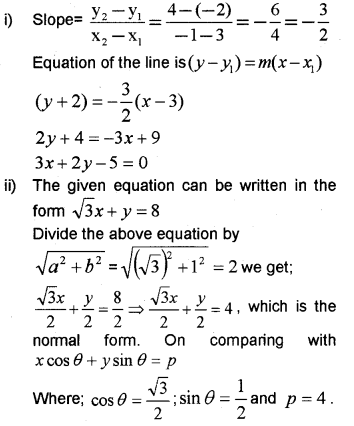
i) Find the equation of the line passing through the points (3,- 2) and (- 1,4). (MARCH-2015)
ii) Reduce the equation √3x + y – 8 = 0 into normal form.
iii) If the angle between two lines is \(\frac { π }{ 4 }\) and slope of one of the lines is \(\frac { 1 }{ 2 }\), find the slope of the other line.
Answer:

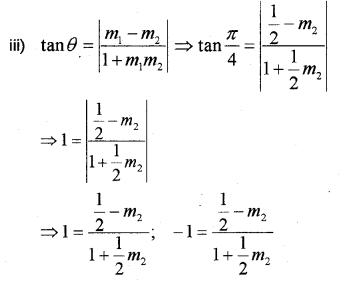
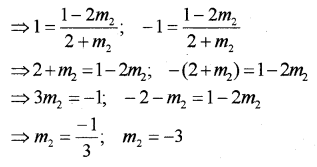
Question 4.
i) The Slope of a line ‘ L¹‘ making an angle 135° with direction of the positive direction of x-axis is (IMP-2015)
(a)1
(b)- 1
(c)√3
(d)-√3
ii) Find the equation of the line L² perpendicular to L¹ and passing through the point (- 2, 3).
iii) Find the equation of a line passing
through the intersection of x + 2y – 3 = 0 and 4x – y + 7 = 0 and which is parallel to 5x + 4y – 20 = 0 .
Answer:
i) tan(135°) = tan(90 + 45) = -1
ii) Slope of line L²= 1
Equation of line L² passing through (- 2,3)
is y – 3 = 1(x + 2)
=>y – 3 = x + 2
=>x – y + 5 = 0
iii) let the equation line passing through the intersecting point is
x + 2y – 3 + k( 4x – y + 7) = 0
=> (1 + 4k)x + (2 – k)y – 3 + 7k = 0

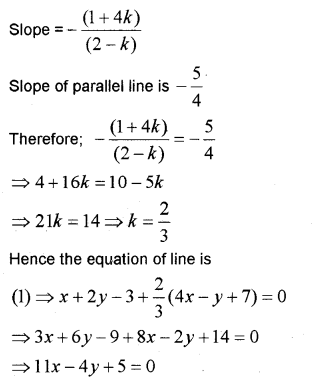
Question 5.
Which one of the following pair of straight lines are parallel? (MARCH-2016)
a) x – 2y – 4 = 0;2x – 3y – 4 = 0
b) x – 2y – 4 = 0;x – 2y – 5 = 0
c) 2x – 3y – 8 = 0,3x – 3y – 8 = 0
d) 2x – 3y – 8 = 0;3x – 2y – 8 = 0
ii) Equation of a straight line is 3x – 4y + 10=0. Convert it into the intercept form and write the x-intercept and write the x-intercept and y- intercept.
iii) Find the equation of the line perpendicular to the line x – 7y + 5 = 0 and having x-intercept 3.
Answer:

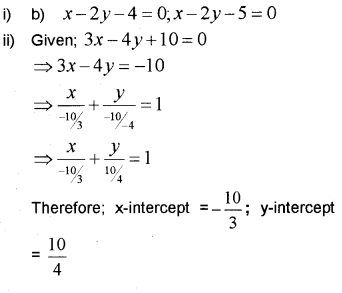
iii) The equation of the perpendicular line will be 7x + y + k = 0.
Since x – intercept is 3, the line passes through the point (3,0). So we have;
7(3) + 0+k = 0
=>21 + 0 + k = 0
=>k = – 21
Therefore the equation is 7x + y – 21 = 0.

Question 6.
Which is the slope of the line perpendicular to the line with slope –\(\frac { 3 }{ 2 }\)?(MAY-2016)
(a) –\(\frac { 3 }{ 2 }\)
(b) –\(\frac { 2 }{ 3 }\)
(c) \(\frac { 3 }{ 2 }\)
(d) \(\frac { 2 }{ 3 }\)
ii) Find the equation of the line intersecting the x-axis at a distance of 3 units to the left of origin with slope – 2.
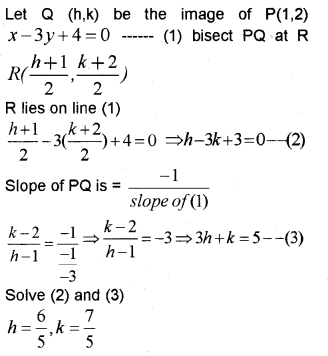
iii) Assume that straight tines work as the plane mirror for a point, find the image of the point (1,2) in the line x – 3y + 4 = 0
Answer:
i) (d) \(\frac { 2 }{ 3 }\)
ii) Slope is m=- 2 and point is (- 3,0)
Equation is y – yx = mix – xx)
=> y – 0 = – 2(x + 3)
=> y = – 2x – 6
iii)

Plus One Maths Chapter Wise Previous Questions and Answer