Plus One Chemistry Notes Chapter 5 States of Matter

Kerala Plus One Chemistry Notes Chapter 5 States of Matter

Introduction
The observable characteristics of chemical systems represent the bulk properties of matter. Chemical properties do not depend the physical state of matter but chemical reactions do.

Inter Molecular Forces
Intermolecular forces are the forces of attraction and repulsion between interacting particles (atoms and molecules). This term does not include the electrostatic forces that exist between the two oppositely charged ions and the forces that hold atoms of a molecule together i.e., covalent bonds.

Dispersion Forces Or London Forces
A nonpolar atom or molecule has a positive centre surrounded by a symmetrical negative electron cloud. The displacement of electron cloud creates an instantaneous dipole temporarily. This instantaneous dipole distorts the electron distribution of other atoms or molecules which are close to it and induces dipole in them also. In this way, a large number of nonpolar molecules become temporarily polar and they mutually attracted by weak attractive forces. These forces are very weak and are known to operate in all types of molecules.

Dipole-Dipole Forces
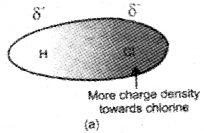
These type of interactions occur in polar molecules having permanent dipoles such as HCl, HBr, H₂S, etc. Such molecules possess partial charges of opposite sign at their ends. The positive end of one molecule attracts the negative end of the other molecule and vice versa. A simple example is the of H-Cl in which chlorine being more electronegative acquires slight negative charge whereas hydrogen becomes slightly positively charged. The dipole-dipole inter-action then takes place in H-Cl as follows.

Dipole-Induced Dipole Forces
These type of interactions are found in a mixture, containing polar and nonpolar molecules. When a nonpolar molecule is brought neara polar molecule, the positive end of the polar molecule attracts the electron cloud of the nonpolar molecule. Thus a polarity is induced in the nonpolar molecule. Then there will be attractive interacting between the polar molecule and the induced dipole of the nonpolar molecule.

Hydrogen Bond
Hydrogen bond can be defined as the attractive force which binds hydrogen atom of one molecule with the electronegative atom (F, O or N) of another molecule. When hydrogen is bonded to strongly electronegative element ‘X’, the electron pair shared between the two atoms moves far away from hydrogen atom. As a result, the hydrogen atom becomes, highly electropositive with respect to the other atom ‘X’. Since there is displacement of electrons towards X, the hydrogen acquires fractional positive charge (δ⁺) while ‘X’ attain fractional negative charge (δ^–). This results in the formation of a polar molecule having electrostatic force of attraction which can be represented as: H^δ+ – X^δ-

The magnitude of H-bonding depends on the physical state of the compound. It is maximum in the solid state and minimum in the gaseous state. Thus, the hydrogen bonds have strong influence on the structure and properties of the compounds.

Types of Hydrogen Bonds
There are two types of hydrogen bonds

1. Intermolecular hydrogen bond
2. Intramolecular hydrogen bond

1. Intermolecular hydrogen bond:
It is formed between two different molecules of the same or different compounds. For example, H-bond in case of HF molecule, alcohol or water molecules, etc.

2. Intramolecular hydrogen bond:
It is formed when hydrogen atom is in between the two highly electronegative (F, O, N) atoms present within the same molecule. For example, in o-Nitrophenol the hydrogen is in between the two oxygen atoms as shown below:

Thermal Energy
Thermal energy is the energy of a body arising from motion of its atoms or molecules. It is directly proportional to the temperature of the substance. The movement of particles using thermal energy is called thermal motion.

Intermolecular Forces Vs Thermal Interactions
Intermolecular forces tend to keep the molecules together but thermal energy of the molecules tends to keep them apart. Three states of matter are the result of balance between intermolecular forces and the thermal energy of the molecules.

The Gaseous State

• Gases are highly compressible.
• Gases exert pressure equally in all directions.
• Gases have much lower density than the solids and liquids.
• The volume and the shape of gases are not fixed. These assume volume and shape of the container.
• Gases mix evenly and completely in all proportions without any mechanical aid.

The Gas Laws

Boyle’s Law (Pressure – Volume Relationship)
On the basis of his experiments, Robert Boyle reached to the conclusion that at constant temperature, the pressure of a fixed amount of gas
varies inversely with its volume. This is known as Boyle’s law. It can be written as p ∝ \(\frac{1}{V}\)
where temperature(T) and number of moles(n)are constant.

If a fixed amount of gas at constant temperature T occupying volume V₁ at pressure p₁ undergoes expansion, so that volume becomes V₂ and pressure becomes p₂, then according to Boyle’s law :
p₁V₁ = p₂V₂ = constant
\(\Rightarrow \frac{p_{1}}{p_{2}}=\frac{V_{2}}{V_{1}}\)

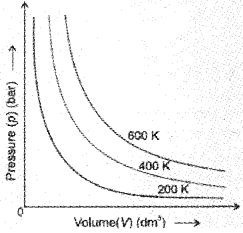
Here T is constant and the graph is called isotherm

Charles’ Law (Temperature – Volume Relationship)
Charles’ law, which states that pressure remaining constant, the volume of a fixed mass of a gas is directly proportional to its absolute temperature. According to this law

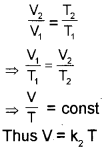
Here we use new temperature scale called the kelvin temperature scale or Absolute temperature scale, t °C in Celsius scaleis equal to (273.15+t) kelvin in kelvin scale.

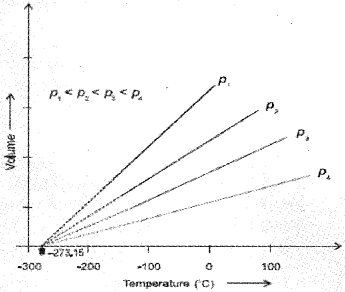
Each line of the volume vs temperature graph is called isobar. The lowest hypothetical or imaginary temperature at which gases are supposed to occupy zero volume is called Absolute zero.
We can see that the volume of the gas at – 273.15 °C will be zero.

Gay Lussac’s Law (Pressure-Temperature Relationship)
The relationship between pressure and temperature was given by Joseph Gay Lussac and is known as Gay Lussac’s law. It states that at constant volume, pressure of a fixed amount of a gas varies directly with the temperature. Mathematically,
P ∝ T
⇒ \(\frac{p}{T}\) = constant
This relationship can be derived from Boyle’s law and Charles’ Law.Each line of Pressure vs temperature (kelvin) graph at constant molar volume is called isochore.

Avogadro Law (Volume -Amount Relationship)
In 1811 Italian scientist Amedeo Avogadro tried to combine conclusions of Dalton’s atomic theory and Gay Lussac’s law of combining volumes which is now known as Avogadro law. It states that equal volumes of all gases under the same conditions of temperature and pressure contain equal number of molecules.

Mathematically we can write v α n where n is the number of moles.

The number of molecules in one mole of a gas has been determined to be 6.022 *1023and is known as Avogadro constant. A gas that follows Boyle’s law, Charles’ law and Avogadro law strictly is called an ideal gas.

Ideal Gas Equation
The combination of Boyle’s law, Charles’ law, and Avagadro’s law leads to an equation which gives the combined effect of change of temperature and pressure on the volume of a gas.
According to Boyle’s law, V α \(\frac{1}{P}\) ——- (i) (at constant T and n)
According to Charles’ Law, V α T ——- (ii) (at constant P and n)
According to Avogardro’s Law, V α n ——- (iii) (at constant T and P

Where R is a constant known as the universal gas constant. The equation is known as ideal gas equation.

Density and Molar Mass of a Gaseous Substance
Ideal gas equation can be rearranged as follows:

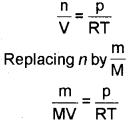
we get \(\frac{d}{M}=\frac{p}{R T}\)
(where d is the density)
On rearranging equation we get the relationship for calculating molar mass of a gas.
\(M=\frac{d R T}{p}\)

Dalton’s Law of Partial Pressures
The law was formulated by John Dalton in 1801. It states that the total pressure exerted by the mix-ture of non-reactive gases is equal to the sum of the partial pressures of individual gases.
P_Total = P₁ + P₂ + P₃ + ………. (at constant T, V)

where p_total is the total pressure exerted by the mixture of gases and p₁, p₂, p₃ etc. are partial pressures of gases.

Partial pressure in terms of mole fraction

Kinetic Molecular Theory Of Gases
Maxwell, Boltzmann, and others put forward a theoretical model of the gas. The theory is known as
Kinetic molecular theory of gases or microscopic
model of gases.
Postulates of kinetic molecular theory.

1.  All gases are made up of a large number of extremely small particles called molecules.
2. The molecules are separated from one another by large distances so that the actual volume of the molecules is negligible as compared to the total volume of gas.
3. The molecules are in a state of continuous rapid motion in all directions. During their motion, they keep on colliding with one another and also with the walls of the container.
4. Molecular collisions are perfectly elastic i.e. there is no net loss or gain of energy in their collisions. However, there may be redistribution of energy during such collisions.
5. There are no attractive forces between the molecules. They move completely independent of each other.
6. The pressure exerted by the gas is due to the bombardment of its molecules on the walls of the container.
7. At any instant, different molecules possess different velocities and hence different energies. However, the average kinetic energy of the molecules is directly proportional to its absolute temperature.

Behaviour Of Real Gases:
Deviation From Ideal Gas Behaviour
There are two types of curves are seen in the graph. In the curves for dihydrogen and helium, as the pressure increases the value of pV also increases. The second type of plot is seen in the case of other gases like carbon monoxide and methane. In these plots first, there is a negative deviation from ideal behaviour, the pV value decreases with increase in pressure and reaches to a minimum value characteristic of a gas. After that pV value starts increasing. The curve then crosses the line for ideal gas and after that shows positive deviation continuously. It is thus, found that real gases do not follow ideal gas equation perfectly under all conditions.

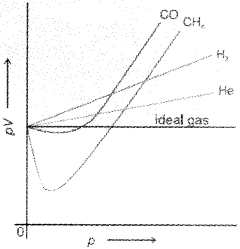
We find that two assumptions of the kinetic theory do not hold good. These are

1. There is no force of attraction between the molecules of a gas.
2. Volume of the molecules of a gas is negligibly small in comparison to the space occupied by the gas.

If assumption (a) is correct, the gas will never liquify. This means that forces of repulsion are powerful enough and prevent squashing of molecules in tiny volume. If assumption (b) is correct, the pressure vs volume graph of experimental data (real gas) and that theoritically calculated from Boyles law (ideal gas) should coincide.

The volume occupied by the molecules also becomes significant because instead of moving in volume V, these are now restricted to volume (V-nb) where nb is approximately the total volume occupied by the molecules themselves. Here, b is a constant. Having taken into account the corrections for pressure and volume, we can rewrite equation as This equation is known as van der Waals’ equation.

Value of ‘a’ is measure of magnitude of intermolecular attractive forces within the gas and is independent of temperature and pressure.
Real gases show ideal behaviour when conditions of temperature and pressure are such that the intermolecular forces are practically negligible. The real gases show ideal behaviour when pressure approaches zero.

The deviation from ideal behaviour can be measured in terms of compressibility factor Z, which is the ratio of product pV and nRT. Mathematically
\(z=\frac{p V}{n R T}\)

For ideal gas Z = 1 at all temperatures and pressures because pV = nRT.
At high pressure, all the gases have Z > 1. These are more difficult to compress. At intermediate pressures, most gases have Z < 1. The temperature at which a real gas obeys ideal gas law over an appreciable. range of pressure is called Boyle temperature or Boyle point.

Liquefaction Of Gases
The highest temperature at which liquefaction of the gas first occurs is called Critical temperature (T_c). Volume of one mole of the gas at critical temperature is called critical volume (V_c) and pressure at this temperature is called critical pressure (P_c).
The critical temperature, pressure, and volume are called critical constants.

Liquid State
Intermolecular forces are stronger in liquid state than in gaseous state.

Vapour Pressure
The pressure exerted by the vapour on the walls of the container is known as vapour pressure.

Surface Tension
Liquids tend to minimize their surface area. The molecules on the surface experience a net downward force and have more energy than the molecules in the bulk, which do not experience any net force. This characteristic property of liquids is known as surface tention. Liquids tend to have minimum number of molecules at their surface due to surface tention.

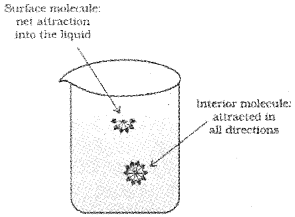
If surface of the liquid is increased by pulling a molecule from the bulk, attractive forces will have to be overcome. This will require expenditure of energy. The energy required to increase the surface area of the liquid by one unit is defined as surface energy.

Viscosity
It is a common observation that certain liquids flow faster than others. For example, liquid like water, ether, etc. flow rapidly while liquids like glycerine, castor oil, honey, etc. flow slowly. These differences in flow rates result from a property known as viscosity. Every liquid has some internal resistance to flow. This internal resistance to flow possessed by a liquid is called its viscosity. Liquids which flow slowly have high internal resistance and are said to have high viscosity. On the other hand, liquids which flow rapidly have low internal resistance and are said to have low viscosity.

Viscosity is also related to intermolecular forces in liquids. If the intermolecular forces are large, the vis-cosity will be high. Viscosity of a liquid decreases with rise in temperature. This is because at higher temperature the attractive forces between molecules are overcome by the increased kinetic energies of the molecules.

Ncert Supplementary Syllabus

Kinetic Energy And Molecular Speeds
Molecules of gases remain in continuous motion. While moving they collide with each other and with the walls of the container. This results in change of their speed and redistribution of energy. So the speed and energy of all the molecules of the gas at any instant are not the same. Thus, we can obtain only average value of speed of molecules. If there are n number of molecules in a sample
and their individual speeds are u₁, u₂, ……… u_n, then average speed of molecules u_av can be calculated as follows:
\(u_{a v}=\frac{u_{1}+u_{2}+\ldots u_{n}}{n}\)

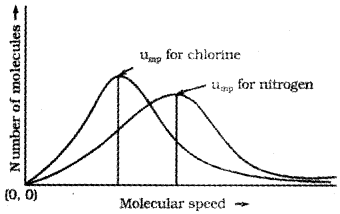
Maxwell and Boltzmann have shown that actual distribution of molecular speeds depends on temperature and molecular mass of a gas. Maxwell derived a formula for calculating the number of molecules possessing a particular speed. Fig. A(1) shows schematic plot of number of molecules vs. molecular speed at two different temperatures T₁ and T₂ (T₂ is higher than T₁). The distribution of speeds shown in the plot is called Maxwell-Boltzmann distribution of speeds.

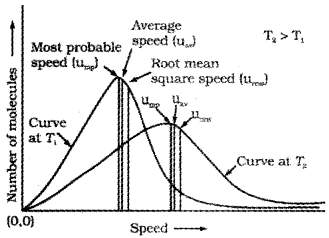
The graph shows that number of molecules possessing very high and very low speed is very small. The maximum in the curve represents speed possessed by maximum number of molecules. This speed is called most probable speed, u_mp. This is very close to the average speed of the molecules. On increasing the temperature most probable speed increases. Also, speed distribution curve broadens at higher temperature. Broadening of the curve shows that number of molecules moving at higher speed increases. Speed distribution also depends upon mass of molecules. At the same temperature, gas molecules with heavier mass have slower speed than lighter gas molecules. For example, at the same temperature, lighter nitrogen molecules move faster than heavier chlorine molecules. Hence, at any given temperature, nitrogen molecules have higher value of most probable speed than the chlorine molecules. Though at a particular temperature the individual speed of molecules keeps changing, the distribution of speeds remains same.

The kinetic energy of a particle is given by the expression:
Kinetic Energy = \(\frac{1}{2}\) mu²
Therefore, if we want to know average translational kinetic energy, \(\frac{1}{2} m \overline{u^{2}}\) , for the movement of a gas particle in a straight line, we require the value of mean of square of speeds, \(\overline{u^{2}}\), of all molecules. This is represented as follows:
\(u^{2}=\frac{u_{1}^{2}+u_{2}^{2}+\ldots . u_{n}^{2}}{n}\)

The mean square speed is the direct measure of the average kinetic energy of gas molecules. If we take the square root of the mean of the square of speeds then we get a value of speed which is different from most probable speed and average speed. This speed is called root mean square speed and is given by the expression as follows:
\(u_{m s}=\sqrt{u_{2}}\)

Root mean square speed, average speed and the most probable speed have following relationship:
u_rms u_av u_mp

The ratio between the three speeds is given below:
u_mp : u_av : u_rms :: 1 : 1.128 : 1.224

Plus One Chemistry Notes