How do you find the Perimeter of a Polygon?
Polygon
A polygon is a simple closed figure that consist of line segments only. Thus, a polygon is a closed rectilinear figure.
The following figures are examples of polygons :
 Regular polygon
Regular polygon
A regular polygon is a polygon in which all the sides are equal.
Perimeter of a Polygon
The sum of the length of all sides of a closed figure is called the perimeter of the figure.
Perimeter of some polygons are given in the table below :
| Figure | Name of the figure | Perimeter |
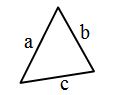 | Scalene Triangle | (a + b + c) Sum of the length of sides |
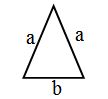 | Isosceles Triangle | (2a + b) 2 × Length of equal sides + Length of unequal side |
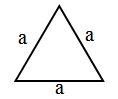 | Equilateral Triangle | 3a 3 × Length of the side of triangle |
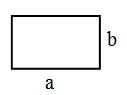 | Rectangle | 2(a + b) 2 (Sum of the length and breadth) |
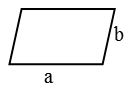 | Parallelogram | 2(a + b) 2 (Length + breadth) |
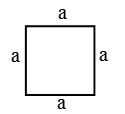 | Square | 4a 4 × Length of the side of square |
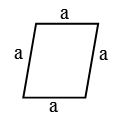 | Rhombus | 4a 4 × Length of the side of rhombus |
Note :
The perimeter of a regular polygon = Number of sides × Length of one side.
Perimeter of a Polygon Problems with Solutions
1. Find the perimeter of the following closed figure :
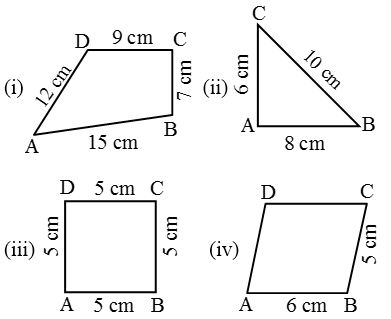
Solution:
(i) Perimeter of quadrilateral ABCD
= AB + BC + CD + DA
= 15 cm + 7 cm + 9 cm + 12 cm = 43 cm
(ii) Perimeter of the triangle ABC
= AB + BC + CA
= 8 cm + 10 cm + 6 cm = 24 cm
(iii) Perimeter of a square
= 4 × length of a side
= 4 × 5 cm = 20 cm.
(iv) Perimeter of a parallelogram
= 2 (length + breadth)
= 2 (6 cm + 5 cm)
= 22 cm.
2. Find the perimeter of the following figures and identify which figure has the greater perimeter?
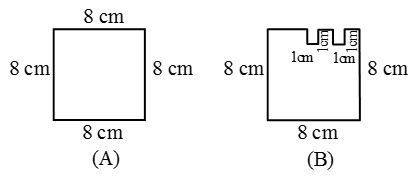
Solution:
Perimeter of figure A, which is a square of side 8 cm = 4 × 8 cm = 32 cm.
Similarly the perimeter of figure B, which is not a square, because two square pieces of side 1 cm are cut off along one side of the square
= 8 cm + 8 cm + (8 cm + 1 cm + 1 cm + 1 cm + 1 cm) + 8 cm = 36 cm.
Clearly, figure B has the greater perimeter than figure A.
Note : When a shape is cut off from a given shape then the perimeter of the new shape so obtained is increased.
3. Nisha wants to fence the rectangular garden in front of her house. Its length is 6 m and breadth is 3 m. The side which is not to be fenced is 6 m in length. Find the cost of fencing at the rate of ₹ 275 per m.
Solution:
The garden is in shape of a rectangle. Its one of the sides (6 m) is not to be fenced. Therefore, the length of fence required is the perimeter of the garden excluding one side.
= 6 m + 3 m + 3 m
= 12 m
The cost of fencing at the rate of ₹ 275 per meter.
= ₹ 275 × 12
= ₹ 3300.
