Notation
What’s the story on this notation between things that are congruent and those that are equal?
The notation used in geometry can often be confusing. The major problems seem to develop when working with segments and angles. Let’s see if we can clarify “what” gets used “when”.
Basic knowledge:
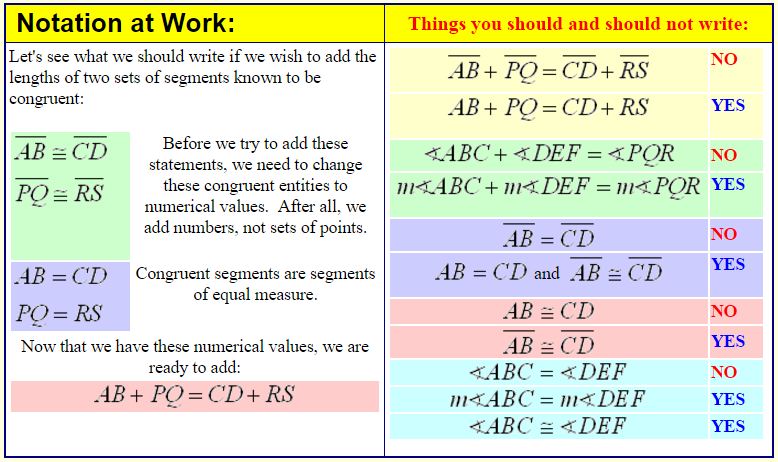
- \(\overline { AB }\) with the bar on top, means the actual segment itself.
- AB without the bar on top, means the length of the segment labeled A and B.
- ∢ABC means the actual angle itself.
- m∢ABC means the measure of the angle labeled A, B and C.
When to use congruent:
- Figures are congruent.
- Segments are congruent.
- Angles are congruent.
- Triangles are congruent.
- This refers to the actual diagrams themselves.
\(\overline { AB }\) ≅ \(\overline { CD }\)
∢ABC ≅ ∢DEF
When to use equal:
- Numerical values are equal.
- When an expression represents a length or measure, equal should be used.
AB=CD
m∢ABC ≅ m∢DEF