NCERT Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3 - A Plus Topper.com

NCERT Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3 are part of NCERT Solutions for Class 10 Maths. Here are we have given Chapter 8 Introduction to Trigonometry Class 10 NCERT Solutions Ex 8.3.

• Introduction to Trigonometry Class 10 Ex 8.1
• Introduction to Trigonometry Class 10 Ex 8.2
• Introduction to Trigonometry Class 10 Ex 8.4

NCERT Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3

NCERT Solutions for Class 10 Maths

Page No : 189

Question 1. Evaluate :
(i) \(\frac { { sin18 }^{ 0 } }{ { cos72 }^{ 0 } } \)
(ii) \(\frac { { tan26 }^{ 0 } }{ { cot64 }^{ 0 } } \)
(iii) cos 48° – sin 42°
(iv) cosec 31° – sec 59°

Solution:

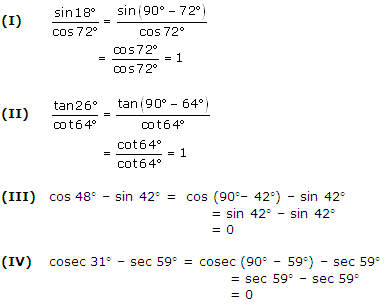
Question 2. Show that :
(i) tan 48° tan 23° tan 42° tan 67° = 1
(ii) cos 38° cos 52° – sin 38° sin 52° = 0

Solution:

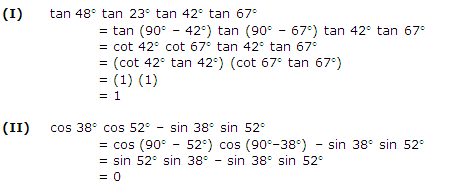
Question 3. If tan 2A = cot (A – 18°), where 2A is an acute angle, find the value of A.

Solution:
Given that
tan 2A = cot (A – 18⁰)
cot (90⁰ – 2A) = cot (A -18⁰)
90⁰ – 2A = A – 18⁰
108⁰ = 3A
A = 36⁰

Question 4. If tan A = cot B, prove that A + B = 90°.

Solution:
Given that
tan A = cot B
tan A = tan (90⁰ – B)
A = 90⁰ – B
A + B = 90⁰

Question 5. If sec 4A = cosec (A – 20°), where 4A is an acute angle, find the value of A.

Solution:
Given that
Sec 4A = cosec (A – 20⁰)
Cosec (90⁰ – 4A) = cosec (A – 20⁰)
90⁰ – 4A = A – 20⁰
110⁰ = 5A
A = 22⁰

Page No : 190

Question 6. If A, B and C are interior angles of a triangle ABC, then
Show that \(sin\left( \frac { B+C }{ 2 } \right) =cos\frac { A }{ 2 } \)

Solution:
We know that for a triangle ∆ABC
∠A + ∠B + ∠C = 180:
∠B + ∠C = 180 – ∠A
\(\frac { \angle B+\angle C }{ 2 } ={ 90 }^{ 0 }-\frac { \angle A }{ 2 } \)
\(sin\left( \frac { B+C }{ 2 } \right) =sin\left( { 90 }^{ 0 }-\frac { A }{ 2 } \right) \)
\(=cos\left( \frac { A }{ 2 } \right) \)

Question 7. Express sin 67° + cos 75° in terms of trigonometric ratios of angles between 0° and 45°.

Solution:
sin 67⁰ + cos 75⁰
= sin (90⁰ – 23⁰) + cos (90⁰ – 15⁰)
= cos 23⁰ + sin 15⁰

We hope the NCERT Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3, drop a comment below and we will get back to you at the earliest.