Math Labs with Activity - Verify the Identity (a-b)² = (a² - 2ab+b²)

Math Labs with Activity – Verify the Identity (a-b)² = (a² – 2ab+b²)

OBJECTIVE

To verify the identity (a-b)² = (a² – 2ab+b²)

Materials Required

1. A piece of cardboard
2. A sheet of glazed paper
3. A sheet of white paper
4. A pair of scissors
5. A geometry box

Procedure
We take distinct values of a and b.
Step 1: Paste the white paper on the cardboard. Draw a square ABCD of side a units.
Step 2: Calculate the value of (a – b). On the glazed paper, construct two rectangles each having length (a-b) units and breadth b units. Also, construct a square of side b units.
Step 3: Cut the square and the two rectangles from the glazed paper and place them on the white paper. Arrange these inside the square ABCD as shown in Figure 11.1.

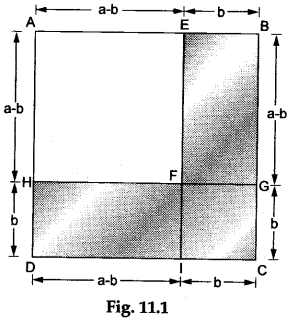
Step 4: Label the diagram as shown in Figure 11.1. Record your observations.

Observations and Calculations
We observe that the area of square AEFH=(a-b)² square units.
Also, area of square AEFH
= area of square ABCD – area of rect. EBGF – area of rect. HFID – area of square FGCI
i. e., (a-b)² = a²-(a-b)b-(a-b)b-b²
=> (a-b)² =a²-ab+b²-ab+b²-b²
=> (a-b)² = (a² – 2ab+b²).

Result
The identity (a-b)² = (a² – 2ab+b²) is verified.

Math Labs with ActivityMath LabsMath Lab ManualScience LabsScience Practical Skills