A Plus Topper

Improve your Grades

Math Labs with Activity - Pythagoras' theorem (Method 3)

by

Math Labs with Activity – Pythagoras theorem (Method 3)

OBJECTIVE

To verify Pythagoras’ theorem (Method 3)

Materials Required

  1. A piece of cardboard
  2. Two sheets of white paper
  3. A pair of scissors
  4. A geometry box
  5. A tube of glue

Theory
Pythagoras’ theorem: In a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

Procedure
Step 1: Paste a sheet of white paper on the cardboard.
On this paper, draw a right-angled triangle ABC, right angled at C. Let the lengths of the sides AB, BC and CA be c, a and b units respectively (see Figure 11.1).
Step 2: Make an exact replica of this ΔABC on the other paper.
Construct a square with the side AB as one of its sides. Now, each side of this square is equal to c units.
Similarly, construct two squares with sides measuring a units and b units along the sides and CA of the ΔABC. Label the diagram as shown in Figure 11.2. Also, shade the squares as shown in Figure 11.2.
Step 3: Produce the side DA of the square DEBA to meet the side IH of the square ACHI at M. At point M, draw NM perpendicular to AM, so that N lies on the side CH of the square ACHI.
Step 4: Produce the side EB of the square DEBA to meet the side CG of the square BFGC at P.
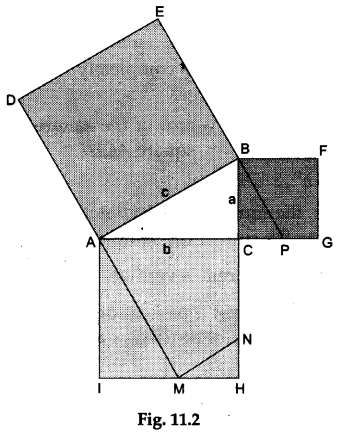
Math Labs with Activity - Pythagoras' theorem (Method 3) 1
Step 5: Cut the squares DEBA, BFGC and ACHI. Also, cut the square BFGC along the line BP and the square ACHI along the lines AM and MN. We thus have a square DEBA, two quadrilaterals—BFGP and ACNM—and three triangles—BCP, AIM and NHM.
Step 6: Arrange the two quadrilaterals and the three triangles on the square DEBA as shown in Figure 11.3.
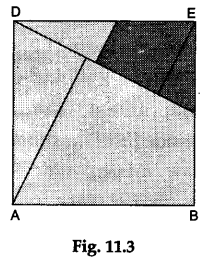
Math Labs with Activity - Pythagoras' theorem (Method 3) 2

Observations and Calculations
We observe that all the parts of the squares BFGC and ACHI, i.e., two quadrilaterals and three triangles completely cover the square DEBA. Therefore,
area of the square DEBA = area of the square BFGC + area of the square ACHI
i.e., c² = a² + b².
In other words, the square of the hypotenuse of right-angled ΔABC is equal to the sum of the squares of the other two sides:

Result
Pythagoras’ theorem is verified.

Remarks:
This method is just a process of verification of Pythagoras’ theorem and cannot be used as a proof of the theorem.

Math Labs with ActivityMath LabsScience Practical SkillsScience Labs