Math Labs with Activity - Find the Centroid of a Given Triangle

Math Labs with Activity – Find the Centroid of a Given Triangle

OBJECTIVE

To find the centroid of a given triangle by the method of paper folding

Materials Required

1. A sheet of white paper
2. A geometry box

Theory
The median of a triangle corresponding to any side is the line segment joining the midpoint of that side with the opposite vertex. The medians of a triangle are concurrent. The point of intersection of all the three medians of a triangle is called its centroid. Since all the medians meet at a single point, it is sufficient to find the point of intersection of only two medians to obtain the centroid of a triangle.

Procedure
Step 1: Draw any triangle ABC on the sheet of white paper. We have to find the centroid of this triangle.
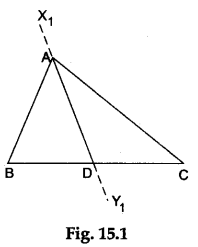
Step 2: Fold the paper along the line that passes through the point A and cuts the line BC such that the point B falls on the point C. Make a crease and unfold the paper. Draw a line X₁Y₁ along the crease. Label the point D where the line X₁Y₁ intersects the line BC.
Then, AD is the median of ΔABC corresponding to ,the side BC as shown in Figure 15.1.

Step 3: Fold the paper along the line that passes through the point B and cuts the line AC such that the point A falls on the point C. Make a crease and unfold the paper. Draw a line X₂Y₂ along the crease. Label the point E where the line X₂Y₂ intersects the line AC. Then, BE is the median of ΔABC corresponding to the side AC as shown in Figure 15.2.

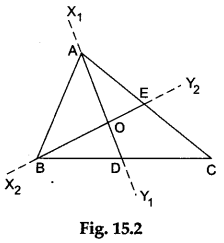
Step 4: Label the point O where the median AD intersects the median BE.

Observation
The point O where the two medians AD and BE of the given triangle ABC intersect is the centroid of ΔABC.

Result
The point O is the centroid of the given triangle ABC. Remark The teacher must ask the students to find the centroid of an obtuse-angled triangle and also of a right-angled triangle following the method described above.

Math Labs with ActivityMath LabsMath Lab ManualScience LabsScience Practical Skills