Linear Equations In One Variable

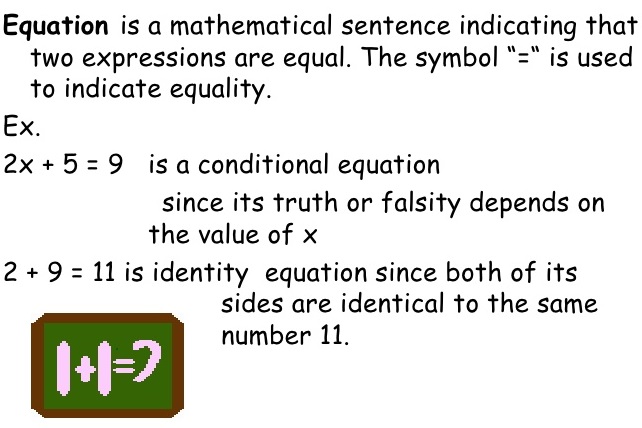
A statement of equality of two algebraic expressions, which involve one or more unknown quantities is known as an equation.
A linear equation is an equation which involves linear polynomials.
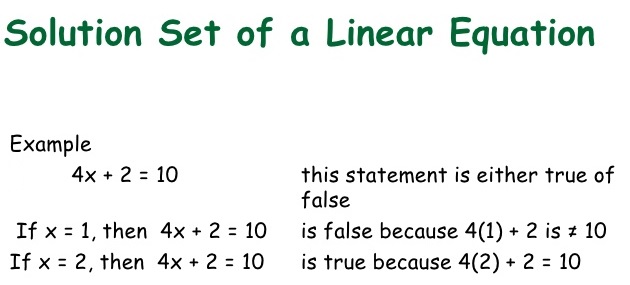
A value of the variable which makes the two sides of the equation equal is called the solution of the equation.
Same quantity can be added/subtracted to/from both the sides of an equation without changing the equality.
Both the sides of an equation can be multiplied/divided by the same non-zero number without changing the equality.

GENERAL FORM OF LINEAR EQUATION IN TWO VARIABLES

ax + by + c = 0, a ≠ 0, b ≠ 0 or any one from a & b can zero.

Read More:

General Form Of Linear Equation In Two Variables Example Problems With Solutions

Example 1: Express the following linear equations in general form and identify coefficients of x, y and constant term.
Solution:

Make linear equation by the following statements:

Example 2: The cost of 2kg of apples and 1 kg of grapes on a day was found to be 160. After a month, the cost of 4 kg of apples and 2 kg of grapes is 300. Represent the situation algebraically.
Solution: Let cost of per kg apples & grapes are x & y respectively then by Ist condition:
2x + y = 160 ……(i)
& by IInd condition: 4x + 2y = 300 …..(ii)

Example 3: The coach of a cricket team buys 3 bats and 6 balls for 3900. Later, she buys another bat and 3 more balls of the same kind for 1300. Represent this situation algebraically.
Solution: Let cost of a bat and a ball are x & y respectively. According to questions
3x + 6y = 3900 …..(i)
& x + 3y = 1300 …..(ii)

https://www.youtube.com/watch?v=fB1evicj5po

Example 4: 10 students of class IX took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys.
Solution: Let no. of boys and girls are x & y then according to question
x + y = 10 ……(i)
& y = x + 4 ……(ii)

Example 5: Half the perimeter of a rectangular garden, whose length is 4 m more than its width, is
36 m.
Solution: Let length & breadth are x m and y m.
∴ according to question 1/2 perimeter = 36
1/2 [2(l + b)] = 36
⇒ x + y = 36 …..(i)
also length = 4 + breadth
x = 4 + y ..…(ii)

Example 6: The difference between two numbers is 26 and one number is three times the other.
Solution: Let the numbers are x and y & x > y
∴ x – y = 26 ……(i)
and x = 3y ……(ii)

Example 7: The larger of two supplementary angles exceeds the smaller by 18 degrees.
Solution: Sol. Let the two supplementary angles are x and y & x > y
Then x + y = 180° ……(i)
and x = y + 18° ……(ii)

Example 8: A fraction becomes 9/11, if 2 is added to both the numerator and the denominator. If, 3 is added to both the numerator and the denominator it becomes 5/6.
Solution: Let fraction is x/y
Now according to question \(\frac{x+2}{y+2}=\frac{9}{11}\)
⇒ 11x + 22 = 9y + 18
⇒ 11x – 9y = – 4 …..(i)
and
\(\frac{x+3}{y+3}=\frac{5}{6}\)
⇒ 6x + 18 = 5y + 15
⇒ 6x – 5y = –3 ….(ii)

Example 9: Five years hence, the age of Sachin will be three times that of his son. Five years ago, Sachin’s age was seven times that of his son.
Solution: Let present ages of Sachin & his son are
x years and y years.
Five years hence,
age of Sachin = (x + 5) years & his son’s age = (y + 5) years
according to question (x + 5) = 3(y + 5)
⇒ x + 5 = 3y + 15
⇒ x – 3y = 10 ……(i)
and 5 years ago age of both were (x – 5) years and (y – 5) years respectively
according to question (x – 5) = 7(y – 5)
⇒ x – 5 = 7y – 35
⇒ x – 7y = –30 .…(ii)