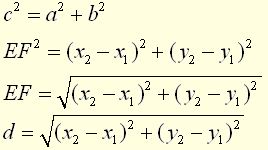Length of a Line Segment (Distance)
When working with Coordinate Geometry, there are many ways to find distances (lengths) of line segments on graph paper.
Let’s examine some of the possibilities:
Method 1:
Whenever the segments are horizontal or vertical, the length can be obtained by counting.
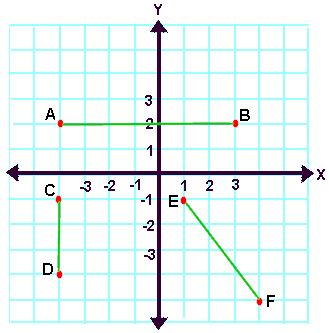When we need to find the length (distance) of a segment such as \(\overline { AB }\) we simply COUNT the distance from point A to point B. (AB = 7)
We can use this same counting approach for \(\overline { CD }\). (CD = 3)
Unfortunately, this counting approach does NOT work for \(\overline { EF }\) which is a diagonal segment.
Method 2:
When working with diagonal segments, the Pythagorean theorem can be used to determine the length.
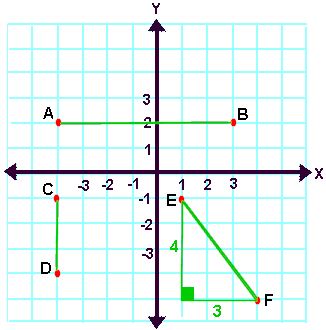Notice how a right triangle was formed with \(\overline { EF }\) as the hypotenuse. The legs of the right triangle are “on” the graph paper and their lengths can be counted. Using the Pythagorean Theorem, we know that:
(EF)2 = 42 + 32
(EF)2 = 25
EF = 5
Use this method of working with the Pythagorean Theorem whenever you forget the Distance Formula!
Method 3:
When working with diagonal segments, use the Distance Formula to determine the length.
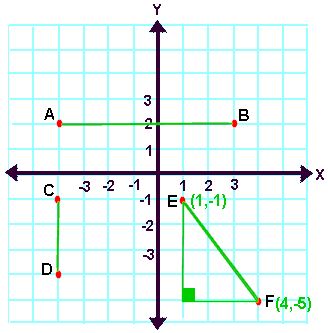The Distance Formula is really just a coordinate geometry way of writing the Pythagorean Theorem. If you cannot remember the Distance Formula, you can always draw a graph and use the Pythagorean Theorem as was done in Method 2.
The advantage of the Distance Formula is that you do not need to draw a picture to find the answer. All you need to know are the coordinates of the endpoints of the segment.
It doesn’t matter which point you start with. Just start with the same point for reading both the x and y coordinates.
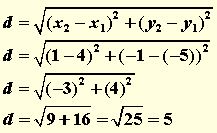
The Distance Formula can be used to find the lengths of all forms of line segments: horizontal, vertical and diagonal.
Deriving the Distance Formula:
Start by drawing a right triangle anywhere on a coordinate grid. Label the endpoints of the segment as (x1, y1) and (x2, y2).
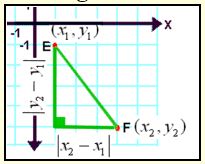Represent the lengths of the legs of the right triangle as the absolute value of the difference of the x and y coordinate values.
Apply the Pythagorean Theorem:
