What are the Laws of Indices?

What are the Laws of Indices?

Definition of indices

If a is any non zero real or imaginary number and m is the positive integer, then a^m = a. a. a. a……. a (m times). Here a is called the base and m is the index, power or exponent.

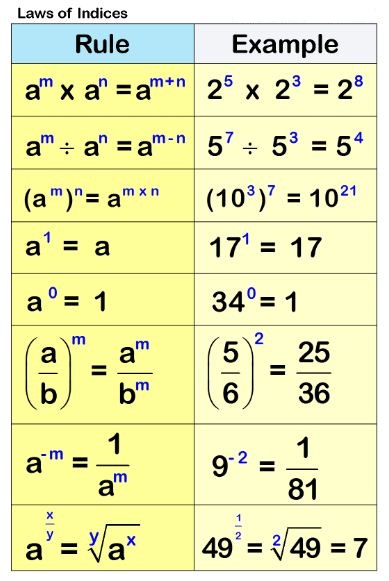
Laws of indices

(7) If x = y, then a^x = a^y, but the converse may not be true.
For example: (1)⁶ = (1)⁸, but 6 ≠ 8

1. If a ≠ ±1 or 0, then x = y.
2. If a = 1, then x, y may be any real number.
3. If a = −1, then x, y may be both even or both odd.
4. If a = 0, then x, y may be any non-zero real number.

But if we have to solve the equations like [f(x)]^ϕ(x) = [f(x)]^ψ(x) then we have to solve :
(a) f(x) = 1
(b) f(x) = −1
(c) f(x) = 0
(d) ϕ(x) = ψ(x)
Verification should be done in (b) and (c) cases
(8) a^m. b^m = (ab)^m is not always true
In real domain, √a√b = √(ab), only when a ≥ 0, b ≥ 0.
In complex domain, √a.√b = √(ab), if at least one of a and b is positive.
(9) If a^x = b^x then consider the following cases :
(i) If a ≠ ±b, then x = 0.
(ii) If a = b ≠ 0, then x may have any real value.
(iii) If a = −b, then  is even.
If we have to solve the equation of the form [f(x)]^ϕ(x) = [g(x)]^ϕ(x) i.e., same index, different bases, then we have to solve
(a) f(x) = g(x),
(b) f(x) = −g(x),
(c) ϕ(x) = 0
Verification should be done in (b) and (c) cases.