Law of Cosines
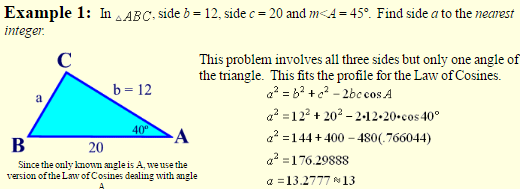
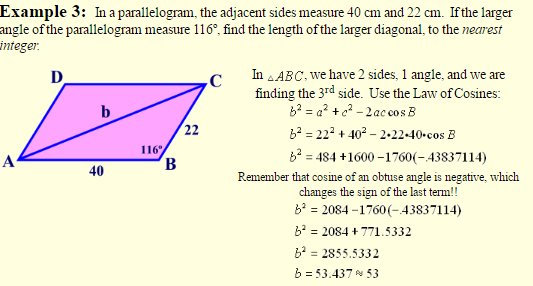
In triangle problems dealing with 2 sides and 2 angles we have seen that the Law of Sines is used to find the missing item. There are many problems, however, that deal with all three sides and only one angle of the triangle. For these problems we have another method of solution called the Law of Cosines.
With the diagram labeled at the left,
the Law of Cosines is as follows:
C2 = a2 + b2 – 2ab Cosc c
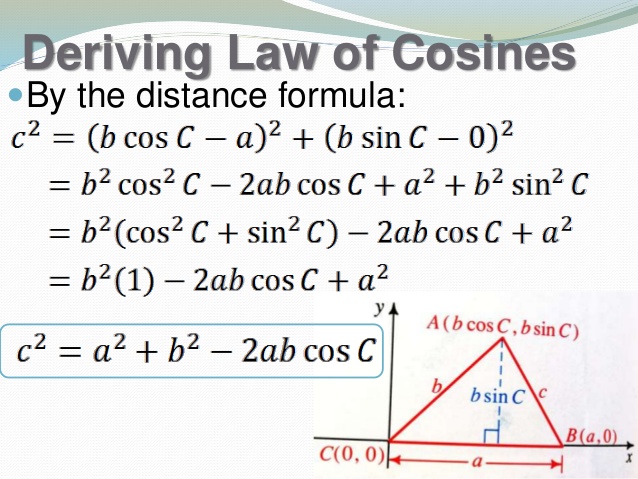Notice that <C and side c are at opposite ends of the formula. Also, notice the resemblance (in the beginning of the formula) to the Pythagorean Theorem.
Since the Law of Cosines is more involved than the Law of Sines, when you see a triangle to solve you first look to see
if you have an angle (or can find one) and a side opposite it. You can do this for ASA, AAS and SSA. In these cases you’d solve using the Law of Sines. However, if the 3 pieces of info you know don’t include an angle and side opposite it, you must use the Law of Cosines. These would be for SAS and SSS (remember you can’t solve for AAA).
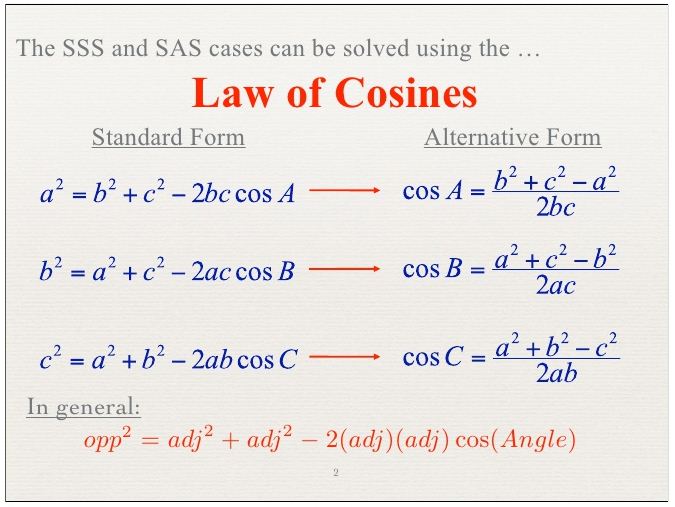We can write the Law of Cosines for each angle around the triangle. Notice in each statement how the pattern of the letters remains the same.
The Law of Cosines can be used to find a missing side for a triangle, or a missing angle. Let’s take a look .
