Kerala SSLC Maths Model Question Papers with Answers Paper 2 are part of Kerala SSLC Maths Previous Year Question Papers with Answers. Here we have given Kerala SSLC Maths Model Question Papers with Answers Paper 2.
| Board | SCERT |
| Class | SSLC Class 10 |
| Subject | Maths |
| Category | Kerala SSLC Previous Question Papers |
Kerala SSLC Maths Model Question Papers with Answers Paper 2 Free Download English Medium
Time Allowed: 2 1/2 hours
Cool off time: 15 Minutes
Maximum Marks: 80
Instructions
- Read each question carefully before writing the answer.
- Give explanations wherever necessary.
- First 15 minutes is cool-off time.
- No need to simplify irrationals like √2, √3, π etc. using approximations unless you are asked to do so.
- Simplification of irrationals like √2, π, etc with their approximate values is not required it not specified on
the question.
Answer any 3 questions from 1 to 4. Each question carries 2 scores. (Scores 3 x 2 = 6)
Question 1.
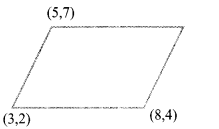
Find the coordinates of the fourth vertex of the parallelogram shown here.
Question 2.
An unbaised die is thrown. What is the probability of getting
i. a multiple of 3
ii. a number 3 or 4
Question 3.
The sum of the first n terms of an arithmetic sequence is 5n2 + 2n.
a. What is the sum of the first two terms of this sequence ?
b. Write the first two terms of the sequence.
Question 4.
Find the median of the marks obatained by 10 students of a class, which are given below. 20, 25, 17, 18, 8, 15, 22,11, 9, 14
Answer any 5 questions from 5 to 11. Each question carries 3 scores. (Scores 5 x 3 = 15)
Question 5.
Considerthe arithmetic sequence 12, 23, 34,…
a. What is the 10th term of this sequence?
b. Is 1111 a term of this sequence? Why?
Question 6.
The hypotenuse of a right triangle is 6 centimeters and one of its angles is 40°
a. Is the side opposite this angle shorter or longer than 3 centimeters? What is the reason?
b. Can we draw a triangle with one side 6 centimeters, one angle 40°and the side opposite this angle 3 centimeters? What is the reason?
Question 7.
‘ O ’ is the centre of circle, A, B, C are the points on the circle, ∠AOB = 80°, ∠BOC= 140° and AB = 7 cm.
a. The radius of the circle ?
b. Length of the chord AC ?
(sin 40°= 0.642, cos 40°= 0.766, sin 60°= 0.866, cos 60°= 0.5)
Question 8.
Two circles with centres Q and R intersect each other at points P and B. A, B, C are the points on the same straight line.
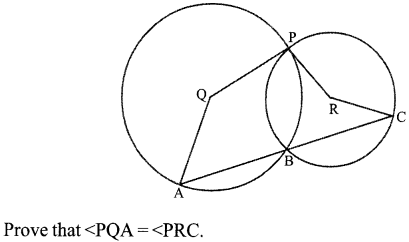
Question 9.
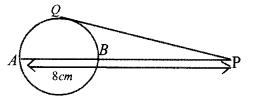
AB in the figure is the diameter and P is a point on its extension. The tangent at the point P touches the circle at Q. Find the radius of the circle?
Question 10.
A toy in the shape of a square on a square pyramid has base edge 16 cm and slant height 10 cm, 500 of these are to be painted and the cost is 80 rupees per square meter .What would be the total cost?
Question 11.
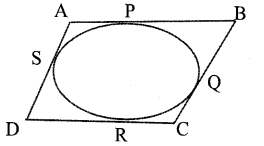
If the sides of aparallelogram touch a circle prove that the parallelogram is a rhombus.
Answer any 7 questions from 12 to 21. Each question carries 4 scores. ( Scores 7 x 4 = 28)
Question 12.
Find out the equation of the circle in the figure.
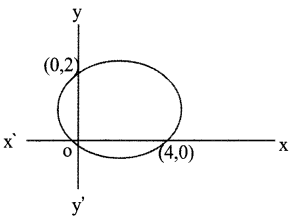
Question 13.
The triangle formed by joining the three tangents in a circle is given in the figure.
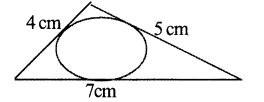
Find the length of the tangents from each vertex to the point of contact.
Question 14.
Find n in the equation 52 x 54 x 56x….x52n = (0.008)-30.
Question 15.
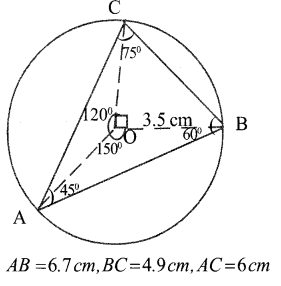
Construct a triangle with two angles 45° and 60° and its circumradius 3.5 centimetres. Measure the sides of this triangle.
Question 16.
Find the terms of an A.P whose sum of the reciprocals of the consecutive terms is 1 /6 and common difference is 5.
Question 17.
A circles is drawn with centre at (3,4) and it passes through the origin.
i. What is the radius of the circle ?
ii. What are the co-ordinates of the points at which it cuts the x-axis and the points were it cuts the y-axis?
iii. Check whether the circle passes through the point (6,8)
Question 18.
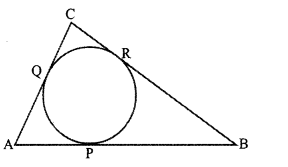
In triangle ABC, the incircle touches AB, AC and BC at points P, Q and R respectively. Calculate
i. The length of PB ?
ii. The length of sides AC and BC.
Given, AB = 8 cm, AP=3 cm and perimeter of triangle is 22 cm.
Question 19.
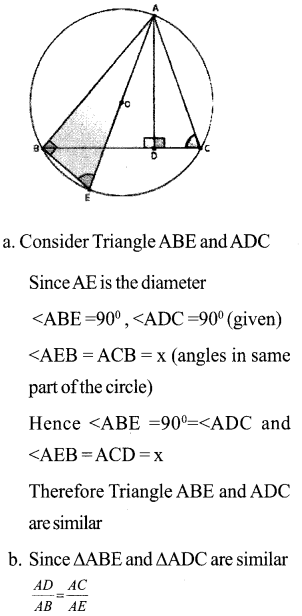
In the figure below, AD is the perpendicular from A to BC and AE is the diameter through A of the circumcircle of Δ ABC.
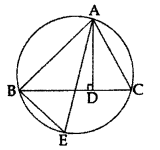
a. Prove that Δ ADC and Δ ABE are similar.
b. Prove that the area of Δ ABC is .
Question 20.
Consider the polynomial p(x) = x3 + x2 + x +1.
a. What is the remainder got on dividing if by x – 1 ?
b. What is the remainder got on dividing it by x + 1 ?
c. What first degree polynomial subtracted from p(x) gives a polynomial which is a multiple of x2 – 1?
Question 21.
The first term of an arithmetic sequence is 6 and the sum of the first 6 terms is 66.
a. What is the 6th term?
b. What is the common difference of the sequence?
c. What are the 6 terms of this sequence ?
Answer any 5 questions from 22 to 28. Each question carries 5 scores.
Question 22.
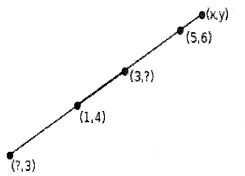
The line passing through the points with coordinates (1, 4) and (5, 6) is drawn:
a. The x-coordinate of a point on this line is 3. What is its y-coordinate?
b. The y-coordinate of a point on this line is 3. What is its x-coordinate?
c. What is the relation between the difference of the x-coordinates and the difference of the y-coordinates of any two points in this line?
d. What is the relation between the x-coordinate and the y-coordinate of any point on this line?
Question 23.
C(x, y) is the point on circle whose diameter is the line joining the points A(0, 1) and B(5, 4)
i. What is the speciality of Δ ABC.
ii. F ind the equation of the circle.
iii How to solve the equation x2 – 5x + 4 = 0 using this circle.
Question 24.
A man 1.8 metre tall standing at the top of a telephone tower, saw the top of a 10 metre high building at a depression of 40° and the base of the building at a depression
of 60°. What is the height of the tower? How far is it from the building?
Question 25.
In the figure P, Q, R and S are the points on the circle with centre at ‘ O ’.If ∠ROS=80°, P and ∠QSR=30°. Compute the following angles.
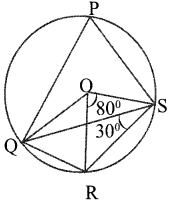
i) ∠OSQ=……………
ii) ∠SQR=………….
in) ∠P=……………..
iv) ∠QOR=………….
Question 26.
The line joining P(-4, 5) and Q(3, 2) intersects the y – axis at R. PM and QN are perpendiculars from P and Q to the x-axis.
i. Find the ratio PR :RQ
ii. Co-ordinates of R
iii. Area of the quadrilateral PMNQ
Question 27.
The following table gives the heights of plants in centimetres. If the mean height of plants is 60.95 cm, find the value of k
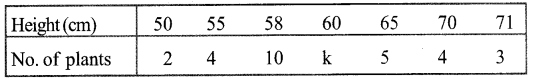
Question 28.
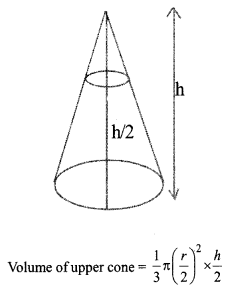
If a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, find the ratio of the volume of the upper part of the one.
Question 29.
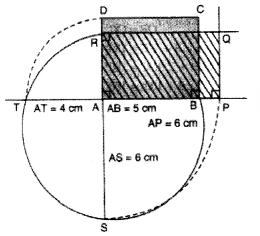
Draw a rectangle of length 5 centimeteres and width 4 centimeteres. Draw a rectangle of the same area with width 6 centimeteres.
ANSWERS
Answer 1.
If A(x1, y1), B(x2, y2), D(x3, y3) are vertices of a parallelogram ABCD, then C has the coordinates (x2+ x3 – x1, y2 + y3 – y3).
x coordinate of fourth vertex = 8 + 5 – 3 = 10
y coordinate of fourth vertex = 7 + 4 – 2 = 9
Coordinate of fourth vertex (10, 9)
Answer 2.
Total no.of outcomes, n (s) = 6
i. Let A denote an event ‘Getting a multiple of 3’
![]()

Answer 3.
a. Sn = 5n2 + 2n
The sum of the first two terms,
S2 = 5 x 22 + 2 x 2 = 20 + 4 = 24
b. The first term S1 = 5 x 12 + 2 x 1 = 7
The second term = 24 – 7 = 17
Answer 4.
Arrange the data in assenting order 8, 9, 11, 14, 15, 17, 18, 20, 22, 25
Here Median =
Answer 5.
a. For the sequence 12, 23, 34…….
x1 = 12, d = x2– x1
= 23 – 12 = 11
∴ 10th term = x10= x1 + 9d = 12 + (9 x 11)
= 12 + 99 = 111
b. Any term of the arithmetic sequence divided by common difference will have same remainder.
Here x1 = 12, d = 11 x1/d = 12/11
Quotient = 1, Remainder = 1 If we divide 1111 by the common difference 11 we get quotient = 101 and remainder = 0.
1111 is divisible by 11 we will not get the same remainder hence 1111 is not a term of this sequence.
Answer 6.
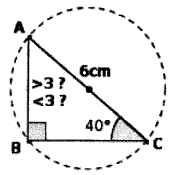
a. Circumcircle of all the right angled triangles will be the midpoint of hypotnuse.
AB/sin 40 = AC.
i.e., AB/sin 40 = 6
∴ AB = sin 40 x 6
sin 30 = 1/2. Hence s
in 30 x 6 = 1/2 x 6 = 3 .
sin 40 > sin 30 Hence
sin 40 x 6 > 3 .
i.e.,AB > 3
∴ Opposite side of the angle 40° is greater than 3 cm.
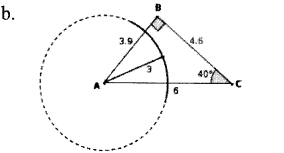
The minimum distance from B to the line drawn through A is the perpendicular distance. From the above problem we can see that when the angle is 40°, the side opposite to 40° angle is greater than 3 cm
Hence we cannot construct a triangle if the side opposite to 40° angle is 3 cm C
Answer 7.
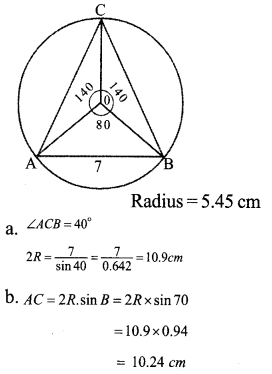
Answer 8.
Join PB
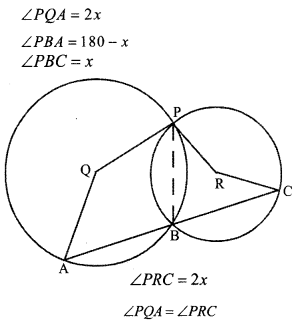
Answer 9.

Answer 10.

Answer 11.
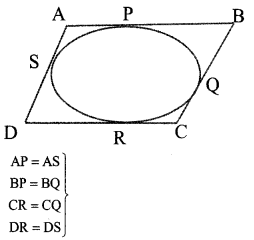

Answer 12.

Answer 13.

Length of tangents 3 cm, 4 cm, 1 cm.
Answer 14.
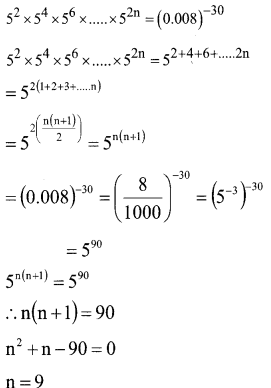
Answer 15.
Answer 16.
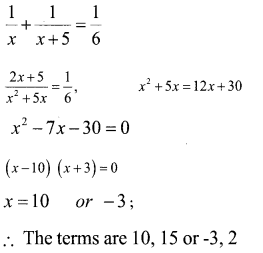
Answer 17.

Answer 18.
i PB = 5 cm
ii. CQ = s – AB = 11 – 8 = 3 cm.
AC = AQ + CQ = 3 + 3 = 6 cm
BC = CR + BR = 3 + 5 = 8 cm
Answer 19.

Answer 20.
p(x) = x3 + x2 + x + 1
a. If p (x) is divided by (x – 1), we get remainder as p(1).
p (1) =(1)3 + (1)2 + (1) + 1
= 1 + 1 + 1 + 1 = 4
∴ p (x) is divided by (x – 1), we get
remainder = 4
b. If p(x) is divided by (x + 1), we get remainder as p(-1).
p (-1) = (-1)3 + (-1)2 + (-1)+1
= -1 + 1 + – 1 + 1=0
p(x) is divided by (x + 1), we get
remainder = 0
c. Let the first degree polynomial=ax+b
Then p(x)= x3 + x2 + x + 1 -ax -b
If x + 1 is a factor of the polynomial
p(x), then p(-1) = 0 hence
(-1)3 + (-1)2 + (-1) + 1-( (ax – 1) + b)
= 0
= -1 + 1 + -1 + 1 – (-a + b) = 0
a – b = 0………….. (1)
If x -1 is a factor of the polynomial p(x), then p (1) = 0 hence
(1)3 + (1)2 + (1) + 1- ((a x 1) + b) = 0
= 1 + 1 + 1 + 1 – (a + b)
= 0 4 – (a + b) = 0
– a – b = -4………… (2)
On solving
a – b = 0……………….. (1)
– a – b = -4……………. (2)
(1) + (2) → -2b = -4
b = -4/-2
∴ b = 2
Substitute the value of b=2 in equation (1)
a – 2 = 0
∴ a = 0 + 2 = 2
Hence first degree polynomial = (ax + b) = (2x + 2)
Answer 21.
a. Here x1 = 6, S6 = 66
S6 = (x1 + x6) x 3
6/2 (first term + last term) = 66
3 (6 + 6th term) = 66
6 + 6th term = 22
6th term = 22 -6 = 16
b. 6th term = x = x, + 5d
16 = 6 + 5d
5d = 16 – 6 = 10
d = 10/5 = 2
Common difference of the sequence = 2
c. First six terms of the sequence
= 6, 8, 10, 12, 14, 16
Answer 22.
a. Slope of the line which joins the
points (1, 4) and (5, 6)
= (y2 – y1) (x2 – x1)
= (6 – 4) / (5 – 1)
= 2/4 = 1/2
If the x coordinates are increased by 1 then the y coordinates are increased by 1/2.
Let a point on the x axis be 1, then the coordinates of y is increased by A.
If the x coordinate is 3 then the y coordinate =4 + 1 = 5
b. Let x be the coordinate of a point having y coordinate 3. Slope of the line which joins the line (1,4) and (x, 3)
= (y2 – y1) (x2 – x1)
1/2 = (3 – 4) / (x – 1)
1/2 = -1 / (x – 1)
1( x – 1) = -1 x 2, x – 1 =-2
∴ x = -2 + 1 = -1
If they coordinate is 3 then the x coordinate is-1.
c. The difference in y coordinate is half the difference in x coordinates. Hence its ratio=2:1
d. Let (x,y)be a point on the line, then the slope of the line which joins the points (1, 4) and (x, y)
= (y2 – y1) / (x2 – x1)
∴ 1/2 = (y- 4) / (x-1)
1(x -1 ) = 2(y – 4)
x – 1 = 2y – 8
x – 2y = – 8 + 1 = -7
x – 2y + 7 = 0
Answer 23.
(i) ΔABC is a right angled triangle.
(ii) Equation of circle:
x2 + (y – 1)2 + (x – 5)2 + (y – 4)2 = 52 + 32
x2 + y2 – 2y+ 1 + x2– 10x
+ 25 + y2 -5y + 16 = 34
2x2 + 2y2 – 10x – 10y + 8 = 0
x2 + y2 – 5x – 5y + 4 = 0
(iii) The point that can cut the x- axis
x2 – 5x + 4 = 0
(x – 4) (x – 1) = 0
x = 1 or 4
Answer 24.
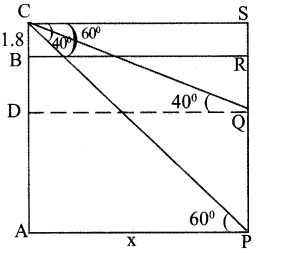
Height of the building PQ = 10 m
Height of the telephone tower is AB
Height of the man BC=1.8 m.
In Δ CQD
DC = DQ x tan 40 (DQ = AP)
= AP x tan 40 (AP = x)
= x tan 40 = 0.839 1x
In Δ CPA
AC = AP x tan 60 = x x √3 x = 1.732x
PQ = AC – CD = 0.8929x
x = = 11.19 ( PQ=10m)
AC= √3 x = 1.732 x 11.19 = 19.4
AB= 19.4-1.8= 17.6 m
Distance AP = x = 11.19m
Answer 25.
i. ∠OS0=20°
(Δ ORS is a isosceles triangle)
(OR, OS – radius of circle)
∠ORS = ∠OSR=(m-&0)/2 =100/2 =50° )
∠QSR = 30° (Given)
∠050 = 50o -∠057? = 50°- 30° = 20° )
ii. ∠SQR= 40°
( The angle made by an arc at any point on the alternate arc is equal to half the angle made at the centre,
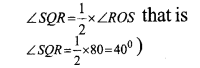
iii. ∠P=IO°
(In Δ ORS ∠QSR=30°, ∠SQR= 40° Sum of the total angle of a triangle is 180°, therefore other angle are
∠QRS = 180 – (30 + 40) = 180 – 7o = 110°
∠P= (180 -∠QRS ( = 180 – 1 10 = 70° )
iv. ∠QOR = 60°
(The central angle of an arc of a circle to be double to angle made by the arc at any point on the alternate arc)
∠QOS = 2 x ∠P= 20 x 70 = 140°)
Answer 26.
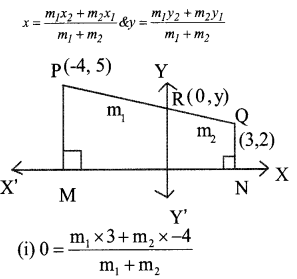
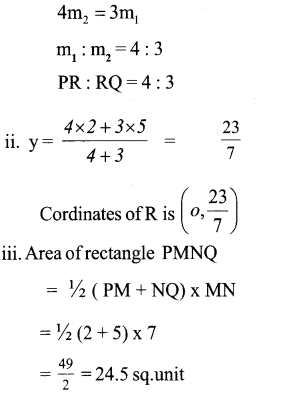
Answer 27.
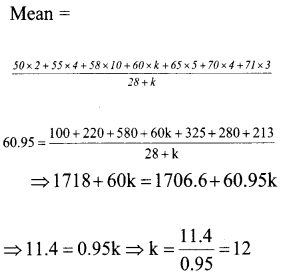
Answer 28.

Answer 29.
Draw a rectangle with given measurements.
We hope the Kerala SSLC Maths Model Question Papers with Answers Paper 2 help you. If you have any query regarding Kerala SSLC Maths Model Question Papers with Answers Paper 2, drop a comment below and we will get back to you at the earliest.
