ISC Physics Question Paper 2015 Solved for Class 12

ISC Class 12 Physics Previous Year Question Papers Solved 2015

Maximum Marks: 70
Time allowed: 3 hours

• Candidates are allowed additional 15 minutes for only reading the paper. They must NOT start writing during this time.
• Answer all questions in Portland ten questions from Part II, choosing four questions from Section A, three questions from
• Section B and three questions from Section C.
• All working, including rough work, should be done on the same sheet as, and adjacent to, the rest of the answer.
• The intended marks for questions or parts of questions are given in brackets [ ].
• Material to be supplied: Log tables including Trigonometric functions.
• A list of useful physical constants is given at the end of this paper.

Part-I
(Answer all questions)

Question 1.
A. Choose the correct alternative (a), (b), (c) or (d) for each of the questions given below :
(i) A short electric dipole (which consists of two point charges, + q and – q) is placed at the center O and inside a large cube (A B C D E F G H) of length L, as shown in figure. The electric flux, emanating through the cube is:

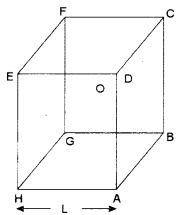
(a) \(q / 4 \pi \in_{0} \mathrm{L}\)
(b) Zero
(c) \(q / 2 \pi \in_{0} \mathrm{L}\)
(d) \(q / 3 \pi \in_{0} \mathrm{L}\)

(ii) The equivalent resistance between points a and f of the network shown in figure is :

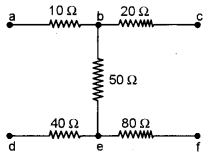
(a) 24 Ω
(b) 110 Ω
(c) 140 Ω
(d) 200 Ω

(iii) A moving electron enters a uniform and perpendicular magnetic field. Inside the magnetic field, the electron travels along :
(a) a straight line
(b) a parabola
(c) a circle
(d) a hyperbola

(iv) A fish which is at a depth of 12 cm in water (μ = 4/3) is viewed by an observer on the bank of a lake. Its apparent depth as observed by the observer is :
(a) 3 cm
(b) 9 cm
(c) 12 cm
(d) 16 cm

(v) If Ep and Ek represent potential energy and kinetic energy respectively, of an orbital electron, then, according to Bohr’s theory :
(a) E_k = – E_p/2
(b) E_k = – E_p
(c) E_k= – 2E_p
(d) E_k = 2E_p

B. Answer all questions given below briefly and to the point:
(i) What is meant by the term Quantization of charge ?
(ii) A resistor R is connected to a cell of emf e and internal resistance r. Potential difference across the resistor R is found to be V State the relation between e, V, R and r.
(iii) Three identical cells each of emf 2 V and internal resistance 1 Ω are connected in series to form a battery. The battery is then connected to a parallel combination of two identical resistors, each of resistance 6 Ω. Find the current delivered by the battery.
(iv) State how magnetic susceptibility is different for the three types of magnetic materials, i.e., diamagnetic, paramagnetic and ferromagnetic materials.
(v) An emf of 2 V is induced in a coil when current in it is changed from 0 A to 10 A in 0.40 sec. Find the coefficient of self-inductance of the coil.
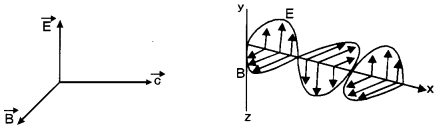
(vi) How are electric vector \(\overrightarrow{(E)}\), magnetic vector \(\overrightarrow{(B)}\) and velocity vector \(\overrightarrow{(C)}\) oriented in an electromagnetic wave ?
(vii) State any two methods by which ordinary light can be polarised.
(viii) A monochromatic ray of light falls on a regular prism. What is the relation between angle of incidence and angle of emergence in the case of minimum deviation ?
(ix) What type of lens is used to correct long-sightedness ?
(x) State any one advantage of using a reflecting telescope in place of a refracting telescope.
(xi) State Moseley’s law.
(xii) Wavelengths of the first lines of the Lyman series, Paschen series and Balmer series, in hydrogen spectrum are denoted by  λ_L,  λ_P and  λ_B, respectively. Arrange these wavelengths in increasing order.
(xiii) What is the significance of binding energy per nucleon of a nucleus of a radioactive element ?
(xiv) Write any one balanced equation representing nuclear fission.
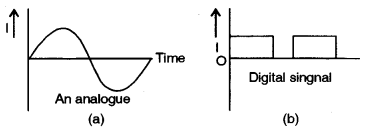
(xv) What is the difference between analogue signal and digital signal ?
Answer.
A. (i) (b)
(ii) (c)
(iii) (c)
(iv) (b)
(v) (a)
B. (i) By quantization of charge, we mean that charge is not continuous but exists in discrete magnitude. The minimum charge is e, the charge on the electron. The charge on a body is always an integral multiple of this minimum charge i.e., Q = ± Ne, where N is an integer.
(ii) The required relation is that
\(r=\frac{e-\mathrm{V}}{\mathrm{V}} \mathrm{R}\)

(iii) The connections are shown in the fig.
Total emf of the cells = 2 + 2 + 2 = 6 V
Internal resistance of the battery =1 + 1 + 1 = 3 Ω
Resistance of the parallel combination \(=\frac{6 \times 6}{6+6}=3 \Omega\)
Total resistance of the circuit = 3 + 3 = 6 Ω
Current delivered by battery = \(\frac{6 \mathrm{V}}{6 \Omega}=1 \mathrm{A}\)

(iv) The magnetic susceptibility is :

• – ve for diamagnetic substances.
• + ve but small for paramagnetic substances.
• + ve but large for ferromagnetic substances.

(v) Here, e = 2 V, dl = 10 – 0= 10A, dt=0.4s

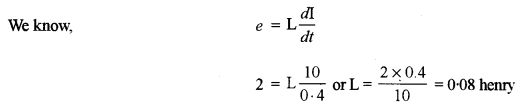
(vi) The three are mutually perpendicular as shown :

(vii) (1) Polarisation by reflection.
(2) By passing unpolarised light through a polaroid.
(viii) In the minimum deviation,
Angle of incidence = Angle of emergence.
(ix) A convex lens of suitable power or focal length.
(x) The image formed by a reflecting telescope is much brighter because there is no loss of light. Further, the image does not suffer from chromatic aberration.
(xi) According to Moseley’s law, the square root of the frequency of characteristic lines in the X-ray spectrum of an element is proportional to the atomic number.
\(\text { i.e., } \quad \sqrt{\mathrm{v}} \propto(\mathrm{Z}-\mathrm{b}), \text { where } b \text { is a constant. }\)
(xii) The correct order is  λL, λB and λp
(xiii) The stability of a nucleus depends upon the binding energy per nucleon.
(xiv) The desired reaction is
\(_{92}^{235} \mathrm{U}+\frac{1}{0} n \longrightarrow_{56}^{141} \mathrm{Ba}+_{35}^{92} \mathrm{Kr}+3_{0}^{1} n+\mathrm{Q}\)
where Q is the energy released in the process.
(xv) An analogue signal varies continues with time. They are single valued function of time. Thus, it can have different values. However, a digital signal has two discreet value 0 and 1.0 correspond to a low level and I to a high level. It is shown in fig. ‘a’ and ‘b’

Part- II
Answer ten questions in this part, choosing four questions from Section A, three questions from Section B and three questions from Section C.

Section-A
(Answer any four questions.)

Question 2.
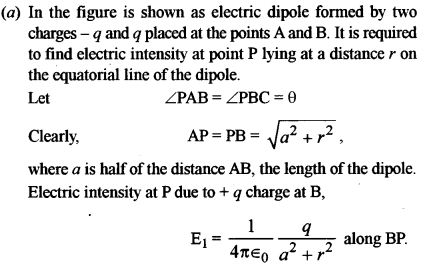
(a) Derive an expression for intensity of electric field at a point in broadside position or on an equatorial line of an electric dipole. [4]
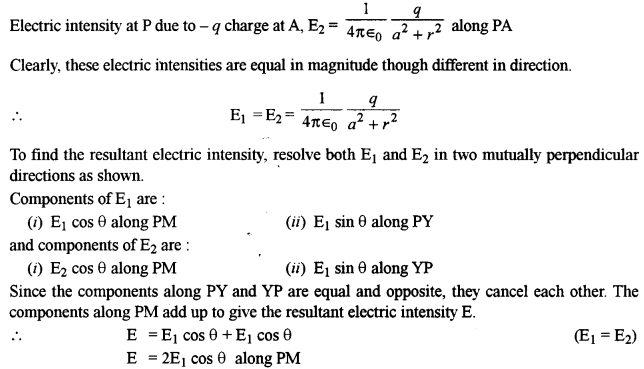
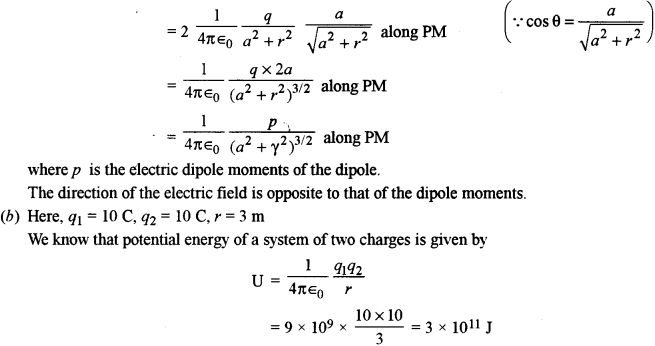
(b) Two point charges of 10 C each are kept at a distance of 3 m in vacuum. Calculate their electrostatic potential energy. [1]
Answer:
(a) In the figure is shown as electric dipole formed by two charges – q and q placed at the points A and B. It is required to find electric intensity at point P lying at a distance r on the equatorial line of the dipole.




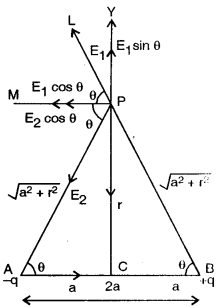
Question 3.
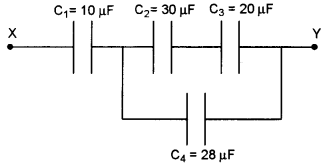
(a) Four capacitors, C₁, C₂, C₃ and C₄ are connected as shown in figure below. Calculate equivalent capacitance of the circuit between points X and Y. [3]

(b) Draw labelled graphs to show how electrical resistance varies with temperature for : [2]
(i) a metallic wire.
(ii) a piece of carbon.
Answer.
(a) The given arrangement of capacitors can be analysed as below. The combination of capacitors C₂ and C₃ of capacity 30 μF and 20 μF are in series, their combined capacity is given by
\(C^{\prime}=\frac{30 \times 20}{50}=12 \mu F\)

The capacitor C’ is in parallel with C₄, their combined capacity C” is given by
\(\mathrm{C}^{\prime \prime}=12+28=40 \mu \mathrm{F}\)
Now, C₁ and C” are in series.
∴ Net capacity of the combination is given by
\(\mathrm{C}=\frac{40 \times 10}{50}=8 \mu \mathrm{F}\)

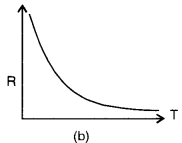
(b) The variation of electrical resistance of a metallic wire and a piece of carbon are as shown below.
For a metallic wire : The resistance of a metal increases with the rise of temperature as shown in fig. (a).

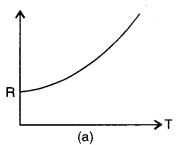
However, in the case of a semiconductor like carbon, the resistance or the resistivity decreases with the rise of temperature as shown in fig. (b).

Question 4.
(a) Two resistors R₁ = 400 Ω and R₂ = 20 Ω are connected in parallel to a battery. If heating power developed in R₁ is 25 W, find the heating power developed in R₂. [2]
(b) With the help of a labelled diagram, show that the balancing condition of a Wheatstone bridge is: [3]
\(\frac{R_{1}}{R_{2}}=\frac{R_{3}}{R_{4}}\)
where the terms have their usual meaning.
Answer.
(a) We know that heat produced per sec. in a conductor when a potential difference V is applied across it is,
\(\mathrm{P}=\frac{\mathrm{V}^{2}}{\mathrm{R}}\)
Since the two resistors are connected to a battery, potential difference across them is the same. If P1 and P2 are the heat produced per sec. in them, then

(b) In the figure is shown a labelled diagram of a balanced Wheatstone bridge, R₁, R₂, R₃, R₄ are the resistances in the four arms of the bridge. The bridge is said to be balanced, when no current flows when the key K₁ is pressed first and then the key K₂. The points B and D are at the same potential. In this case, the same current I₁ flows through AB and BC and the current I₂ flows through AD and DC.
Clearly, from the fig.,


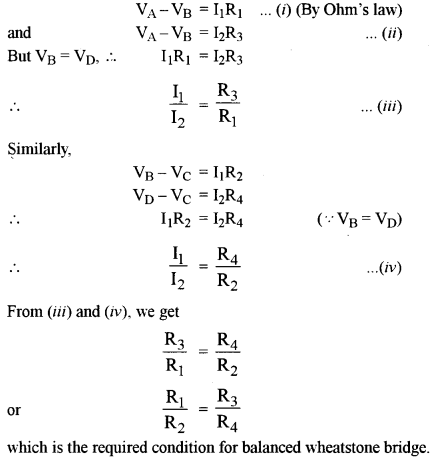
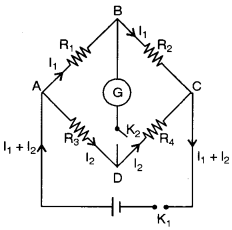
Question 5.
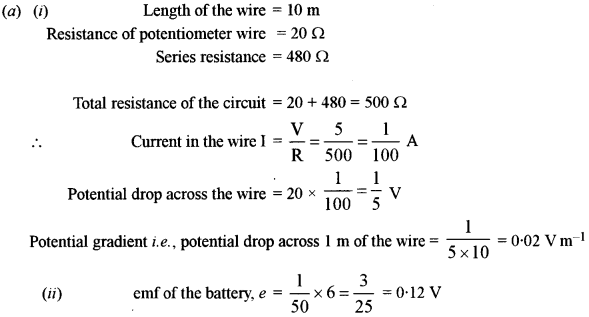
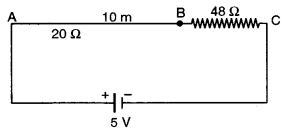
(a) A 10 m long uniform metallic wire having a resistance of 20 Ω is used as a potentiometer wire. This wire is connected in series with another resistance of 480 Ω and a battery of emf 5 V having negligible internal resistance. If an unknown emf e is balanced across 6 m of the potentiometer wire, calculate :
(i) the potential gradient across the potentiometer wire.
(ii) the value of the unknown emf e. [3]

(b) (i) Explain the term hysteresis.
(ii) Name three elements of the earth’s magnetic field which help in defining earth’s magnetic field completely. [2]
Answer:


(b) (i) Hysteresis : Hysteresis curve represents the relation between magnetic flux density \(\overrightarrow{\mathrm{B}}\) and the intensity of magnetising field B. The shape of the curve is as shown when a speciment of a ferromagnetic substance is taken through a cycle of magnetisation. The graph is not a straight line. Clearly, B ≠ 0, when H = 0.

i.e., the magnetic flux density lags behinds the magnetising field. The magnetism left behind in the speciment when H = 0 is called retentivity (OA). A reverse field has to be applied (OB) to demagnetise the speciment. This measures coercivity.

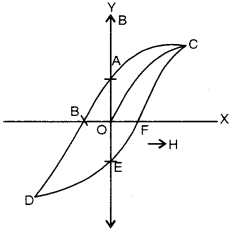
To know completely the earth’s magnetic field at a elements known as the magnetic elements at a place:
(a) Declination
(b) Dip
(c) Horizontal or the vertical component of earth’s field.

Question 6.
(a) Obtain an expression for magnetic flux density B at the center of a circular coil of radius R, having N turns and carrying a current I. [3]
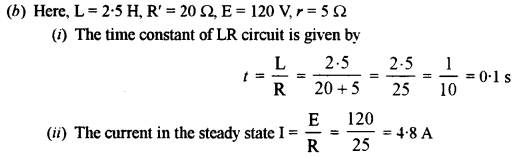
(b) A coil of self inductance 2.5 H and resistance 20 Ω is connected to a battery of emf 120 V having internal resistance of 5 Ω. Find : [2]
(i) The time constant of the circuit.
(ii) The current in the circuit in steady state.
Answer:




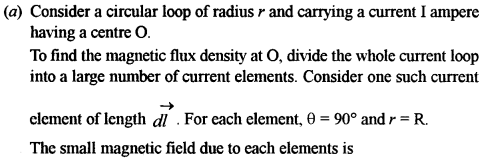
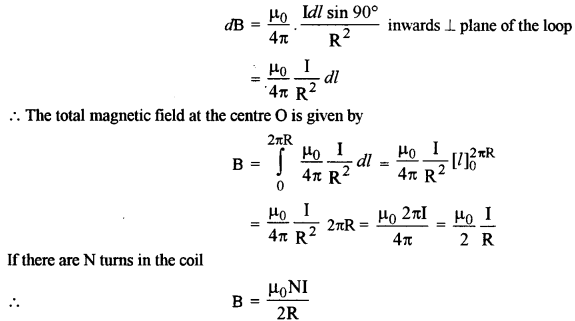
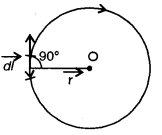
Question 7.
(a) Figure below shows a capacitor C, an inductor L and a resistor R, connected in series to an a.c. supply of 200 V. [4]
Calculate:

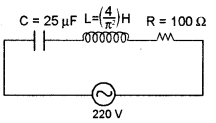
(i) The resonant frequency of the given CLR circuit.
(ii) Current flowing through the circuit.
(iii) Average power consumed by the circuit.
(b) In a series LCR circuit, what is the phase difference between V_Land V_C where V_L is the potential difference across the inductor and V_C is the potential difference across the capacitor ? [ 1 ]
Answer:

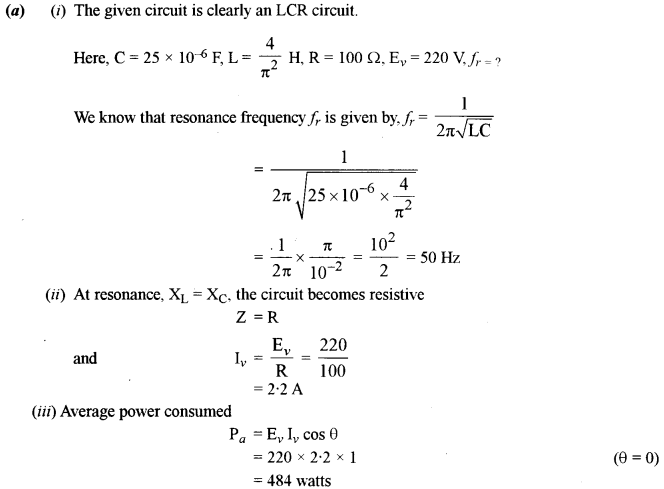
Section-B
Answer any three questions

Question 8.
(a) On the basis of Huygens’s Wave theory of light, show that angle of reflection is equal to angle of incidence. You must draw a labelled diagram for this derivation. [4]
(b) State any one difference between interference of light and diffraction of light. [1]
Answer.
(a) In the figure is shown a wave front AB obliquely incident on a reflecting surface XY at any instant of time. According to Huygens s principle, every point on the incident wave front is a source of a secondary wavelets which travel with the speed of the incident waves. The position of the reflected wave front after a time t is CGD as shown in the fig. The angle of incidence i and of reflection r are as shown.

The time taken by the disturbance to travel from B to C the same as that from A to D. Now, CGD will be a true reflected wave front if
\(\frac{\mathrm{BC}}{c}=\frac{\mathrm{EF}+\mathrm{FG}}{c}\)

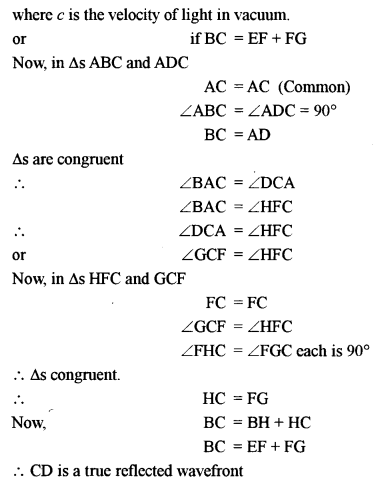
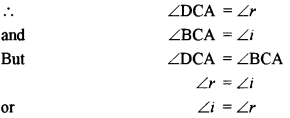
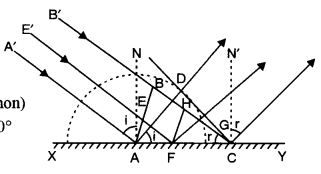
(b) Interference is due to superposition of waves starting from two coherent sources whereas diffraction is the interference between the waves from corresponding points from the two halves of the same wave front. Interference fringes are of the same width but the width of diffraction fringes is not the same.

Question 9.
(a) Laser light of wavelength 630 nm is incident on a pair of slits which are separated by 1.8 mm. If the screen is kept 80 cm away from the two slits, calculate : [3]
(i) fringe separation i.e., fringe width.
(ii) distance of 10th bright fringe from the center of the interference pattern.
(b) Show graphically the intensity distribution in Fraunhofer’s single slit diffraction experiment. Label the axes. [2]
Answer:


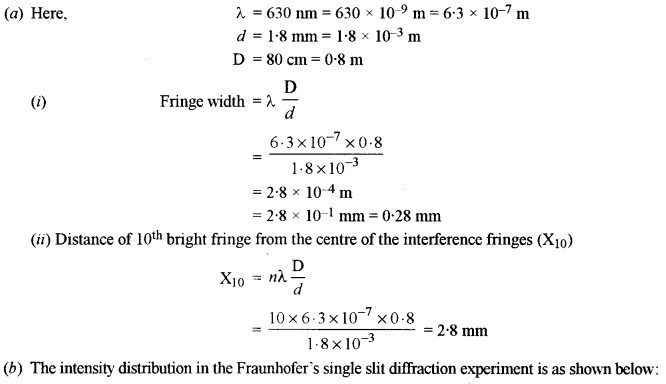
Question 10.
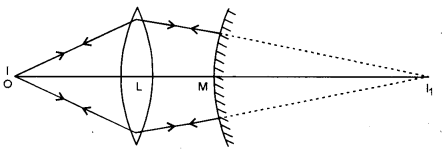
(a) A point object O is placed at a distance of 15 cm from a convex lens L of focal length 10 cm as shown in figure below. On the other side of the lens, a convex mirror M is placed such that its distance from the lens is equal to the focal length of the lens. The final image formed by this combination is observed to coincide with the object O. Find the focal length of the convex mirror. [3]

(b) What is chromatic aberration ? How can it be minimized or eliminated ? [2]
Answer.
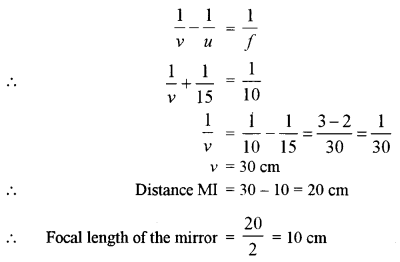
(a) Here, u = – 15 cm, f= + 10 cm
Distance between the mirror and the lens = 10 cm.
Since the image of the object is formed at O itself, it follows the rays of light suffering refraction retrace their path i.e., they strike the mirror normally. The point I₁ is thus center of curvature of the mirror.
Now; for a lens

(b) Chromatic aberration : The focal length of a lens is different for different colors, being more for the red color than for the violet color. When a ray of white light is incident on a lens, it is dispersed. As a result, different colours come to focus at different points on the principal axis. The image of a point object is not a point but is spread along the axis and is coloured. The failure of the lens to bring rays of different colors to focus at the same point is called chromatic aberration. It can be reduced by using a concave lens of suitable material and focal length.

Question 11.
(a) Draw a labelled ray diagram of an image formed by a compound microscope, when the final image lies at the least distance of distinct vision (D). [3]
(b) With regard to an astronomical telescope of refracting type, state how you will increase its : [2]
(i) magnifying power
(ii) resolving power
Answer:
(a)

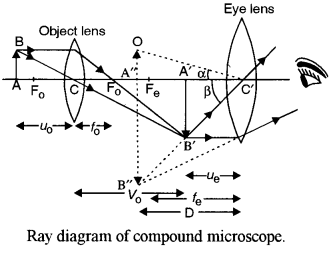
b) (i) The magnifying power of an astronomical telescope is normal adjustment is given by
\(\mathrm{M}=\frac{f_{0}}{f_{e}}\)
Thus, magnifying power can be increased by :
(a) increasing the focal length of the objective lens.
(b) decreasing the focal lengh of the eye lens.
(i) Resolving power of an astronomical telescope is given by
\(\mathrm{RP}=\frac{\mathrm{D}}{1 \cdot 22 \lambda}\)
Thus, RP can be increased by (a) aperture D of the objective should be large (b) λ, should be small

Section-C
Answer any three questions

Question 12.
(a) In an experiment of photoelectric effect, the graph of maximum kinetic energy EK of the emitted photoelectrons versus the frequency v of the incident light is a straight line AB as shown in
figure below :

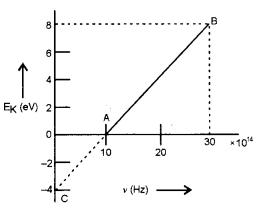
Find :
(i) Threshold frequency of the metal.
(ii) Work function of the metal.
(iii) Stopping potential for the photo electrons emitted by the light of frequency v= 30 × 10¹⁴ Hz.
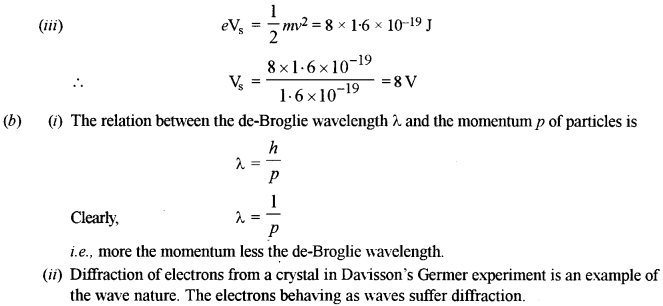
(b) (i) State how de-Broglie wavelength (λ) of moving particles varies with their linear momentum (p). [2]
(ii) State any one phenomenon in which moving particles exhibts wave nature.
Answer:

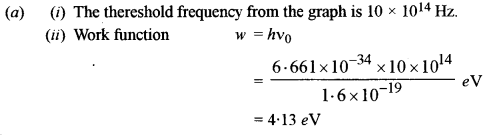
Question 13.
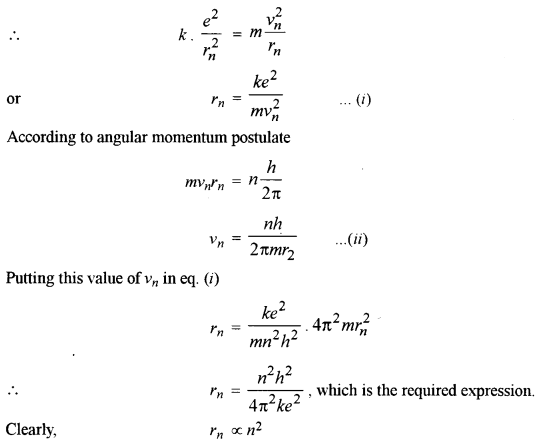
(a) On the basis of Bohr’s theory, derive an expression for the radius of the nth orbit of an electron of hydrogen atom. [3 ]
(b) Using the constants given on end of this Paper, find the minimum wavelength of the emitted X rays, when an X ray tube is operated at 50 kV [2]
Answer.
(a) Let the electrons of mass m be moving with a velocity v_n in an orbit of radius r around the nucleus of hydrogen carrying a charge + e. Since the electrostatic force between the electron and proton supplies the necessary electrostatic force.

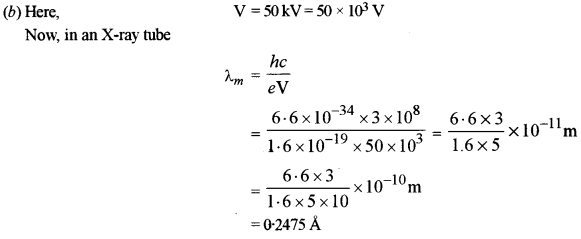
Question 14.
(а) (i) Define half-life of a radioactive substance. [3]
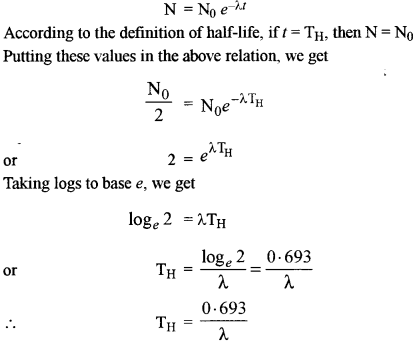
(ii) Using the equation N = N₀ e^-λt, obtain the relation between half-life (T) and decay constant (λ) of a radioactive substance.
(b) With the help of a suitable example and an equation, explain the term pair production. [2]
Answer.
(a) (i) Half-life of a radioactive substance is defined as the time in which the number of radioactive atoms is reduced to half. It is denoted by T_H.
(ii) We know that

(b) Pair production : It is the materialization of energy. The formation of an electron and a positron from a photon-a packet of energy is an example of pair production. The pair production however, takes place in the presence of an atomic nucleus. It is essential for the law of conservation of energy and momentum to be obeyed. Pair production may be represented by
\(\gamma \longrightarrow e^{-}+e^{+}\)
The difference between the energy of γ ray photon and rest mass energy of electrons appear as kinetic energy of the electron.

Question 15.
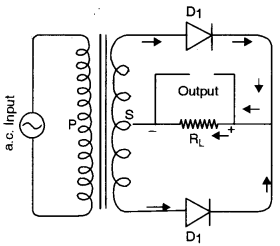
(a) Draw a labelled diagram of a full wave rectifier. Show how output voltage varies with time, if input voltage is a sinusoidal voltage. [3]
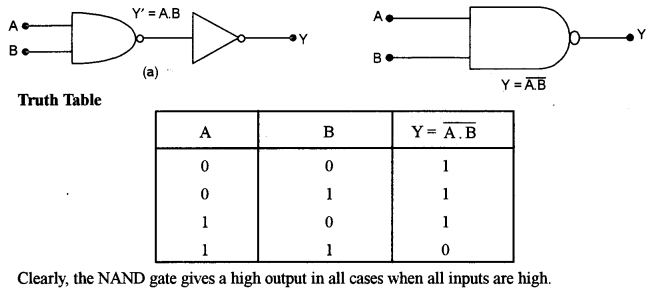
(b) What is a NAND gate ? Write its truth table. [2]
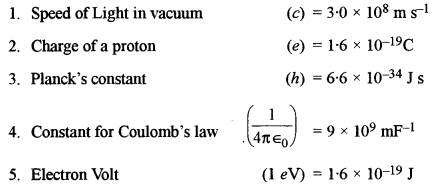
Useful Constants and Relations :

Answer.
(a) The labelled diagram of a full wave rectifier is given alongside :
D₁, D₂ are junction diodes. P and S are the primary and secondary of a transformer.
R_L = Output resistance across the output is taken. Arrows indicate the direction of current when the diodes are forward biased.

(b) NAND gate : It consists of a combination of AND gate and a NOT gate. The ‘NOT’ negates the output of a NAND gate. It is represented by \(Y=\overline{A . B}\).
The schematic representation, symbolic representation and its Truth Table are given below:

ISC Class 12 Physics Previous Year Question Papers