ISC Maths Question Paper 2016 Solved for Class 12

ISC Maths Previous Year Question Paper 2016 Solved for Class 12

Time Allowed: 3 Hours
Maximum Marks: 100

(Candidates are allowed additional 15 minutes for only reading the paper. They must NOT start writing during this time.)

• The Question Paper consists of three sections A, B and C.
• Candidates are required to attempt all questions from Section A and all questions either from Section B or Section C.
• Section A: Internal choice has been provided in three questions of four marks each and two questions of six marks each.
• Section B: Internal choice has been provided in two questions of four marks each.
• Section C: Internal choice has been provided in two questions of four marks each.
• All working, including rough work, should be done on the same sheet as, and adjacent to the rest of the answer.
• The intended marks for questions or parts of questions are given in brackets [ ].
• Mathematical tables and graph papers are provided.

Section – A (80 Marks)

Question 1. [10 × 3]
(i) Find the matrix X for which:
\(\left[\begin{array}{ll}{5} & {4} \\ {1} & {1}\end{array}\right] X=\left[\begin{array}{ll}{1} & {-2} \\ {1} & {3}\end{array}\right]\)
(ii) Solve for x, if:
\(\tan \left(\cos ^{-1} x\right)=\frac{2}{\sqrt{5}}\)
(iii) Prove that the line 2x – 3y = 9 touches the conics y² = -8x. Also, find the point of contact.
(iv) Using L’ Hospital’s rule, evaluate:
\(\lim _{x \rightarrow 0}\left(\frac{1}{x^{2}}-\frac{\cot x}{x}\right)\)
(v) Evaluate: \(\int \tan ^{3} x d x\)
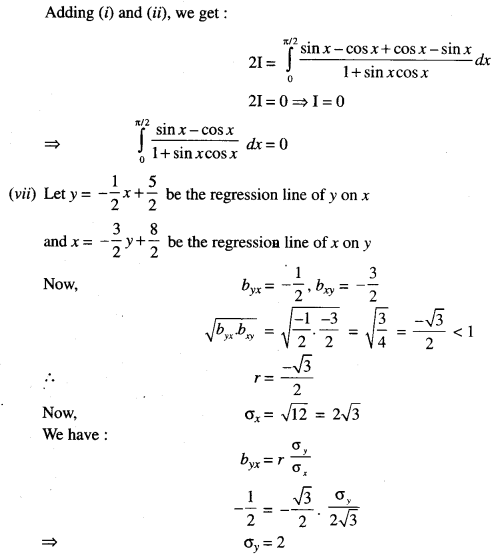
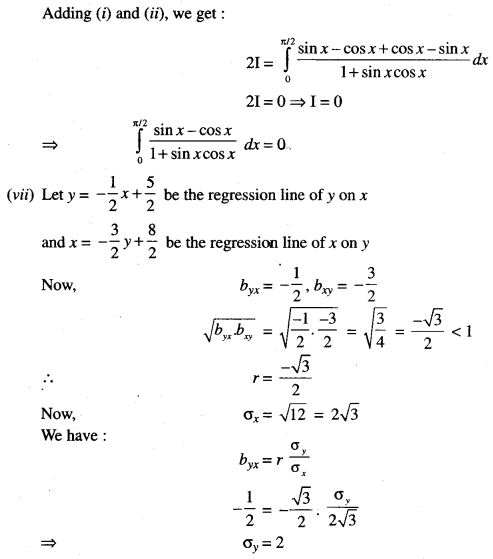
(vi) Using properties of definite integrals, evaluate:
\(\int_{0}^{\pi / 2} \frac{\sin x-\cos x}{1+\sin x \cos x} d x\)
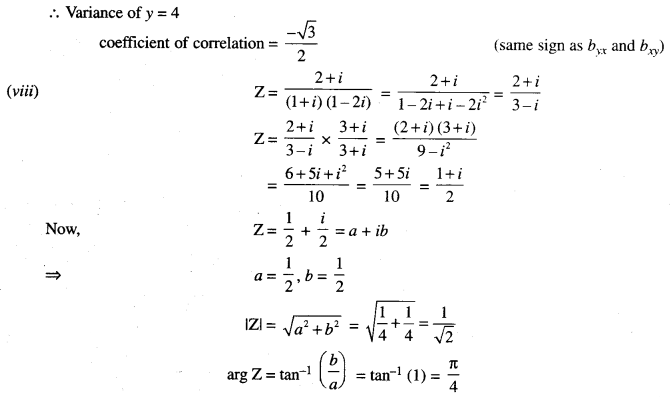
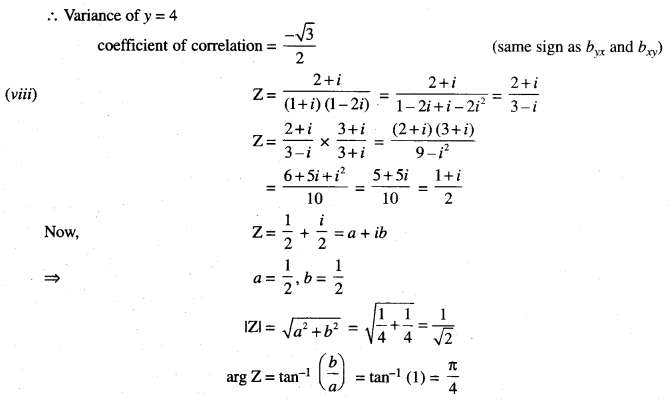
(vii) The two lines of regressions are x + 2y – 5 = 0 and 2x + 3y – 8 = 0 and the variance of x is 12. Find the variance of y and the coefficient of correlation.
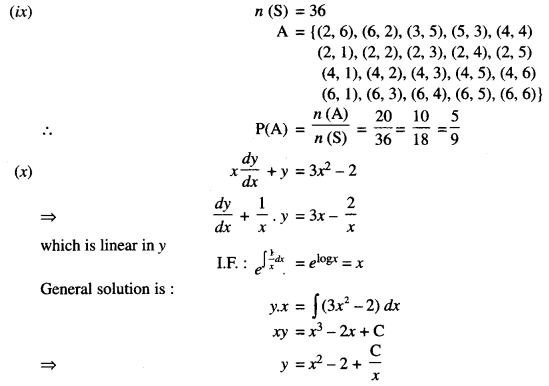
(viii) Express \(\frac{2+i}{(1+i)(1-2 i)}\) in the form of a + ib. Find its modulus and argument.
(ix) A pair of dice is thrown. What is the probability of getting an even number on the first die or a total of 8?
(x) Solve the differential equation: \(x \frac{d y}{d x}+y=3 x^{2}-2\)
Solution:

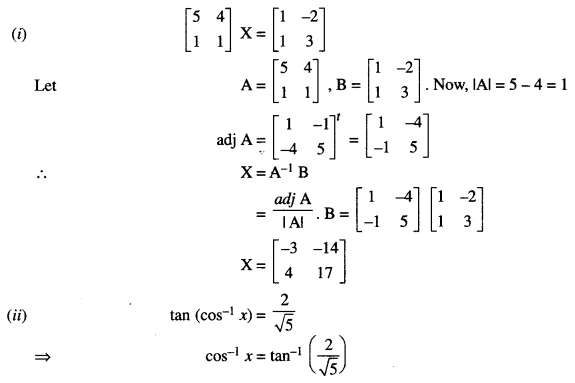
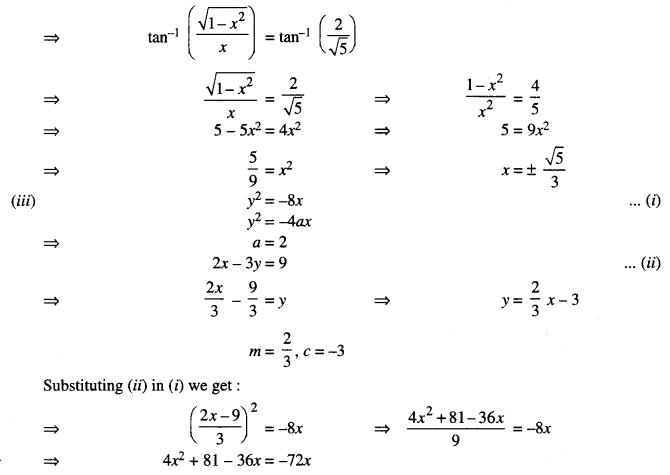
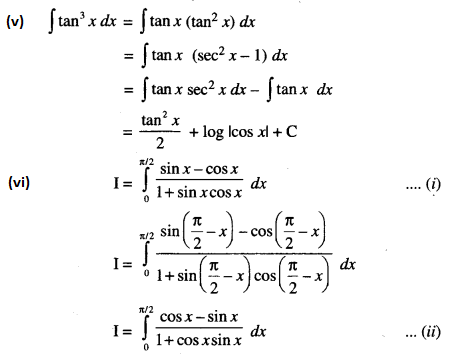
Question 2.
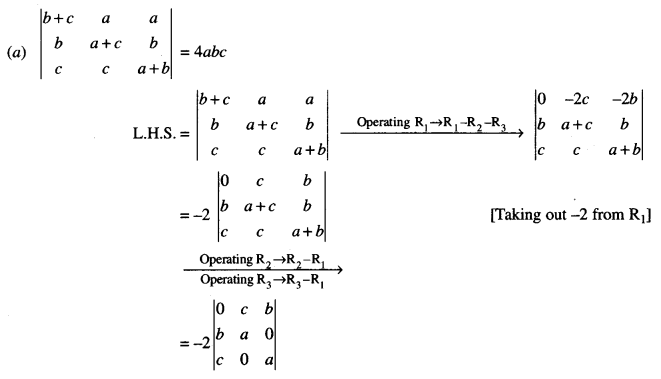
(a) Using properties of determinants, prove that: [5]
\(\left|\begin{array}{ccc}{b+c} & {a} & {a} \\ {b} & {a+c} & {b} \\ {c} & {c} & {a+b}\end{array}\right|=4 a b c\)
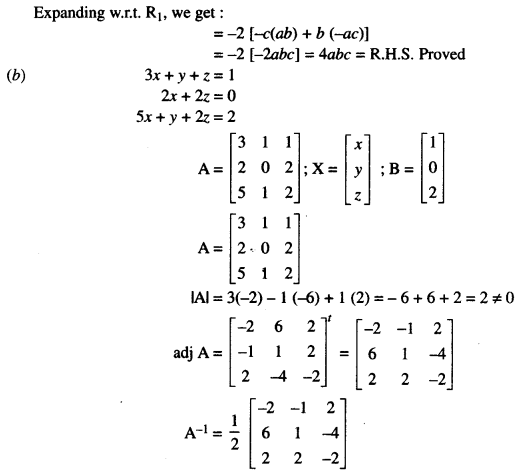
(b) Solve the following system of linear equations using matrix method: [5]
3x + y + z = 1
2x + 2z = 0
5x + y + 2z = 2
Solution:

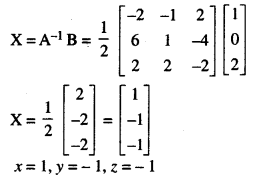
Question 3.
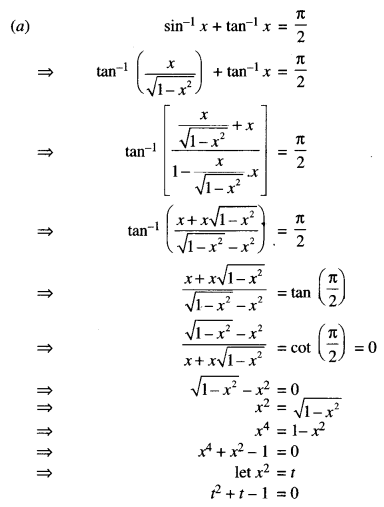
(a) If \(\sin ^{-1} x+\tan ^{-1} x=\frac{\pi}{2}\), prove that \(2 x^{2}+1=\sqrt{5}\) [5]
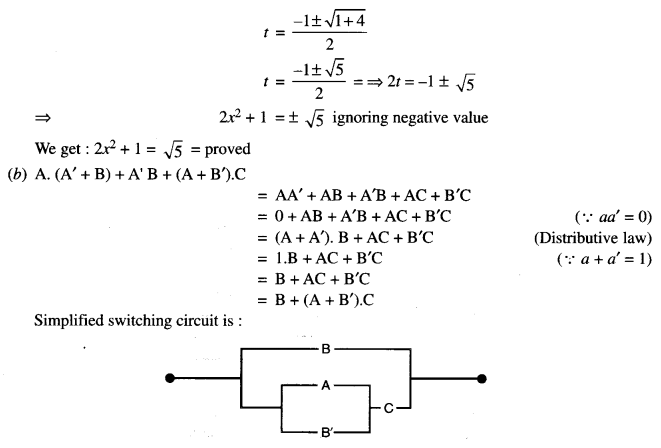
(b) Write the Boolean function corresponding to the switching circuit given below: [5]

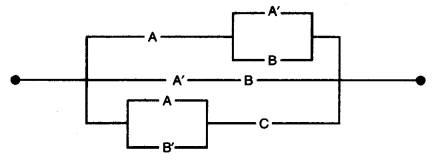
A, B and C represent switches in ‘on’ position and A’, B’ and C’ represent them in ‘off position. Using Boolean algebra, simplify the function and construct an equivalent switching circuit.
Solution:

Question 4.
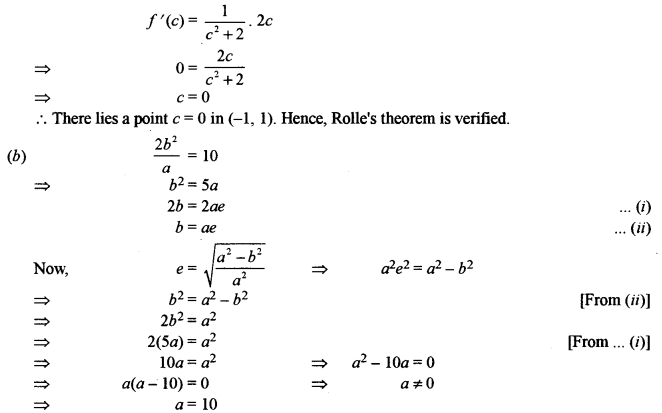
(a) Verily the conditions of Rolle’s Theorem for the following function: [5]
f(x) = log (x² + 2) – log 3 on [-1, 1]
Find a point in the interval, where the tangent to the curve is parallel to x-axis.
(b) Find the equation of the standard ellipse, taking its axes as the coordinate axes, whose minor axis is equal to the distance between the foci and whose length of latus rectum is 10. Also, find its eccentricity. [5]
Solution:
(a) f(x) = log (x² + 2) – log 3 in [-1, 1]
(i) f(x) is continuous in [-1, 1]
(ii) f(x) is derivable in (-1, 1)
(iii) f(-1) = log 3 – log 3 = 0
f(1) = log 3 – log 3 = 0
⇒ f(-1) = f(1) = o
Hence, there exists a point c in (-1, 1) such that f'(c) = 0

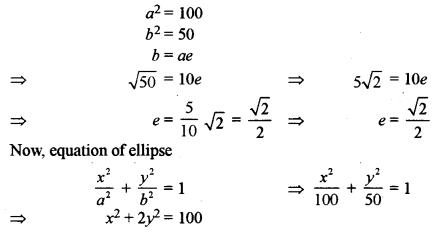
Question 5.
(a) If logy = tan^-1x, prove that: [5]
\(\left(1+x^{2}\right) \frac{d^{2} y}{d x^{2}}+(2 x-1) \frac{d y}{d x}=0\)
(b) A rectangle is inscribed in a semicircle of radius r with one of its sides on the diameter of the semicircle. Find the dimensions of the rectangle to get maximum area. Also, find the maximum area. [5]
Solution:

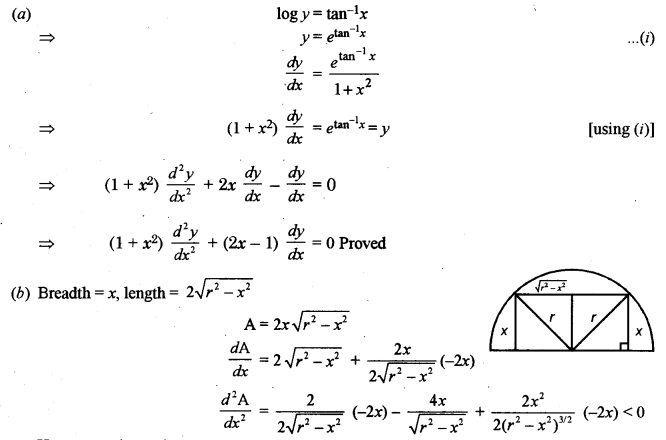
Question 6.
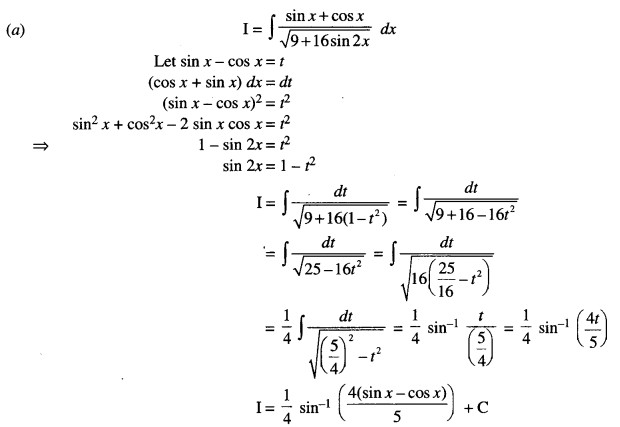
(a) Evaluate: [5]
\(\int \frac{\sin x+\cos x}{\sqrt{9+16 \sin 2 x}} d x\)
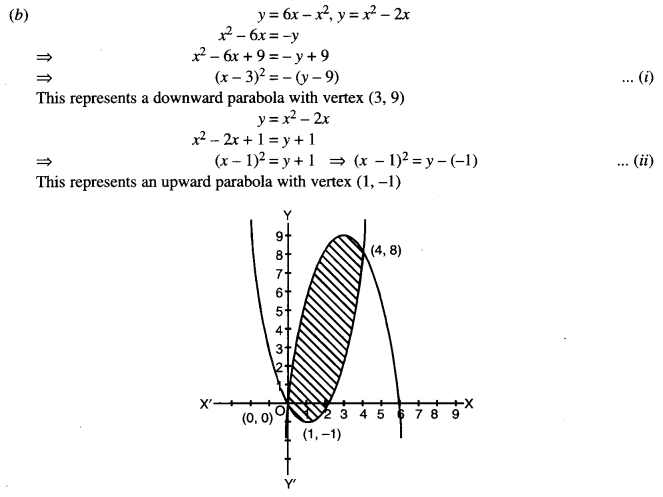
(b) Find the area of the region bound by the curves y = 6x – x² and y = x² – 2x [5]
Solution:

Question 7.
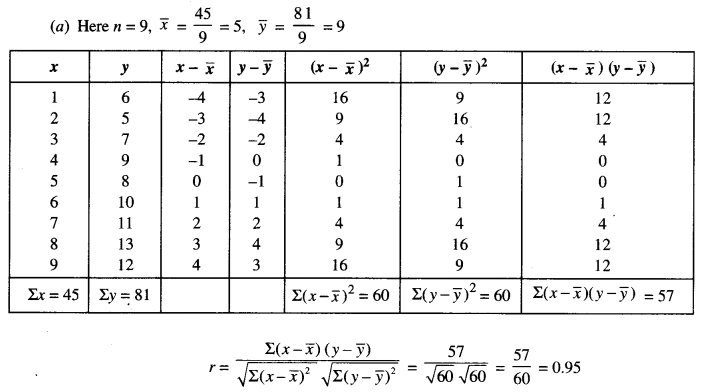
(a) Calculate Karl Pearson’s coefficient of correlation between x and y for the following data and interpret the result: [5]
(1, 6), (2, 5), (3, 7), (4, 9), (5, 8), (6, 10), (7, 11), (8, 13), (9, 12)
(b) The marks obtained by 10 candidates in English and Mathematics are given below: [5]

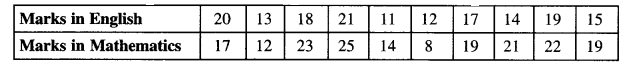
Estimate the probable score for Mathematics if the marks obtained in English are 24.
Solution:


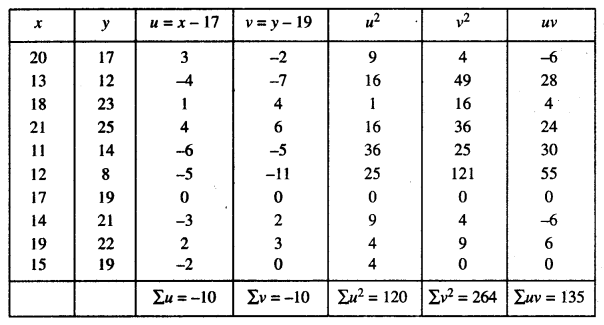
(b) Here n = 10.
Take the marks obtained in English and Mathematics as x and y respectively.
Let for x assumed mean be 17 and for v assumed mean be 19.
We construct the table as follows:

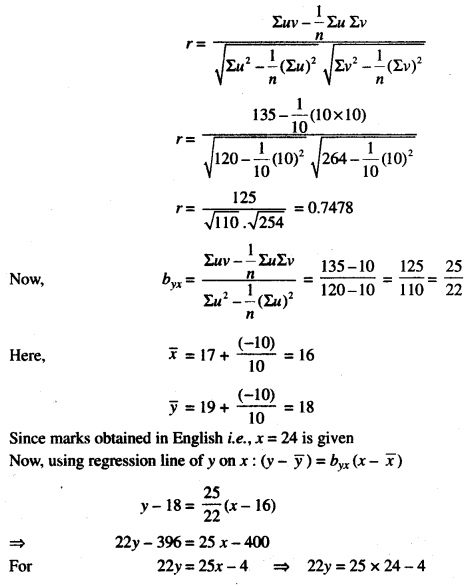
y = \(\frac { 596 }{ 22 }\) = 27.09 = 27 marks approx
Probable marks of mathematics is 27, when marks obtained in English are 24.

Question 8.
(a) A committee of 4 persons has to be chosen from 8 boys and 6 girls, consisting of at least one girl. Find the probability that the committee consists of more girls than boys. [5]
(b) An urn contains 10 white and 3 black balls while another urn contains 3 white and 5 black balls. Two balls are drawn from the first urn and put into the second urn and then a ball is drawn from the second urn. Find the probability that the ball drawn from the second urn is a white ball. [5]
Solution:

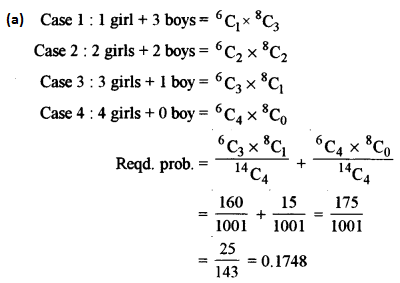
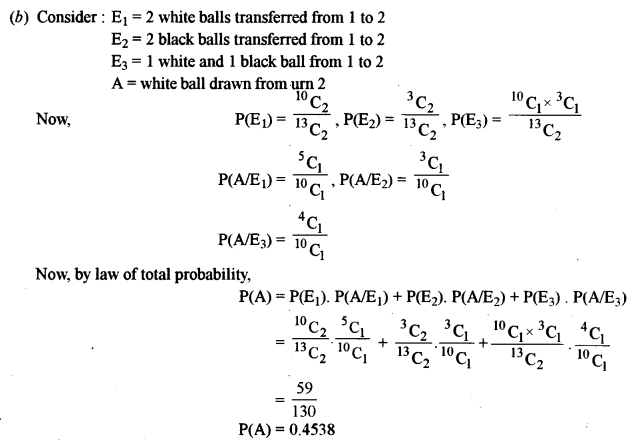
Question 9.
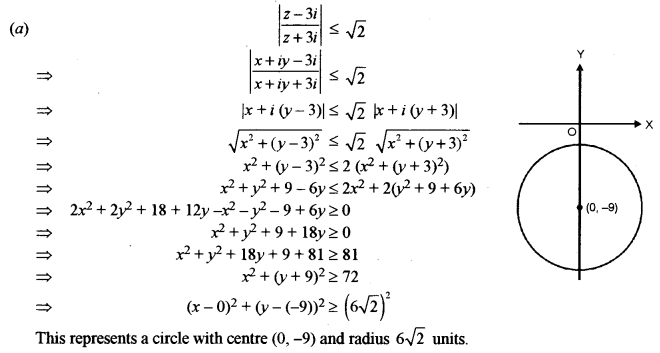
(a) Find the locus of a complex number, z = x + iy, satisfying the relation \(\left|\frac{z-3 i}{z+3 i}\right| \leq \sqrt{2}\). Illustrate the locus of z in the Argand plane. [5]
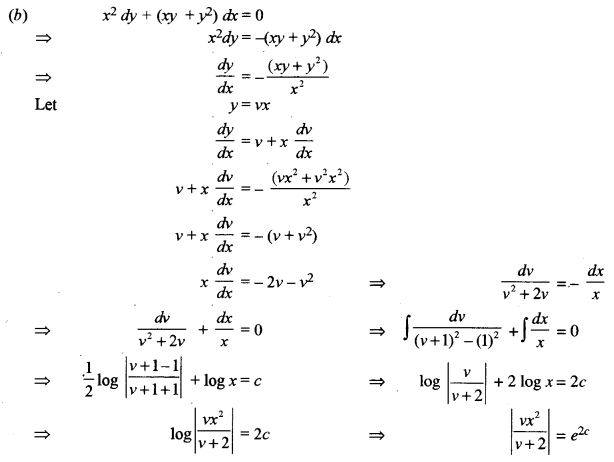
(b) Solve the following differential equation: [5]
x² dy + (xy + y²) dx = 0, when x = 1 and y = 1
Solution:

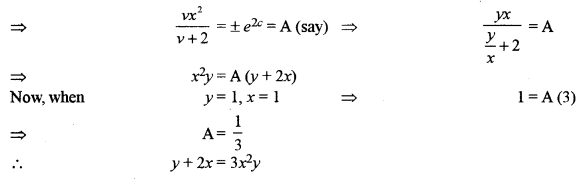
Section – B

Question 10.
(a) For any three vectors \(\vec{a}, \vec{b}, \vec{c}\), show that \(\vec{a}-\vec{b}, \vec{b}-\vec{c}, \vec{c}-\vec{a}\) are coplanar. [5]
(b) Find a unit vector perpendicular to each of the vectors \(\vec{a}+\vec{b}\) and \(\vec{a}-\vec{b}\) where \(\vec{a}=3 \hat{i}+2 \hat{j}+2 \hat{k}\) and \(\vec{b}=\hat{i}+2 \hat{j}-2 \hat{k}\) [5]
Solution:

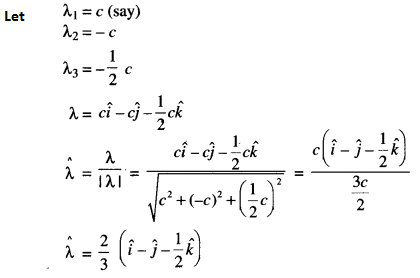
Question 11.
(a) Find the image of the point (2, -1, 5) in the line \(\frac{x-11}{10}=\frac{y+2}{-4}=\frac{z+8}{-11}\). Also, find the length of the perpendicular from the point (2, -1, 5) to the line. [5]
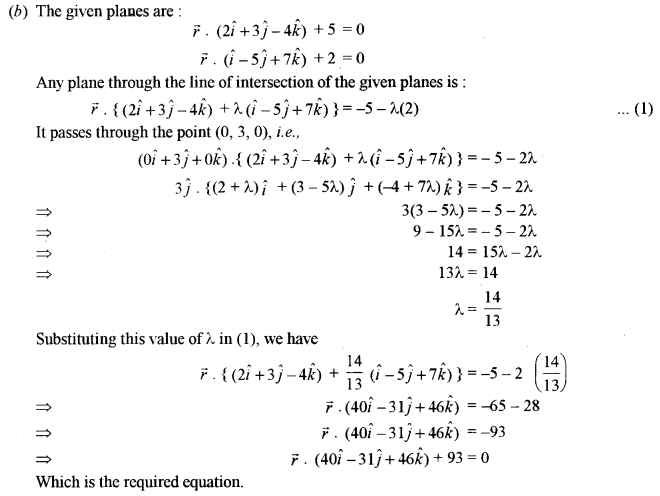
(b) Find the Cartesian equation of the plane, passing through the line of intersection of the planes \(\vec{r} \cdot(2 \hat{i}+3 \hat{j}-4 \hat{k})+5=0 \text { and } \vec{r} \cdot(\hat{i}-5 \hat{j}+7 \hat{k})+2=0\) and intersecting y-axis at (0, 3). [5]
Solution:
(a) The given line \(\frac{x-11}{10}=\frac{y+2}{-4}=\frac{z+8}{-11}\)
Let N be the foot of the perpendicular drawn from the point P(2, -1, 5).
Any point on line (1) is N (11 + 10t, -2 – 4t, -8 – 11t).
Now, direction ratio of NP is: < 9 + 10t, -1 – 4t, -13 -11t >
and direction ratio of line (1) is < 10, -4, -11 >
10(9 + 10t) + 4(1 + 4t) + 11(13 + 11t) = 0
⇒ 90 + 100t + 4 + 16t+ 143+ 121t = 0
⇒ 237t + 237 = 0
⇒ t = -1
N = (1, 2, 3)
Now, image of point P (2, -1, 5) is

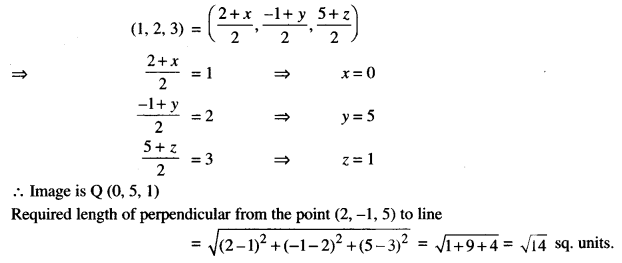
Question 12.
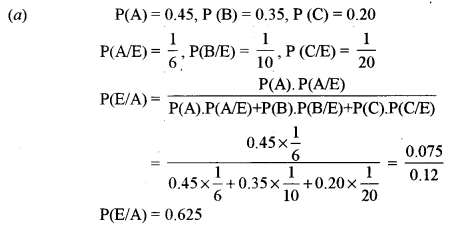
(a) In an automobile factory, certain parts are to be fixed into the chassis in a section before it moves into another section. On a given day, one of the three persons A, B and C carries out this task. A has a 45% chance, B has 35% chance and C has a 20% chance of doing the task. The probability that A, B and C will take more than the allotted time is \(\frac { 1 }{ 6 }\), \(\frac { 1 }{ 10 }\) and \(\frac { 1 }{ 20 }\) respectively.
If it is found that the time taken is more than the allotted time, what is the probability that A has done the task? [5]
(b) The difference between mean and variance of a binomial distribution is 1 and the difference of their squares is 11. Find the distribution. [5]
Solution:

Section – C

Question 13.
(a) A man borrows ₹ 20,000 at 12% per annum, compounded semi-annually and agrees to pay it in 10 equal semi-annual instalments. Find the value of each instalment, if the first payment is due at the end of two years. [5]
(b) A company manufactures two types of products A and B. Each unit of A requires 3 grams of nickel and 1 gram of chromium, while each unit of B requires 1 gram of nickel and 2 grams of chromium. The firm can produce 9 grams of nickel and 8 grams of chromium. The profit is ₹ 40 on each unit of the product of type A and ₹ 50 on each unit of type B. How many units of each type should the company manufacture so as to earn a maximum profit? Use linear programming to find the solution. [5]
Solution:



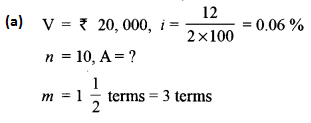
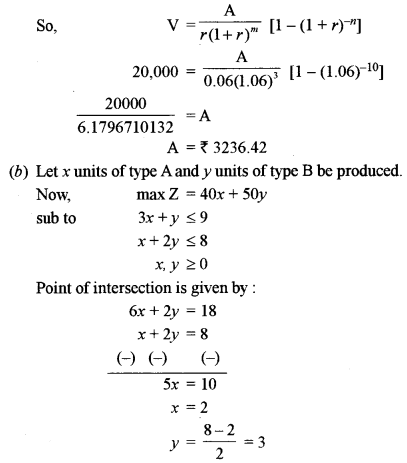
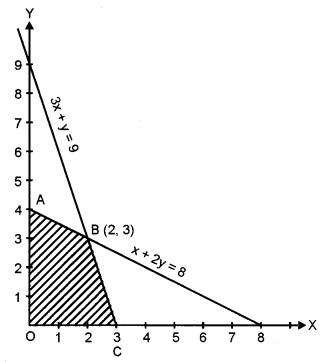
Coordinates of O is (0, 0)
Coordinates of A is (0, 4)
Coordinates of C is (3, 0)
Coordinates of B is (2, 3)
At O, Z = 0
At A, Z = 40 × 0 + 50 × 4 = ₹ 200
At B, Z = 40 × 2 + 50 × 3 = 80 + 150 = ₹ 230
At C, Z = 40 × 3 + 50 × 0 = ₹ 120
The feasible region is the shaded portion.
Maximum profit is ₹ 230 at B (2, 3) i.e., the company produces 2 units of type A product and 3 units of type B product

Question 14.
(a) The demand function is \(x=\frac{24-2 p}{3}\) where x is the number of units demanded and p is the price per unit. Find:
(i) The revenue function R in terms of p.
(ii) The price and the number of units demanded which the revenue is maximum. [5]
(b) A bill of ₹ 1,800 drawn on 10th September 2010 at 6 months was discounted for ₹ 1,782 at a bank. If the rate of interest was 5% per annum, on what date was the bill discounted? [5]
Solution:



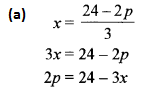
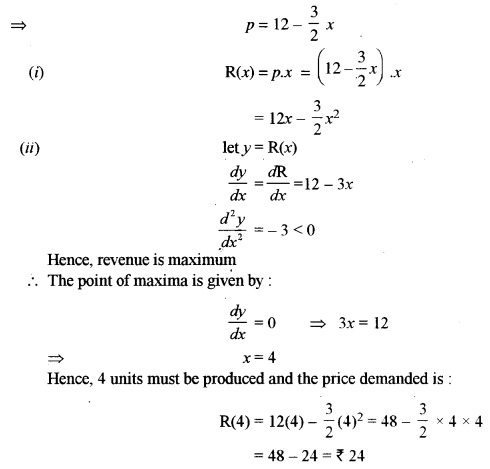
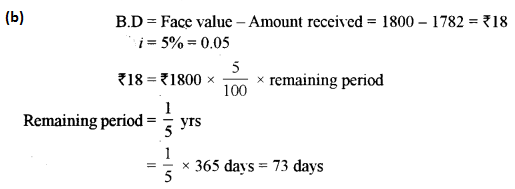
Now, the bill was drawn on 10th September 2010 for 6 months, so legally due date is 13th March 2011. But the bill was encashed 73 days before 13th March 2011. Counting 73 days backwards, 13 days of March + 28 days of February + 31 days of January + 1 day of December 2010 = 73 days
The bill was encashed on 31 – 1 i.e, 30th December 2010

Question 15.
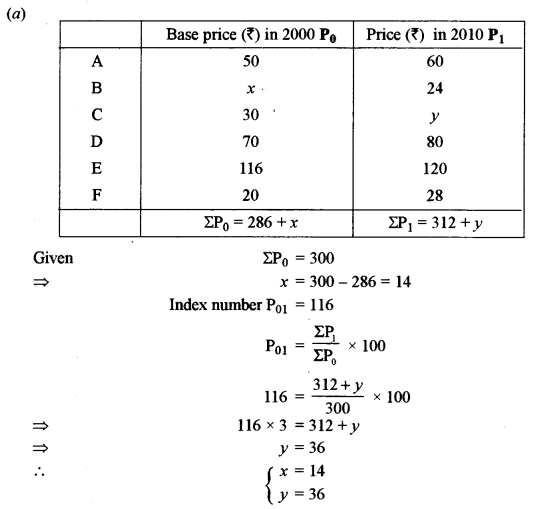
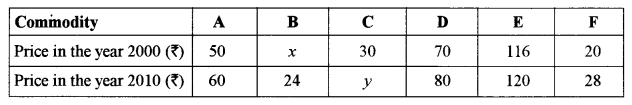
(a) The index number by the method of aggregates for the year 2010, taking 2000 as the base year, was found to be 116. If sum of the prices in the year 2000 is ₹ 300, find the values of x and y in the data given below [5]

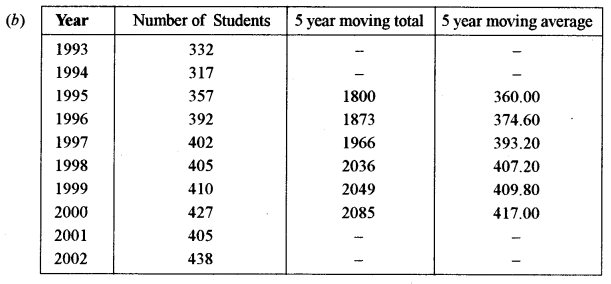
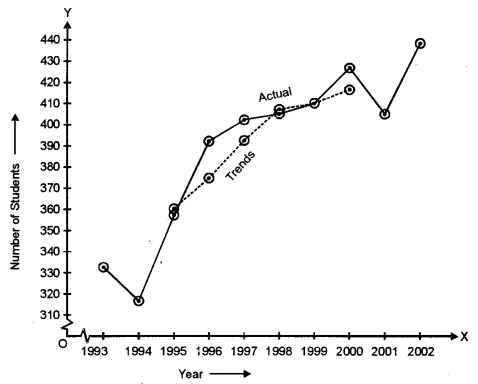
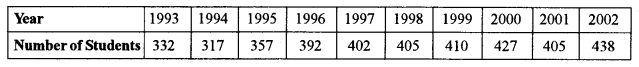
(b) From the details given below, calculate the five-year moving averages of the number of students who have studied in a school. Also, plot these and original data on the same graph paper. [5]

Solution:

ISC Class 12 Maths Previous Year Question Papers