Inverse Variation
(The Opposite of Direct Variation)
In an inverse variation, the values of the two variables change in an opposite manner – as one value increases, the other decreases.
For instance, a biker traveling at 8 mph can cover 8 miles in 1 hour. If the biker’s speed decreases to 4 mph, it will take the biker 2 hours (an increase of one hour), to cover the same distance.
Inverse variation: when one variable increases,
the other variable decreases.
As speed decreases, the time increases.
Notice the shape of the graph of inverse variation.
If the value of x is increased, then y decreases.
If x decreases, the y value increases. We say that y varies inversely as the value of x.
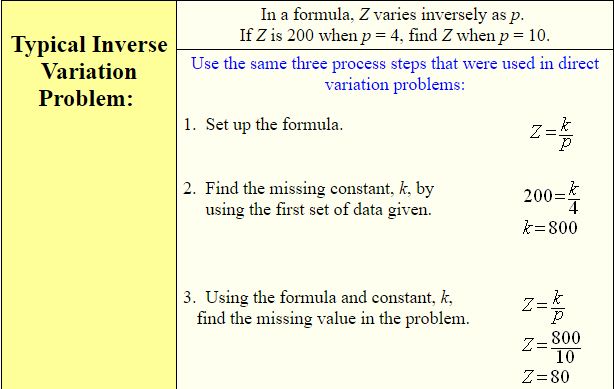
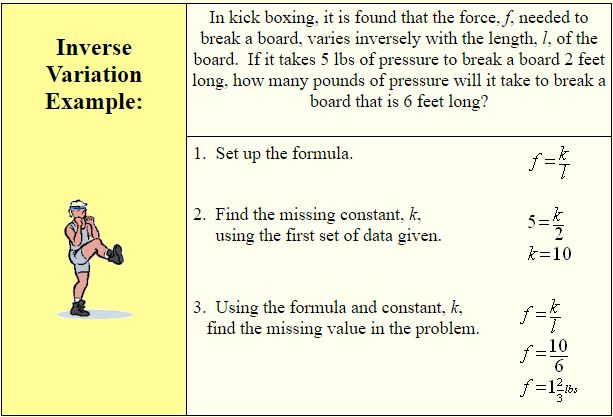
An inverse variation between 2 variables, y and x, is a relationship that is expressed as:
y=k/x
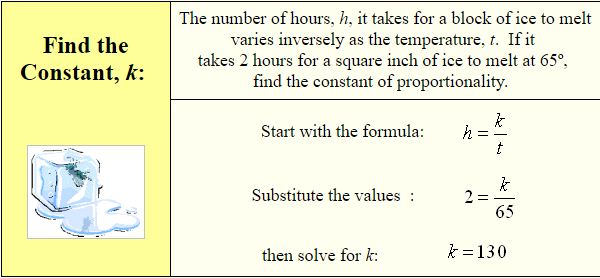
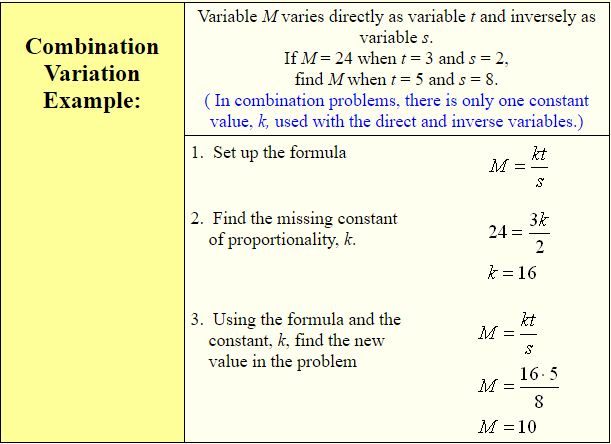
where the variable k is called the constant of proportionality.
As with the direct variation problems, the k value needs to be found using the first set of data.