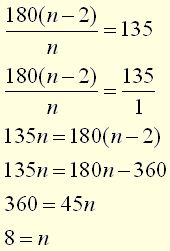Interior Angles of Regular Polygons

Interior Angles of  Regular Polygons

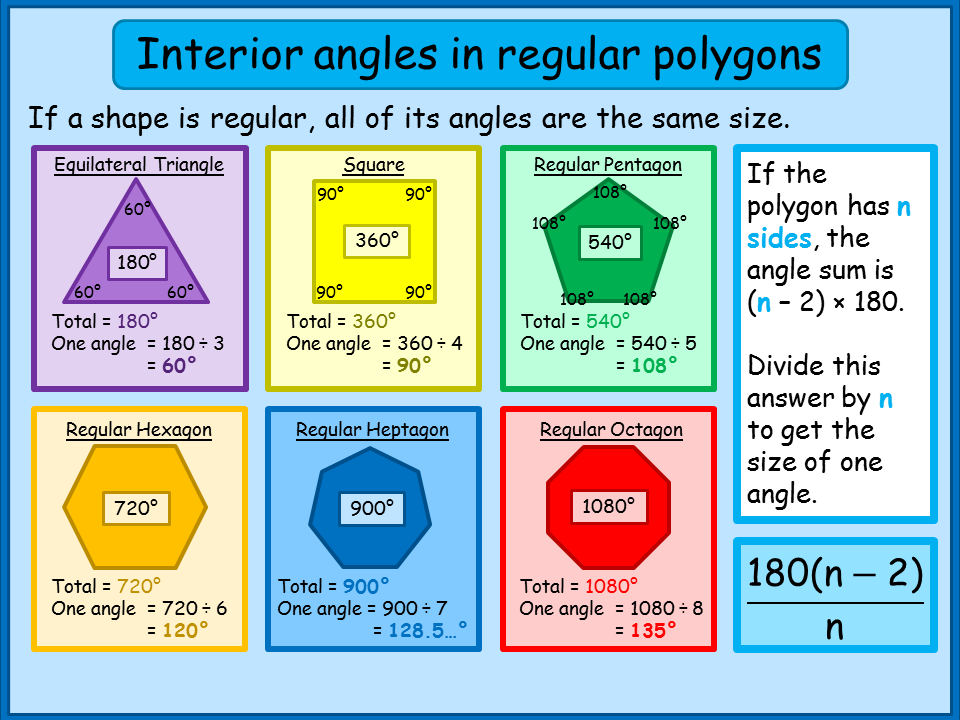
Remember that the sum of the interior angles of a polygon is given by the formula

Sum of interior angles = 180(n – 2)
where n = the number of sides in the polygon.

A polygon is called a REGULAR polygon when all of its sides are of the same length and all of its angles are of the same measure.
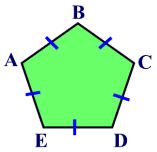A regular polygon is both equilateral and equiangular.

Let’s investigate the regular pentagon seen above.
To find the sum of its interior angles, substitute n = 5 into the formula 180(n – 2) and get 180(5 – 2) = 180(3) = 540°

Since the pentagon is a regular pentagon, the measure of each interior angle will be the same.
To find the size of each angle, divide the sum, 540º, by the number of angles in the pentagon.
(which is the same as the number of sides).
540° ÷ 5 = 108°
There are 108° in each interior angle of a regular pentagon.

This process can be generalized into a formula for finding each interior angle of a REGULAR polygon

Each interior angle of a “regular” polygon is given by
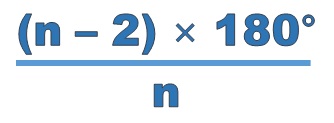where n = the number of sides in the polygon.

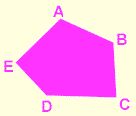
Note: If a polygon is NOT REGULAR (such as the one seen at the right), you cannot use this formula. If the angles of a polygon DO NOT all have the same measure, then you cannot find the measure of any one of them just by knowing their sum.

Examples

Read these questions carefully! If the word “EACH” appears in the question, you will most likely need the formula for “each interior angle” to solve the problem.

1. Find the number of degrees in each interior angle of a regular dodecagon.
It is a regular polygon, so we can use the formula.
In a dodecagon, n = 12.

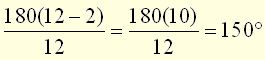
2. Each interior angle of a regular polygon measures 135°. How many sides does the polygon have ?

1. First, set the formula (for each interior angle) equal to the number of degrees given.
2. Cross multiply.
3. Multiply 180 by (n – 2).
4. Subtract 135n from both sides of the equation.
5. Divide both sides of the equation by 45.