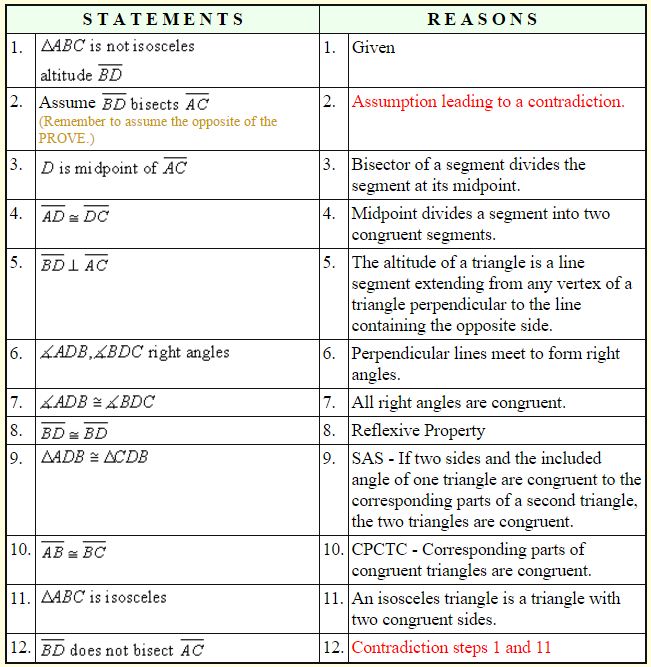
Indirect Proof (Proof by Contradiction)
When trying to prove a statement is true, it may be beneficial to ask yourself, “What if this statement was not true?” and examine what happens. This is the premise of the Indirect Proof or Proof by Contradiction.
Indirect Proof:
Assume what you need to prove is false, and then show that something contradictory (absurd) happens.
Steps in an Indirect Proof:
- Assume that the opposite of what you are trying to prove is true.
- From this assumption, see what conclusions can be drawn. These conclusions must be based upon the assumption and the use of valid statements.
- Search for a conclusion that you know is false because it contradicts given or known information. Oftentimes you will be contradicting a piece of GIVEN information.
- Since your assumption leads to a false conclusion, the assumption must be false.
- If the assumption (which is the opposite of what you are trying to prove) is false, then you will know that what you are trying to prove must be true.
How to Recognize When an Indirect Proof is Needed:
Generally, the word “not” or the presence of a “not symbol” (such as the not equal sign ≠) in a problem indicates a need for an Indirect Proof.
Proof by Contradiction is also known as reductio ad absurdum (which from Latin means reduced to an absurdity).
Example:
(done in a two-column format)
In the accompanying diagram, ∆ABC is not isosceles.
Prove that if altitude \(\overline { BD }\) is drawn, it will not bisect \(\overline { AC }\).
In this example, we must first clearly indicate the GIVEN and the PROVE.
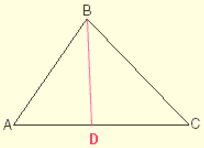Given: ∆ABC is not isosceles, altitude \(\overline { BD }\)
Prove: \(\overline { BD }\) does not bisect \(\overline { AC }\)
