ICSE Maths Previous Year Question Paper 2012 Solved for Class 10
ICSE Paper 2012
MATHEMATICS
(Two hours and a half)
Answers to this Paper must be written on the paper provided separately.
You will not be allowed to write during the first 15 minutes.
This time is to be spent in reading the question paper.
The time given at the head of this Paper is the time allowed for writing the answers.
Attempt all questions from Section A and any four questions from Section B.
All working, including rough work, must be clearly shown and must be done on the same sheet as the rest of the answer. Omission of essential working will result in the loss of marks.
The intended marks for questions or parts of questions are given in brackets [ ].
Mathematical tables are provided.
SECTION-A (40 Marks)
(Attempt all questions from this Section)
Question 1:
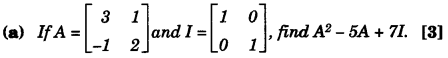
(b) The monthly pocket money of Ravi and Sanjeev are in the ratio 5 : 7. Their expenditures are in the ratio 3 : 5. If each saves Rs. 80 every month, find their monthly pocket money. [3]
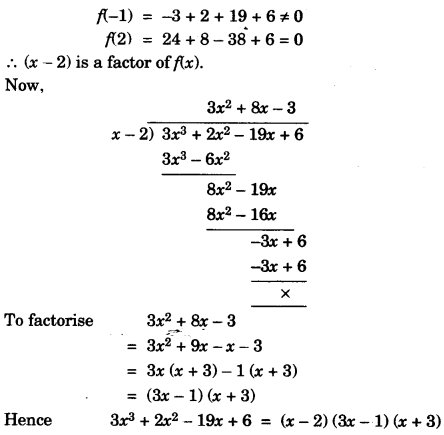
(c) Using the Remainder Theorem factorise completely the following polynomial:
3x3 + 2x2 – 19x + 6
Solution:
(b)
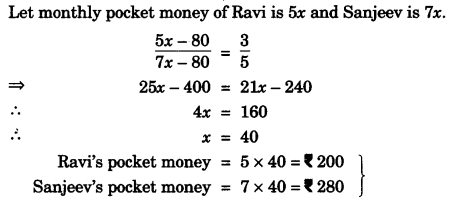
(c)
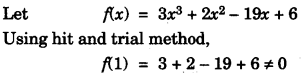
Question 2:
(a) On what sum of money will the difference between the compound interest and simple interest for 2 years be equal to Rs. 25 if the rate of interest charged for both is 5% p.a.? [3]
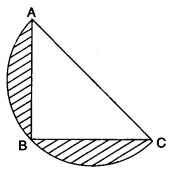
(b) ABC is an isosceles right angled triangle with ∠ABC = 90°. A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region. (Take n = 22/7) [3]
(c) Given a line segment AB joining the points A (-4, 6) and B (8, -3). Find:
(i) the ratio in which AB is divided by the y-axis.
(ii) find the coordinates of the point of intersection.
(iii) the length of AB.
Solution:
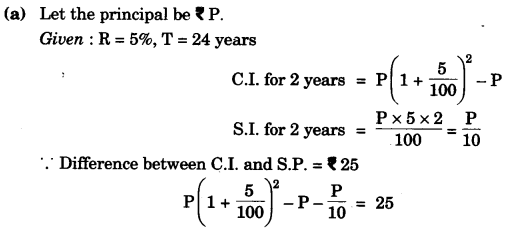

(b)
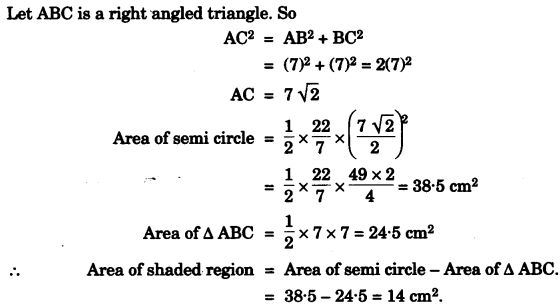
(c)
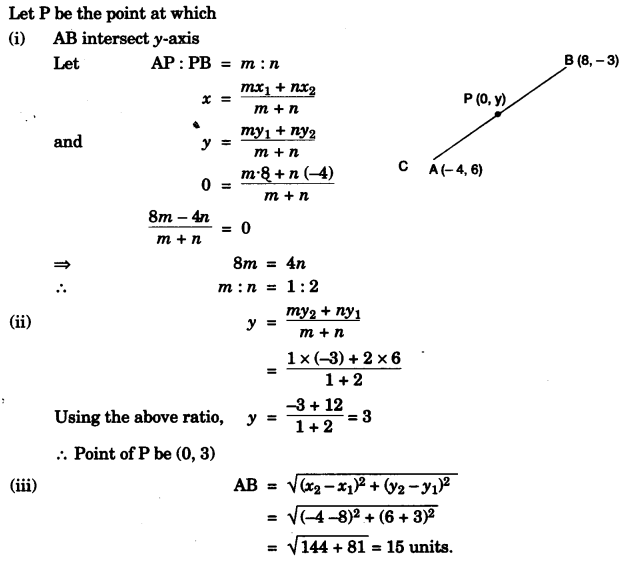
Question 3:
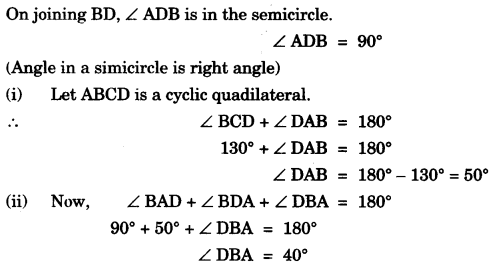
(a) In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of the circle. [3]
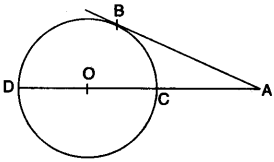
(b) Evaluate without using trigonometric tables: [3]
![]()
(c) Marks obtained by 40 students in a short assessment is given below, where a and b are two missing data.
| Marks | 5 | 6 | 7 | 8 | 9 |
| No. of students | 6 | a | 16 | 13 | 6 |
If the mean of the distribution is 7.2, find a and b. [4]
Solution:
(a)
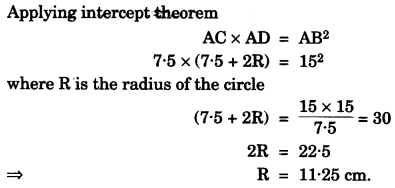
(b)
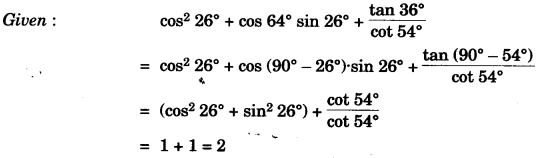
(c)
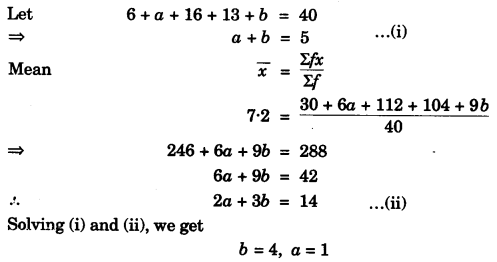
Question 4:
(a) Kiran deposited Rs. 200 per month for 36 months in a bank’s recurring deposit account. If the bank pays interest at the rate of 11% per annum, find the amount she gets on maturity. [3]
(b) Two coins are tossed once. Find the probability of getting:
(i) 2 heads
(ii) at least 1 tail. [3]
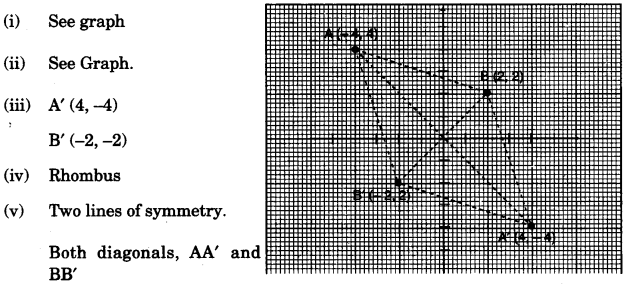
(c) Using graph paper and taking 1 cm = 1 unit along both x-axis and y-axis.
(i) Plot the points A(-4, 4) and B (2, 2)
(ii) Reflect A and B in the origin to get the images A’ and B’ respectively.
(iii) Write down the co-ordinates of A’ and B’.
(iv) Give the geometrical name for the figure ABA’B’.
(v) Draw and name its lines of symmetry. [4]
Solution:
(a)
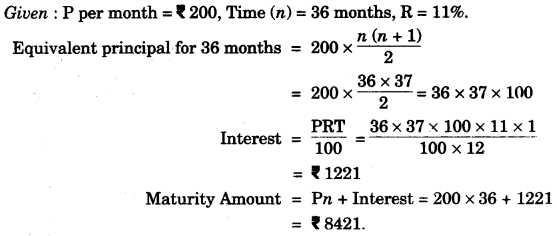
(b)
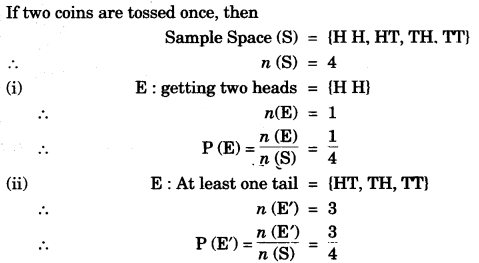
(c)
SECTION-B (40 Marks)
(Attempt any four questions from this Section)
Question 5:
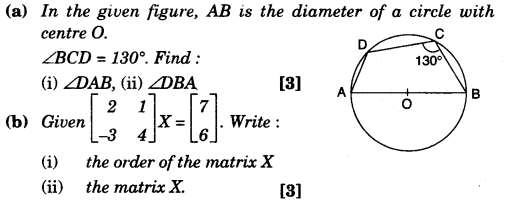
(c) A page from the Savings Bank Account of Mr. Prateek is given below:
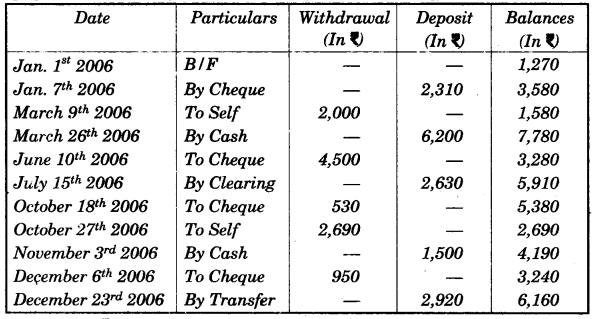
If he receives ₹ 198 as interest on 1st January, 2007, find the rate of interest paid by the bank. [4]
Solution:
(a)
(b)
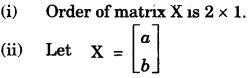
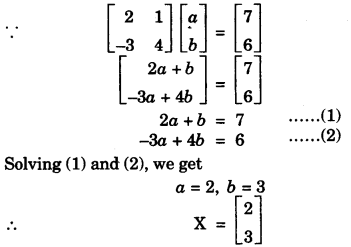
(c)
| Months | Minimum Balance |
| January | 3,580 |
| February | 3,580 |
| March | 1,580 |
| April | 7,780 |
| May | 7,780 |
| June | 3,280 |
| July | 3,280 |
| August | 5,910 |
| September | 5,910 |
| October | 2,690 |
| November | 4,190 |
| December | 3,240 |
| Total | Rs. 52,800 |
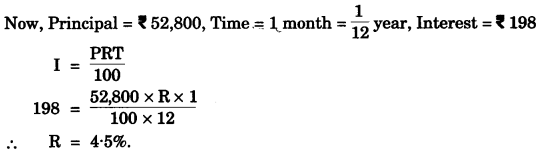
Question 6:
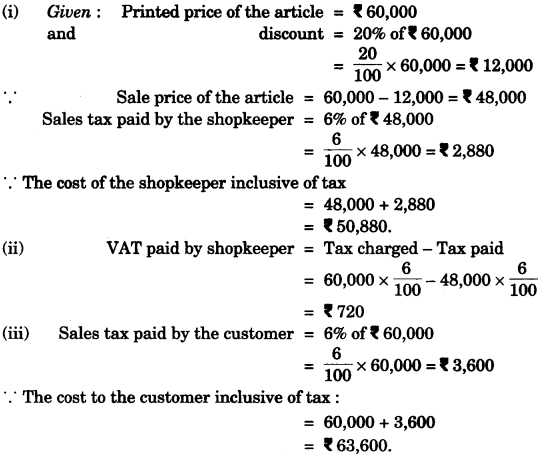
(a) The printed price of an article is ₹ 60,000. The wholesaler allows a discount of 20% to the shopkeeper. The shopkeeper sells the article to the customer at the printed price. Sales tax (under VAT) is charged at the rate of 6% at every stage. Find:
(i) the cost to the shopkeeper inclusive of tax.
(ii) VAT paid by the shopkeeper to the Government.
(iii) the cost to the customer inclusive of tax. [3]
(b) Solve the following inequation and represent the solution set on the number line:
![]()
(c) Without solving the following quadratic equation, find the value of ‘m’ for which the given equation has real and equal roots.
x2 + 2 (m – 1) x + (m + 5) = 0 [3]
Solution:
(a)
(b)
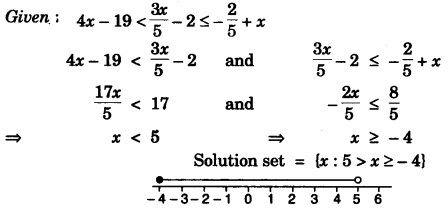
(c)
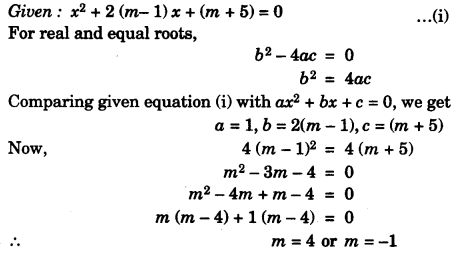
Question 7:
(a) A hollow sphere of internal and external radii 6 cm and 8 cm respectively is melted and recast into small cones of base radius 2 cm and height 8 cm. Find the number of cones. [3]
(b) Solve the following equation and give your answer correct to 3 significant figures:
5x2 – 3x – 4 = 0 [3]
(c) As observed from the top of a 80 m tall lighthouse, the angles of depression of two ships on the same side of the light house in horizontal line with its base are 30° and 40° respectively. Find the distance between the two ships. Give your answer correct to the nearest metre. [4]
Solution:
(a)
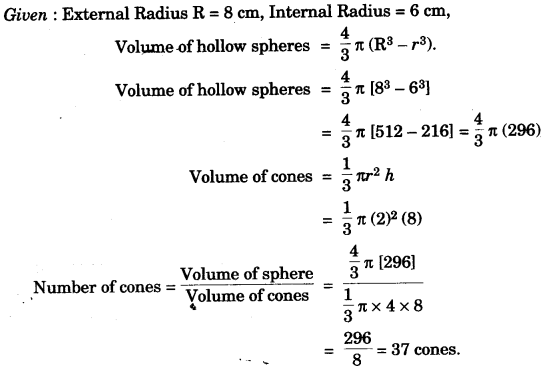
(b)

(c)
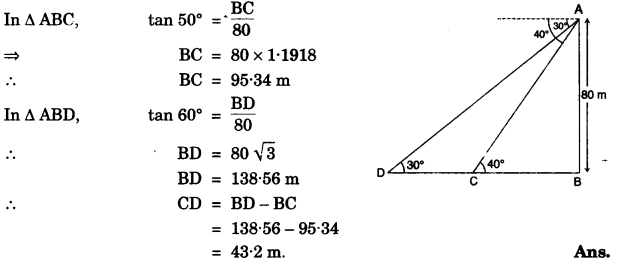
Question 8.
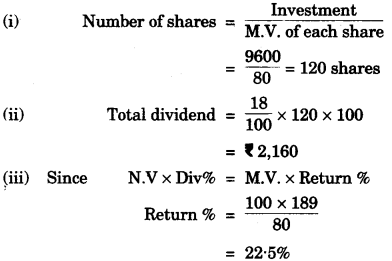
(a) A man invests Rs. 9,600 on Rs. 100 shares at Rs. 80. If the company pays him 18% dividend find:
(i) the number of shares he buys.
(ii) his total dividend.
(iii) his percentage return on the shares.

Solution:
(a)
(b)
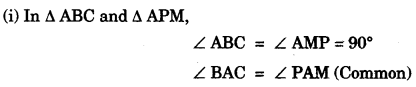
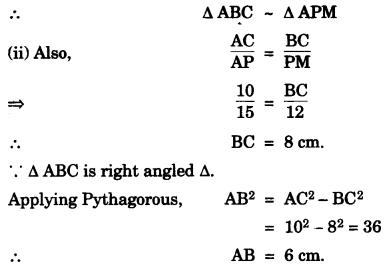
(c)
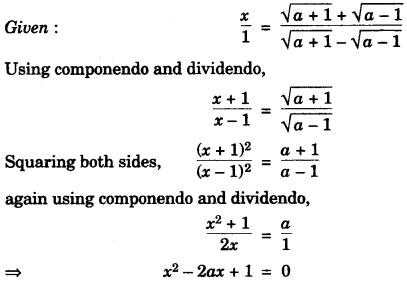
Question 9:
(a) The line through A (-2, 3) and B (4, b) is perpendicular to the line 2x – 4y = 5. Find the value of b. [3]
![]()
(c) A car covers a distance of 400 km at a certain speed. Had the speed been 12 km/h more, the time taken for the journey would have been 1 hour 40 minutes less. Find the original speed of the car. [4]
Solution:
(a)
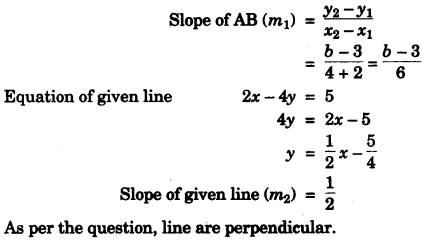
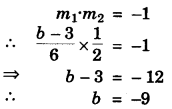
(b)
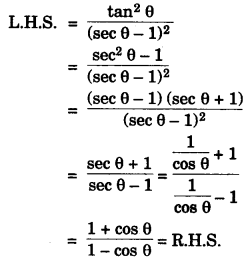
(c)

Question 10:
(a) Construct a triangle ABC in which base BC = 6 cm, AB = 5.5 cm and ∠ABC = 120°.
(i) Construct a circle circumscribing the triangle ABC.
(ii) Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C. [3]
(b) The following distribution represents the height of 160 students of a school.
| Height (in cm) | 140-145 | 145-150 | 150-155 | 155-160 | 160-165 | 165-170 | 170-175 | 175-180 |
| No. of Students | 12 | 20 | 30 | 38 | 24 | 16 | 12 | 8 |
Draw an ogive for the given distribution taking 2 cm = 5 cm of height on one axis and 2 cm = 20 students on the other axis. Using the graph, determine:
(i) The median height.
(ii) The interquartile range.
(iii) The number of students whose height is above 172 cm. [6]
Solution:
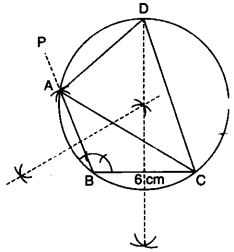
(a) (i) Steps of constructions:
(1) Draw a line segment BC = 6 cm.
(2) Construct ∠CBP = 120°.
(3) Cut BA = 5.5 cm from BP.
(4) Join A to C.
(5) Construct perpendicular bisectors of AB and BC, intersecting at O. Join AO.
(6) Taking as the centre and OA as radius draw a circle, passing through, A, B, and C.
(ii) (1) Extend the right bisector of BC intersecting the circle at D.
(2) Join A to D and C to D.
(3) ABCD is required cyclic
(b)
Question 11:
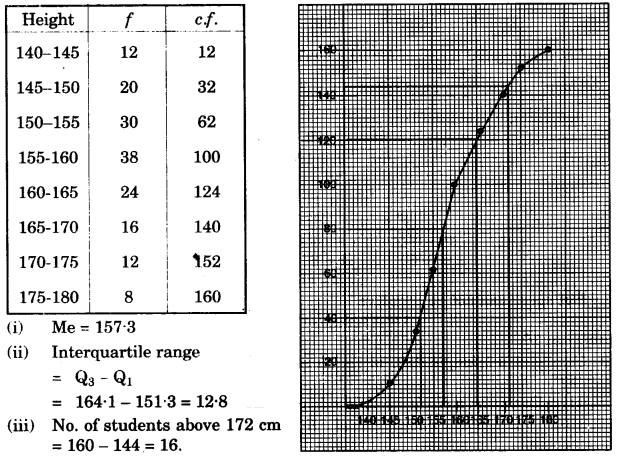
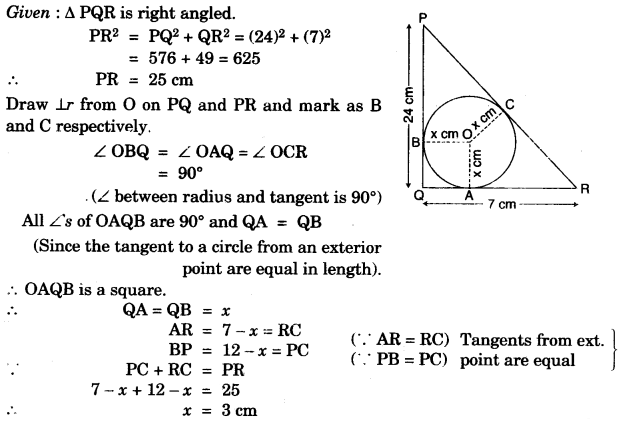
(a) In triangle PQR, PQ = 24 cm, QR = 7 cm and ∠PQR = 90°. Find the radius of the inscribed circle. [3]
(b) Find the mode and median of the following frequency distribution: [3]
| x | 10 | 11 | 12 | 13 | 14 | 15 |
| f | 1 | 4 | 7 | 5 | 9 | 3 |
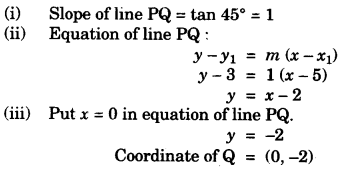
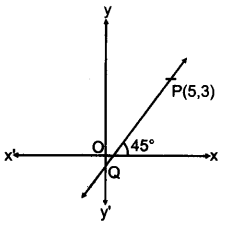
(c) The line through P(5, 3) intersects y axis at Q.
(i) Write the slope of the line.
(ii) Write the equation of the line.
(iii) Find the co-ordinates of Q. [4]
Solution:
(a)
(b) Mode is the value of the highest frequency.
∴ Mode = 14
For Median, we write the data in ascending order,
10, 11, 11, 11, 11, 12, 12, 12, 12, 12, 12, 12, 13, 13, 13, 13, 13, 14, 14, 14, 14, 14, 14, 14, 14, 14, 15, 15, 15.
∵ Median is the middle most value.

(c)