ICSE Maths Previous Year Question Paper 2009 Solved for Class 10
ICSE Paper 2009
MATHEMATICS
(Two hours and a half)
Answers to this Paper must be written on the paper provided separately.
You will not be allowed to write during the first 15 minutes.
This time is to be spent in reading the question paper.
The time given at the head of this Paper is the time allowed for writing the answers.
Attempt all questions from Section A and any four questions from Section B.
All working, including rough work, must be clearly shown and must be done on the same sheet as the rest of the answer. Omission of essential working will result in the loss of marks.
The intended marks for questions or parts of questions are given in brackets [ ].
Mathematical tables are provided.
SECTION-A (40 Marks)
(Attempt all questions from this Section)
Question 1:
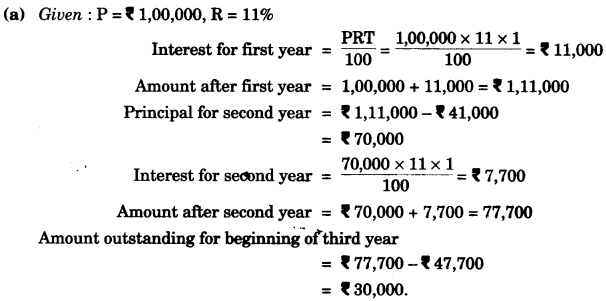
(a) Mr. Dubey borrows Rs. 1,00,000 from State Bank of India at 11% per annum compound interest. He repays Rs. 41,000 at the end of the first year and Rs. 47,700 at the end of the second year. Find the amount outstanding at the beginning of the third year. [3]
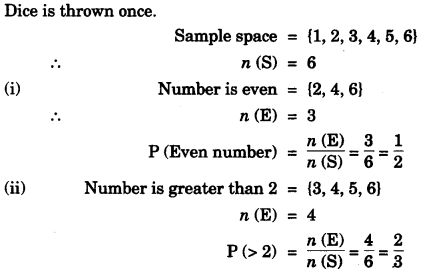
(b) A dice is thrown once. What is the probability that the
(i) number is even
(ii) number is greater than 2? [3]
(c) Find the HCF and LCM of the following polynomials: **
3x3 – 27x2 + 60x and x2 – 16 [4]
** Solution has not given due to out of present syllabus.
Solution:
(a)
(b)
Question 2:
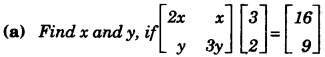
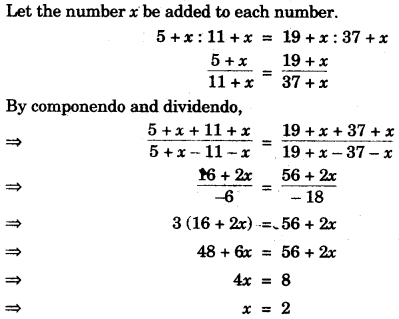
(b) What least number must be added to each of the numbers 5, 11,19 and 37 so that they are in proportion? [3]
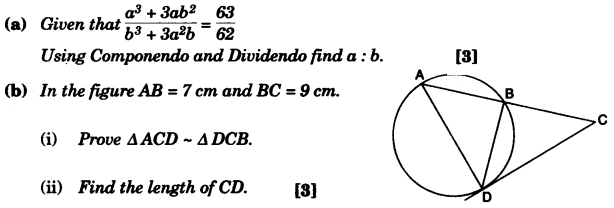
(c) Given that x + 2 and x + 3 are factors of 2x3 + ax2 + 7x – b. Determine the values of a and b. [4]
Solution:
(a)
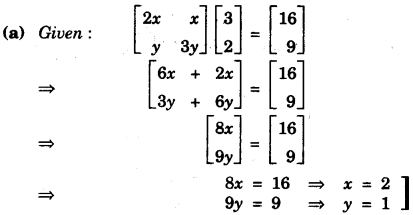
(b)
(c)
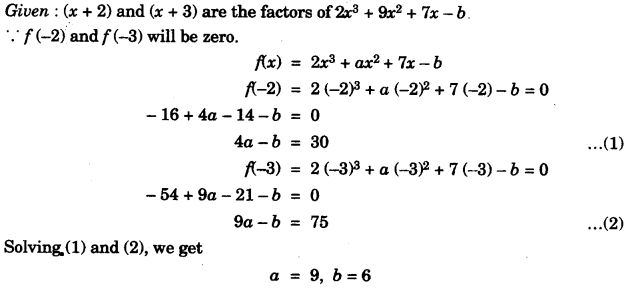
Question 3:
(a) Solve the inequation and represent the solution set on the number line.
![]()
(b) Find the value of p for which the lines
2x + 3y – 7 = 0 and. 4y – px – 12 = 0 are perpendicular to each other. [3]
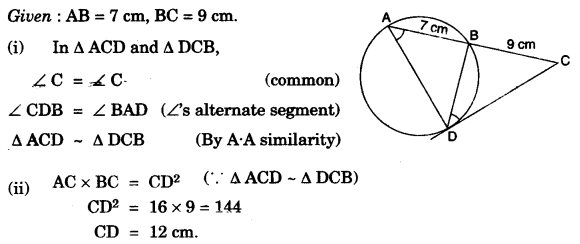
(c) In the given figure O is the centre of the circle, ∠BAD = 75° and chord BC = chord CD. Find: (i) ∠BOC (ii) ∠OBD (iii) ∠BCD. [4]
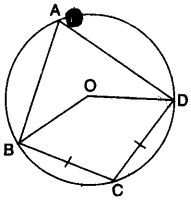
Solution:
(a)
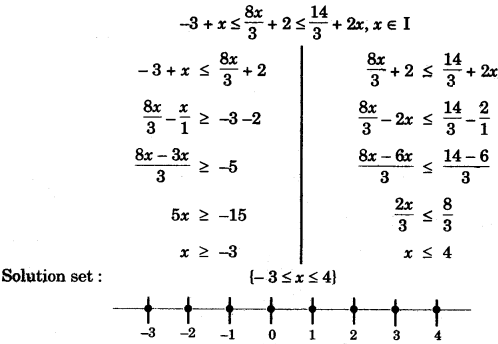
(b)
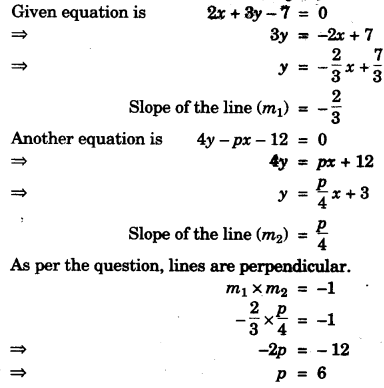
(c)
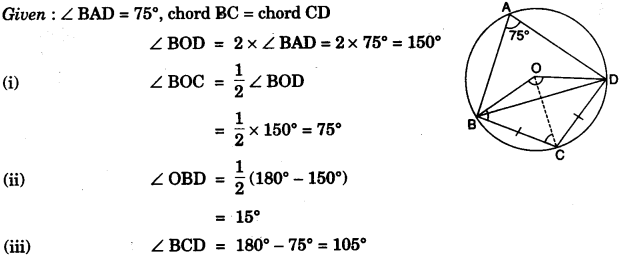
Question 4:
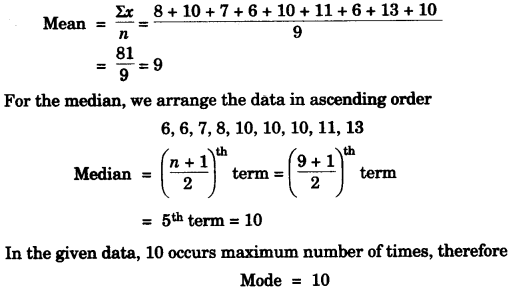
(a) Find the mean, median and mode of the following distribution:
8, 10, 7, 6, 10, 11, 6, 13, 10. [3]
(b) Without using trigonometric tables evaluate the following:
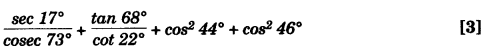
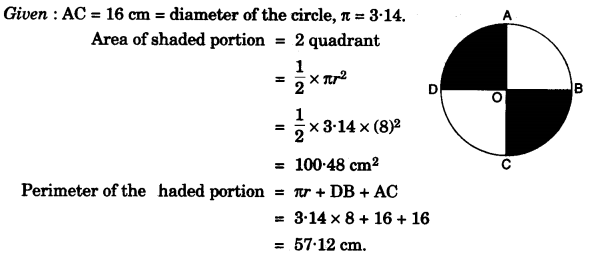
(c) AC and BD are two perpendicular diameters of a circle with centre O. If AC = 16 cm, calculate the area and perimeter of the shaded part. (Take π = 3.14) [4]
Solution:
(a)
(b)
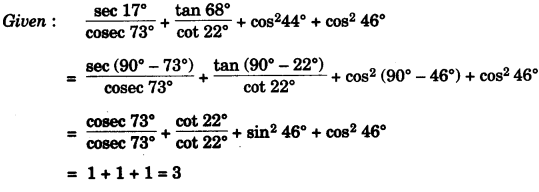
(c)
SECTION-B (40 Marks)
(Attempt any four questions from this Section)
Question 5:
(a) A shopkeeper bought a TV at a discount of 30% of the listed price of Rs. 24,000. The shopkeeper offers a discount of 10% of the listed price to his customer. If the VAT (Value Added Tax) is 10%.
Find: (i) the amount paid by the customer.
(ii) the VAT to be paid by the shopkeeper. [3]
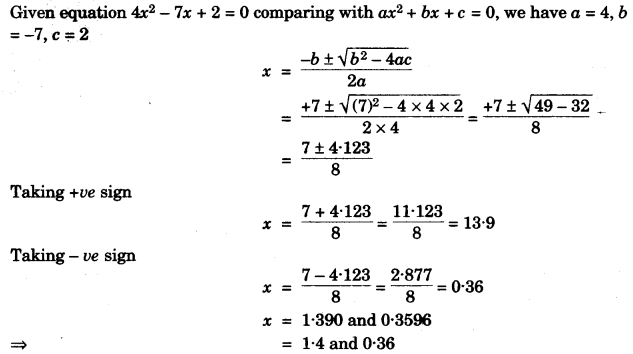
(b) Solve the following quadratic equation and give the answer correct to two significant figures.
4x2 – 7x + 2 = 0 [3]
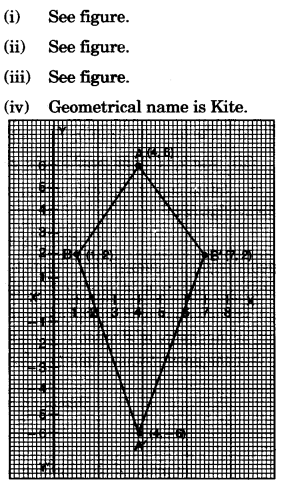
(c) Use graph paper to answer this question.
(i) Plot the points A (4, 6) and B (1, 2).
(ii) A’ is the image of A when reflected in X-axis.
(iii) B’ is the image of B when B is reflected in the line AA’.
(iv) Give the geometrical name for the figure AB A’B’. [4]
Solution:
(a)
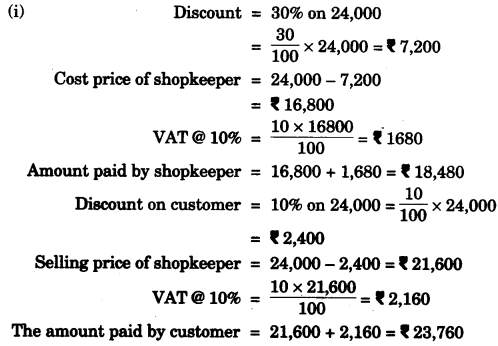
(ii) VAT to be paid by shopkeeper = ₹ 2,160 – ₹ 1,680 = ₹ 480
(b)
(c)
Question 6:

(b) Prove the following identity :
![]()
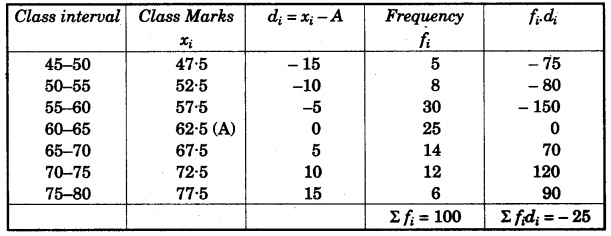
(c) The following table gives the wages of workers in a factory:
| Wages in Rs. | 45-50 | 50-55 | 55-60 | 60-65 | 65-70 | 70-75 | 75-80 |
| No. of workers | 5 | 8 | 30 | 25 | 14 | 12 | 6 |
Calculate the mean by the short cut method. [4]
Solution:
(a)
(b)
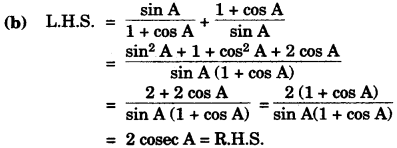
(c)
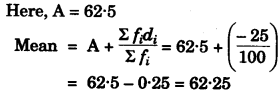
Question 7:
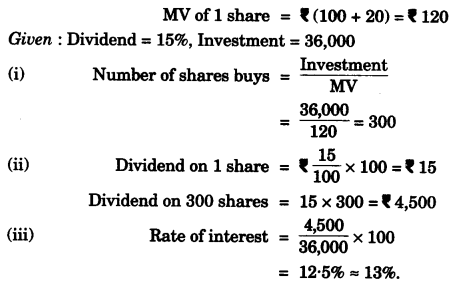
(a) Amit Kumar invests Rs. 36,000 in buying Rs. 100 shares at Rs. 20 premium. The dividend is 15% per annum. Find:
(i) The number of shares he buys
(ii) His yearly dividend
(iii) The percentage return on his investment.
Give your answer correct to the nearest whole number. [3]
(b) What sum of money will amount to Rs. 9,261 in 3 years at 5% per annum compound interest? [3]
(c) Mr. Mishra has a Savings Bank Account in Allahabad Bank. His pass book entries are as follows:
| Date | Particulars | Withdrawals (in Rs.) | Deposits (in Rs.) | Balance (in Rs.) |
| Jan. 4, 2007 | By Cash | — | 1000.00 | 1000.00 |
| Jan. 11, 2007 | By Cheque | — | 3000.00 | 4000.00 |
| Feb. 3, 2007 | By Cash | — | 2500.00 | 6500.00 |
| Feb. 7, 2007 | To Cheque | 2000.00 | — | 4500.00 |
| March 3, 2007 | By Cash | — | 5000.00 | 9500.00 |
| March 25, 2007 | By Cash | — | 2000.00 | 11,500.00 |
| June 7, 2007 | By Cash | — | 3500.00 | 15000.00 |
| Aug. 29, 2007 | To Cheque | 1000.00 | — | 14000.00 |
Rate of interest paid by the bank is 4.5% per annum. Mr. Mishra closes his account on 30th October, 2007. Find the interest he receives. [4]
Solution:
(a)
(b)
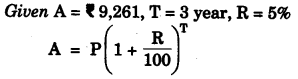
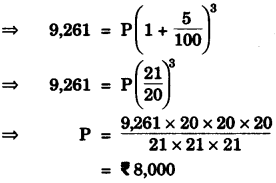
(c)
Question 8:
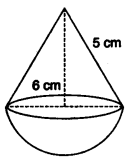
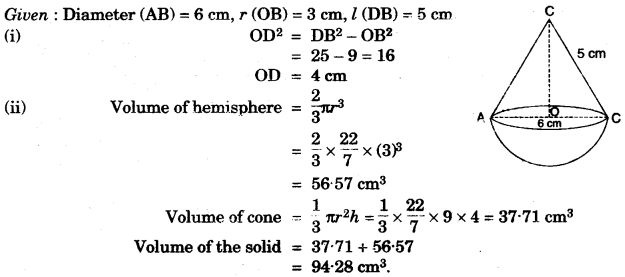
(c) The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6 cm each and the slant height of the cone is 5 cm. Calculate:
(i) the height of the cone.
(ii) the volume of the solid. [4]
Solution:
(a)
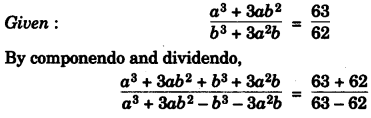
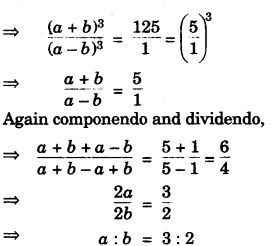
(b)
(c)
Question 9:
(a) Attempt this question on graph paper.
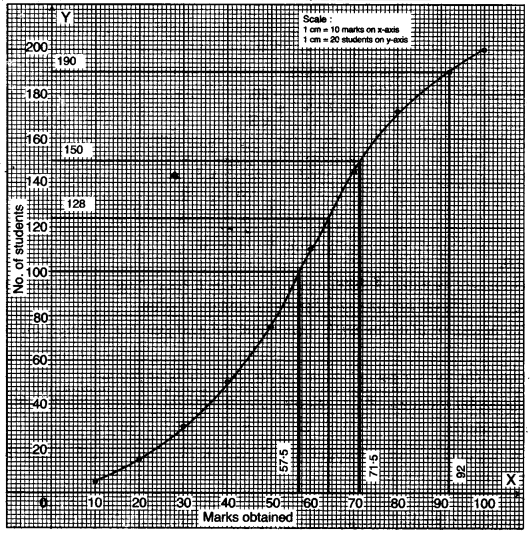
Marks obtained by 200 students in examination are given below:
| Marks | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 | 90-100 |
| No. of Students | 5 | 10 | 14 | 21 | 25 | 34 | 36 | 27 | 16 | 12 |
Draw an Ogive for the given distribution taking 2 cm = 10 marks on one axis and 2 cm = 20 students on the other axis.
From the graph find:
(i) the Median
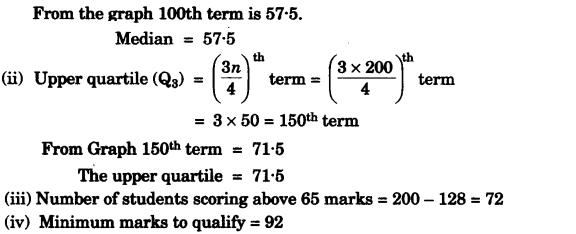
(ii) the Upper Quartile
(iii) Number of students scoring above 65 marks.
(iv) If 10 students qualify for merit scholarship, find the minimum marks required to qualify. [6]
(b) From two points A and B on the same side of a building, the angles of elevation of the top of the building are 30° and 60° respectively. If the height of the building is 10m, find the distance between A and B correct to two decimal places. [4]
Solution:
(a)
| Marks | No. of students | cf |
| 0-10 | 5 | 5 |
| 10-20 | 10 | 15 |
| 20-30 | 14 | 29 |
| 30-40 | 21 | 50 |
| 40-50 | 25 | 75 |
| 50-60 | 34 | 109 |
| 60-70 | 36 | 145 |
| 70-80 | 27 | 172 |
| 80-90 | 16 | 188 |
| 90-100 | 12 | 200 |
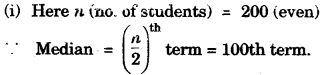
(b)

Question 10:
(a) Mrs. Goswami deposits Rs. 1000 every month in a recurring deposit account for 3 years at 8% interest per annum. Find the matured value. [3]
(b) Find the equation of a line with x intercept = 5 and passing through the point (4, -7). [3]
(c) In a school the weekly pocket money of 50 students is as follows:
| Weekly pocket money in Rs. | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 | 90-100 |
| No. of students | 2 | 8 | 12 | 14 | 8 | 6 |
Draw a histogram and a frequency polygon on the same graph. Find the mode from the graph. [4]
Solution:
(a)
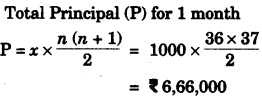
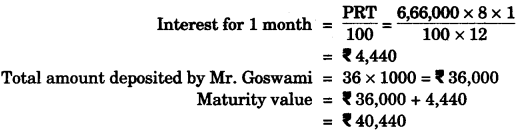
(b)
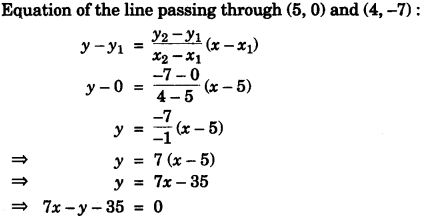
(c)
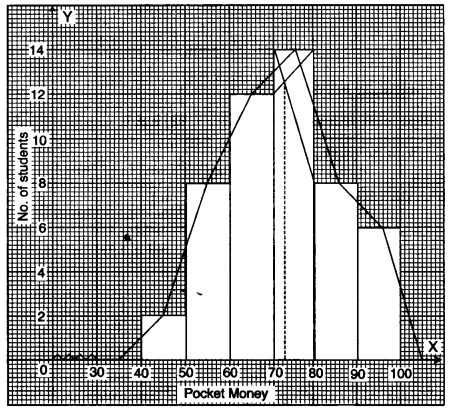
So, from the graph, Mode = 73
Question 11:
(a) The model of a building is constructed with scale factor 1 : 30.
(i) If the height of the model is 80 cm, find the actual height of the building in metres.
(ii) If the actual volume of a tank at the top of the building is 27m3, find the volume of the tank on the top of the model. [2]
(b) The speed of an express train is x km/h and the speed of an ordinary train is 12 km/h less than that of the express train. If the ordinary train takes one hour longer than the express train to cover a distance of 240 km, find the speed of the express train. [4]
(c) Using ruler and compasses construct
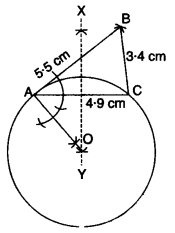
(i) a triangle ABC in which AB = 5.5 cm, BC = 3.4 cm and CA = 4.9 cm.
(ii) the locus of points equidistant from A and C.
(iii) a circle touching AB at A and passing through C. [4]
Solution:
(a)
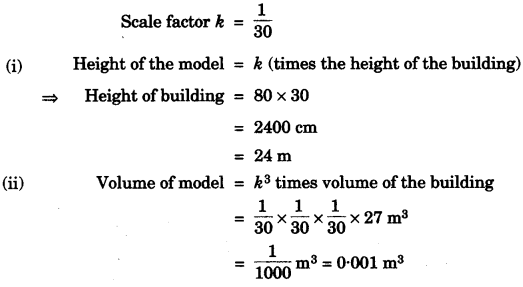
(b)

(c) Steps of construction:
1. Draw ΔABC with given values.
2. Draw XY perpendicular bisector of AC.
3. Draw perpendicular of AB at A which cuts perpendicular XY at O.
4. Draw a circle at centre O which touching AB at A and passing through C i.e., required circle.