Geometric Mean

Geometric Mean

If a, G, b are in G.P., then G is called Geometric Mean (G.M.) between a and b.

1. If a, G₁, G₂, G₃, …. G_n, b are in G.P. then G₁, G₂, G₃, …. G_n are called n G.M.’s between a and b.
2. Insertion of geometric means:
   1. Single G.M. between a and b: If a and b are two real numbers then single G.M. between a and \(b=\sqrt { ab }\).
   2. n G.M.’s between a and b: If G₁, G₂, G₃, …. G_n are n G.M.’s between a and b, then
      

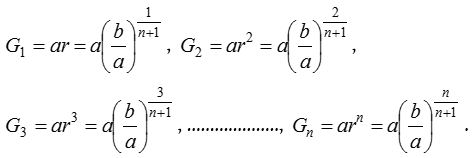
Properties of G.P.

1. If all the terms of a G.P. be multiplied or divided by the same non-zero constant, then it remains a G.P., with the same common ratio.
2. The reciprocal of the terms of a given G.P. form a G.P. with common ratio as reciprocal of the common ratio of the original G.P.
3. If each term of a G.P. with common ratio r be raised to the same power k, the resulting sequence also forms a G.P. with common ratio r^k.
4. In a finite G.P., the product of terms equidistant from the beginning and the end is always the same and is equal to the product of the first and last term. i.e., if a₁, a₂, a₃, …… a_n be in G.P.
   Then a₁a_n = a₂a_n-1 = a₃a_n-2 = a₄a_n-3 = a_r.a_n-r+1
5. If the terms of a given G.P. are chosen at regular intervals, then the new sequence so formed also forms a G.P.
6. If a₁, a₂, a₃, …… a_n is a G.P. of non-zero, non-negative terms, then log a₁, log a₂, log a₃, …… log a_n is an A.P. and vice-versa.
7. Three non-zero numbers a, b, c are in G.P., iff b² = ac.
8. If first term of a G.P. of n terms is a and last term is l, then the product of all terms of the G.P. is (al)^n/2.
9. If there be n quantities in G.P. whose common ratio is r and S_m denotes the sum of the first m terms, then the sum of their product taken two by two is \(\frac { r }{ r+1 } { S }_{ n }{ S }_{ n-1 }\).
10. If a^x1, a^x2, a^x3, ……, a^xn are in G.P., then x₁, x₂, x₃ …… x_n will be are in A.P.

Geometric Mean Problems with Solutions

1. If be the geometric mean of x and y, then

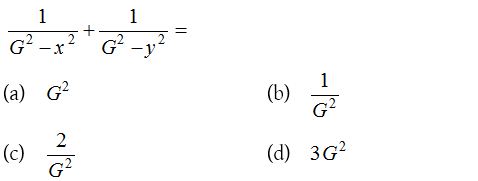
Solution:

2. If three geometric means be inserted between 2 and 32, then the third geometric mean will be
(a) 8
(b) 4
(c) 16
(d) 12
Solution:

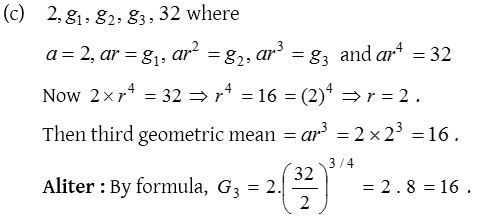
3. If five G.M.’s are inserted between 486 and 2/3 then fourth G.M. will be
(a) 4
(b) 6
(c) 12
(d) – 6
Solution:

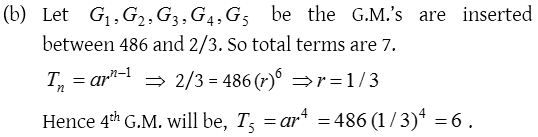
4. The G.M. of roots of the equation x² – 18x + 9 = 0 is
(a) 3
(b) 4
(c) 2
(d) 1
Solution:

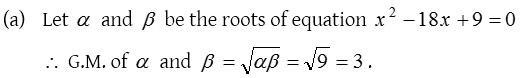
5. The G.M. of the numbers 3, 3², 3³, …. 3ⁿ is

Solution:

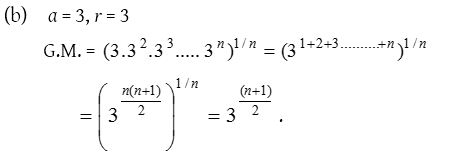
6. The product of three geometric means between 4 and 1/4 will be
(a) 4
(b) 2
(c) -1
(d) 1
Solution:

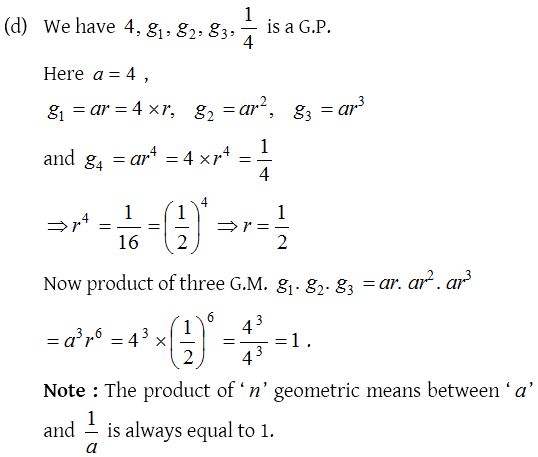
7. The two geometric means between the number 1 and 64 are
(a) 1 and 64
(b) 4 and 16
(c) 2 and 16
(d) 8 and 16
Solution:
(b) Let 1,a, b, 64  ⇒ a² = b and b² = 64a ⇒ a = 4 and b = 16.